How are integer and floating-point types stored in memory

Catalog

1 data type

1.1 Basic classification of types ：

2 Shaping storage in memory

2.1 Three forms of binary

2.2 Introduction of big and small endings

 3 Floating point storage in memory

3.1 Floating point storage rules

Preface

Hello, everyone ！ In this issue, I will share with you how integer and floating-point data are stored in memory .

1 data type

We already know the basic built-in types ：

The meaning of type ：

1. Use this type to exploit the size of memory space （ Size determines the range of use ）.

2. How to look at the perspective of memory space .

1.1 Basic classification of types ：

Plastic surgery Family ：

char

     unsigned char

     signed char

short

     unsigned short [int]

     signed short [int]

int

    unsigned int

    signed int

long

    unsigned long [int]

    signed long [int]

 char Depending on the compiler , stay VS In the compiler char yes signed char.

Floating point family ：

float  

double

Among them float Low type accuracy , Small storage range .

and double High type accuracy , Large storage range . 

Construction type ： 

> An array type

> Type of structure struct

> Enumeration type enum

> Joint type union

Pointer types

int *pi;

char *pc;

float* pf;

void* pv;

Empty type ： 

void Indicates empty type （ No type ）

Usually applied to the return type of a function 、 The parameters of the function 、 Pointer types .

What I want to share with you today is Plastic surgery Family and Floating point family How is it stored in memory .

2 Shaping storage in memory

2.1 Three forms of binary

Original code ： Express directly in binary .

Inverse code ： The sign bit of the original code remains unchanged , Other bits are reversed .

Complement code ： Inverse code +1.

Be careful ：

1 The original code of positive number 、 The inverse code is the same as the complement code . The binary transformation rules of negative numbers are as follows .

2 There are two ways to change the complement code into the original code ：

Method 1

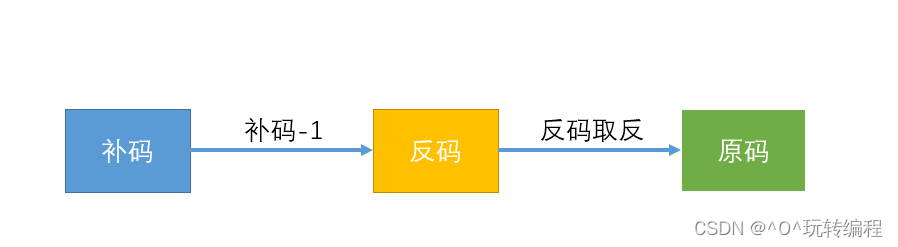
  Method 2

  So what form of binary is plastic stored in memory ？

In fact, shaping is stored in memory in the form of complement .

In computer system , All values are represented and stored by complements . The reason lies in , Use complement , You can combine sign bits and numeric fields One processing ;

meanwhile , Addition and subtraction can also be handled in a unified way （CPU Only adders ） Besides , Complement code and original code are converted to each other , Its operation process It's the same , No need for additional hardware circuits .

  What is this ?

Actually, I just learned here , I'm also a little confused , Why use complement to save ？ Let me give you an example

1-1 How is the result of this operation carried out

1 The original code of （ stay 32 A platform ）：00000000000000000000000000000001

-1 The original code of （ stay 32 A platform ）：10000000000000000000000000000001

-1 The inverse of （ stay 32 A platform ）：111111111111111111111111111111111110

-1 Complement （ stay 32 A platform ）：111111111111111111111111111111111111

because CPU Only adders , therefore 1-1---->1+（-1）

Obviously, whether it is 1 The original code of is still the inverse code and -1 The result cannot be obtained by adding the original code or complement of .

And the complement can 100000000000000000000000000000000（ here 33）, And we are 32 Bit platform , So the end result is 00000000000000000000000000000000（0, Is it amazing to see Jue's complement here . therefore ： Integers store complements in memory .

  Although we know that what is stored in memory is a complement , But we still don't know how the complement is stored in memory , Next we will talk about the big and small endings .

2.2 Introduction of big and small endings

Let's use integer 1 To understand the

Big end byte order storage

Is to put a data High byte order The content of is in Low address , Low byte order The content of is High address .

  We use it 16 Hexadecimal represents distribution

Small end byte order storage  

  Is to put a data High byte order The content of is in High address , Low byte order The content of is Low address .

  The following is the vs Storage distribution in

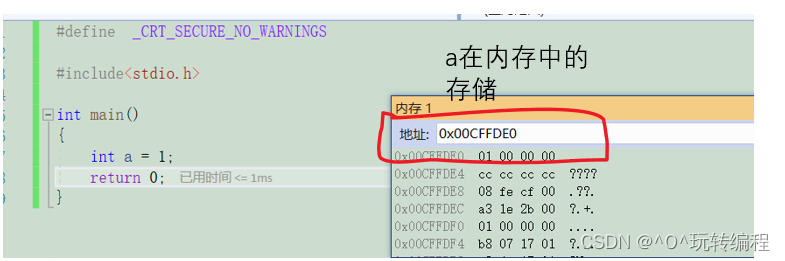
The storage of byte order at the big and small end is related to hardware , Some hardware is small end storage , There is big end storage .

 3 Floating point storage in memory

Many people will think , Shaping is stored in memory in the form of complement , The storage mode depends on the size , So how is floating-point type stored ？

From the above, we may see that it is definitely not related to the storage method of shaping .

3.1 Floating point storage rules

According to international standards IEEE（ Institute of electrical and Electronic Engineering ） 754, Any binary floating point number V It can be expressed in the following form ：

(-1)^S * M * 2^E

(-1)^S The sign bit , When S=0,V Is a positive number ; When S=1,V It's a negative number .

M Represents a significant number , Greater than or equal to 1, Less than 2.

2^E Indicates the index bit .

that 1.0 I can write this as .(-1)^0* 1.0*2^0（2^n Power is the form of binary scientific calculation ）

Two of them S = 0,M = 1.0,E = 0

that 9.5 We can write

（-1）^0*1.0011*2^3

and 9.6 Well ？

We find that we can never show 0.6, Can only keep approaching this number , So the storage of floating-point in memory is a The exact value Instead of a Accurate value .

IEEE 754 Regulations ：

about 32 Floating point number of bits , The highest 1 Bits are sign bits s, And then 8 Bits are exponents E, The rest 23 Bits are significant numbers M.

about 64 Floating point number of bits , The highest 1 Bits are sign bits S, And then 11 Bits are exponents E, The rest 52 Bits are significant numbers M.

IEEE 754 For significant figures M And the index E, There are some special rules . 

As I said before , 1≤M<2 , in other words ,M It can be written. 1.xxxxxx In the form of , among xxxxxx Represents the fractional part . IEEE 754 Regulations , Keep it in the computer M when , By default, the first digit of this number is always 1, So it can be Give up , Save only the back xxxxxx part . For example preservation 1.01 When the Hou , Save only 01, Wait until you read , Put the first 1 Add . The purpose of this , It's saving 1 Significant digits . With 32 position Floating point numbers, for example , Leave to M Only 23 position , Will come first 1 After giving up , It's equivalent to being able to save 24 Significant digits .

As for the index E, The situation is more complicated .

First ,E For an unsigned integer （unsigned int） 

It means , If E by 8 position , Its value range is 0~255; If E by 11 position , Its value range is 0~2047. however , We know , In scientific counting E You can have negative numbers , therefore IEEE 754 Regulations , In memory E The true value of must be added with an intermediate number , about 8 Bit E, This middle number yes 127; about 11 Bit E, In the middle The number is 1023. such as ,2^10 Of E yes 10, So save it as 32 When floating-point numbers are in place , Must be saved as 10+127=137, namely 10001001.

  Index E Fetching from memory can be further divided into three cases ：

E Not all for 0 Or not all of them 1

At this time , Floating point numbers are represented by the following rules , The index E Of Subtract... From the calculated value 127（ or 1023）, obtain True value , then Significant figures M Add the first 1. such as ： 0.5（1/2） The binary form of is 0.1, Since it is stipulated that the positive part must be 1, That is to move the decimal point to the right 1 position , Then for 1.0*2^(-1), Its order code is -1+127=126, Expressed as 01111110, And the mantissa 1.0 Remove the integer part and make it 0, A filling 0 To 23 position 00000000000000000000000, Then its binary representation is :0 01111110 00000000000000000000000

 E All for 0 

At this time , The exponent of a floating point number E be equal to 1-127（ perhaps 1-1023） That's the true value , Significant figures M No more first 1, It's reduced to 0.xxxxxx Decimals of . This is to show that ±0, And close to 0 A very small number of .

E All for 1         

At this time , If the significant number M All for 0, Express ± infinity （ It depends on the sign bit s）;

Okay , How many floating-point rules are introduced .

Conclusion

Just to summarize , The storage of shaping is through In the form of complement （ Through the form of big and small ends ), Floating point storage mainly stores A sign bit E Binary bit level of M The exact number of digits . If you get something , Let's support San Lian ！