当前位置:网站首页>Add Modulo 10 (规律循环节,代码实现细节)
Add Modulo 10 (规律循环节,代码实现细节)
2022-08-03 08:11:00 【lovesickman】
Add Modulo 10 (规律循环节,代码实现细节)
题目描述
You are given an array of $ n $ integers $ a_1, a_2, \dots, a_n $
You can apply the following operation an arbitrary number of times:
- select an index $ i $ ( $ 1 \le i \le n $ ) and replace the value of the element $ a_i $ with the value $ a_i + (a_i \bmod 10) $ , where $ a_i \bmod 10 $ is the remainder of the integer dividing $ a_i $ by $ 10 $ .
For a single index (value $ i $ ), this operation can be applied multiple times. If the operation is applied repeatedly to the same index, then the current value of $ a_i $ is taken into account each time. For example, if $ a_i=47 $ then after the first operation we get $ a_i=47+7=54 $ , and after the second operation we get $ a_i=54+4=58 $ .
Check if it is possible to make all array elements equal by applying multiple (possibly zero) operations.
For example, you have an array $ [6, 11] $ .
- Let’s apply this operation to the first element of the array. Let’s replace $ a_1 = 6 $ with $ a_1 + (a_1 \bmod 10) = 6 + (6 \bmod 10) = 6 + 6 = 12 $ . We get the array $ [12, 11] $ .
- Then apply this operation to the second element of the array. Let’s replace $ a_2 = 11 $ with $ a_2 + (a_2 \bmod 10) = 11 + (11 \bmod 10) = 11 + 1 = 12 $ . We get the array $ [12, 12] $ .
Thus, by applying $ 2 $ operations, you can make all elements of an array equal.
输入格式
The first line contains one integer $ t $ ( $ 1 \le t \le 10^4 $ ) — the number of test cases. What follows is a description of each test case.
The first line of each test case contains one integer $ n $ ( $ 1 \le n \le 2 \cdot 10^5 $ ) — the size of the array.
The second line of each test case contains $ n $ integers $ a_i $ ( $ 0 \le a_i \le 10^9 $ ) — array elements.
It is guaranteed that the sum of $ n $ over all test cases does not exceed $ 2 \cdot 10^5 $ .
输出格式
For each test case print:
- YES if it is possible to make all array elements equal;
- NO otherwise.
You can print YES and NO in any case (for example, the strings yEs, yes, Yes and YES will be recognized as a positive answer) .
样例 #1
样例输入 #1
10
2
6 11
3
2 18 22
5
5 10 5 10 5
4
1 2 4 8
2
4 5
3
93 96 102
2
40 6
2
50 30
2
22 44
2
1 5
样例输出 #1
Yes
No
Yes
Yes
No
Yes
No
No
Yes
No
提示
The first test case is clarified above.
In the second test case, it is impossible to make all array elements equal.
In the third test case, you need to apply this operation once to all elements equal to $ 5 $ .
In the fourth test case, you need to apply this operation to all elements until they become equal to $ 8 $ .
In the fifth test case, it is impossible to make all array elements equal.
In the sixth test case, you need to apply this operation to all elements until they become equal to $ 102 $ .
打表找循环节。
我的:
/* 1 2 4 8 16 3 6 12 14 18 */
void solve(){
cin>>n;
set<int>st;
bool ok = 1;
bool A = 0;// 1
bool B = 0;// 3
bool C = 0;// 5
fo(i,1,n){
int x;cin>>x;
int t = x+x%10;
if(t%5==0){
if(!C){
C=1;
st.insert(t);
}
else{
if(st.find(t)!=st.end());
else ok = 0;
}
}
t %= 20;
if(t == 1 || t == 2 || t == 4 || t == 8 || t == 16){
if(!A){
A=1;
st.insert(1);
}
}
if(t == 3 || t == 6 || t == 12 || t == 14 || t == 18){
if(!B){
B=1;
st.insert(3);
}
}
}
if(st.size()!=1){
ok = 0;
}
cout<<(ok ? "YES" : "NO")<<endl;
}
#include <bits/stdc++.h>
using namespace std;
typedef long long LL;
const int INF = 0x3f3f3f3f;
const LL mod = 1e9 + 7;
const int N = 300005;
int a[N];
int main() {
int _;
scanf("%d", &_);
while (_--) {
int n;
scanf("%d", &n);
vector<int> b;
set<int> c;
for (int i = 1; i <= n; i++) {
scanf("%d", &a[i]);
if (a[i] % 10 == 5)
b.push_back(a[i] + 5);
else if (a[i] % 10 == 0)
b.push_back(a[i]);
else {
int x = a[i];
while (x % 10 != 6) {
x += x % 10;
}
c.insert(x % 20);
}
}
if (b.size() > 0) {
int ok = b.size() == n;
for (auto x : b)
if (x != b[0]) ok = 0;
puts(ok ? "YES" : "NO");
} else {
puts(c.size() == 1 ? "YES" : "NO");
}
}
return 0;
}
/** * author: tourist * created: 01.08.2022 17:43:24 **/
#include <bits/stdc++.h>
using namespace std;
#ifdef LOCAL
#include "algo/debug.h"
#else
#define debug(...) 42
#endif
int main() {
ios::sync_with_stdio(false);
cin.tie(0);
int tt;
cin >> tt;
while (tt--) {
int n;
cin >> n;
vector<int> a(n);
bool any = false;
for (int i = 0; i < n; i++) {
cin >> a[i];
while (a[i] % 10 != 0 && a[i] % 10 != 2) {
a[i] += a[i] % 10;
}
any |= (a[i] % 10 == 0);
}
if (any) {
cout << (a == vector<int>(n, a[0]) ? "Yes" : "No") << '\n';
continue;
}
int val = a[0] % 20;
bool ok = true;
for (int i = 0; i < n; i++) {
ok &= (a[i] % 20 == val);
}
cout << (ok ? "Yes" : "No") << '\n';
}
return 0;
}
边栏推荐
猜你喜欢
随机推荐
进程的创建
ceph简介
Gauva的ListenableFuture
How does Mysql query two data tables for the same fields in two tables at the same time
unity的game界面里有canvas的线框?如何隐藏掉?
如何使用电子邮件营销在五个步骤中增加产品评论
二进制日志过期时间设置expire_logs_days
ArcEngine (six) use the tool tool to realize the zoom in, zoom out and translation of the pull box
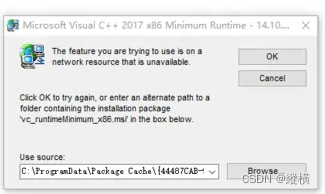
vs 2022无法安装 vc_runtimeMinmum_x86错误
ArcEngine (5) use the ICommand interface to achieve zoom in and zoom out
swiper分类菜单双层效果demo(整理)
行业洞察 | 如何更好的实现与虚拟人的互动体验?
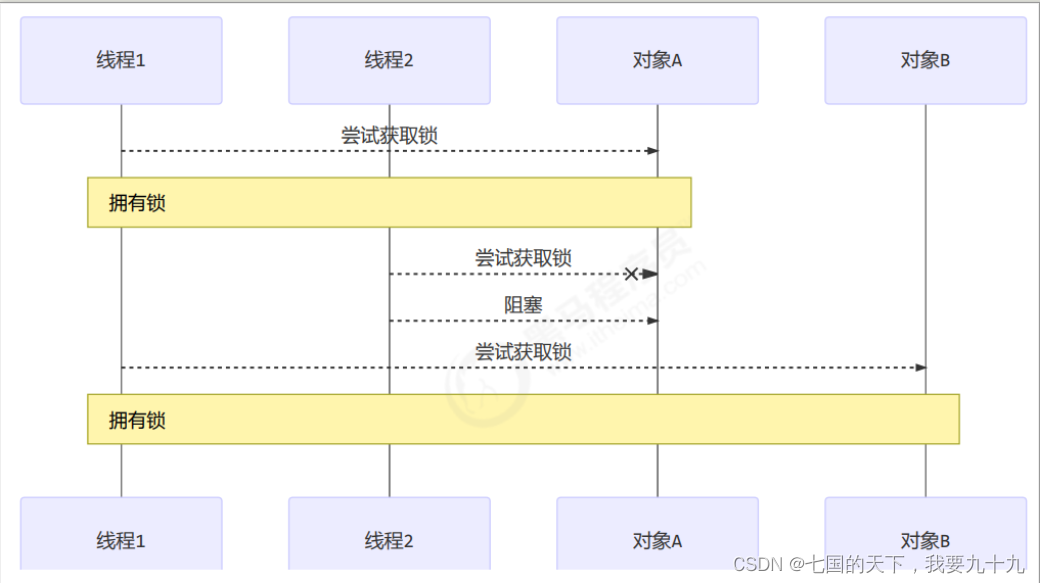
Redis分布式锁
IDEA的database使用教程(使用mysql数据库)
IDEA2021.2安装与配置(持续更新)
ArcEngine (3) zoom in and zoom out through the MapControl control to achieve full-image roaming
进程信息
《剑指Offer》刷题之打印从1到最大的n位数
ArcEngine(六)用tool工具实现拉框放大缩小和平移
requests库