当前位置:网站首页>2022杭电多校 第6场 1008.Shinobu Loves Segment Tree 规律题
2022杭电多校 第6场 1008.Shinobu Loves Segment Tree 规律题
2022-08-05 10:08:00 【HeartFireY】
题目分析
本题解时间复杂度 O ( T log n ) O(T\log n) O(Tlogn),标程 O ( T log 2 n ) O(T \log^2 n) O(Tlog2n)
发现每个节点的按顺序 b u i l d build build产生的加法序列的规律:(若干个 1 1 1)+(相同个数个 2 , 3 , 4... 2,3,4... 2,3,4...)+(若干个相同数字),然后发现加 2 , 3 , 4 , . . . 2,3,4,... 2,3,4,...的次数与层节点个数有关。即为加 2 ⌊ l o g 2 x ⌋ 2^{ {\lfloor log_2{x} \rfloor}} 2⌊log2x⌋次 2 , 3 , 4 , … 2,3,4,\dots 2,3,4,…。
这个规律可以通过 1. 1. 1.分析线段树 b u i l d build build性质 2. 2. 2.暴力打表(牛逼) 发现
那么我们可以写成式子:假设加法序列长度为 s i z siz siz,加 1 1 1的个数为 c n t 1 cnt_1 cnt1,那么该节点 r t rt rt的加和可以表示为:
c n t 1 + ( ( ( s i z − c n t 1 ) ( 2 ⌊ l o g 2 x ⌋ ) + 1 ) × ( ( s i z − c n t 1 ) ( 2 ⌊ l o g 2 x ⌋ ) + 2 ) 2 − 1 ) × 2 ⌊ l o g 2 x ⌋ + [ ( s i z − c n t 1 ) m o d ( 2 ⌊ l o g 2 x ⌋ ) ) ] × ( ( s i z − c n t 1 ) ( 2 ⌊ l o g 2 x ⌋ ) + 2 ) cnt_1 + (\frac{(\frac{(siz - cnt1)}{(2^{ {\lfloor log_2{x} \rfloor}})} + 1) \times (\frac{(siz - cnt1)}{(2^{ {\lfloor log_2{x} \rfloor}})} + 2)}{2} - 1) \times 2^{ {\lfloor log_2{x} \rfloor}} + [(siz - cnt_1) \mod (2^{ {\lfloor log_2{x} \rfloor}}))] \times (\frac{(siz - cnt1)}{(2^{ {\lfloor log_2{x} \rfloor}})} + 2) cnt1+(2((2⌊log2x⌋)(siz−cnt1)+1)×((2⌊log2x⌋)(siz−cnt1)+2)−1)×2⌊log2x⌋+[(siz−cnt1)mod(2⌊log2x⌋))]×((2⌊log2x⌋)(siz−cnt1)+2)加的数字个数 s i z siz siz的规律,对于当前节点 r t rt rt:
- ⌈ ( r t − 2 ⌊ l o g 2 r t ⌋ ) / 2 ⌉ m o d 2 = = 1 , s i z − = 2 ⌊ l o g 2 r t ⌋ − 2 \lceil(rt - 2^{\lfloor log_2{rt} \rfloor}) / 2 \rceil \mod2 == 1, siz -= 2^{\lfloor log_2{rt} \rfloor - 2} ⌈(rt−2⌊log2rt⌋)/2⌉mod2==1,siz−=2⌊log2rt⌋−2
- ⌈ ( r t − 2 ⌊ l o g 2 r t ⌋ ) / 2 ⌉ m o d 2 = = 0 , s i z − = 2 ⌊ l o g 2 r t ⌋ − 1 \lceil(rt - 2^{\lfloor log_2{rt} \rfloor}) / 2 \rceil \mod2 == 0, siz -= 2^{\lfloor log_2{rt} \rfloor - 1} ⌈(rt−2⌊log2rt⌋)/2⌉mod2==0,siz−=2⌊log2rt⌋−1
求 s i z siz siz时,可以令 s i z = n siz = n siz=n,然后沿着树上路径(实际上对应数字的二进制位)向上走,然后减去当前节点对应该减的值。
1 1 1的规律:可以 O ( 1 ) O(1) O(1)求:
c n t 1 = { 2 ( ⌊ l o g 2 x ⌋ − 1 ) , ( x − 2 ⌊ l o g 2 x ⌋ + 1 ) m o d 2 = 1 2 ⌊ l o g 2 x ⌋ , ( x − 2 ⌊ l o g 2 x ⌋ + 1 ) m o d 2 = 0 cnt_1 =\left\{\begin{matrix} 2^{({\lfloor log_2{x} \rfloor} - 1)},\ (x - 2^{ {\lfloor log_2{x} \rfloor}} + 1) \mod 2 =1 \\ 2^{ {\lfloor log_2{x} \rfloor}},\ (x - 2^{ {\lfloor log_2{x} \rfloor}} + 1) \mod 2 = 0 \end{matrix}\right. cnt1={ 2(⌊log2x⌋−1), (x−2⌊log2x⌋+1)mod2=12⌊log2x⌋, (x−2⌊log2x⌋+1)mod2=0注意,当 s i z < 0 siz < 0 siz<0时,特判输出 0 0 0,对第一层求 s i z siz siz时也要特判避免溢出。同时对 n = 1 n = 1 n=1的 4 4 4种情况也要特判,避免溢出。
Code
#include <bits/stdc++.h>
#pragma gcc optimize("O2")
#pragma g++ optimize("O2")
#define int long long
#define endl '\n'
using namespace std;
const int N = 2e5 + 10, MOD = 1e9 + 7;
inline void solve(){
int n = 0, x = 0; cin >> n >> x;
if(n == 1 && x == 1){
cout << 1 << endl; return; }
else if(n == 1 && x != 1){
cout << 0 << endl; return; }
if(x == 1){
cout << (n * (n + 1) / 2) << endl; return; }
//cout << "FUCK:" << get_range(x) << endl;
//if(x > get_range(x)){ cout << 0 << endl; return; }
int ceng = log2(x), ceng_fir = (1 << (ceng));
int cnt1 = ((x - ceng_fir + 1) % 2) ? (1 << (ceng - 1)) : ceng_fir;
if((x - ceng_fir + 1) == 0) cnt1 = ceng_fir;
// cnt_1 -> 1的个数
int siz = n;
int rt = x;
while(rt != 2 && rt != 3){
// cout << rt << " BEF | ";
int ceng_now = log2(rt) + 1, ser = rt - (1 << (ceng_now - 1)) + 1;
int md = ser % 4;
if(md == 1 || md == 2) siz -= (1 << (ceng_now - 3));
if(md == 3 || md == 0) siz -= (1 << (ceng_now - 2));
rt >>= 1;
// cout << rt << " AFT " << endl;
// cout << siz << endl;
}
if(rt != 1) siz -= 1;
if(siz <= 0){
cout << 0 << endl;
return;
}
if(siz <= cnt1){
cout << siz << endl;
return;
}
int yu = (siz - cnt1) % (ceng_fir), m = (siz - cnt1) / (ceng_fir);
int ans = yu * (m + 2) + cnt1 + ceng_fir * ((m + 1) * (m + 2) / 2 - 1);
cout << ans << endl;
}
signed main(){
ios_base::sync_with_stdio(false), cin.tie(0);
cout << fixed << setprecision(12);
int t = 1; cin >> t;
while(t--) solve();
return 0;
}
边栏推荐
- 自定义过滤器和拦截器实现ThreadLocal线程封闭
- Tanabata romantic date without overtime, RPA robot helps you get the job done
- QSS 选择器
- The century-old Nordic luxury home appliance brand ASKO smart wine cabinet in the three-temperature area presents the Chinese Valentine’s Day, and tastes the love of the delicacy
- matcher中find,matches,lookingAt匹配字符串的不同之处说明
- LeetCode 216. Combined Sum III (2022.08.04)
- What is CRM Decision Analysis Management?
- Voice-based social software development - making the most of its value
- 无题六
- Confessing in the era of digital transformation: Mai Cong Software allows enterprises to use data in the easiest way
猜你喜欢
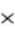
2022华数杯数学建模A题环形振荡器的优化设计思路思路代码分享
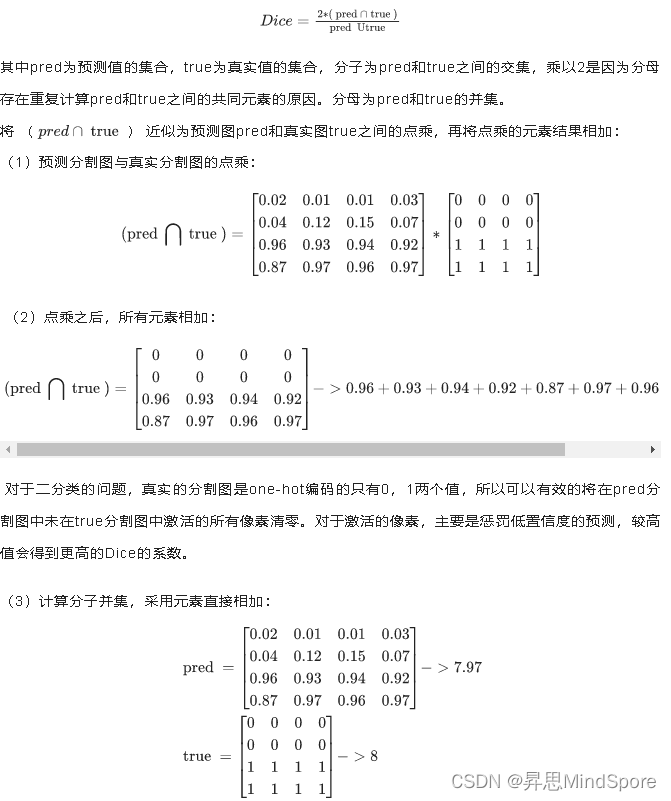
Complete image segmentation efficiently based on MindSpore and realize Dice!
Huawei's lightweight neural network architecture GhostNet has been upgraded again, and G-GhostNet (IJCV22) has shown its talents on the GPU
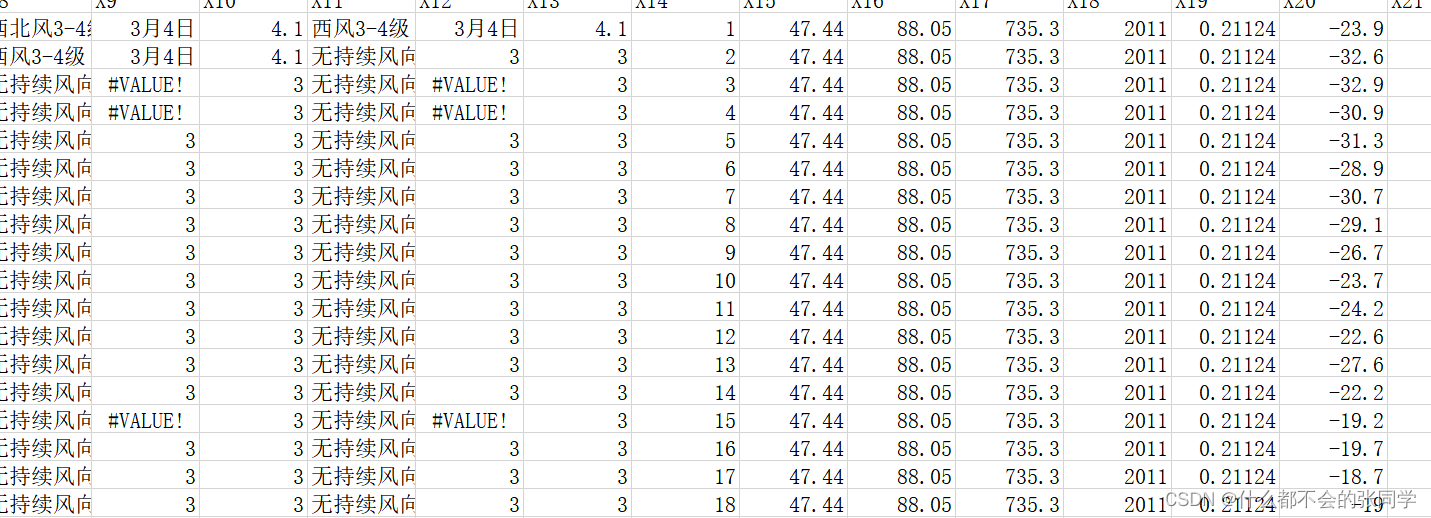
气象数据数据处理实例——matlab字符串切割匹配与R语言日期匹配(数据拼接)

Pytorch深度学习快速入门教程 -- 土堆教程笔记(三)
egg框架使用(一)
创建一个 Dapp,为什么要选择波卡?

After Keil upgrades to AC6, what changes?
MySQL事务
【MindSpore Easy-Diantong Robot-01】You may have seen many knowledge quiz robots, but this one is a bit different
随机推荐
DFINITY 基金会创始人谈熊市沉浮,DeFi 项目该何去何从
2022华数杯数学建模思路分析交流
什么是CRM决策分析管理?
企业的数字化转型到底是否可以买来?
Tanabata romantic date without overtime, RPA robot helps you get the job done
Oracle temporary table space role
入门 Polkadot 平行链开发,看这一篇就够了
Seata source code analysis: initialization process of TM RM client
无题四
The difference between find, matches, lookingAt matching strings in matcher
无题五
High-quality DeFi application building guide to help developers enjoy DeFi Summer
STM32+ULN2003 drives 28BYJ4 stepper motor (forward and reverse according to the number of turns)
IDEA执行Test操作导致数据插入时出现了重复数据
无题八
Keil升级到AC6后,到底有哪些变化?
你最隐秘的性格在哪?
After Keil upgrades to AC6, what changes?
MySQL事务
第五章:多线程通信—wait和notify