当前位置:网站首页>[combinatorics] permutation and combination (set combination, one-to-one correspondence model analysis example)
[combinatorics] permutation and combination (set combination, one-to-one correspondence model analysis example)
2022-07-03 14:37:00 【Programmer community】
Arrange and combine reference blogs :
- 【 Combinatorial mathematics 】 Basic counting principle ( The principle of addition | Multiplication principle )
- 【 Combinatorial mathematics 】 Examples of permutation and combination of sets ( array | Combine | Circular arrangement | binomial theorem )
- 【 Combinatorial mathematics 】 Permutation and combination ( Arrange and combine content summary | Select the question | Set arrangement | Set combination )
- 【 Combinatorial mathematics 】 Permutation and combination ( Examples of permutations )
- 【 Combinatorial mathematics 】 Permutation and combination ( Multiset arrangement | Full Permutation of multiple sets | Multiset incomplete permutation The repetition of all elements is greater than the number of permutations | Multiset incomplete permutation The repetition of some elements is less than the number of permutations )
- 【 Combinatorial mathematics 】 Permutation and combination ( The combinatorial number of multiple sets | The repetition of all elements is greater than the number of combinations | The combinatorial number of multiple sets deduction 1 Division line derivation | The combinatorial number of multiple sets deduction 2 Derivation of the number of nonnegative integer solutions of indefinite equations )
- 【 Combinatorial mathematics 】 Permutation and combination ( Example of the number of combinations of multiple sets | Three counting models | Select the question | Multiple set combinatorial problem | Nonnegative integer solutions of indefinite equations )
- 【 Combinatorial mathematics 】 Permutation and combination ( Two counting principles 、 Set arrangement example | Set arrangement 、 Example of circle arrangement )
One 、 Set combination 、 One to one correspondence model analysis example
take
2
n
2n
2n Personal share
n
n
n Group , Each group
2
2
2 people , How many ways are there ?
First determine whether the problem is a selection problem , Is the element repeated , Whether the selection is orderly ,
- Non repeatable elements , Orderly selection , Corresponding Arrangement of sets
- Non repeatable elements , Unordered selection , Corresponding A combination of sets
- Repeatable elements , Orderly selection , Corresponding Arrangement of multiple sets
- Repeatable elements , Unordered selection , Corresponding Combination of multiple sets
2
n
2n
2n personal , People must not repeat , Divide into
n
n
n Group , There is no difference in the grouping here , Equivalent to the division of sets ;
There are also restrictions , Each group can only put
2
2
2 Elements ;
Original simple model , Such as classification ( Add ) , Step by step ( Multiplication ) , Set arrangement , Set combination , Multiset arrangement , Combination of multiple sets , There is no corresponding model , Cannot be used directly ;
It's not a simple selection problem ;
Here we need to consider Groups are different , There is no difference between groups Two cases ;
If there is a difference in grouping , Divide into
n
n
n Group , Put the first
1
1
1 Group , choose
2
2
2 personal , Put it again
2
2
2 Group , choose
2
2
2 personal ,
⋯
\cdots
⋯ The plan is It can be calculated ;
There is no difference in grouping , At this time, we need to observe There are differences in grouping and There is no difference between The difference between :
There is no difference in grouping , Get a way , Then on
n
n
n Groups are arranged in full , Yes
n
!
n!
n! There are two ways to arrange them , The number of schemes with different groups is obtained ;
There will be Number of schemes with different grouping And Grouping does not distinguish the number of schemes Establish a correspondence :
branch
Group
no
Yes
District
other
Fang
case
Count
×
n
!
=
branch
Group
Yes
District
other
Fang
case
Count
Grouping does not distinguish the number of schemes \times n! = Number of schemes with different grouping
branch Group no Yes District other Fang case Count ×n!= branch Group Yes District other Fang case Count
Number of schemes with different grouping It can be calculated , then Divide
n
!
n!
n! , You can get Number of schemes with no difference in grouping ;
There are differences in grouping , according to Step by step processing The plan :
① The first
1
1
1 Step : from
2
n
2n
2n Of the elements , selection
2
2
2 Elements , Yes
C
(
2
n
,
2
)
C(2n , 2)
C(2n,2) Kind of plan ;
② The first
2
2
2 Step : from
2
n
−
2
2n - 2
2n−2 Of the elements , selection
2
2
2 Elements , Yes
C
(
2
n
−
2
,
2
)
C(2n - 2 , 2)
C(2n−2,2) Kind of plan ;
③ The first
3
3
3 Step : from
2
n
−
4
2n - 4
2n−4 Of the elements , selection
2
2
2 Elements , Yes
C
(
2
n
−
4
,
2
)
C(2n - 4 , 2)
C(2n−4,2) Kind of plan ;
⋮
\vdots
⋮
④ The first
n
n
n Step : from
2
n
−
(
2
n
−
2
)
2n - ( 2n - 2 )
2n−(2n−2) Of the elements , selection
2
2
2 Elements , Yes
C
(
2
n
−
(
2
n
−
2
)
,
2
)
C(2n - ( 2n - 2 ) , 2)
C(2n−(2n−2),2) Kind of plan ; That is to say
1
1
1 Kind of plan ;
Permutation and combination formula
- array :
P
(
n
,
r
)
=
n
!
(
n
−
r
)
!
P(n,r) = \dfrac{n!}{(n-r)!}
P(n,r)=(n−r)!n!
- Combine :
C
(
n
,
r
)
=
P
(
n
,
r
)
r
!
=
n
!
r
!
(
n
−
r
)
!
C(n, r) = \dfrac{P(n,r)}{r!} = \dfrac{n!}{r!(n-r)!}
C(n,r)=r!P(n,r)=r!(n−r)!n!
Step by step processing You need to use the multiplication principle , take
n
n
n Multiply the number of schemes in steps :
N
=
C
(
2
n
,
2
)
C
(
2
n
−
2
,
2
)
C
(
2
n
−
4
,
2
)
⋯
C
(
2
n
−
(
2
n
−
2
)
,
2
)
=
2
n
!
2
!
×
(
2
n
−
2
)
!
×
(
2
n
−
2
)
!
2
!
×
(
2
n
−
4
)
!
⋯
(
2
n
−
(
2
n
−
2
)
)
!
2
!
×
(
2
n
−
(
2
n
−
2
)
−
2
)
!
⏟
n
individual
branch
Step
phase
ride
front
after
can
With
about
fall
very
many
rank
ride
=
(
2
n
)
!
(
2
!
)
n
\begin{array}{lcl} N &=& C(2n , 2) C(2n - 2 , 2) C(2n - 4 , 2) \cdots C(2n - ( 2n - 2 ) , 2) \\\\ &=& \begin{matrix} \underbrace{ \cfrac{2n!}{2! \times (2n-2)!} \times \cfrac{(2n-2)!}{2! \times (2n-4)!} \cdots \cfrac{(2n - ( 2n - 2 ))!}{2! \times (2n - ( 2n - 2 ) - 2)!} } \\ n Multiply by steps \end{matrix} Many factorials can be reduced before and after \\\\ &=& \cfrac{(2n)!}{(2!)^n} \end{array}
N===C(2n,2)C(2n−2,2)C(2n−4,2)⋯C(2n−(2n−2),2)
2!×(2n−2)!2n!×2!×(2n−4)!(2n−2)!⋯2!×(2n−(2n−2)−2)!(2n−(2n−2))!n individual branch Step phase ride front after can With about fall very many rank ride (2!)n(2n)!
The number of schemes with different grouping is
(
2
n
)
!
(
2
!
)
n
\cfrac{(2n)!}{(2!)^n}
(2!)n(2n)! individual ;
according to
branch
Group
no
Yes
District
other
Fang
case
Count
×
n
!
=
branch
Group
Yes
District
other
Fang
case
Count
Grouping does not distinguish the number of schemes \times n! = Number of schemes with different grouping
branch Group no Yes District other Fang case Count ×n!= branch Group Yes District other Fang case Count
The formula ;
Number of schemes with different grouping It can be calculated , then Divide
n
!
n!
n! , You can get Number of schemes with no difference in grouping ;
The end result is
(
2
n
)
!
(
2
!
)
n
n
!
\cfrac{(2n)!}{(2!)^n n!}
(2!)nn!(2n)!
This problem is not simple to use Original simple model , Such as classification ( Add ) , Step by step ( Multiplication ) , Set arrangement , Set combination , Multiset arrangement , Combination of multiple sets ;
Instead, it will be an Uncomputable model , Corresponding to a computable model , Then calculate the model The repeatability of
边栏推荐
- 【北大青鸟昌平校区】互联网行业中,哪些岗位越老越吃香?
- SSH访问控制,多次失败登录即封掉IP,防止暴力破解
- JVM garbage collector
- Similarities and differences between Allegro, OrCAD, net alias, port, off page connector and how to select them
- Zzuli:1040 sum of sequence 1
- 洛谷P5194 [USACO05DEC]Scales S 题解
- Add ZABBIX calculation type itemcalculated items
- Why is this error reported when modifying records in the database
- 分布式事务(Seata) 四大模式详解
- Detailed explanation of four modes of distributed transaction (Seata)
猜你喜欢

NPM install is stuck with various strange errors of node NPY
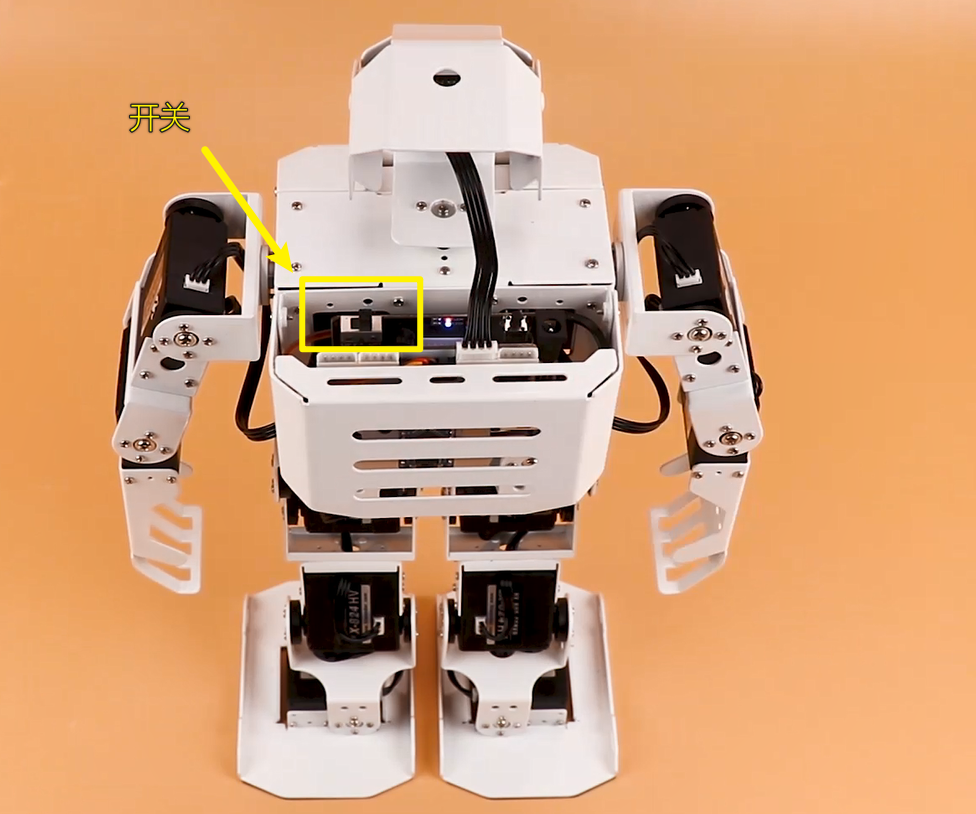
Tonybot humanoid robot starts for the first time 0630

puzzle(016.3)千丝万缕
NFT new opportunity, multimedia NFT aggregation platform okaleido will be launched soon
![洛谷P5018 [NOIP2018 普及组] 对称二叉树 题解](/img/89/da1a3a38e02671628f385de0f30369.png)
洛谷P5018 [NOIP2018 普及组] 对称二叉树 题解

puzzle(016.4)多米诺效应
Dllexport and dllimport

使用并行可微模拟加速策略学习
556. The next larger element III
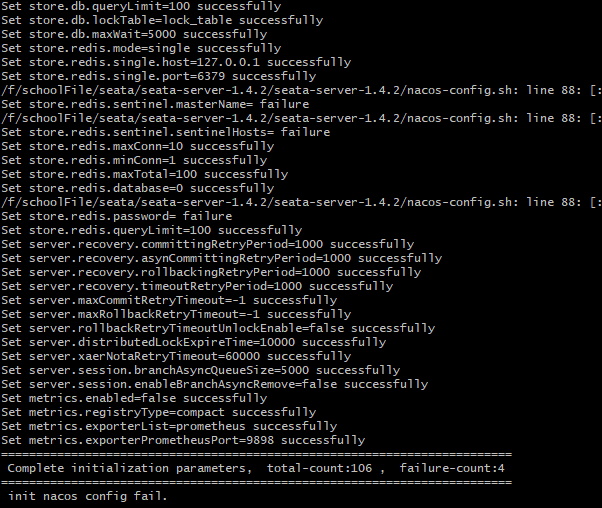
分布式事务(Seata) 四大模式详解
随机推荐
Zzuli:1045 numerical statistics
如何查询淘宝天猫的宝贝类目
Luogu p3065 [usaco12dec]first! G problem solution
7-22 tortoise and rabbit race (result oriented)
LNMP环境mail函数不能发送邮件解决
Zzuli: cumulative sum of 1050 factorials
Timecho of Tianmou technology completed an angel round financing of nearly 100 million yuan to create a native timing database of the industrial Internet of things
Etcd cluster permission management and account password usage
Detailed explanation of four modes of distributed transaction (Seata)
Get permissions dynamically
Jiuyi cloud black free encryption free version source code
Doris学习笔记之数据表的创建
论文分享:Generating Playful Palettes from Images
Thread.sleep和TimeUnit.SECONDS.sleep的区别
Find books ()
Statistical capital consonants
7-14 sum integer segments (C language)
全文检索引擎Solr系列—–全文检索基本原理
Zzuli:1041 sum of sequence 2
tonybot 人形机器人 红外遥控玩法 0630