当前位置:网站首页>[combinatorics] recursive equation (the non-homogeneous part is an exponential function and the bottom is the characteristic root | example of finding a special solution)
[combinatorics] recursive equation (the non-homogeneous part is an exponential function and the bottom is the characteristic root | example of finding a special solution)
2022-07-03 17:23:00 【Programmer community】
List of articles
- One 、 The nonhomogeneous part is Exponential function And The bottom is the case of characteristic root
- Two 、 The nonhomogeneous part is Exponential function And The bottom is the case of characteristic root Example
One 、 The nonhomogeneous part is Exponential function And The bottom is the case of characteristic root
Linear Nonhomogeneous recurrence equation with constant coefficients :
H
(
n
)
−
a
1
H
(
n
−
1
)
−
⋯
−
a
k
H
(
n
−
k
)
=
f
(
n
)
H(n) - a_1H(n-1) - \cdots - a_kH(n-k) = f(n)
H(n)−a1H(n−1)−⋯−akH(n−k)=f(n) ,
n
≥
k
,
a
k
≠
0
,
f
(
n
)
≠
0
n\geq k , a_k\not= 0, f(n) \not= 0
n≥k,ak=0,f(n)=0
The left side of the above equation And “ Linear homogeneous recurrence equation with constant coefficients ” It's the same , But not on the right
0
0
0 , It's based on
n
n
n Of function
f
(
n
)
f(n)
f(n) , This type of recursive equation is called “ Linear Nonhomogeneous recurrence equation with constant coefficients ” ;
The nonhomogeneous part is Exponential function And The bottom is the case of characteristic root :
If the above “ Linear Nonhomogeneous recurrence equation with constant coefficients ” Of Nonhomogeneous part
f
(
n
)
f(n)
f(n) It's an exponential function ,
β
n
\beta^n
βn ,
If
β
\beta
β yes
e
e
e Heavy characteristic root ,
The special solution form of the nonhomogeneous part is :
H
∗
(
n
)
=
P
n
e
β
n
H^*(n) = P n^e \beta^n
H∗(n)=Pneβn ,
P
P
P Is constant ;
The above special solution
H
∗
(
n
)
=
P
n
e
β
n
H^*(n) = P n^e \beta^n
H∗(n)=Pneβn , Into the recursive equation , Solve the constant
P
P
P Value , Then the complete special solution is obtained ;
“ Linear Nonhomogeneous recurrence equation with constant coefficients ” The general solution is
H
(
n
)
=
H
(
n
)
‾
+
H
∗
(
n
)
H(n) = \overline{H(n)} + H^*(n)
H(n)=H(n)+H∗(n)
Use the above solution Special solution , And recurrence equation General solution of homogeneous part , Form the complete general solution of the recursive equation ;
Two 、 The nonhomogeneous part is Exponential function And The bottom is the case of characteristic root Example
Recurrence equation :
H
(
n
)
−
5
H
(
n
−
1
)
+
6
H
(
n
−
2
)
=
2
n
H(n) - 5H(n-1) + 6H(n-2)=2^n
H(n)−5H(n−1)+6H(n−2)=2n, Seeking special solution ?
View its characteristic root :
The standard form of recurrence equation is :
H
(
n
)
−
5
H
(
n
−
1
)
+
6
H
(
n
−
2
)
=
2
n
H(n) - 5H(n-1) + 6H(n-2)=2^n
H(n)−5H(n−1)+6H(n−2)=2n ,
Homogeneous part is
H
(
n
)
−
5
H
(
n
−
1
)
+
6
H
(
n
−
2
)
=
0
H(n) - 5H(n-1) + 6H(n-2)=0
H(n)−5H(n−1)+6H(n−2)=0
Write the characteristic equation :
x
2
−
5
x
+
6
=
0
x^2 - 5x + 6 = 0
x2−5x+6=0 ,
Characteristic root
q
1
=
2
,
q
2
=
3
q_1= 2, q_2 = 3
q1=2,q2=3
Find the recurrence equation Special solutions corresponding to Nonhomogeneous parts ,
The standard form of recurrence equation is :
H
(
n
)
−
5
H
(
n
−
1
)
+
6
H
(
n
−
2
)
=
2
n
H(n) - 5H(n-1) + 6H(n-2)=2^n
H(n)−5H(n−1)+6H(n−2)=2n
The nonhomogeneous part is
2
n
2^n
2n , It's an exponential function , But the bottom line is
1
1
1 Heavy characteristic root ,
At this time, the bottom is
e
e
e The special solution is constructed by repeating the special solution form of the characteristic root
H
∗
(
n
)
=
P
n
e
β
n
H^*(n) = P n^e \beta^n
H∗(n)=Pneβn
The form of the special solution is
H
∗
(
n
)
=
P
n
1
2
n
=
P
n
2
n
H^*(n) = P n^1 2^n = Pn2^n
H∗(n)=Pn12n=Pn2n , among
P
P
P Is constant ;
The special solution is substituted into the above recursive equation :
P
n
2
n
−
5
P
(
n
−
1
)
2
n
−
1
+
6
P
(
n
−
2
)
2
n
−
2
=
2
n
Pn2^n - 5P(n-1)2^{n-1} + 6P(n-2)2^{n-2} = 2^n
Pn2n−5P(n−1)2n−1+6P(n−2)2n−2=2n
All items are constructed
2
n
2^n
2n
P
n
2
n
−
5
P
(
n
−
1
)
2
n
2
+
6
P
(
n
−
2
)
2
n
4
=
2
n
Pn2^n - \cfrac{5P(n-1)2^{n}}{2} + \cfrac{6P(n-2)2^n}{4} = 2^n
Pn2n−25P(n−1)2n+46P(n−2)2n=2n
Divide left and right by
2
n
2^n
2n
P
n
−
5
P
(
n
−
1
)
2
+
3
P
(
n
−
2
)
2
=
1
Pn - \cfrac{5P(n-1)}{2} + \cfrac{3P(n-2)}{2} = 1
Pn−25P(n−1)+23P(n−2)=1
P
n
−
5
P
n
2
+
5
P
2
+
3
P
n
2
−
3
P
=
1
Pn - \cfrac{5Pn}{2} + \cfrac{5P}{2} + \cfrac{3Pn}{2} -3P = 1
Pn−25Pn+25P+23Pn−3P=1
5
P
2
−
3
P
=
1
\cfrac{5P}{2} -3P = 1
25P−3P=1
−
P
2
=
1
-\cfrac{P}{2} = 1
−2P=1
P
=
−
2
P=-2
P=−2
The form of special solution
H
∗
(
n
)
=
P
n
2
n
H^*(n) = Pn2^n
H∗(n)=Pn2n , among
P
P
P Constant value is
−
2
-2
−2 ;
The special solution is
H
∗
(
n
)
=
−
2
n
2
n
H^*(n) = -2n2^n
H∗(n)=−2n2n
边栏推荐
- visual studio “通常每个套接字地址(协议/网络地址/端口)只允许使用一次“
- How to train mask r-cnn model with your own data
- Installation and configuration of network hard disk NFS
- Is AI too slow to design pictures and draw illustrations? 3 sets of practical brushes to save you
- Redis: operation commands for list type data
- 一位普通程序员一天工作清单
- SVN如何查看修改的文件记录
- Comparison of kotlin collaboration + retro build network request schemes
- 在iptables防火墙下开启vsftpd的端口
- 大变局!全国房价,跌破万元大关
猜你喜欢
![[RT thread] NXP rt10xx device driver framework -- Audio construction and use](/img/85/32a83eaa4b7f5b30d4d7c4f4c32791.png)
[RT thread] NXP rt10xx device driver framework -- Audio construction and use
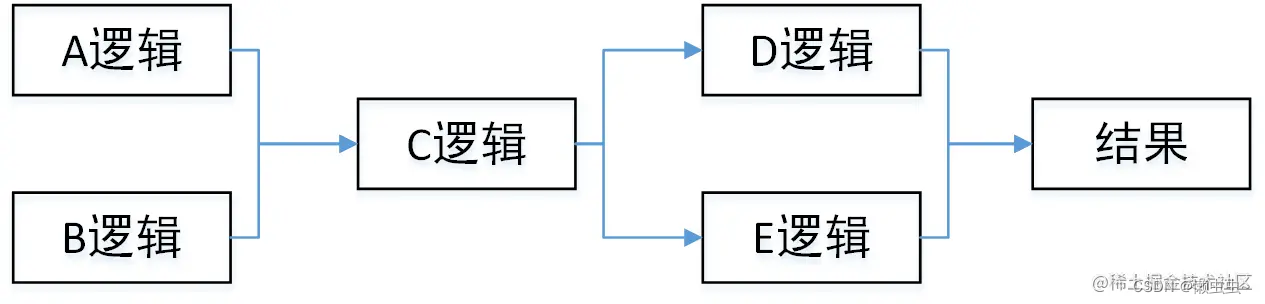
Talk about several methods of interface optimization

Prepare for the golden three silver four, 100+ software test interview questions (function / interface / Automation) interview questions. win victory the moment one raises one 's standard
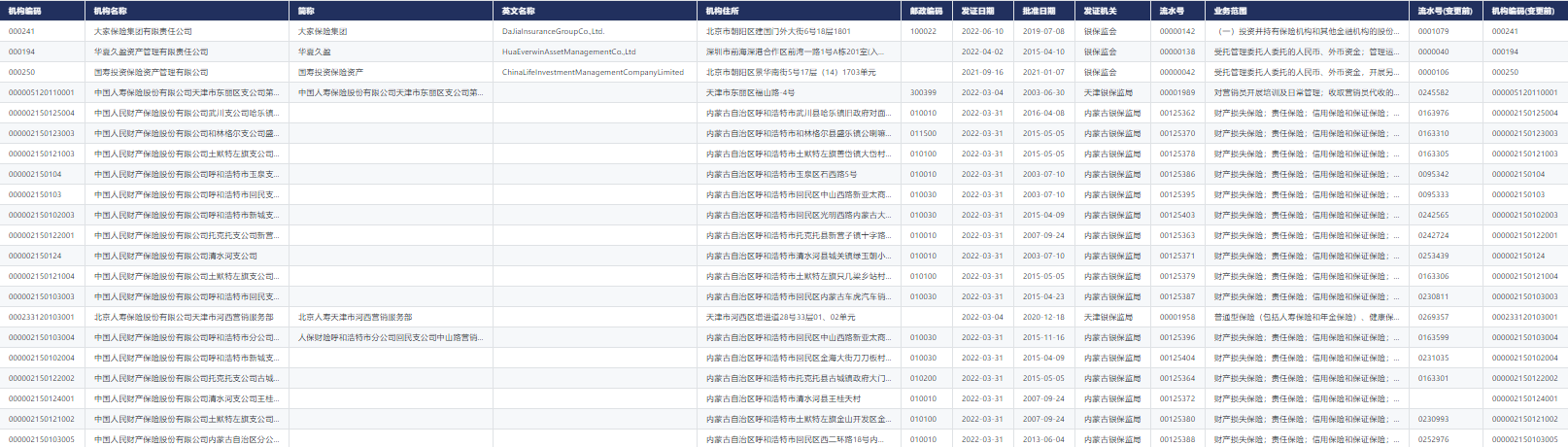
New library online | cnopendata complete data of Chinese insurance institution outlets
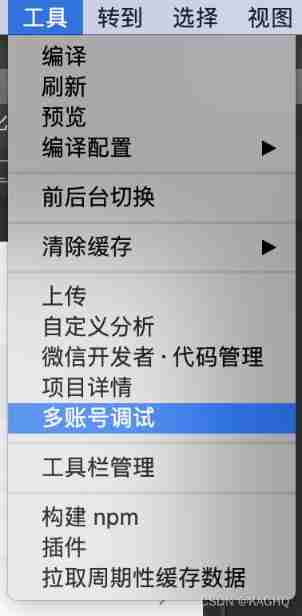
Applet setting multi account debugging

Select 3 fcpx plug-ins. Come and see if you like them
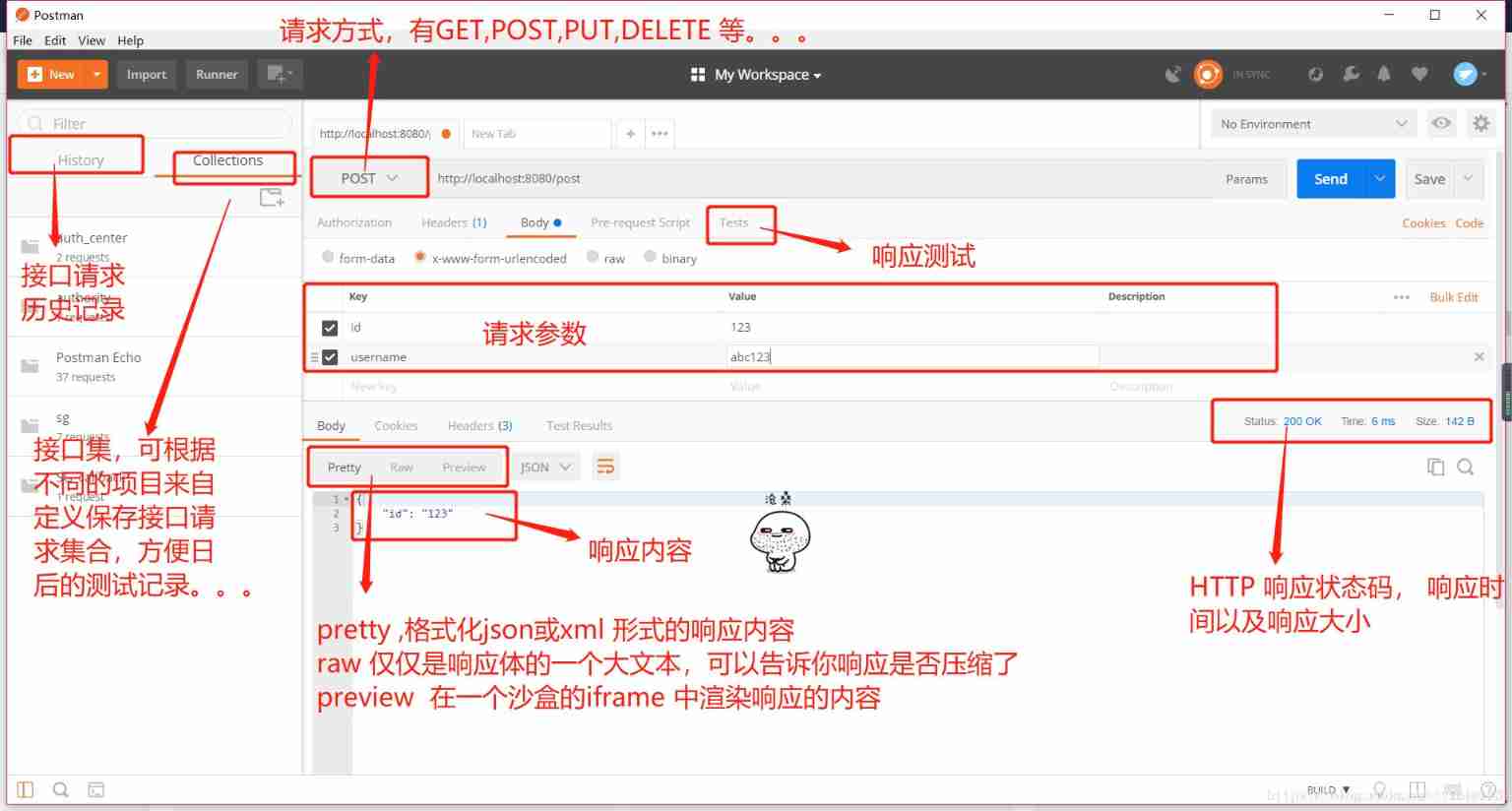
The most complete postman interface test tutorial in the whole network, API interface test

Thread pool: the most common and error prone component of business code

人生还在迷茫?也许这些订阅号里有你需要的答案!

One brush 149 force deduction hot question-10 regular expression matching (H)
随机推荐
[combinatorics] recursive equation (characteristic equation and characteristic root | example of characteristic equation | root formula of monadic quadratic equation)
i++与++i的区别:通俗易懂的讲述他们的区别
Where is the database account used when running SQL tasks in data warehouse tasks configured
Simple use of unity pen XR grab
IntelliJ 2021.3 short command line when running applications
Kubernetes resource object introduction and common commands (V) - (NFS & PV & PVC)
Examination questions for the assignment of selected readings of British and American Literature in the course examination of Fujian Normal University in February 2022
Svn full backup svnadmin hotcopy
Dagong 21 autumn "power plant electrical part" online operation 1 [standard answer] power plant electrical part
Play with fancy special effects. This AE super kit is for you
PS screen printing brush 131, many illustrators have followed suit
How SVN views modified file records
鸿蒙第三次培训
First day of rhcsa study
Collection of the most beautiful graduation photos in the graduation season, collection of excellent graduation photos
The difference between i++ and ++i: tell their differences easily
Kotlin学习快速入门(7)——扩展的妙用
Electronic Science and technology 20th autumn "Microcomputer Principle and application" online assignment 2 [standard answer]
New library online | cnopendata China bird watching record data
Squid 服务启动脚本