当前位置：网站首页>Do you really think binary search is easy

Do you really think binary search is easy

2022-07-06 07:21:00 【Walnut is surnamed Hu, and butterfly is also surnamed Hu】

Basic binary search template analysis , Refer to the leetcode A great god labuladong The antithesis of

Here we first introduce the concept of search interval

Standard bisection template

int a[] = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10};
int target = 0;
cin>>target;
int left = 0;
int right = sizeof(a) / sizeof(a[0]) - 1;//
while(left <= right)//??
{
    int mid = (left + right) >> 1;
    if(a[mid] == target)
    {
        return mid;
    }
    else if(a[mid] < target)
    {
        left = mid + 1;//??
    }
    else
    {
        right = mid - 1;//??
    }
}
return -1;// Did not find

1.while Conditions in brackets

What we use here is [ ,] An interval closed on both sides . It's just right It points to the subscript of the last common element . If we use [ , ] Words , Then we while The conditions inside must be written left <= right, It can't be <, Because we have a closed interval here, it means that the condition for the end of the cycle is [left + 1, left], This interval does not exist , So no common elements are missing . But if our ending condition is left < right Words , So the end condition is [left, left], We can find that there are also missing common elements , So it's wrong . This is Search range . Another example , Suppose we write right = sizeof(a) / sizeof(a[0]) Words , So it means [ ,), because right Actually, it doesn't exist , Suppose we write left <= right, So the end condition is [left + 1, left）, The interval does not exist , But we cycled once more , And this is more than one cycle Probably It will make the loop fall into a wireless loop . If write left < right, So the end condition is [left , left), There is also nothing missing .

2.left = mid + 1？？ right = mid - 1？？

Why do you write this ？ The reason is simple , Or use the search interval ,[ ,] The scope of our search is [left, mid - 1] and [mid+ 1, right], because mid We have already found , There is no need to look for it again , If you want to put mid Taking it into account may lead to a dead cycle , Because the interval has intersection , If the final result happens to be within the intersection range, it will cycle all the time . But if we use [ , ) Words , So the scope is [left, mid) and [mid + 1, right), you 're right , this is it .

The method of searching the interval is introduced , Next, continue to look down .

#include<iostream>
using namespace std;
int main()
{
    int a[] = { 1,2,3,4,5,6,7,8,9,10 };
    int left = 0;
    int right = sizeof(a) / sizeof(a[0]) - 1;
    int target = 0;
    cin >> target;
    while (left <= right)
    {
        int mid = (left + right) / 2;
        if (a[mid] < target)
        {
            left = mid;
        }
        else if (a[mid] > target)
        {
            right = mid;
        }
        else
        {
            cout << mid;
            break;
        }
    }
    return 0;
}

Of course, for this standard template , We don't follow the rules exactly , Write left = mid,right = mid It's fine too , It's just [left, mid] and [mid, right], It's just that the number of cycles may be a little more .

About binary search

Traditional binary search ： Orderly , Find the position of a number . But in fact, binary search is not so rigid , Binary search is just a way to find , The time complexity is zero o(logN), Because when the topic requires time complexity, it must be o(logN) We will probably use two-point search . As a way to find , Binary search adopts the strategy of cutting half each time , Each reduction of half allows us to sort half of the data at one time , Instead of a total or several data , So we should pay more attention to the traditional conditions of binary search , But the strategy of cutting half at a time .

First , We want to be efficient , Cut it in half at one time , Then we must get half the chips cut at one time , When we can cut it in half at a time, it shows that the data has some rules , Order is the simplest rule , This is also one of the reasons why we cannot correctly understand the binary search . In my submission ： If you can find , Then you can use binary search . Binary search actually has one kind Close to The meaning of is in it , Let's first look at a set of codes for the simplest binary search , Take a look at the traditional model of binary search ：

int a[] = {1, 2, 3, 4, 5, 6, 7, 8, 9, 10};
int target = 0;
cin>>target;
int left = 0;
int right = sizeof(a) / sizeof(a[0]) - 1;
while(left <= right)
{
    int mid = (left + right) >> 1;
    if(a[mid] == target)
    {
        cout<<mid<<endl;
        break;
    }
    else if(a[mid] < target)
    {
        left = mid + 1;
    }
    else
    {
        right = mid - 1;
    }
}

Exclude half of the data at a time , until Section Keep approaching the right answer , Find the right answer .

The variation of binary search

The binary search variant here still stays on the basis of traditional binary search , Only slightly modified , The preconditions here are still orderly , Nothing has changed in essence , Be careful , In fact, order is a law , As long as we have laws that can replace order , The premise is that this rule must be able to eliminate half of the data at one time , I will explain one by one later .

seek target >= x The leftmost boundary of the number of

Using the approximation property of binary search , But the difference is that we have found all the binary search this time , Do not give exit halfway , Look at the code first ：

Here is a popular solution on the Internet , I will upgrade it later ：

int a[] = {1, 2, 2, 2, 3, 4, 5};
int target = 2;// The boundary we are looking for is 2
int left = 0;
int right = sizeof(a) / sizeof(a[0]) - 1;
while(left <= right)
{
    int mid = (right + left) / 2;
    if(target == a[mid])
    {
        right = mid - 1;// Do not exit when found , Rather let right Continue to approach , Why right, Because the description is looking for the leftmost boundary of the number 
    }
    else if(target > a[mid])
    {
        left = mid + 1;
    }
    else
    {
        right = mid - 1;
    }
}
cout<<left<<endl;// Find boundary , Print or return if it is a function 
// perhaps  cout<<right + 1<<endl;

This is similar to the standard template of binary search , If you want to use [ , ) Of course, it can be changed . The point is how to get the boundary ？

    if(target == a[mid])
    {
        right = mid - 1;// Do not exit when found , Rather let right Continue to approach , Why right, Because the description is looking for the leftmost boundary of the number 
    }

That's the key , When we find the answer, we don't let the binary search stop , But let it keep approaching , Until you find the right answer .

Why in the end left perhaps right + 1 It is the result.

our left and right What does that mean? ？left and right It represents the left and right boundaries of the interval , Why can we use binary search ？ The answer is to use the left and right boundaries not only to compress and shrink , Finally, lock to a specific number , It's the same here , Our aim is to find target >= x The leftmost border of , therefore left It's the initiative ,left It's the left border , This left boundary will eventually press target The location of , Why? right Not necessarily the right answer , because right Are passive , As a right boundary, it cannot stand on the position of the left boundary . Here's the picture

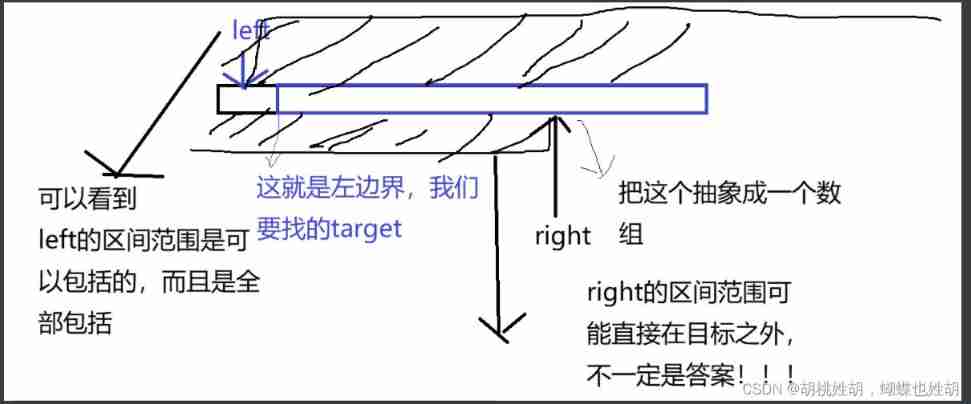
So why right + 1 It can also be the answer ？ Because at the end of the cycle left = right + 1, It's that simple .

No return found -1 Do you ？

This is not the same as looking for numbers , Because we let binary search complete , So finally, if there is no boundary we want in our array , Well, it's very likely that left perhaps right Directly outside the scope of the subscript . Therefore, it is necessary to verify before deciding to return left still -1

Check here left Cross border situation of , because left It's the initiative

if(left > sizeof(a) / sizeof(a[0]) || a[left] != target)// The first condition is target Bigger than all , The second is target Smaller than all ,left Still in 0, So just judge 0 Is it what we want .

seek target <= x The rightmost boundary of the number of

Let's put it another way

int a[] = {1, 2, 2, 2, 3, 4, 5};
int target = 2;// The boundary we are looking for is 2
int left = 0;
int right = sizeof(a) / sizeof(a[0]);
while(left < right)
{
    if(a[left] == target)
    {
        left = mid + 1;// Must write mid + 1, The search interval was mentioned earlier ,
    }
    else if(a[left] < target)
    {
        left = mid + 1;
    }
    else
    {
        right = mid;
    }
}
// Check right Cross border situation of 
if(right < 0 || a[right] != target)
{
    return -1;
}
return right - 1;// Return here right Be right , Because the end condition of the loop is left = right, So back left - 1 It's fine too

Why subtract one here ？

Because I have an open section on my right , Final right It must be in the right position in the interval , But because of the open interval , therefore right - 1 It's just target The location of . If you write a closed interval, write it directly right That's all right. .

improvement ： We use a variable to hold the boundary value , Finally, return the subscript of the variable , Deal with many situations in this way

int a[] = {1, 2, 2, 2, 3, 4, 5};
int target = 2;// The boundary we are looking for is 2
int left = 0;
int right = sizeof(a) / sizeof(a[0]) - 1;
int temp;// Define temporarily stored variables 
while(left <= right)
{
    int mid = (right + left) / 2;
    if(a[mid] >= target)
    {
        temp = mid;
        right = mid - 1;
    }
    else
    {
        left = mid + 1;
    }
}
return temp;

Application of binary search , Is binary search very rigid ？

34. Find the first and last positions of the elements in the sort array

Model application ： Look for a number in the range ：

class Solution {
public:
    int searchRangeleft(vector<int>& nums, int target)
    {
        int left = 0;
        int right = nums.size() - 1;
        while(left <= right)
        {
            int mid = (left + right) / 2;
            if(nums[mid] < target)
            {
                left = mid + 1;
            }
            else
            {
                right = mid - 1;
            }
        }
        if(left > nums.size() - 1 || nums[left] != target)
        {
            return -1;
        }
        else
        {
            return left;
        }
    }
    int searchRangeright(vector<int>& nums, int target)
    {
        int left = 0;
        int right = nums.size() - 1;
        while(left <= right)
        {
            int mid = (left + right) / 2;
            if(nums[mid] > target)
            {
                right = mid - 1;
            }
            else
            {
                left = mid + 1;
            }
        }
        if(right < 0 || nums[right] != target)
        {
            return -1;
        }
        else
        {
            return right;
        }
    }
    vector<int> searchRange(vector<int>& nums, int target) {
        if(nums.size() == 0)
        {
            vector<int> result{-1, -1};
            return result;
        }
        vector<int> result;// Something used to save the results 
        int lefttemp = searchRangeleft(nums, target);
        int righttemp = searchRangeright(nums, target);
        result.push_back(lefttemp);
        result.push_back(righttemp);
        return result;
    }
};

There is nothing to explain this problem. In fact . To be exact, it's quite traditional .

Problems can be converted ：

class Solution {
public:
int helper(vector<int>& nums, int target)// Here is the right boundary of the search
{
int left = 0;
int right = nums.size() - 1;
while(left <= right)
{
int mid = (left + right) / 2;
if(nums[mid] <= target)
{
left = mid + 1;
}
else
{
right = mid - 1;
}
}
return right;
}
int search(vector<int>& nums, int target) {
// Code optimization , Use a function to unify , There's no need to write 2 individual
return helper(nums, target) - helper(nums, target - 1);// Right border and right border - 1
}
};

35. Search insert location

class Solution {
public:
int searchInsert(vector<int>& nums, int target) {
// Problem transformation , Convert to search greater than or equal to target A subscript of , That is greater than or equal to target The boundary of the
int left = 0;
int right = nums.size() - 1;
while(left <= right)
{
int mid = (left + right) / 2;
if(nums[mid] >= target)
{
right = mid - 1;
}
else
{
left = mid + 1;
}
}
return left;
}
};

852. The peak index of the mountain array

Mountain array problem , Local maximum problem

class Solution {

public:

    int peakIndexInMountainArray(vector<int>& arr) {

        // Here is exactly the same

        int left = 1;

        int right = arr.size() - 2;

        while(left <= right)// What this means is that we can't start from the beginning and end , This is determined by the definition of the mountain array

            int mid = (left + right) / 2;

            if(arr[mid] >= arr[mid + 1])

                right = mid - 1;

            else

                left = mid + 1;

        return left;

};

Local minimum problem

Just find a local minimum in a completely unordered array

Local minimum definition ：a[mid - 1] < a[mid] < a[mid + 1], We promise to give a length greater than 3 Array of

int left = 1;
int right = nums.size() - 2;
while(left <= right)
{
int mid = (left + right) / 2;
if(nums[mid] < nums[mid + 1] && nums[mid] < nums[mid - 1])
{
return mid;
}
else
{
if(nums[mid] > nums[mid - 1])
{
left = mid + 1;
}
else
{
right = mid + 1;
}
}
}

Trisection

The trisection method does not divide the interval into three , Instead, veto one-third of each time , Usually used to find the peak , Peak problem

852. The peak index of the mountain array

Usually we use 「 Two points 」 Check the value , You need to ensure that the sequence itself meets 「 Dichotomy 」： When selecting an endpoint （ At baseline ） after , combination 「 A period of satisfaction & Another paragraph is not satisfied 」 To achieve “ Half ” Search results .

seeing the name of a thing one thinks of its function ,「 Three points 」 It is to use two endpoints to divide the interval into three , Then approach the target value by rejecting one-third of the interval each time .

Concrete , Because the peak element is the global maximum , Therefore, we can divide the current interval into l, m1、m1, m2 and m2, r Three paragraph , If meet arr[m1] > arr[m2]arr[m1]>arr[m2], It shows that the peak element cannot exist with m2, r in , Give Way r = m2 - 1r=m2−1 that will do . Another interval analysis is the same .

（ The idea comes from ： Gongshui Sanye ）

class Solution {

public:

    int peakIndexInMountainArray(vector<int>& arr) {

        int left = 0;

        int right = arr.size() - 1;// It can be written here , because /3 The nature of allows us not to abandon the head and tail

        while(left <= right)

            int third1 = left + (right - left) / 3;

            int third2 = right - (right - left) / 3;

            if(arr[third1] > arr[third2])

                right = third2 - 1;

            else// The implicit condition here is arr[third1] <= arr[third2], Here we can see that we are dominated by the right , So we should return to the right

                left = third1 + 1;

        return right;

};

153. Look for the minimum value in the rotation sort array

Ideas ： The array is divided into two segments because of rotation ： But we still have Dichotomy , in other words , You can still use binary search to find .

class Solution {
public:
int findMin(vector<int>& nums) {
int left = 0;
int right = nums.size() - 1;
if(nums[left] <= nums[right])
{
return nums[0];
}
while(left <= right)
{
if(right - left == 1)
{
return nums[right];
}
int mid = (left + right) / 2;
if(nums[mid] > nums[left])
{
left = mid;// This anti routine writing , Instead, it guarantees left It must not go through ,right Not even
}
else
{
right = mid;
}
}
return -1;
}
};

The depth of the summary ：

We have a little time , To jump out of the traditional template , But keep the template in mind , For example, the above question , I don't use templates , And it's written left = mid, and right = mid, This will result in left Never go out of its field ,right Not even , Continuous circulation will eventually lead to a difference between them , And then back again . Using this principle , That is to use left = mid When , We are in a passive State of , We can't escape from any one at this time field , Instead, we can only wait for others to collide with us .

class Solution {

public:

    int findMin(vector<int>& nums) {

        int low = 0;

        int high = nums.size() - 1;

        while (low < high) {

            int pivot = low + (high - low) / 2;

            if (nums[pivot] < nums[high]) {

                high = pivot;

            else {

                low = pivot + 1;

        return nums[high];// The end condition of the loop is high = low, So we write high perhaps low It's all possible

};

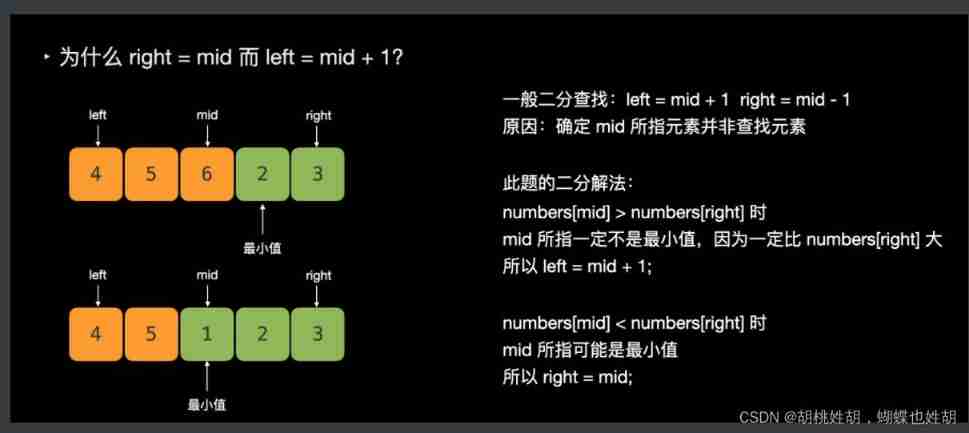
This code is also the code of the above question , But the more this code hides a lot of information .

1. We used [ , ], however while Inside <, The purpose is that when we end the cycle low = high Of , And it must be biased towards high The location of , because high = pivot, and if The conditions inside indicate that it will not cross the boundary left over there . This is caused by the nature of this problem .

2. Our comparison needs a standard , It can be high, It can also be low, Why did we choose high？. For this question , This kind of writing is approximate ,while There is no halfway exit in the cycle , The above method doesn't matter , Because there is no exit , So we must not be satisfied while The conditions inside can exit , But this happens to be when the exit conditions are equal , And at this time, the missing element can be obtained from the search interval is the answer itself , But if we don't write low = pivot + 1 Words , We will never get low = high, Because they can't cross their fields , So the following low It must be written like this , It can't be changed , So at this time, we should take high As a standard , The reason is simple , Because in order to high As a standard high Never cross the line , At this time low As a standard, if the time is right low Will run to strange places . therefore high = pivot And with high As a standard, it is a serial condition , Cannot be separated

To make a long story short ： We'd better use the right value for comparison , It must be ！！！ Why high？ Again ,4 5 1 2 3, If we use left,nums[pivot] < nums[left], Can you judge which side it is ？ All elements on the left must be larger than those on the right , So if you judge that a certain element is better than a certain value on the right , And the value on the right is always smaller on the right , Then this value must also be on the right , If you use the left side to judge , The value on the left , It may also be smaller than the value on the left .

Look again ：

class Solution {

public:

    int findMin(vector<int>& nums) {

        int low = 0;

        int high = nums.size() - 1;

        while (low <= high) {

            int pivot = low + (high - low) / 2;

            if (nums[pivot] <= nums[high]) {

                high = pivot;

            else {

                low = pivot + 1;

        return nums[high];// The end condition of the loop is high = low, So we write high perhaps low It's all possible

};

In the computer world ,÷ An integer is going to the small side .

This code will Dead cycle .

[3,4,5,1,2], Because when we low = high When , The cycle is not over yet , At this time pivot It must still be where it is , Then it happens again high = pivot, Keep cycling , Cannot break the balance . What's the problem ？ if (nums[pivot] <= nums[high]). Because of this = and high = pivot This passive state overlaps , So this happens , Here we also get some enlightenment , That's it When your cycle condition is low == high It's not over yet , If you write a passive state below , Then the condition of this passive state must not be =.

Let's get rid of this =,

class Solution {

public:

    int findMin(vector<int>& nums) {

        int low = 0;

        int high = nums.size() - 1;

        while (low <= high) {

            int pivot = low + (high - low) / 2;

            if (nums[pivot] < nums[high]) {

                high = pivot;

            else {

                low = pivot + 1;

        return nums[high];// The end condition of the loop is high = low, So we write high perhaps low It's all possible

};

You can find oj After that .return nums[low - 1] It's fine too , We can look at it in two ways ：1. The end condition of the loop 2. If you want to end stiffness in the same state , Can only be low = pivot + 1, conversely , If you write the conditions , If you can't reach this key node , Then there must be problems . Then if we change while There will be no dead cycle if there are conditions inside ：

class Solution {

public:

    int findMin(vector<int>& nums) {

        int low = 0;

        int high = nums.size() - 1;

        while (low < high) {

            int pivot = low + (high - low) / 2;

            if (nums[pivot] < nums[high]) {// This is the time , You can add an equal sign here or not

                high = pivot;

            else {

                low = pivot + 1;

        return nums[high];// The end condition of the loop is high = low, So we write high perhaps low It's all possible

};

（ Images from leetcode Official explanation ）

About while Add the equals sign in the brackets of , My suggestion is not to add , Because after adding it, you have a greater possibility of causing a dead cycle , So don't wait , One of the missing answers in this question is the answer , That's all right. . The explanation we made earlier is just to make the topic more profound .

The difficulty increases again ：

154. Look for the minimum value in the rotation sort array II

The difference between this question and the previous one is ： Repetition is allowed here , In fact, we only need to add a small piece of code on the original basis

class Solution {

public:

    int findMin(vector<int>& nums) {

        // This method does not need to be considered as much as above , Because we have conditions that can be judged inside the loop

        int left = 0;

        int right = nums.size() - 1;

        if(nums.size() == 1)// It must be judged here , Because it can't be written below =

            return nums[0];

        if(nums[left] < nums[right])// You can't write here =[3, 1, 3]

            return nums[left];

        while(left <= right)

            if(right - left == 1)

                return nums[right];

            int mid = (left + right) / 2;

            if(nums[mid] == nums[left] && nums[left] == nums[right])// It shows that we cannot make a good judgment , At this time, take a linear approach

                int result = nums[left];

                for(int i = left + 1; i < right; ++i)// Here we no longer need to judge left and right The location of

                    if(nums[i] < result)

                        return nums[i];

            if(nums[mid] >= nums[left])

                left = mid;

            else

                right = mid;

        return -1;

};

The overall framework of the topic is the same as before , There is no essential change

Or so ：

class Solution {
public:
int findMin(vector<int>& nums) {
int left = 0;
int right = nums.size() - 1;
while(left < right)
{
int mid = (left + right) / 2;
if(nums[mid] < nums[right])
{
right = mid;
}
else if(nums[mid] > nums[right])
{
left = mid + 1;
}
else
{
right--;// Reduce the scale of the problem
}
}
return nums[left];
}
};