当前位置：网站首页>Summary of leetcode's dynamic programming 4
Summary of leetcode's dynamic programming 4
2022-07-06 06:25:00 【nuist__ NJUPT】
leetcode Summary of dynamic programming 4
1- The longest palindrome subsequence
Topic link ： The title link is here ！！！
Ideas ： Dynamic programming
dp[i][j]: Indicates from subscript i To the subscript j Maximum palindrome sequence of
if s[i]==s[j]
The recurrence formula is as follows ：
dp[i][j] = dp[i+1][j-1]+2 ;
if s[i]!=s[j]
The recurrence formula is as follows ：
dp[i][j] = Math.max(dp[i+1][j],dp[i][j-1]) ;
class Solution {
public int longestPalindromeSubseq(String s) {
int n = s.length() ;
int [][] dp = new int [n][n] ;
for(int i=0; i<n; i++){
dp[i][i] = 1 ;
}
for(int i=n-1; i>=0; i--){
for(int j=i+1; j<n; j++){
if(s.charAt(i) != s.charAt(j)){
dp[i][j] = Math.max(dp[i+1][j],dp[i][j-1]) ;
}else{
dp[i][j] = dp[i+1][j-1]+2 ;
}
}
}
return dp[0][n-1] ;
}
}
2- One and zero
Topic link ： The title link is here ！！！
Ideas ： Deformation of knapsack problem
This problem is very similar to the classic knapsack problem , But there is only one capacity different from the classical knapsack problem , This problem has two capacities , That is... In the selected string subset 0 and 1 The maximum number of .
The classical knapsack problem can be solved by two-dimensional dynamic programming , The two dimensions are goods and capacity . This problem has two capacities , Therefore, it is necessary to use three-dimensional dynamic programming to solve , The three dimensions are string 、0 Capacity and 1 The capacity of .
Define 3D array dp, among dp[i][j][k] In front of i In strings , Use j individual 0 and k individual 1 The maximum number of strings that can be obtained in the case of .
The equation of state transfer is as follows ：
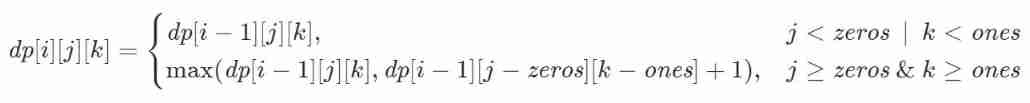
class Solution {
public int findMaxForm(String[] strs, int m, int n) {
int len = strs.length ;
int [][][] dp = new int [len+1][m+1][n+1] ;
for(int i=1; i<=len; i++){
int [] zero =count(strs[i-1]) ;
int zeros = zero[0], ones = zero[1] ;
for(int j=0; j<=m; j++){
for(int k=0; k<=n; k++){
if(j>=zeros && k>=ones){
dp[i][j][k] = Math.max(dp[i-1][j][k],dp[i-1][j-zeros][k-ones]+1) ;
}else{
dp[i][j][k] = dp[i-1][j][k] ;
}
}
}
}
return dp[len][m][n] ;
}
public int [] count(String s){
int [] zeros = new int [2] ;
for(int i=0; i<s.length(); i++){
zeros[s.charAt(i)-'0']++ ;
}
return zeros ;
}
}
3- Use the minimum cost to climb the stairs
Topic link ： The title link is here ！！！
Ideas ：dp[i]: Means to climb to the current i The minimum cost of location
dp[0]=0
dp[1]=0
Recursive expression ：
dp[i] = Math.min(dp[i-1]+cost[i-1],dp[i-2]+cost[i-2]) ;
class Solution {
public int minCostClimbingStairs(int[] cost) {
int [] dp = new int [cost.length+1] ;
for(int i=2; i<=cost.length; i++){
dp[i] = Math.min(dp[i-1]+cost[i-1],dp[i-2]+cost[i-2]) ;
}
return dp[cost.length];
}
}
4- Change for II
Topic link ： The title link is here ！！！
Ideas ： use dp[x] Indicates that the sum of the amounts equals x Number of coin combinations , The goal is to seek dp[amount]. The boundary of dynamic programming is dp[0]=1. Only when no coin is selected , The sum of the amount is 0, So only 1 A combination of coins .
class Solution {
public int change(int amount, int[] coins) {
int [] dp = new int [amount+1] ;
dp[0] = 1 ;
for(int coin : coins){
for(int i=coin; i<=amount; i++){
dp[i] += dp[i-coin] ;
}
}
return dp[amount] ;
}
}
5- A beautiful arrangement
Topic link ： The title link is here ！！！
Ideas 1：dfs+ Mark
Every round of search , As long as the current number and subscript can be taken from each other , Then accumulate the permutation number , Continue to search . Each search needs to mark the number that has been searched .
class Solution {
public int countArrangement(int n) {
return dfs(n,1,new boolean[n+1]) ;
}
public int dfs(int n, int i, boolean [] vis){
if(i>n){
return 1 ;
}
int ans = 0 ;
for(int num=1; num<=n; num++){
if(!vis[num] &&(i%num==0 || num%i==0)){
vis[num] = true ;
ans += dfs(n,i+1,vis) ;
vis[num] = false ;
}
}
return ans ;
}
}
Ideas 2： backtracking
We can use backtracking to solve this problem , Put the number into the target arrangement from left to right .
In particular , We define functions backtrack(i,n), Indicates an attempt to move to the location i Put in number . among n Indicates the length of the arrangement . In the current function , We first find a qualified unused number , Then recursively backtrack(i+1,n), When the function is finished , Go back to the current layer , Let's try the next qualified unused number .
Back in the process , We can use vis Array marks which numbers have been used , Every time we choose a number idx, We will vis[idx] Marked as true, When backtracking is complete , Let's set it to false.
Specially , To optimize backtracking efficiency , We can preprocess the number of qualified for each location , Using arrays match preservation . When we try to position i When you put in the number , We just need to traverse match[i] that will do .
class Solution {
List<Integer> [] match ;
boolean [] vis ;
int num ;
public int countArrangement(int n) {
match = new List[n+1] ;
vis = new boolean[n+1] ;
for(int i=1; i<=n; i++){
match[i] = new ArrayList<>() ;
}
for(int i=1; i<=n; i++){
for(int j=1; j<=n; j++){
if(i%j==0 || j%i==0){
match[i].add(j) ;
}
}
}
backtrack(1,n) ;
return num ;
}
public void backtrack(int i, int n){
if(i==n+1){
num++ ;
return ;
}
for(int idx : match[i]){
if(!vis[idx]){
vis[idx] = true ;
backtrack(i+1,n) ;
vis[idx] = false ;
}
}
}
}
6- The number of paths out of bounds
Topic link ： The title link is here ！！！
Ideas ： Search for + Mark
Search in four directions in each round , If you can get out of bounds, you will accumulate 1, The steps are 0 when , Can't go out of bounds , return 0, After the search is completed, you need to mark the answers that have been searched in the current position under a certain number of steps , When the next round of search encounters , Just go back .
class Solution {
int [] dx = {
-1,1,0,0} ;
int [] dy = {
0,0,-1,1} ;
int [][][]cache ;
int mod = 1000000007;
public int findPaths(int m, int n, int maxMove, int startRow, int startColumn) {
cache = new int [m][n][maxMove+1] ;
for(int i=0; i<m; i++){
for(int j=0; j<n; j++){
for(int k=1; k<=maxMove; k++){
cache[i][j][k] = -1 ;
}
}
}
return dfs(startRow,startColumn,maxMove,m,n) ;
}
public int dfs(int x, int y, int maxMove, int m, int n){
if(x<0 || y<0 || x>=m || y>=n){
return 1 ;
}
if(maxMove==0){
return 0 ;
}
if(cache[x][y][maxMove]!=-1){
return cache[x][y][maxMove] ;
}
int ans = 0 ;
for(int i=0; i<4; i++){
int tx = x + dx[i] ;
int ty = y + dy[i] ;
ans += dfs(tx,ty,maxMove-1,m,n) ;
ans = ans % mod ;
}
cache[x][y][maxMove] = ans ;
return ans ;
}
}
Ideas ： Dynamic programming
The state of dynamic planning is determined by the number of moves 、 Rows and columns determine , Definition dp[i][j][k] Indicates that the ball moves i After times, it is located in the coordinate (j,k) Number of paths for .
class Solution {
int [] dx = {
-1,1,0,0} ;
int [] dy = {
0,0,-1,1} ;
int mod = 1000000007 ;
public int findPaths(int m, int n, int maxMove, int startRow, int startColumn) {
int [][][] dp = new int [maxMove+1][m][n] ;
dp[0][startRow][startColumn] = 1;
int sum = 0 ;
for(int i=0; i<maxMove; i++){
for(int j=0; j<m; j++){
for(int k=0; k<n; k++){
int count = dp[i][j][k] ;
if(count > 0){
for(int x=0; x<4; x++){
int tx = j + dx[x] ;
int ty = k + dy[x] ;
if(tx>=0 && tx<m && ty>=0 && ty<n){
dp[i+1][tx][ty] = (dp[i+1][tx][ty]+count) % mod ;
}else{
sum = (sum+count)%mod ;
}
}
}
}
}
}
return sum ;
}
}
7- Deletion of two strings
Topic link ： The title link is here ！！！
Ideas ：dp[i][j] Said to i-1 String word1, With j-1 a null-terminated string word2 To achieve equality , The minimum number of elements to delete .
if word1[i-1] be equal to word2[j-1] You don't need to delete , The recurrence formula is as follows ：
dp[i][j] = dp[i-1][j-1] ;
if word1[i-1] It's not equal to word2[j-1], You need to delete , There are three possibilities , The recurrence formula is as follows ：
dp[i][j] = Math.min(dp[i-1][j]+1,Math.min(dp[i][j-1]+1,dp[i-1][j-1]+2)) ;
class Solution {
public int minDistance(String word1, String word2) {
int [][] dp = new int [word1.length()+1][word2.length()+1] ;
for(int i=1; i<word1.length()+1; i++){
dp[i][0] = i ;
}
for(int j=1; j<word2.length()+1; j++){
dp[0][j] = j ;
}
for(int i=1; i<word1.length()+1; i++){
for(int j=1; j<word2.length()+1; j++){
if(word1.charAt(i-1)==word2.charAt(j-1)){
dp[i][j] = dp[i-1][j-1] ;
}else{
dp[i][j] = Math.min(dp[i-1][j]+1,Math.min(dp[i][j-1]+1,dp[i-1][j-1]+2)) ;
}
}
}
return dp[word1.length()][word2.length()] ;
}
}
8- Palindrome string
Topic link ： The title link is here ！！！
Ideas ：dp[j][i] From position j To the position i Is it a palindrome string .
If s.charAt(i)==s.charAt(j)
And (i-j<2 || dp[j+1][i-1])
It must be a palindrome string .
class Solution {
public int countSubstrings(String s) {
boolean [][] dp = new boolean [s.length()][s.length()] ;
int cnt = 0 ;
for(int i=0; i<s.length(); i++){
for(int j=0; j<=i; j++){
if(s.charAt(i)==s.charAt(j)&&(i-j<2 || dp[j+1][i-1])){
dp[j][i] = true ;
cnt ++ ;
}
}
}
return cnt ;
}
}
9- The number of longest increasing subsequences
Topic link ： The title link is here ！！！
determine dp The meaning of arrays and subscripts
For this topic, we will maintain two arrays together .
dp[i]：i Before （ Include i） The length of the longest increasing subsequence is dp[i]
cnt[i]： With nums[i] String ending with , The number of longest increasing subsequences is cnt[i]
Determine the recurrence formula
In the longest ascending subsequence , The state transition we give is ：
if (nums[i] > nums[j]) dp[i] = max(dp[i], dp[j] + 1);
namely ： Location i The longest increasing subsequence length of be equal to j from 0 To i-1 The longest ascending subsequence at each position + 1 The maximum of .
This question is not so simple , We need to consider two dimensions , One is dp[i] Update , One is cnt[i] Update .
So how to update cnt[i] Well ？
With nums[i] String ending with , The number of longest increasing subsequences is cnt[i].
So in nums[i] > nums[j] On the premise of , If in [0, i-1] Within the scope of , eureka j, bring dp[j] + 1 > dp[i], It indicates that a longer increasing subsequence has been found .
So in order to j Increment the number of subsequences for the longest substring at the end , Is the latest in i Increment the number of subsequences for the longest substring at the end , namely ：cnt[i] = cnt[j].
stay nums[i] > nums[j] On the premise of , If in [0, i-1] Within the scope of , eureka j, bring dp[j] + 1 == dp[i], It shows that two increasing subsequences of the same length are found .
So in order to i Increment the number of subsequences for the longest substring at the end You should add j Increment the number of subsequences for the longest substring at the end , namely ：cnt[i] += cnt[j];
class Solution {
public int findNumberOfLIS(int[] nums) {
if(nums.length<=1){
return nums.length ;
}
int [] dp = new int [nums.length] ;
int [] cnt = new int [nums.length] ;
Arrays.fill(dp,1) ;
Arrays.fill(cnt,1) ;
int max = 0 ;
for(int i=1; i<nums.length; i++){
for(int j=0; j<i; j++){
if(nums[i]>nums[j]){
if(dp[j]+1 > dp[i]){
dp[i] = dp[j]+1 ;
cnt[i] = cnt[j] ;
}else if(dp[j]+1==dp[i]){
cnt[i] += cnt[j] ;
}
}
}
max = Math.max(max, dp[i]) ;
}
int ans = 0 ;
for(int i=0; i<dp.length; i++){
if(max==dp[i]){
ans += cnt[i] ;
}
}
return ans ;
}
}
10- A keyboard with only two keys
Topic link ： The title link is here ！！！
Ideas ：1、 If n Is a prime number , So the result is n, Because the least is to copy , Then paste one by one .
2、 If n Is a composite number , Then his result is the sum of the results of the factorization .
such as n by 8 ,
that dp[8] = dp[4]+dp[2]; because ,8 = 42
dp[4] = dp[2]+dp[2]; because ,4 = 22
Because because 2 It's a prime number ,dp[2] = 2;
therefore dp[8] = 6 That's what we're asking for .
class Solution {
public int minSteps(int n) {
int [] dp = new int [n+1] ;
for(int i=2; i<=n; i++){
dp[i] = i ;
for(int j=2;j<=Math.sqrt(i);j++){
if(i%j==0){
dp[i] = dp[j] + dp[i/j] ;
break ;
}
}
}
return dp[n];
}
}
A journey of a thousand miles begins with a single step. , take your time , It will be soon ！！！
边栏推荐
- Caused by:org. gradle. api. internal. plugins . PluginApplicationException: Failed to apply plugin
- Postman核心功能解析-参数化和测试报告
- Black cat takes you to learn UFS protocol Chapter 4: detailed explanation of UFS protocol stack
- 职场进阶指南：大厂人必看书籍推荐
- 全链路压测：构建三大模型
- Summary of the post of "Web Test Engineer"
- Qt：无法定位程序输入点XXXXX于动态链接库。
- Win10 cannot operate (delete, cut) files
- MFC 动态创建的对话框及改变控件的大小和位置
- Mise en œuvre d’une fonction complexe d’ajout, de suppression et de modification basée sur jeecg - boot
猜你喜欢
随机推荐
leetcode 24. Exchange the nodes in the linked list in pairs
记一个基于JEECG-BOOT的比较复杂的增删改功能的实现
sourceInsight中文乱码
Simulation volume leetcode [general] 1062 Longest repeating substring
Resttemplate and feign realize token transmission
黑猫带你学UFS协议第18篇：UFS如何配置逻辑单元（LU Management）
基于JEECG-BOOT的list页面的地址栏参数传递
記一個基於JEECG-BOOT的比較複雜的增删改功能的實現
生物医学英文合同翻译，关于词汇翻译的特点
leetcode 24. 两两交换链表中的节点
Simulation volume leetcode [general] 1218 Longest definite difference subsequence
The whole process realizes the single sign on function and the solution of "canceltoken" of undefined when the request is canceled
黑猫带你学eMMC协议第10篇：eMMC读写操作详解（read ＆ write）
模拟卷Leetcode【普通】1061. 按字典序排列最小的等效字符串
技术分享 | 常见接口协议解析
职场进阶指南：大厂人必看书籍推荐
Database - current read and snapshot read
Isam2 operation process
MFC 动态创建的对话框及改变控件的大小和位置
Cannot create PoolableConnectionFactory (Could not create connection to database server. 错误