当前位置:网站首页>Using matlab to solve the linear optimization problem based on matlab dynamic model of learning notes _11 】 【
Using matlab to solve the linear optimization problem based on matlab dynamic model of learning notes _11 】 【
2022-08-03 23:52:00 【crooked babi】
题目:

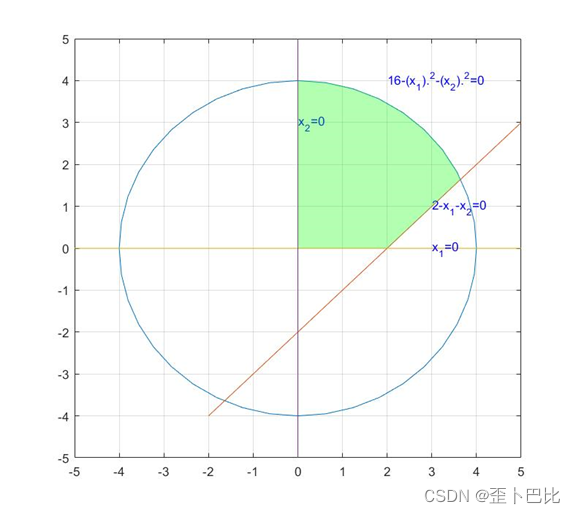
(1)Draw the feasible domain range
question1.m
%% Mark the function
r=4;
a1=0;
a2=0;
theta=0:pi/20:2*pi;
x_1=a1+r*cos(theta);
x_2=a2+r*sin(theta);
plot(x_1,x_2);
hold on
text(2,4,'16-(x_1)^2-(x_2)^2=0','color','b'); %在坐标点(6.8,4)显示x1=7this function line
L2=[-2,-4;5,3];
plot(L2(:,1),L2(:,2));hold on %x2最大值为3
text(3,1,'2-x_1-x_2=0','color','b'); %从点L2(:,1)到点L2(:,2)
L3=[-5,0;5 0];
plot(L3(:,1),L3(:,2));hold on
text(3,0,'x_1=0','color','b')
L4=[0,-5;0,5];
plot(L4(:,1),L4(:,2));
text(0,3,'x_2=0','color','b')
grid on
%% 填充
[X1,X2]=meshgrid(0:0.01:5,0:0.01:5);%draw area
idX1=(X1.*X1+X2.*X2<=16)&(-X2+X1<=2)&(X1>=0)&(X2>=0);
X1=X1(idX1);
X2=X2(idX1);
k=convhull(X1,X2); %计算面积
h=fill(X1(k),X2(k),'g'); %绿色填充
set(h,'edgealpha',0,'facealpha',0.3) %边界,透明度
(2)利用fminconSolve separately without constraints、Constrain the optimal solution
Matlab中的fminconThe function can be used to find the minimum of a constrained nonlinear multivariate function,This is used to find the optimal solution here.
fmincon函数参考:
MatlabSolve nonlinear programs,fmincon函数的用法总结_Xiao Zhu~的博客-CSDN博客_fmincon函数用法
官方帮助文档:https://ww2.mathworks.cn/help/optim/ug/fmincon.html? searchHighlight=fmincon&s_tid=srchtitle_fmincon_1
(2.1)Unconstrained optimal solution
目标函数fun1.m:
%目标函数
function f=fun1(x)
f=(x(1)-2).^2+(x(2)-5).^2
end
Unconstrained optimal solutionquestion2_1.m:
%% Mark the function
r=4;
a1=0;
a2=0;
theta=0:pi/20:2*pi;
x_1=a1+r*cos(theta);
x_2=a2+r*sin(theta);
plot(x_1,x_2);
hold on
text(2,4,'16-(x_1)^2-(x_2)^2=0','color','b'); %在坐标点(6.8,4)显示x1=7this function line
L2=[-2,-4;5,3];
plot(L2(:,1),L2(:,2));hold on %x2最大值为3
text(3,1,'2-x_1-x_2=0','color','b'); %从点L2(:,1)到点L2(:,2)
L3=[-5,0;5 0];
plot(L3(:,1),L3(:,2));hold on
text(3,0,'x_1=0','color','b')
L4=[0,-5;0,5];
plot(L4(:,1),L4(:,2));
text(0,3,'x_2=0','color','b')
grid on
%% 填充
[X1,X2]=meshgrid(0:0.01:5,0:0.01:5);%draw area
idX1=(X1.*X1+X2.*X2<=16)&(-X2+X1<=2)&(X1>=0)&(X2>=0);
X1=X1(idX1);
X2=X2(idX1);
k=convhull(X1,X2); %计算面积
h=fill(X1(k),X2(k),'g'); %绿色填充
set(h,'edgealpha',0,'facealpha',0.3) %边界,透明度
%问题2.1主函数
options=optimset;
x0=[0;0];%给定初值
lb=[0;0];%Function lower bound
ub=[5;5];%upper limit of the function
[x,y]=fmincon('fun1',x0,[],[],[],[],lb,ub)
%加标注
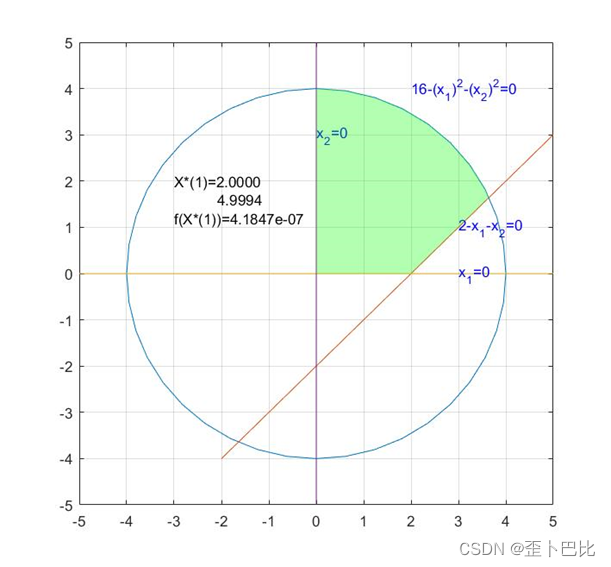
text(-3,2,'X*(1)=2.0000')
text(-2.1,1.6,'4.9994')
text(-3,1.2,'f(X*(1))=4.1847e-07')
(2.2)Constrain the optimal solution
目标函数fun1.m:
%目标函数
function f=fun1(x)
f=(x(1)-2).^2+(x(2)-5).^2
end
Nonlinear constraint functionfun2.m:
%Nonlinear constraint function
function[g,h]=fun2(x)
%matlab中默认g<=0,If it does not correspond, it needs to be reversed
g(1)=-16+x(2).^2+x(1).^2;
g(2)=-2+x(1)+x(2);
h=[];%Use null instead when there is no equality constraint
end
Unconstrained optimal solutionquestion2_2.m:
%% Mark the function
r=4;
a1=0;
a2=0;
theta=0:pi/20:2*pi;
x_1=a1+r*cos(theta);
x_2=a2+r*sin(theta);
plot(x_1,x_2);
hold on
text(2,4,'16-(x_1)^2-(x_2)^2=0','color','b'); %在坐标点(6.8,4)显示x1=7this function line
L2=[-2,-4;5,3];
plot(L2(:,1),L2(:,2));hold on %x2最大值为3
text(3,1,'2-x_1-x_2=0','color','b'); %从点L2(:,1)到点L2(:,2)
L3=[-5,0;5 0];
plot(L3(:,1),L3(:,2));hold on
text(3,0,'x_1=0','color','b')
L4=[0,-5;0,5];
plot(L4(:,1),L4(:,2));
text(0,3,'x_2=0','color','b')
grid on
%% 填充
[X1,X2]=meshgrid(0:0.01:5,0:0.01:5);%draw area
idX1=(X1.*X1+X2.*X2<=16)&(-X2+X1<=2)&(X1>=0)&(X2>=0);
X1=X1(idX1);
X2=X2(idX1);
k=convhull(X1,X2); %计算面积
h=fill(X1(k),X2(k),'g'); %绿色填充
set(h,'edgealpha',0,'facealpha',0.3) %边界,透明度
%问题2.2主函数
options=optimset;
x0=[0;0];%给定初值
lb=[0;0];%Function lower bound
ub=[5;5];%upper limit of the function
[x,y]=fmincon('fun1',x0,[],[],[],[],lb,ub,'fun2')
%加标注
text(-3,2,'X*(2)=0.0000')
text(-2.1,1.6,'2.0000')
text(-3,1.2,'f(X*(2))=13') 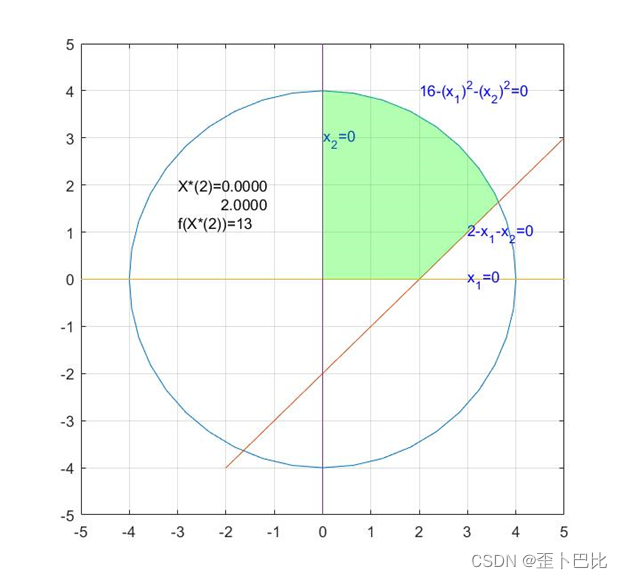
(3)Linearly constrained optimal solution
目标函数fun1.m:
%目标函数
function f=fun1(x)
f=(x(1)-2).^2+(x(2)-5).^2
end
Linear constraint functionfun3.m:
%Linear constraint function
function[g,h]=fun3(x)
%matlab中默认g<=0,If it does not correspond, it needs to be reversed
g(1)=-16+x(2).^2+x(1).^2;
g(2)=-2+x(1)+x(2);
%线性约束条件
h=x(1)-x(2);
end
Linearly constrained optimal solutionquestion3.m:
%% Mark the function
r=4;
a1=0;
a2=0;
theta=0:pi/20:2*pi;
x_1=a1+r*cos(theta);
x_2=a2+r*sin(theta);
plot(x_1,x_2);
hold on
text(2,4,'16-(x_1)^2-(x_2)^2=0','color','b'); %在坐标点(6.8,4)显示x1=7this function line
L2=[-2,-4;5,3];
plot(L2(:,1),L2(:,2));hold on %x2最大值为3
text(3,1,'2-x_1-x_2=0','color','b'); %从点L2(:,1)到点L2(:,2)
L3=[-5,0;5 0];
plot(L3(:,1),L3(:,2));hold on
text(3,0,'x_1=0','color','b')
L4=[0,-5;0,5];
plot(L4(:,1),L4(:,2));
text(0,3,'x_2=0','color','b')
grid on
%% 填充
[X1,X2]=meshgrid(0:0.01:5,0:0.01:5);%draw area
idX1=(X1.*X1+X2.*X2<=16)&(-X2+X1<=2)&(X1>=0)&(X2>=0);
X1=X1(idX1);
X2=X2(idX1);
k=convhull(X1,X2); %计算面积
h=fill(X1(k),X2(k),'g'); %绿色填充
set(h,'edgealpha',0,'facealpha',0.3) %边界,透明度
%问题3主函数
options=optimset;
x0=[0;0];%给定初值
lb=[0;0];%Function lower bound
ub=[5;5];%upper limit of the function
[x,y]=fmincon('fun1',x0,[],[],[],[],lb,ub,'fun3')
%加标注
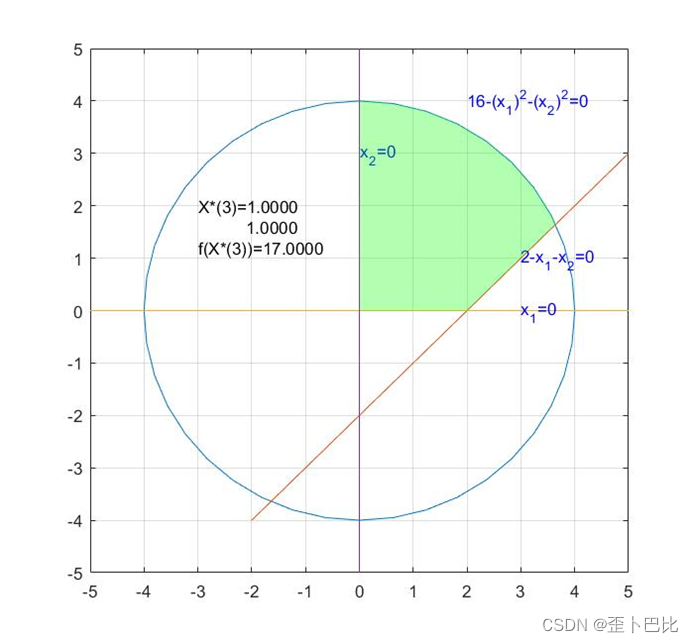
text(-3,2,'X*(3)=1.0000')
text(-2.1,1.6,'1.0000')
text(-3,1.2,'f(X*(3))=17.0000')
边栏推荐
- [2022强网杯] polydiv和gamemaster
- In V8 how arrays (with source code, picture and text easier to understand)
- OpenCV 图像拼接
- sqlnet.ora文件与连接认证方式的小测试
- The world's first mass production, with the most fixed points!How does this AVP Tier1 lead?
- 3D Semantic Segmentation - 2DPASS
- SolidEdge ST8安装教程
- Deep integration of OPC UA and IEC61499 (1)
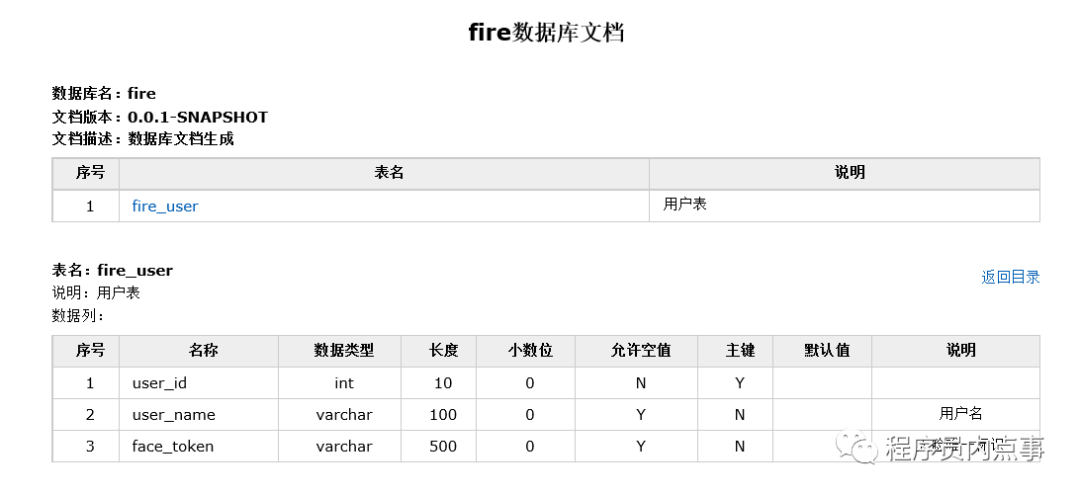
- jav一键生成数据库文档
- 七夕活动浪漫上线,别让网络拖慢和小姐姐的开黑时间
猜你喜欢
随机推荐
简单了解下 TCP,学习握手和挥手以及各种状态到底是怎么样的
Zilliz 2023 Fall Campus Recruitment Officially Launched!
RSS订阅微信公众号初探-feed43
全球首款量产,获定点最多!这家AVP Tier1如何实现领跑?
Unity2021 releases WebGL fog effect disappearing problem
vscode插件设置——Golang开发环境配置
跨域的学习
栈的压入、弹出序列
XSLT – 服务器端概述
汉字风格迁移---结合本地和全局特征学习的中文字体迁移
电子邮件安全或面临新威胁!
corn表达式 具体详解与案例
V8中的快慢数组(附源码、图文更易理解)
Scala基础【正则表达式、框架式开发原则】
ts用法大全
现货白银需要注意八大事项
射频芯片(RFIC)的协议之5G及其调制
Another MySQL masterpiece published by Glacier (send the book at the end of the article)!!
Go编译原理系列7(Go源码调试)
Shell 用法梳理总结