当前位置:网站首页>7 RSA Cryptosystem
7 RSA Cryptosystem
2022-07-04 17:33:00 【Grilled little fat sheep with charcoal...】
RSA introduction
RSA Cryptographic schemes are sometimes called Rivest-Shamir-Adleman Algorithm , It is currently the most widely used asymmetric cryptographic scheme .RSA stay USA( Except for other countries ) The duration of the patent lasts until 2000 year .
RSA Widely applied , But it is often used in practice :
- Encryption of small pieces of data , Especially for key transmission
- digital signature , such as Internet Digital certificate on .
Be careful :RSA Encryption is not intended to replace symmetric passwords , And it's better than things like AES Your password is much slower . This is mainly because RSA( Or other public key algorithms ) Many calculations are involved in the implementation . therefore , The main purpose of encryption features is to securely exchange keys of symmetric passwords ( Key transmission ). actually ,RSA Usually similar to AES Used together with symmetric passwords , Among them, the symmetric password is really used to encrypt a large amount of data .
RSA The underlying one-way function is the integer factorization problem : Multiplication of two large primes is very simple in calculation ( People can do it with pen and paper ), But it is very difficult to factorize the product result .
encryption and decryption
RSA Both encryption and decryption are in the integer ring Z n Z_n Zn To finish the , Modular computing plays a central role .
Suppose you use RSA Encrypt plaintext x x x, And it means x x x The bit string of is Z n = 0 , 1 , . . , n − 1 Z_n = {0,1,..,n-1} Zn=0,1,..,n−1 Internal elements . So plaintext x x x The binary value represented must be less than n n n. For ciphertext , This principle also holds . Encryption with public key and decryption with key can be expressed as :
RSA encryption : Given public key ( n , e ) = k p u b (n, e) = k_{pub} (n,e)=kpub And plaintext x x x, Then the encryption function is : y = e k p u b ( x ) ≡ x e m o d n (1) y = e_{k_{pub}}(x) \equiv x^e mod n \tag 1 y=ekpub(x)≡xemodn(1) among x , y ∈ Z n x,y \in Z_n x,y∈Zn
RSA Decrypt : Given private key d = k p r d = k_{pr} d=kpr And ciphertext y y y, Then the decryption function is : x = d k p r ( y ) ≡ y d m o d n (2) x = d_{k_{pr}}(y) \equiv y^d mod n \tag 2 x=dkpr(y)≡ydmodn(2) among x , y ∈ Z n x,y \in Z_n x,y∈Zn
In the actual x , y , n x, y, n x,y,n and d d d Are very long numbers , Usually it is 1024 Bit or longer . value e Sometimes called crypto index or public index ; Private key d Sometimes called decryption index or secret index . If Alice I want to transmit an encrypted message to Bob, She needs to have Bob The public key (n,e), and Bob Will use his own private key d To decrypt . The next summary will discuss how to generate these three important parameters d,e and n. Now we can discuss RSA Some requirements of cryptosystem ;
- Because the attacker can get the public key ( n , e ) = k p u b (n, e) = k_{pub} (n,e)=kpub, therefore , For a given public key value e e e and n n n, Determine private key d d d In calculation
It must be infeasible . - Due to plaintext x x x It only depends on the modulus n n n Size , So once RSA The number of bits encrypted cannot exceed l l l, among l l l refer to n n n Bit length of .
- Calculation x e m o d n x^e mod n xemodn( That is, encryption ) and y d m o d n y^d mod n ydmodn( Decryption ) It should be relatively simple . This means that we need a method that can quickly calculate the exponent of long integers .
- Given a n n n It should correspond to many keys / Public key for , otherwise , The attacker can launch a brute force attack ( The fact proved that , This requirement is easy to meet ).
Key generation and correctness verification
A salient feature of all asymmetric schemes is , They all have a handshake stage to calculate the public key and private key . Key generation depends on the public key scheme , So it's very complicated . Here are RSA The steps involved in computing public and private keys in cryptosystems .
RSA Key generation :
Output : Public key : k p u b k_{pub} kpub = (n, e) And a private key : k p r = ( d ) k_{pr} = (d) kpr=(d)
- Choose two prime numbers p p p and q q q.
- Calculation n = p ∗ q n = p * q n=p∗q.
- Calculation Φ ( n ) = ( p − 1 ) ∗ ( q − 1 ) \Phi(n) = (p - 1)*(q - 1) Φ(n)=(p−1)∗(q−1).
- Select the public index that meets the following conditions e ∈ { 1 , 2 , . . . . . , Φ ( n ) − 1 } e \in \{1, 2, ....., \Phi(n) - 1\} e∈{ 1,2,.....,Φ(n)−1}: g c d ( e , Φ ( n ) ) = 1 . gcd(e, \Phi(n)) = 1. gcd(e,Φ(n))=1.
- Calculate the private key that meets the following conditions d d d: d ∗ e ≡ 1 m o d Φ ( n ) d*e \equiv 1 mod \Phi(n) d∗e≡1modΦ(n)
step 4 In the request g c d ( e , Φ ( n ) ) = 1 gcd(e, \Phi(n)) = 1 gcd(e,Φ(n))=1 It's to make sure that e e e The existence module of the inverse element of Φ ( n ) \Phi(n) Φ(n), So the private key d d d Always there .
There are two important parts in key generation : Step of selecting two large primes 1 And the steps of calculating the public and private keys 4 and 5. step 1 The generation of prime numbers in is very complex . Using the extended Euclidean algorithm (EEA) The key can be calculated immediately d d d and e e e. In the actual , People usually start with 0 < e < Φ ( n ) 0 < e < \Phi(n) 0<e<Φ(n) Select a public parameter in the range , And e e e The value of must meet the condition g c d ( e , Φ ( n ) ) = 1 gcd(e, \Phi(n))=1 gcd(e,Φ(n))=1. The input parameter n n n and e e e For Euclidean algorithm (EEA) You can get the following relationship : g c d ( Φ ( n ) , e ) = s ⋅ Φ ( n ) + t ⋅ e gcd(\Phi(n), e) = s\cdot\Phi(n) + t\cdot e gcd(Φ(n),e)=s⋅Φ(n)+t⋅e
If g c d ( Φ ( n ) , e ) = 1 gcd(\Phi(n), e) = 1 gcd(Φ(n),e)=1, be e e e Is a valid public key . Parameters obtained by using the extended Euclidean algorithm t t t That is to say e e e Inverse element , So there is : d = t m o d Φ ( n ) . d = t mod \Phi(n). d=tmodΦ(n).
Example :Alice Want to send an encrypted message to Bob. First ,Bob Execution steps 1~5 Calculation RSA Parameters , And send his public key to Alice. Alice Then send the message (x=4) encryption , And will get the ciphertext y Send to Bob. Last ,Bob Decrypt with his own private key y. Be careful : Both private key index and public key index meet the conditions e ∗ d = 3 ∗ 7 ≡ 1 m o d Φ ( n ) e*d = 3*7 \equiv 1 mod\Phi(n) e∗d=3∗7≡1modΦ(n)
Be careful : Both private key index and public key index meet the conditions e ∗ d = 3 ∗ 7 ≡ 1 m o d Φ ( n ) e*d = 3*7 \equiv 1 mod\Phi(n) e∗d=3∗7≡1modΦ(n)
In practice RSA The parameters will be very, very large RSA modulus n Should be at least 1024 Bit length , This makes p and q The bit length of is at least 512. Here is the bit length 1024 Of RSA Examples of parameters .
p = E O D F D 2 C 2. A 288 A C E B C 705 E F A B 30 E 4447541 A 8 C 5447 A 37185 C S A 9 C B 98389 C E 4 D E 19199 A A 3069 B 404 F D 98 C 801568 C B 9170 E B 712 B F 10 B 4955 C E 9 C 9 D C 8 C E 6855 C 612 3 h p=EODFD2C2.A288ACEBC705EFAB30E4447541A8C5447A37185CSA9CB98389CE4DE19199AA3069B404FD98C801568CB9170EB712BF10B4955CE9C9DC8CE6855C6123_h p=EODFD2C2.A288ACEBC705EFAB30E4447541A8C5447A37185CSA9CB98389CE4DE19199AA3069B404FD98C801568CB9170EB712BF10B4955CE9C9DC8CE6855C6123h
q = E B E O F C F 21866 F D 9 A 9 F O D 72 F 7994875 A 8 D 92 E 67 A E E 4 B 515136 B 2 A 778 A 8048 B 149828 A E A 30 B D O B A 34 B 977982 A 3 D 42168 F 594 C A 99 F 3981 D D A B F A B 2369 F 22964011 5 h q=EBEOFCF21866FD9A9FOD72F7994875A8D92E67AEE4B515136B2A778A8048B149828AEA30BDOBA34B977982A3D42168F594CA99F3981DDABFAB2369F229640115_h q=EBEOFCF21866FD9A9FOD72F7994875A8D92E67AEE4B515136B2A778A8048B149828AEA30BDOBA34B977982A3D42168F594CA99F3981DDABFAB2369F229640115h
n = C F 33188211 F D F 6052 B D B B 1 A 37235 E O A B B 5978 A 45 C 71 F D 381491 A D 12 F C 76 D A 0544 C 47568 A C 83 D 855 D 47 C A 8 D 8 A 779579 A B 72 E 635 D O B 0 A A A C 22 D 28341 E 998 E 90 F 82122 A 2 C 06090 F 43 A 37 E 0203 C 2 B 72 E 401 F D 06890 E C 8 E A D 4 F 07 E 686 E 906 F O 1 B 2468 A E 7 B 30 C B D 670255 C 1 F E D E 1 A 2762 C F 4392 C 0759499 C C O A B E C F F 008728 D 9 A 11 A D F h n=CF33188211FDF6052BDBB1A37235EOABB5978A45C71FD381491AD12FC76DA0544C47568AC83D855D47CA8D8A779579AB72E635DOB0AAAC22D28341E998E90F82122A2C06090F43A37E0203C2B72E401FD06890EC8EAD4F07E686E906FO1B2468AE7B30CBD670255C1FEDE1A2762CF4392C0759499CCOABECFF008728D9A11ADF_h n=CF33188211FDF6052BDBB1A37235EOABB5978A45C71FD381491AD12FC76DA0544C47568AC83D855D47CA8D8A779579AB72E635DOB0AAAC22D28341E998E90F82122A2C06090F43A37E0203C2B72E401FD06890EC8EAD4F07E686E906FO1B2468AE7B30CBD670255C1FEDE1A2762CF4392C0759499CCOABECFF008728D9A11ADFh
e = 40 B 028 E 1 E 4 C C F 07537643101 F F 72444 A O B E 1 D 7682 F 1 E D B 553 E 3 A B 4 F 6 D D 8293 C A 1945 D B 12 D 796 A E 9244 D 60565 C 2 E B 692 A 89 B 8881 D 58 D 278562 E D 60066 D D 8211 E 67315 C F 89857167206120405 B O 8 B 54 D 10 D 4 E C 4 E D 4253 C 75 F A 74098 F E 3 F 7 F B 751 F F 5121353 C 554391 E 114 C 85 B 5649725 E 9 B D 5685 D 6 C 9 C T E E D 8 E E 442366353 D C 39 , d = C 21 A 93 E E 75148 D 4 F B F D 77285 D 79 D 6768 C 58 E B F 283743 D 2889 A 395 F 266 C 78 F 4 A 28 E 86 F 545960 C 2 C E O 1 E B 8 A D 5246905163 B 28 D O B 8 B A A B B 959 C C 03 F 4 E C 499186168 A E 9 E D 6 D 88058898907 E 61 C T c C C C 584 D 65 D 801 C F E 32 D F C 983707 F 87 F 5 A A 6 A E 4 B 9 E 77 B 9 C E 630 E 2 C O D F 05841 B 5 E 4984 D 059435 D 7270 D 500514891 F 7 B 77 B 804 B E D 8 1 h e=40B028E1E4CCF07537643101FF72444AOBE1D7682F1EDB553E3AB4F6DD8293CA1945DB12D796AE9244D60565C2EB692A89B8881D58D278562ED60066DD8211E67315CF89857167206120405BO8B54D10D4EC4ED4253C75FA74098FE3F7FB751FF5121353C554391E114C85B5649725E9BD5685D6C9CTEED8EE442366353DC39,d=C21A93EE75148D4FBFD77285D79D6768C58EBF283743D2889A395F266C78F4A28E86F545960C2CEO1EB8AD5246905163B28DOB8BAABB959CC03F4EC499186168AE9ED6D88058898907E61CTcCCC584D65D801CFE32DFC983707F87F5AA6AE4B9E77B9CE630E2CODF05841B5E4984D059435D7270D500514891F7B77B804BED81_h e=40B028E1E4CCF07537643101FF72444AOBE1D7682F1EDB553E3AB4F6DD8293CA1945DB12D796AE9244D60565C2EB692A89B8881D58D278562ED60066DD8211E67315CF89857167206120405BO8B54D10D4EC4ED4253C75FA74098FE3F7FB751FF5121353C554391E114C85B5649725E9BD5685D6C9CTEED8EE442366353DC39,d=C21A93EE75148D4FBFD77285D79D6768C58EBF283743D2889A395F266C78F4A28E86F545960C2CEO1EB8AD5246905163B28DOB8BAABB959CC03F4EC499186168AE9ED6D88058898907E61CTcCCC584D65D801CFE32DFC983707F87F5AA6AE4B9E77B9CE630E2CODF05841B5E4984D059435D7270D500514891F7B77B804BED81h
Verification of correctness :
RSA The core : d k p r ( y ) = d k p r ( e k p u b ( x ) ) ≡ x e d ≡ x d e ≡ x m o d n (3) d_{k_{pr}}(y) = d_{k_{pr}}(e_{k_{pub}}(x)) \equiv {x^e}^d \equiv x^{de} \equiv x mod n \tag 3 dkpr(y)=dkpr(ekpub(x))≡xed≡xde≡xmodn(3) Let's prove that RSA The reason why the scheme is feasible .
prove : It needs to be proved that decryption is the inverse function of encryption , namely d k p r ( e k p u b ( x ) ) = x d_{k_{pr}}(e_{k_{pub}}(x)) = x dkpr(ekpub(x))=x. First, let's explain the construction rules of public key and private key : d ∗ e ≡ 1 m o d Φ ( n ) d*e \equiv 1 mod\Phi(n) d∗e≡1modΦ(n). Using the definition of modulo operator , This expression is equivalent to : d ∗ e ≡ 1 + t ∗ Φ ( n ) d*e \equiv 1 + t*\Phi(n) d∗e≡1+t∗Φ(n)
among t Is an integer . Bring this expression into the equation ( 3 ) (3) (3) Available in : d k p r ( y ) ≡ x d e ≡ x 1 + t ∗ Φ ( n ) ≡ x t ∗ Φ ( n ) ∗ x 1 ≡ ( x Φ ( n ) ) t ∗ x m o d n (4) d_{k_{pr}}(y) \equiv x^{de} \equiv x^{1+t*\Phi(n)} \equiv x^{t*\Phi(n)} * x^1 \equiv (x^{\Phi(n)})^t *x mod n \tag 4 dkpr(y)≡xde≡x1+t∗Φ(n)≡xt∗Φ(n)∗x1≡(xΦ(n))t∗xmodn(4)
That means we need to prove x ≡ ( x Φ ( n ) ) t ∗ x m o d n x \equiv (x^{\Phi(n)})^t *x mod n x≡(xΦ(n))t∗xmodn. According to Euler's theorem , I.e g c d ( x , n ) = 1 gcd( x, n )= 1 gcd(x,n)=1, Then there are 1 ≡ x Φ ( n ) m o d n 1 \equiv x^{\Phi(n)} mod n 1≡xΦ(n)modn, Get a small promotion immediately : 1 ≡ 1 t ≡ ( x Φ ( n ) ) t m o d n (5) 1\equiv 1^t \equiv (x^{\Phi(n)})^t mod n \tag 5 1≡1t≡(xΦ(n))tmodn(5)
among ,t For any integer . This proof process is discussed in two cases :
Case one : g c d ( x , n ) = 1 gcd(x,n)=1 gcd(x,n)=1
In this case, Euler's theorem holds , The equation 5 5 5 Insert 4 4 4, obtain : d k p r ≡ ( x Φ ( n ) ) t ∗ x ≡ 1 ∗ x ≡ x m o d n . Prove BI d_{k_{pr}} \equiv (x^{\Phi(n)})^t *x \equiv 1*x \equiv x mod n. Certificate completion dkpr≡(xΦ(n))t∗x≡1∗x≡xmodn. Prove BI
This part of the proof shows , To and RSA modulus n n n Plaintext value of Coprime x x x for , The decryption function is indeed the inverse of the encryption function .
The second case : g c d ( x , n ) = g c d ( x , p ⋅ q ) ≠ 1 gcd(x,n)= gcd(x, p·q)≠ 1 gcd(x,n)=gcd(x,p⋅q)=1
because p p p and q q q They are all prime numbers , You must have any of the following factors : x = r ∗ p or person x = s ∗ q x = r*p perhaps x = s*q x=r∗p or person x=s∗q
among r , s r,s r,s All are integers , And meet r < q and s < p r<q and s<p r<q and s<p. In order not to lose generality , hypothesis x = r ⋅ p x=r·p x=r⋅p, Then you can get g c d ( x , q ) = 1 gcd(x, q)=1 gcd(x,q)=1. The following form of Euler's theorem still holds : 1 ≡ 1 t ≡ ( x Φ ( q ) ) t m o d q 1\equiv 1^t \equiv (x^{\Phi(q)})^t mod q 1≡1t≡(xΦ(q))tmodq
among t For any positive integer . Let's see next ( x Φ ( n ) ) t (x^{\Phi(n)})^t (xΦ(n))t: ( x Φ ( n ) ) t ≡ ( x ( p − 1 ) ( q − 1 ) ) t ≡ ( ( x Φ ( q ) ) t ) p − 1 ≡ 1 p − 1 = 1 m o d q . (x^{\Phi(n)})^t \equiv (x^{(p-1)(q-1)})^t \equiv ((x^{\Phi(q)})^t)^{p-1} \equiv 1^{p-1} = 1 mod q. (xΦ(n))t≡(x(p−1)(q−1))t≡((xΦ(q))t)p−1≡1p−1=1modq.
Use the definition of modulo operators , This expression is equivalent to : ( x Φ ( n ) ) t = 1 + u ∗ q , (x^{\Phi(n)})^t = 1 + u*q, (xΦ(n))t=1+u∗q,
among , u u u Is any integer . Multiply this equation by x x x Available : x ∗ ( x Φ ( n ) ) t = x + x ∗ u ∗ q = x + ( r ∗ p ) ∗ u ∗ q = x + r ∗ u ∗ ( p ∗ q ) = x + r ∗ u ∗ n x*(x^{\Phi(n)})^t = x + x*u*q= x + (r*p)*u*q= x + r * u * (p*q)=x + r * u * n x∗(xΦ(n))t=x+x∗u∗q=x+(r∗p)∗u∗q=x+r∗u∗(p∗q)=x+r∗u∗n
namely x ∗ ( x Φ ( n ) ) t = x m o d n (6) namely x*(x^{\Phi(n)})^t = x mod n \tag 6 namely x∗(xΦ(n))t=xmodn(6)
Put the equation 6 6 6 Put it into the equation 4 4 4 You can get the desired result : d k p r = ( x Φ ( n ) ) t ∗ x ≡ x m o d n . Prove BI d_{k_{pr}} = (x^{\Phi(n)})^t*x\equiv x mod n. Certificate completion dkpr=(xΦ(n))t∗x≡xmodn. Prove BI
Reference material :《 In simple terms, cryptography 》–Christof Paar,Jan Pelzl Writing
边栏推荐
- How to choose one plus 10 pro and iPhone 13?
- Firewall basic transparent mode deployment and dual machine hot standby
- Oppo Xiaobu launched Obert, a large pre training model, and promoted to the top of kgclue
- ARTS_20220628
- R语言plotly可视化:plotly可视化互相重叠的直方图(historgram)、并在直方图的顶部边缘使用geom_rug函数添加边缘轴须图Marginal rug plots
- R语言plotly可视化:plotly可视化多分类变量小提琴图(multiple variable violin plot in R with plotly)
- 51 single chip microcomputer temperature alarm based on WiFi control
- 公司要上监控,Zabbix 和 Prometheus 怎么选?这么选准没错!
- 解决el-input输入框.number数字输入问题,去掉type=“number“后面箭头问题也可以用这种方法代替
- PingCode 性能测试之负载测试实践
猜你喜欢
Perfectly integrated into win11 style, Microsoft's new onedrive client is the first to see

It's too convenient. You can complete the code release and approval by nailing it!
VSCode修改缩进不成功,一保存就缩进四个空格
PingCode 性能测试之负载测试实践
【云原生】服务网格是什么“格”?
To sort out messy header files, I use include what you use

leetcode:421. The maximum XOR value of two numbers in the array

完美融入 Win11 风格,微软全新 OneDrive 客户端抢先看
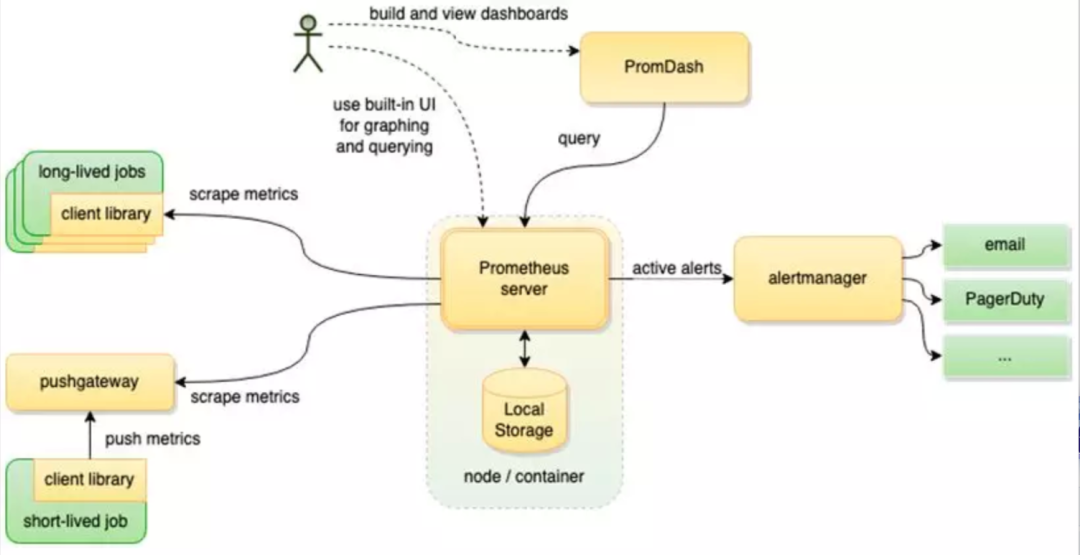
The company needs to be monitored. How do ZABBIX and Prometheus choose? That's the right choice!

Kunming Third Ring Road Closure project will pass through these places. Is there one near your home?
随机推荐
The company needs to be monitored. How do ZABBIX and Prometheus choose? That's the right choice!
整理混乱的头文件,我用include what you use
网页游戏引擎
Display opencv drawn pictures on MFC picture control control
leetcode刷题目录总结
Great Wall Securities security does not open a securities account
The 18th IET AC / DC transmission International Conference (acdc2022) was successfully held online
Using win10 scheduling task program to automatically run jar package at fixed time
The Ministry of human resources and Social Security announced the new construction occupation
新享科技发布小程序UniPro小优 满足客户移动办公场景
R language plot visualization: plot visualizes overlapping histograms and uses geom at the top edge of the histogram_ The rug function adds marginal rug plots
NFT流动性市场安全问题频发—NFT交易平台Quixotic被黑事件分析
2022年国内云管平台厂商哪家好?为什么?
Firewall basic transparent mode deployment and dual machine hot standby
[acwing] 58 weeks 4489 Longest subsequence
动态规划股票问题对比
[Acwing] 58周赛 4490. 染色
The test experience "tortured" by the PMP test is worth your review
Which domestic cloud management platform manufacturer is good in 2022? Why?
【HCIA持续更新】网络管理与运维