当前位置:网站首页>Binary search tree (features)
Binary search tree (features)
2022-07-07 16:36:00 【Joey Liao】
List of articles
First ,BST Everyone should be familiar with the characteristics of :
- about BST Each node of
node, The values of the left subtree nodes are higher thannodeIt's worth less , The values of the right subtree nodes are greater thannodeIt's worth a lot . - about BST Each node of node, Its left subtree and right subtree are BST.
Binary search trees are not complicated , But I think it can be regarded as half of the data structure field , Based on the direct BST The data structure of is AVL Trees , Red and black trees and so on , Has the property of self-equilibrium , Can provide logN Level addition, deletion, query and modification efficiency ; also B+ Trees , Structures such as line segment trees are based on BST The idea to design .
From the perspective of doing algorithm problems BST, Except for its definition , Another important property :BST The middle order traversal result of is ordered ( Ascending ).
in other words , If you enter a tree BST, The following code can BST The values of each node in the are printed in ascending order :
void traverse(TreeNode root) {
if (root == null) return;
traverse(root.left);
// Middle order traversal code position
print(root.val);
traverse(root.right);
}
Search for the first K Small elements
Force to buckle 230 topic 「 Binary search tree K Small elements 」
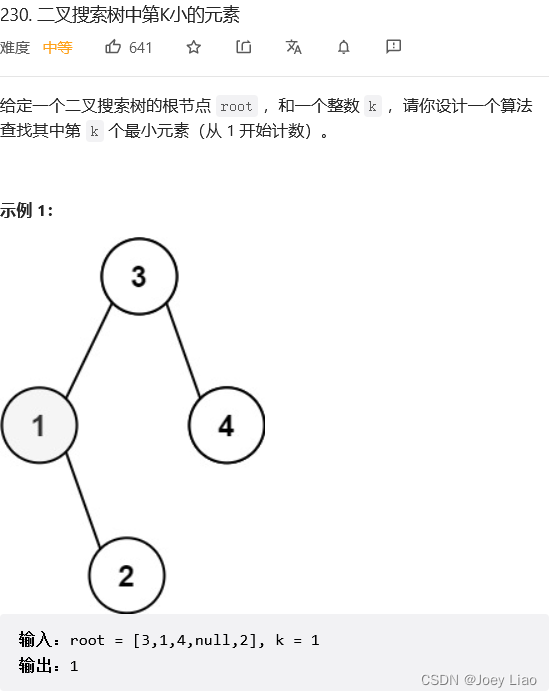
, A direct idea is to sort in ascending order , Then find the second k Two elements .BST The middle order traversal of is actually the result of ascending sorting , Find the first k An element is certainly not difficult .
int kthSmallest(TreeNode root, int k) {
// utilize BST Middle order ergodic property of
traverse(root, k);
return res;
}
// Records of the results
int res = 0;
// Record the ranking of the current element
int rank = 0;
void traverse(TreeNode root, int k) {
if (root == null) {
return;
}
traverse(root.left, k);
/* Middle order traversal code position */
rank++;
if (k == rank) {
// Find No k Small elements
res = root.val;
return;
}
/*****************/
traverse(root.right, k);
}
If we follow the method we just mentioned , utilize 「BST Medium order traversal is the result of ascending sorting 」 This nature , Every time you look for k Small elements should be traversed once in the middle order , The worst time complexity is O(N),N yes BST Number of nodes .
Need to know BST The nature is very awesome , Improved self balance like red black tree BST, Add, delete, check and change are O(logN) Complexity , Let you count a number k Minor elements , The time complexity should be O(N), A little inefficient .
So back to the question , Want to find the second k Small elements , Or find a ranking of k The elements of , If you want to achieve logarithmic complexity , The key is that each node has to know its own ranking .
For example, you asked me to find a ranking of k The elements of , The current node knows that it ranks No m, Then I can compare m and k Size :
If m == k, Obviously, I found the second k Elements , Just return to the current node .
If k < m, That means ranking No k The element of is in the left subtree , So you can search the left subtree k Elements .
If k > m, That means ranking No k The element of is in the right subtree , So you can go to the right subtree to search for the second k - m - 1 Elements .
In this way, the time complexity can be reduced to O(logN) 了 .
that , How to let each node know its ranking ?
That's what we said before , Additional information needs to be maintained in the binary tree node . Each node needs to record , How many nodes does the binary tree with its own root have .
in other words , We TreeNode The fields in should be as follows :
class TreeNode {
int val;
// The total number of nodes in the tree with this node as the root
int size;
TreeNode left;
TreeNode right;
}
With size Field , Plus BST The property that nodes are small on the left and large on the right , For each node node You can go through node.left Deduce node Ranking , So as to achieve the logarithmic algorithm we just mentioned .
Of course ,size Fields need to be properly maintained when adding or deleting elements , The force buckle provides TreeNode It's not size Of this field , So we can only use this problem BST The feature of middle order traversal realizes , But the optimization idea we mentioned above is BST Common operations for , It is still necessary to understand .
BST Transform cumulative tree
Force to buckle 538 Title and 1038 The questions are all this question Convert binary search tree to accumulation tree
In fact, it is the middle order traversal of binary tree , use sum To record the current cumulative sum
/** * Definition for a binary tree node. * public class TreeNode { * int val; * TreeNode left; * TreeNode right; * TreeNode() {} * TreeNode(int val) { this.val = val; } * TreeNode(int val, TreeNode left, TreeNode right) { * this.val = val; * this.left = left; * this.right = right; * } * } */
class Solution {
int sum=0;
public TreeNode convertBST(TreeNode root) {
traverse(root);
return root;
}
public void traverse(TreeNode root){
if(root==null){
return ;
}
traverse(root.right);
sum+=root.val;
root.val=sum;
traverse(root.left);
}
}
边栏推荐
- 两类更新丢失及解决办法
- hellogolang
- MySQL数据库基本操作-DQL-基本查询
- [summary of knowledge] summary of notes on using SVN in PHP
- PHP has its own filtering and escape functions
- 2022 the 4th China (Jinan) International Smart elderly care industry exhibition, Shandong old age Expo
- Laravel 服务提供者实例教程 —— 创建 Service Provider 测试实例
- Laravel5.1 Routing - routing packets
- PHP实现执行定时任务的几种思路详解
- Communication mode between application program and MATLAB
猜你喜欢
Pycharm terminal enables virtual environment

Unity3d click events added to 3D objects in the scene
Balanced binary tree (AVL)
Multiplication in pytorch: mul (), multiply (), matmul (), mm (), MV (), dot ()
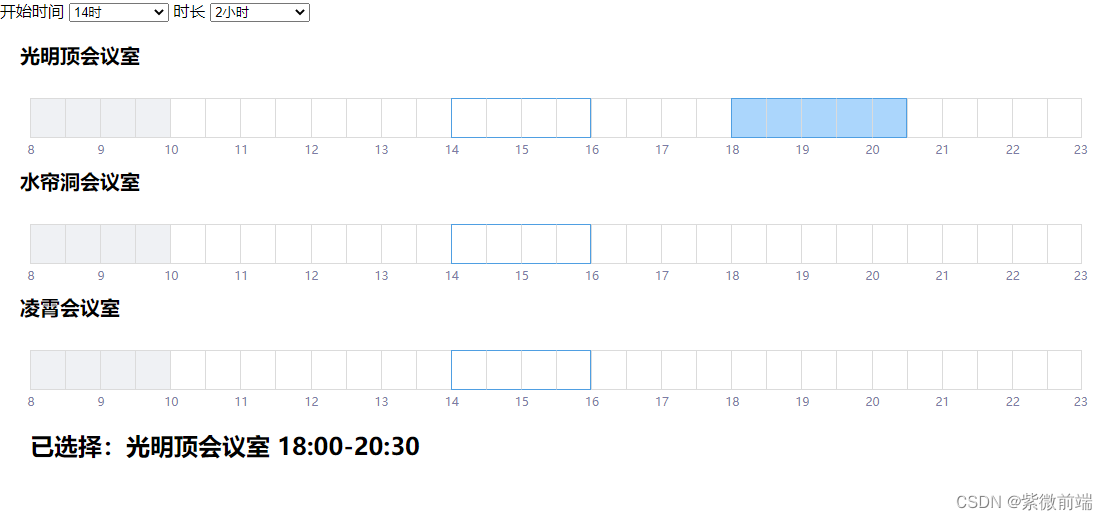
Imitate the choice of enterprise wechat conference room
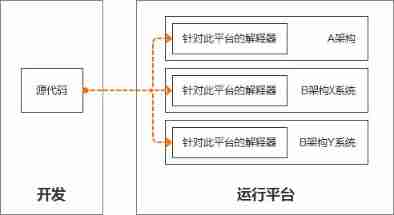
The difference and working principle between compiler and interpreter

网关Gateway的介绍与使用
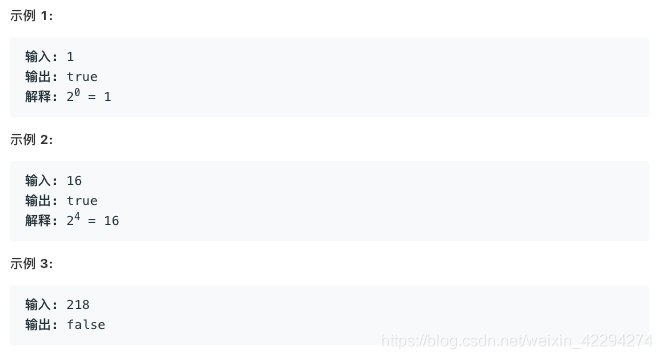
Power of leetcode-231-2
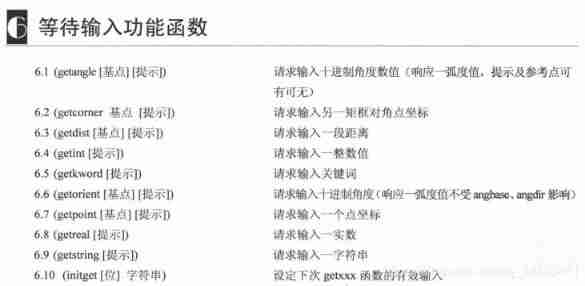
AutoLISP series (1): function function 1
MySQL数据库基本操作-DQL-基本查询
随机推荐
01tire+ chain forward star +dfs+ greedy exercise one
Opportunity interview experience summary
PHP实现执行定时任务的几种思路详解
[flower carving experience] 15 try to build the Arduino development environment of beetle esp32 C3
Cesium(3):ThirdParty/zip. js
Power of leetcode-231-2
Tidb cannot start after modifying the configuration file
谈谈 SAP iRPA Studio 创建的本地项目的云端部署问题
Find tags in prefab in unity editing mode
Bidding announcement: Fujian Rural Credit Union database audit system procurement project (re bidding)
水平垂直居中 方法 和兼容
Laravel 中config的用法
thinkphp3.2.3中设置路由,优化url
Opencv configuration 2019vs
Xcode Revoke certificate
Imitate the choice of enterprise wechat conference room
[Android -- data storage] use SQLite to store data
Pycharm terminal enables virtual environment
Continuous creation depends on it!
如何快速检查钢网开口面积比是否符合 IPC7525