[linear algebra] determinant of order 1.3 n

First study the third-order determinant ：

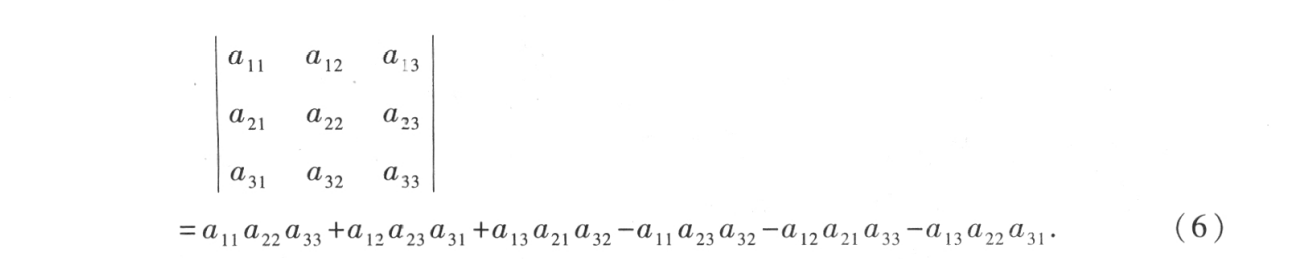
  Easy to see ：

1) (6) Each term of the formula is exactly the product of three elements , These three elements are on different lines 、 Different columns .

Therefore, any item can be written as .

The first subscript of each item here ( Line mark ) Arrange in standard order 123.

namely ：

And the second subscript of each item ( Column mark ), Line up p1p2p3, It is 1,2,3 An arrangement of three numbers . This arrangement shares 6 Kind of , Corresponding (6) Formula Co containing 6 term .

2） The sign of each item is compared with the arrangement of column marks

The trinomial column with a positive sign is 123,231,312.( Are even permutations ）

  The arrangement of three column labels with minus sign is 132,213,321.( They are all odd permutations ）

The calculation shows that The first three permutations are even permutations , The last three permutations are all odd permutations .

therefore , The sign of each item can be expressed as , among t by Column label arrangement Of Reverse order number .

All in all , The third-order determinant can be written as ：

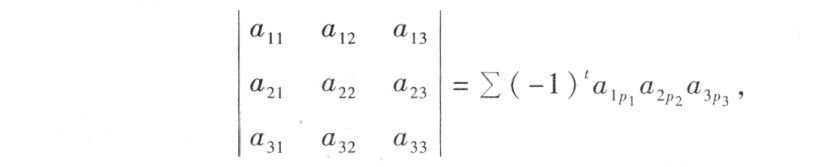
  among t To arrange p1p2p3 In reverse order ,∑ It means to sum all sorts of permutations .

Generalize determinant to general form ：

Equipped with Number , Line up n That's ok n A list of numbers ：

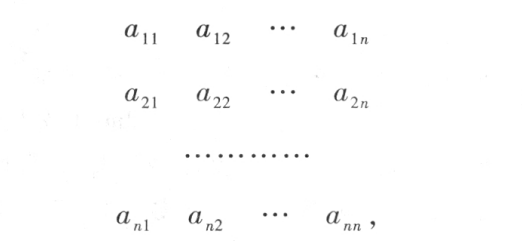
  Make the table in different rows and columns n The product of numbers , And prefixed with symbols , Get the shape of

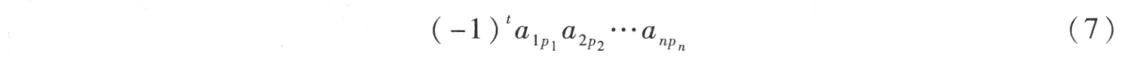
  among p1p2...pn For natural Numbers 1,2...,n An arrangement of ,t The number in reverse order for this arrangement .

Because this arrangement has n! individual , Thus, it is shaped like (7) The terms of formula have n! term .

All this n! Algebraic sum of terms

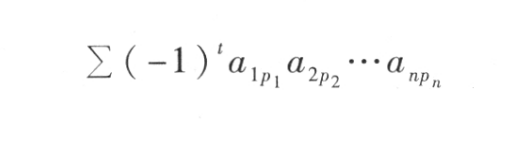
  be called n Step determinant , Write it down as ：

  A shorthand det(), Among them For determinant (i,j) element .

When n=1 when , First order determinant |a|=a, Be careful not to confuse with the absolute value number .

All the elements below are diagonals 0 The determinant of is called Upper triangular determinant .

The elements above the main diagonal are 0 The determinant of is called Lower triangular determinant .

The elements above and below the main diagonal are 0 The determinant of is called Diagonal determinants .