当前位置:网站首页>2020 CCPC 威海 - A. Golden Spirit(思维),D. ABC Conjecture(大数分解 / 思维)
2020 CCPC 威海 - A. Golden Spirit(思维),D. ABC Conjecture(大数分解 / 思维)
2022-07-05 20:08:00 【小酒窝.】
A. Golden Spirit
题意
一道河上有座桥,两岸分别有 n n n 个人。
现这 2 n 2n 2n 个人都要到对岸去,游玩至少 x x x 分钟后回到原来的河岸。
要帮助这 2 n 2n 2n 个人渡河。
每次渡河最多带一个人,时间为 t t t。
问,满足所有人的要求最少花多少时间?
思路
在最理想的情况下,帮助者没有一点时间浪费,当他把所有人都运到其对应对岸后,立即可以将该河岸首次运过来的人运回去。
但是可能甚至首次运过来的人都没有到所需的游玩时间,所以帮助者可能需要等待。
假设有河岸 A 和 B,起初帮助者在河岸 A,那么将所有人分别运到其对应河岸后,帮助者位于河岸 A。
这时就面临一个问题,帮助者是继续在该河岸 A 等待首次运到河岸 A 的人,还是自己到对岸 B 去等首次运到河岸 B 的人?
前者所需时间为:运输所有人的时间 4nt + 等待时间(最少游玩时间 x - 首人已经游玩时间 2nt-2t)
后者所需时间为:运输所有人的时间 4nt + 自己到对岸的时间 t + 等待时间(最少游玩时间 x - 首人已经游玩时间 2nt + t - t)
因为不确定两者的大小,所以需要将两者取 min。
Code:
#include<bits/stdc++.h>
using namespace std;
const int N = 200010, mod = 1e9+7;
int T, n, m;
int a[N];
signed main(){
cin >> T;
while(T--)
{
int x, t;
cin >> n >> x >> t;
int ans1 = 4*n*t + t + max(0ll, x - (2*n*t + t - t));
int ans2 = 4*n*t + max(0ll, x - (2*n*t - 2*t));
cout << min(ans1, ans2) << endl;
}
return 0;
}
经验
- 当不确定所有情况哪种最优时,需要将所有情况的答案取最小值;
- 有些情况下不要写太多的 if else,而是尽量将其抽象到一种大情况中。
D. ABC Conjecture
题意
给定一个数 c,判断是否存在 a, b 满足下面条件:
- a + b = c
- abc 的所有质因子的乘积 不超过 c
1 ≤ c ≤ 1 0 18 1≤c≤10^{18} 1≤c≤1018
思路
打表发现:能够满足的 c 分解的所有质因子都只出现一次。
所以对于给定的 c,只需判断其是否存在出现不止一次的质因子即可。
但是 c 很大,而常用的分解质因数代码的时间复杂度为O(logn),会超时。
所以需要用特殊的方法来做。
方法1:
- 按照分解质因数的思路将 c 的所有不超过 1e6 的质因数分解,判断其个数是否出现两次及以上。
- 如果 1e6 内的质因数筛完之后发现 c 不为1,那么说明 c 还存在最多两个大于 1e6 的质因数(假设存在3个,那么相乘后大于1e18,矛盾)。
我们不是要求出这两个质因子,而只是想判断这两个质因子是否相同。所以将最后的 c 取根号取整,判断其是否为平方数。如果是平方数,那么最后的两个质因子就是相同的。
Code1:
#include<bits/stdc++.h>
using namespace std;
#define int long long
#define endl '\n'
const int N = 1000010, mod = 1e9+7;
int T, n, m;
int a[N];
int f[N];
int prim[N];
int cnt;
int maxa = 1e6;
signed main(){
Ios;
cin >> T;
while(T--)
{
cin >> n;
int flag = 0;
for(int i=2; i<=maxa; i++)
{
if(n % i == 0)
{
int cnt = 0;
while(n % i == 0) n /= i, cnt++;
if(cnt != 1) flag = 1;
}
}
if(n != 1){
int sq = sqrt(n);
if(sq * sq == n) flag = 1;
}
if(flag) cout << "yes\n";
else cout << "no\n";
}
return 0;
}
方法2
直接上大数质因子分解模板,判断是否有质因子出现两次及以上。
Code:
#include <bits/stdc++.h>
using namespace std;
typedef long long ll;
ll pr;
ll pmod(ll a, ll b, ll p) {
return (a * b - (ll)((long double)a / p * b) * p + p) % p; }
ll gmod(ll a, ll b, ll p)
{
ll res = 1;
while (b)
{
if (b & 1)
res = pmod(res, a, p);
a = pmod(a, a, p);
b >>= 1;
}
return res;
}
inline ll gcd(ll a, ll b)
{
if (!a)
return b;
if (!b)
return a;
int t = __builtin_ctzll(a | b);
a >>= __builtin_ctzll(a);
do
{
b >>= __builtin_ctzll(b);
if (a > b)
{
ll t = b;
b = a, a = t;
}
b -= a;
} while (b);
return a << t;
}
bool Miller_Rabin(ll n)
{
if (n == 46856248255981ll || n < 2)
return false;
if (n == 2 || n == 3 || n == 7 || n == 61 || n == 24251)
return true;
if (!(n & 1) || !(n % 3) || !(n % 61) || !(n % 24251))
return false;
ll m = n - 1, k = 0;
while (!(m & 1))
k++, m >>= 1;
for (int i = 1; i <= 20; ++i)
{
ll a = rand() % (n - 1) + 1, x = gmod(a, m, n), y;
for (int j = 1; j <= k; ++j)
{
y = pmod(x, x, n);
if (y == 1 && x != 1 && x != n - 1)
return 0;
x = y;
}
if (y != 1)
return 0;
}
return 1;
}
ll Pollard_Rho(ll x)
{
ll n = 0, m = 0, t = 1, q = 1, c = rand() % (x - 1) + 1;
for (ll k = 2;; k <<= 1, m = n, q = 1)
{
for (ll i = 1; i <= k; ++i)
{
n = (pmod(n, n, x) + c) % x;
q = pmod(q, abs(m - n), x);
}
t = gcd(x, q);
if (t > 1)
return t;
}
}
map<long long, int> m;
void fid(ll n)
{
if (n == 1)
return;
if (Miller_Rabin(n))
{
pr = max(pr, n);
m[n]++;
return;
}
ll p = n;
while (p >= n)
p = Pollard_Rho(p);
fid(p);
fid(n / p);
}
int main()
{
int T;
ll n;
scanf("%d", &T);
while (T--)
{
m.clear();
scanf("%lld", &n);
pr = 0;
fid(n);
int flag = 0;
for (map<long long, int>::iterator c = m.begin(); c != m.end(); c++)
if(c->second != 1) flag = 1;
if(flag) printf("yes\n");
else printf("no\n");
}
return 0;
}
打表代码:
#include<bits/stdc++.h>
using namespace std;
#define int long long
#define pb push_back
#define endl '\n'
const int N = 200010, mod = 1e9+7;
int T, n, m;
int a[N];
vector<int> v, v1, v2;
set<int> st, s;
void div(int x, vector<int> &v)
{
v.clear();
for(int i=2;i<=x/i;i++)
{
if(x%i == 0)
{
while(x%i == 0) x /= i;
v.push_back(i);
}
}
if(x != 1) v.push_back(x);
}
signed main(){
for(int i=1;i<=1000;i++)
{
st.clear();
div(i, v);
for(int x : v) st.insert(x);
int flag = 0;
for(int j=1;j<i;j++)
{
s = st;
int x = j, y = i - x;
div(x, v1);
div(y, v2);
for(int x : v1) s.insert(x);
for(int x : v2) s.insert(x);
int sum = 1;
for(int x : s){
sum *= x;
}
if(sum < i){
flag = 1;
break;
}
}
if(!flag){
cout << i << ":";
for(int x : st) cout << x << " ";
cout << endl;
}
}
return 0;
}
经验
- 没有思路的时候先打个表;
- 判断一个数是不是平方数,sqrt(n)要取整之后再相乘;
- 大数质因子分解模板。
边栏推荐
- Leetcode brush questions: binary tree 18 (largest binary tree)
- Four methods of random number generation | random | math | threadlocalrandom | securityrandom
- Debezium series: modify the source code to support drop foreign key if exists FK
- Where is the operation of new bonds? Is it safer and more reliable to open an account
- 95后阿里P7晒出工资单:狠补了这个,真香...
- CADD课程学习(7)-- 模拟靶点和小分子相互作用 (半柔性对接 AutoDock)
- 14. Users, groups, and permissions (14)
- 深度學習 卷積神經網絡(CNN)基礎
- 无卷积骨干网络:金字塔Transformer,提升目标检测/分割等任务精度(附源代码)...
- 【数字IC验证快速入门】6、Questasim 快速上手使用(以全加器设计与验证为例)
猜你喜欢
Redis cluster simulated message queue

Oracle-表空间管理
【数字IC验证快速入门】8、数字IC中的典型电路及其对应的Verilog描述方法
Leetcode skimming: binary tree 17 (construct binary tree from middle order and post order traversal sequence)

After 95, Alibaba P7 published the payroll: it's really fragrant to make up this
【数字IC验证快速入门】2、通过一个SoC项目实例,了解SoC的架构,初探数字系统设计流程
leetcode刷题:二叉树13(相同的树)
third-party dynamic library (libcudnn.so) that Paddle depends on is not configured correctl
Two pits exported using easyexcel template (map empty data columns are disordered and nested objects are not supported)
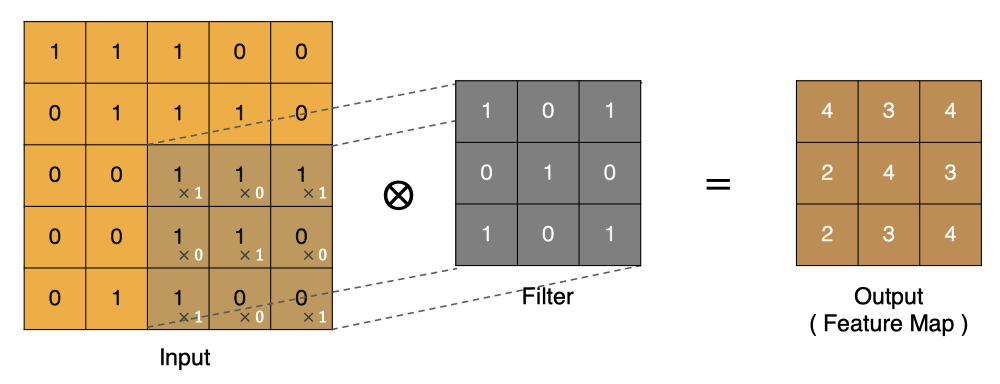
Base du réseau neuronal de convolution d'apprentissage profond (CNN)
随机推荐
Is it safe for Anxin securities to open an account online?
leetcode刷题:二叉树18(最大二叉树)
No matter how busy you are, you can't forget safety
Wechat applet regular expression extraction link
微信小程序正则表达式提取链接
Unity编辑器扩展 UI控件篇
如何安全快速地从 Centos迁移到openEuler
【数字IC验证快速入门】3、数字IC设计全流程介绍
A solution to PHP's inability to convert strings into JSON
JVMRandom不可设置种子|问题追溯|源码追溯
Debezium series: modify the source code to support drop foreign key if exists FK
Debezium series: PostgreSQL loads the correct last submission LSN from the offset
【c语言】快速排序的三种实现以及优化细节
Zero cloud new UI design
Let's talk about threadlocalinsecurerandom
Two pits exported using easyexcel template (map empty data columns are disordered and nested objects are not supported)
Debezium series: parsing the default value character set
浅浅的谈一下ThreadLocalInsecureRandom
Cocos2d-x项目总结中的一些遇到的问题
Debezium series: record the messages parsed by debezium and the solutions after the MariaDB database deletes multiple temporary tables