shiyou的数值分析作业

想让APP和微信一样，可以流畅运行小程序？ | 体验即送大疆、华为、樱桃键盘！>>>

突然想起来可以做做数值分析的作业，于是把室友的数值分析作业拿过来练手，写一篇博客分享一下。其实我这个菜鸟把程序写复杂了，有很多可以简化的地方，由于本菜鸟其它作业还没写完，就不去简化了，大家可以自行改正啦。

---

数值分析上机题

首先说一下自己的疑惑，对于第一题python怎么实现对ln(x)直接调用求导呢？是直接用泰勒展开后对多项式求导吗？第二题是用Newton迭代法，要求出迭代初始值在什么范围可以得到收敛解，这里如果用迭代程序去实现的话也会比较麻烦，那有没有更好的方法去求解呢？希望有大神可以帮忙留言解惑，多谢。

一、题1——对数的近似求解

1.题目描述

**题目：**这里偷下懒，直接贴出原题的截图吧。

2.python实现

不多BB，程序在这：

import numpy as np
from sympy import * #用于求导积分等科学计算
import math as m

x = Symbol('x')#x 变量
t = Symbol('t')
y1 = 1/(1+x) #公式
y2 = -1/(1+x) #公式
y3 = 2/(1-x**2) #公式

def func(m):
    res = m
    for j in range(1,m):
        res *= j
    return res

def ln_Tyalor(y):
    Tl_expr = y * (t-x)
    for i in range(1, 10):
        fac = func(i+1)
        f_n = diff(y, x, i)
        Tl_expr += (f_n / fac)*(t-x)**(i+1)
    return Tl_expr.subs({
   
    x:0})

#print(ln_Tyalor(y1))
sexpr1 = ln_Tyalor(y1)
sexpr2 = ln_Tyalor(y2)
sexpr3 = ln_Tyalor(y3)
A = sexpr1.subs({
   
    t:1}).evalf()
B = sexpr2.subs({
   
    t:-1/2}).evalf()
C = sexpr3.subs({
   
    t:1/3}).evalf()
print('ln2的值：', m.log(2, m.e))
print('方程ln(1+x)的10阶泰勒展开计算结果为：', A,'\n','估计误差为：', abs(m.log(2, m.e)-A))
print('方程ln(1/(1+x))的10阶泰勒展开计算结果为：', B,'\n','估计误差为：', abs(m.log(2, m.e)-B))
print('方程ln((1+x)/(1-x))的10阶泰勒展开计算结果为：', C,'\n','估计误差为：', abs(m.log(2, m.e)-C))

3.输出结果

ln2的值： 0.6931471805599453
方程ln(1+x)的10阶泰勒展开计算结果为： 0.645634920634921 
 估计误差为： 0.0475122599250246
方程ln(1/(1+x))的10阶泰勒展开计算结果为： 0.693064856150793 
 估计误差为： 8.23244091517905e-5
方程ln((1+x)/(1-x))的10阶泰勒展开计算结果为： 0.693146047390827 
 估计误差为： 1.13316911820593e-6

一、题2——方程求根的Newton法

1.题目描述

**题目：**还是截图方便。

2.python实现

这个程序写的不好，由于写完后，exp（）函数老是报错说：整型数据不是exp对象的属性，改了之后也没实现自己的想法，那就这样吧，没时间啦，大家自己搞吧。。。。。

import numpy as np
from sympy import * #用于求导积分等科学计算
import math as m

def f(x):
    return 3*x**2 - m.exp(x) #该方程有3个根，初步估计在[-1,0],[0,1],[3,4]

def fdiff(x):
    return 6*x - m.exp(x)

def g(x):
    return 6*x - m.exp(x)#该方程有3个根，初步估计在[0,1],[2,3]

def gdiff(x):
    return 6 - m.exp(x)

a = float(input('请输入计算区间的下界a(浮点型）: '))
b = float(input('请输入计算区间的上界b(浮点型）: '))
c = float(input('请输入迭代初始值(浮点型）: '))
n = input('请输入要求解的函数，f代表f(x),g代表g(x): ')

if n =='f':

    if f(a) * f(b)> 0:
        print('在此区间内函数有多个根或者无根')
    elif f(a) * f(b) == 0:
        print('f(a) = ', '%f'%f(a))
        print('f(b) = ', '%f'%f(b))
    else:
        fcount = 0
        y = c - f(c) / fdiff(c)
        while (abs(c - y) >= 0.5e-9) & (fdiff(c) != 0):
            x2 = c - f(c) / fdiff(c)
            y = c
            c = x2
            fcount += 1
        print('函数给出的求根区间是：', [a, b])
        print("函数f(x）的Newton迭代次数：%f,函数f(x）的迭代计算所得的根为:%.8f"%(fcount,c))

elif n =='g':

    if g(a) * g(b)> 0:
        print('在此区间内函数有多个根或者无根')
    elif g(a) * g(b) == 0:
        print('g(a) = ', '%f'%g(a))
        print('g(b) = ', '%f'%g(b))
    else:
        gcount = 0
        y = c - g(c) / gdiff(c)
        while (abs(c - y) >= 0.5e-9) & (gdiff(c) != 0):
            x2 = c - g(c) / gdiff(c)
            y = c
            c = x2
            gcount += 1
        print('函数给出的求根区间是：', [a, b])
        print("函数g(x）的Newton迭代次数：%f,函数g(x）的迭代计算所得的根为:%.8f"%(gcount,c))

3.输出结果

这里说明一下，输入的[a, b]是你想判断该区间有没有根；c是迭代初始值；f代表f（x)，g代表g(x)；这几个参数请自己输入。

请输入计算区间的下界a(浮点型）: -1.0
请输入计算区间的上界b(浮点型）: 4.0
请输入迭代初始值(浮点型）: -3.0
请输入要求解的函数，f代表f(x),g代表g(x): f
函数给出的求根区间是： [-1.0, 4.0]
函数f(x）的Newton迭代次数：7.000000,函数f(x）的迭代计算所得的根为:-0.45896227

一、题3——Hilbert矩阵的条件数

1.题目描述

**题目：**你懂的。

2.python实现

import numpy as np
import matplotlib.pyplot as plt
plt.rcParams['font.sans-serif'] = ['simhei']

n = int(input('请输入Hilbert方阵的最大维数:' ))
def max_sum_rows(X):
    sum_row_list1 = []
    for i in range(len(X)):
        count = 0
        for j in range(len(X)):
            count += abs(X[i][j])
        sum_row_list1.append(count)
    return max(sum_row_list1)

inf_fanshu = []
Hilbert_cond = []
for i in range(1, n+1):
    X = 1./(np.arange(1, i+1) + np.arange(0, i)[:, np.newaxis])
    invX = np.linalg.inv(X)
    a1 = max_sum_rows(invX)
    a2 = max_sum_rows(X)
    inf_fanshu.append(a2)
    H_cond = a1 * a2
    Hilbert_cond.append(H_cond)

#计算10,20……100的无穷范数条件数
Hilbert_cond_test = []
for j in range(n):
    if (j+1)%10 == 0:
        Hilbert_cond_test.append(Hilbert_cond[j])
print('生成维数从10,20……100的Hilbert矩阵的行范数条件数：\n', Hilbert_cond_test)

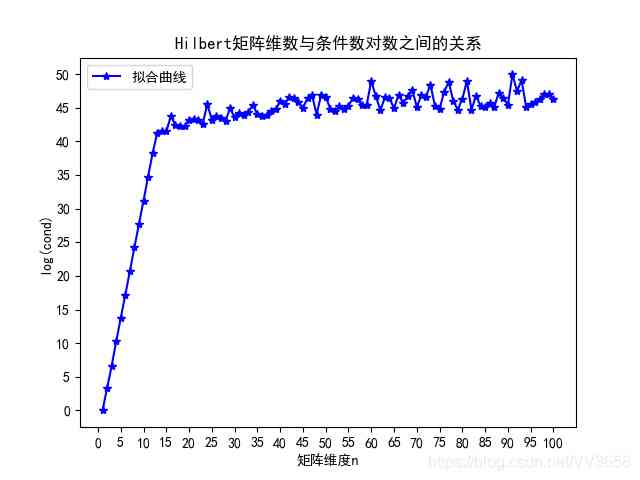
plt.title('Hilbert矩阵维数与条件数对数之间的关系')
plt.plot((list(range(1,n+1))), np.log(Hilbert_cond),c ='b', marker='*',label='拟合曲线')
plt.legend()
plt.xlabel('矩阵维度n')
plt.xticks(np.arange(0, n+1, 5))
plt.yticks(np.arange(0, 55, 5))
plt.ylabel('log(cond)')
plt.show()

#求解Hilbert方程的解和对应的无穷条件数
r_A_A_acc_list = []
r_B_list = []
r_cond = []
r_B_cond = []
for i in range(1,n+1):
    A = np.ones((i,1))*1
    X = 1. / (np.arange(1, i + 1) + np.arange(0, i)[:, np.newaxis])
    B = X@A
    A_acc = np.linalg.inv(X)@B
    r_A_A_acc = A - A_acc
    r_B = B - X @ A_acc
    r_A_A_acc_list.append(r_A_A_acc)
    r_B_list.append(r_B)
    r_cond.append(abs(r_A_A_acc[:]).max()) #x-x_acc的无穷范数
    r_B_cond.append(abs(r_B[:]).max())#b-Hx_acc的无穷范数

print('维数为10,50,100时的x-x_acc计算结果：\n', r_A_A_acc_list[9] ,r_A_A_acc_list[49] , r_A_A_acc_list[99])
print('维数为10,50,100时的b-Hx_acc计算结果：\n',r_B_list[9], r_B_list[49], r_B_list[99])
print('维数为10,50,100时的x-x_acc矩阵的无穷条件数计算结果：\n', r_cond[9], r_cond[49], r_cond[99])
print('维数为10,50,100时的b-Hx_acc矩阵的无穷条件数计算结果：\n', r_B_cond[9], r_B_cond[49], r_B_cond[99])

3.输出结果

输入你想计算的Hilbert方阵的最大维数就行，其它交给程序。

请输入Hilbert方阵的最大维数:100
生成维数从10,20……100的Hilbert矩阵的行范数条件数：
 [35356847610517.12, 6.008376652086652e+18, 8.396589803249062e+18, 9.491653209312077e+19, 1.7763569870536153e+20, 1.9301974218850052e+21, 3.9847310708042826e+19, 1.3450693870678838e+20, 5.444272740462528e+19, 1.3244131088115743e+20]

这里输出的图片如下：

这里是第四问的输出结果：

维数为10,50,100时的x-x_acc计算结果：
 [[-2.54168641e-04]
 [ 2.16242671e-03]
 [-5.54656982e-03]
 [ 5.08880615e-03]
 [ 9.15527344e-04]
 [-4.02832031e-03]
 [ 1.46484375e-03]
 [ 4.88281250e-04]
 [-1.22070312e-04]
 [-6.10351562e-05]] [[ 8.01768149e+02]
 [ 3.33788188e+04]
 [-1.59537467e+06]
 [ 1.98594595e+07]
 [-1.31128704e+08]
·················
 [-4.04700000e+03]
 [ 6.50000000e+01]
 [-2.25000000e+01]
 [ 3.30000000e+01]
 [-7.30000000e+01]] [[ 1.05255071e+04]
 [-1.31934071e+06]
 [ 4.56146227e+07]
 [-7.42843201e+08]
 [ 6.95696228e+09]
 [-4.13027099e+10]
 ················
 [-4.79000000e+02]
 [ 5.12100000e+03]
 [-1.91900000e+03]
 [ 1.77000000e+02]]
 维数为10,50,100时的b-Hx_acc计算结果：
 [[1.27400597e-05]
 [2.15601768e-05]
 [1.73587501e-05]
 [1.43429989e-05]
 [1.23056437e-05]
 [1.08422262e-05]
 [9.72981380e-06]
 [8.84754702e-06]
 [8.12566450e-06]
 [7.52108032e-06]] [[ 13.49201973]
 [  7.51301334]
 [ -4.25849823]
 [-14.49468816]
 [-21.55733783]
 [-26.46828937]
···············
 [-28.3616173 ]
 [-28.05340528]
 [-27.75051982]
 [-27.45291237]] [[-22.02594035]
 [-22.62163018]
 [-20.05848154]
 [-17.70284588]
 [-16.01064382]
 [-14.79343569]
···············
 [ -3.83066178]
 [ -3.79759674]
 [ -3.76500334]
 [ -3.73286444]
 [ -3.70119334]]

维数为10,50,100时的x-x_acc矩阵的无穷条件数计算结果：
 0.00554656982421875 7824513409.0 998313040247.0
维数为10,50,100时的b-Hx_acc矩阵的无穷条件数计算结果：
 2.1560176801216357e-05 38.404672581436365 22.62163018366762

结语

分享出来仅供相互学习，相互探讨，如所写有误，请多多包涵。希望能相互学习，共同进步，欢迎各位大佬留言评论。