当前位置:网站首页>[combinatorics] recursive equation (general solution structure of recursive equation with multiple roots | linear independent solution | general solution with multiple roots | solution example of recu
[combinatorics] recursive equation (general solution structure of recursive equation with multiple roots | linear independent solution | general solution with multiple roots | solution example of recu
2022-07-03 17:06:00 【Programmer community】
List of articles
- One 、 Linear independent solution
- Two 、 There is a general solution under the double root
- Two 、 There is a general solution under the double root
- 3、 ... and 、 Examples of solving recursive equations with multiple roots
- Four 、 Summary of the solution of recurrence equation formula
One 、 Linear independent solution
Linear independent solution :
If
q
q
q It's a recursive equation
e
e
e Heavy characteristic root , be
q
n
,
n
q
n
,
n
2
q
n
,
⋯
,
n
e
−
1
q
n
q^n , nq^n , n^2q^n , \cdots , n^{e-1}q^n
qn,nqn,n2qn,⋯,ne−1qn
It's a recursive equation Linearly independent solutions ;
e
e
e Is the multiplicity of the characteristic root ;
Two 、 There is a general solution under the double root
q
1
,
q
2
,
⋯
,
q
t
q_1, q_2, \cdots , q_t
q1,q2,⋯,qt It's a recursive equation Unequal characteristic roots , Yes
t
t
t Unequal characteristic roots ,
q
i
q_i
qi The multiplicity of is
e
i
e_i
ei ,
A characteristic root
q
i
q_i
qi , Its repeatability is
e
i
e_i
ei , The Characteristic root Corresponding Items in the general solution yes :
H
i
(
n
)
=
(
c
i
1
+
c
i
2
n
+
⋯
+
c
i
e
i
n
e
i
−
1
)
q
i
n
H_i(n) = (c_{i1} + c_{i2}n + \cdots + c_{ie_i}n^{e_i - 1})q_i^n
Hi(n)=(ci1+ci2n+⋯+cieinei−1)qin
Of the above general solution Coefficient , contain
e
i
e_i
ei The item , this
e
i
e_i
ei Beyond the constants of the terms
n
n
n The power is taken from
0
0
0 To
e
i
−
1
e_i - 1
ei−1 ,
The general solution of the recurrence equation is :
H
(
n
)
=
∑
i
=
1
t
H
i
(
n
)
H(n) = \sum\limits_{i=1}^tH_i(n)
H(n)=i=1∑tHi(n)
Two 、 There is a general solution under the double root
The general solution form under the double root is listed :
1 . Number of characteristic elements :
q
1
,
q
2
,
⋯
,
q
t
q_1, q_2, \cdots , q_t
q1,q2,⋯,qt Is the characteristic root of recursive equation , Unequal characteristic roots
t
t
t ;
2 . according to Characteristic root Write the items in the general solution
H
i
(
n
)
H_i(n)
Hi(n) : Characteristic root
q
i
q_i
qi , Repeatability
e
i
e_i
ei , among
i
i
i The value is
0
0
0 To
t
t
t; The first
i
i
i The general solution term corresponding to characteristic roots , Write it down as
H
i
(
n
)
H_i(n)
Hi(n) ;
- ( 1 ) form : Coefficient term multiply
q
i
n
q_i^n
qin ;
- ( 2 ) Coefficient term :
- ① Number : Yes
e
i
e_i
ei term ; Number of coefficient terms , Is the repeatability of the characteristic root ;
- ② form : constant multiply
n
n
n
e
i
−
1
n^{e_i-1}
nei−1 , Here you are
e
i
e_i
ei It's a constant ;
- 1 > constant : The constant subscript is from
c
i
1
c_{i1}
ci1 To
c
i
e
i
c_{ie_i}
1
1
1 To
e
i
e_i
ei ;
ciei , The right part of the subscript is
- 2 >
n
n
0
0
0 To
e
i
−
1
e_i - 1
ei−1 ;
n Power of power : The value of power is from
- 3 > Suggested arrangement : constant and The next power , It's best to arrange them from small to large , Constant subscript And
n
n
n The power of Difference between
1
1
1 ;
n Power of power ; Such as :
- 1 > constant : The constant subscript is from
- ① Number : Yes
- ( 3 ) General explanation
i
i
H
i
(
n
)
=
(
c
i
1
+
c
i
2
n
+
⋯
+
c
i
e
i
n
e
i
−
1
)
q
i
n
H_i(n) = (c_{i1} + c_{i2}n + \cdots + c_{ie_i}n^{e_i - 1})q_i^n
Hi(n)=(ci1+ci2n+⋯+cieinei−1)qin
i term :
3 . Write a general solution :
- ( 1 ) Number of general solutions : Number of characteristic elements
t
t
t ;
- ( 2 ) General solution composition : The general solution term corresponding to each characteristic root , Put it all together , Is the complete general solution ;
- ( 3 ) final result :
H
(
n
)
=
∑
i
=
1
t
H
i
(
n
)
H(n) = \sum\limits_{i=1}^tH_i(n)
H(n)=i=1∑tHi(n)
3、 ... and 、 Examples of solving recursive equations with multiple roots
Solution :
1 . Characteristic equation :
( 1 ) The standard form of recurrence equation : Write the recurrence equation Standard form , All items are to the left of the equal sign , On the right is
0
0
0 ;
The recursive equation is now in standard form ;
( 2 ) Number of terms of characteristic equation : determine Number of terms of characteristic equation , And The recurrence equation has the same number of terms ;
5
5
5 term ;
( 3 ) The characteristic equation is sub idempotent : The highest power is Number of terms of characteristic equation
−
1
-1
−1 , Lowest power
0
0
0 ;
x
x
x The power of is from
0
0
0 To
4
4
4 ;
( 4 ) Write There is no coefficient The characteristic equation of ;
x
4
+
x
3
+
x
2
+
x
+
1
=
0
x^4 + x^3 + x^2 + x + 1 = 0
x4+x3+x2+x+1=0
( 5 ) The coefficients of the recursive equation will be deduced bit by bit Copy Into the characteristic equation ;
x
4
+
x
3
−
3
x
2
−
5
x
−
2
=
0
x^4 + x^3 - 3x^2 -5 x -2 = 0
x4+x3−3x2−5x−2=0
2 . Solution characteristic root : take Characteristic equation Characteristic root Work it out ,
x
=
−
b
±
b
2
−
4
a
c
2
a
x = \cfrac{-b \pm \sqrt{b^2 - 4ac}}{2a}
x=2a−b±b2−4ac
The characteristic root solved is
−
1
,
−
1
,
−
1
,
2
-1, -1, -1, 2
−1,−1,−1,2 ;
3 . Construct the general solution of recurrence equation :
( 1 ) No double root : structure
c
1
q
1
n
+
c
2
q
2
n
+
⋯
+
c
k
q
k
n
c_1q_1^n + c_2q_2^n + \cdots + c_kq_k^n
c1q1n+c2q2n+⋯+ckqkn Linear combination of forms , This linear combination is the of recursive equations general solution ;
( 2 ) Double root : Refer to the following “ The general solution form under the double root is listed ” Content ;
The situation here belongs to the situation with double roots , Refer to the following solution :
Heavy root
−
1
-1
−1 Items need to be in accordance with The general solution rule of multiple roots Write ;
Non double root
2
2
2 , May, in accordance with the The general form Write , namely
c
4
2
n
c_42^n
c42n ,
c
4
c_4
c4 Is constant ,
4
4
4 This is the number
4
4
4 A characteristic root ;
The heavy root is
−
1
-1
−1 , The repeatability is
3
3
3 ;
H
1
(
n
)
H_1(n)
H1(n) Represents the heavy root item , This item is composed of Coefficient term multiply
(
−
1
)
n
(-1)^n
(−1)n form ;
There is
3
3
3 term ; The form of each coefficient term is constant multiply
n
n
n The power of ;
Constant use
c
1
,
c
2
,
c
3
c_1, c_2, c_3
c1,c2,c3 Express ,
n
n
n The power of The value is
0
0
0 To
2
2
2 ( Number of coefficient terms
−
1
-1
−1 ) ;
Write
−
1
-1
−1 The general solution term corresponding to the characteristic root :
H
1
(
n
)
=
(
c
1
+
c
2
n
+
c
3
n
2
)
(
−
1
)
n
H_1(n) = (c_1 + c_2n + c_3n^2)(-1)^n
H1(n)=(c1+c2n+c3n2)(−1)n
The complete general solution is :
H
(
n
)
=
(
c
1
+
c
2
n
+
c
3
n
2
)
(
−
1
)
n
+
c
4
2
n
H(n) = (c_1 + c_2n + c_3n^2)(-1)^n + c_42^n
H(n)=(c1+c2n+c3n2)(−1)n+c42n
4 . Find the constant in the general solution :
( 1 ) Substitute the initial values to obtain the equations : Substitute the initial value of the recursive equation into the general solution , obtain
k
k
k individual
k
k
k Finite element equations , adopt Solve the equations , obtain Constants in general solutions ;
{
(
c
1
+
0
c
2
+
0
2
c
3
)
(
−
1
)
0
+
2
0
c
4
=
F
(
0
)
=
1
(
c
1
+
1
c
2
+
1
2
c
3
)
(
−
1
)
1
+
2
1
c
4
=
F
(
1
)
=
0
(
c
1
+
2
c
2
+
2
2
c
3
)
(
−
1
)
2
+
2
2
c
4
=
F
(
2
)
=
1
(
c
1
+
3
c
2
+
3
2
c
3
)
(
−
1
)
3
+
2
3
c
4
=
F
(
3
)
=
2
\begin{cases} ( c_1 + 0c_2 + 0^2c_3 )(-1)^0 + 2^0c_4 = F(0) = 1 \\\\ ( c_1 + 1c_2 + 1^2c_3 )(-1)^1 + 2^1c_4 = F(1) = 0 \\\\ ( c_1 + 2c_2 + 2^2c_3 )(-1)^2 + 2^2c_4 = F(2) = 1 \\\\ ( c_1 + 3c_2 + 3^2c_3 )(-1)^3 + 2^3c_4 = F(3) = 2 \end{cases}
⎩⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎨⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎧(c1+0c2+02c3)(−1)0+20c4=F(0)=1(c1+1c2+12c3)(−1)1+21c4=F(1)=0(c1+2c2+22c3)(−1)2+22c4=F(2)=1(c1+3c2+32c3)(−1)3+23c4=F(3)=2
It is reduced to :
{
c
1
+
c
4
=
1
−
c
1
−
c
2
−
c
3
+
2
c
4
=
0
c
1
+
2
c
2
+
4
c
3
+
4
c
4
=
1
−
c
1
−
3
c
2
−
9
c
3
+
8
c
4
=
2
\begin{cases} c_1 +c_4= 1 \\\\ -c_1 - c_2 - c_3 + 2c_4 = 0 \\\\ c_1 +2 c_2 +4 c_3 + 4c_4= 1 \\\\ -c_1 - 3c_2 - 9c_3 + 8c_4= 2 \end{cases}
⎩⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎨⎪⎪⎪⎪⎪⎪⎪⎪⎪⎪⎧c1+c4=1−c1−c2−c3+2c4=0c1+2c2+4c3+4c4=1−c1−3c2−9c3+8c4=2
Solve the above
4
4
4 The constant value is :
c
1
=
7
9
,
c
2
=
−
1
3
,
c
3
=
0
,
c
4
=
2
9
c_1 = \cfrac{7}{9}, c_2 = -\cfrac{1}{3}, c_3 = 0, c_4 = \cfrac{2}{9}
c1=97,c2=−31,c3=0,c4=92
( 2 ) Substitute constants to obtain the general solution : Substitute constants into the general solution , You can get the final solution of the recursive equation ;
Complete general solution :
H
(
n
)
=
7
9
(
−
1
)
n
−
1
3
(
−
1
)
n
+
2
9
2
n
H(n) = \cfrac{7}{9} (-1)^n - \cfrac{1}{3} (-1)^n + \cfrac{2}{9}2^n
H(n)=97(−1)n−31(−1)n+922n
Four 、 Summary of the solution of recurrence equation formula
The whole process of solving recursive equations :
1 . Characteristic equation :
( 1 ) The standard form of recurrence equation : Write the recurrence equation Standard form , All items are to the left of the equal sign , On the right is
0
0
0 ;
( 2 ) Number of terms of characteristic equation : determine Number of terms of characteristic equation , And The recurrence equation has the same number of terms ;
( 3 ) The characteristic equation is sub idempotent : The highest power is Number of terms of characteristic equation
−
1
-1
−1 , Lowest power
0
0
0 ;
( 4 ) Write There is no coefficient The characteristic equation of ;
( 5 ) The coefficients of the recursive equation will be deduced bit by bit Copy Into the characteristic equation ;
2 . Solution characteristic root : take Characteristic equation Characteristic root Work it out ,
x
=
−
b
±
b
2
−
4
a
c
2
a
x = \cfrac{-b \pm \sqrt{b^2 - 4ac}}{2a}
x=2a−b±b2−4ac
3 . Construct the general solution of recurrence equation :
( 1 ) No double root : structure
c
1
q
1
n
+
c
2
q
2
n
+
⋯
+
c
k
q
k
n
c_1q_1^n + c_2q_2^n + \cdots + c_kq_k^n
c1q1n+c2q2n+⋯+ckqkn Linear combination of forms , This linear combination is the of recursive equations general solution ;
( 2 ) Double root : Refer to the following “ The general solution form under the double root is listed ” Content ;
4 . Find the constant in the general solution :
( 1 ) Substitute the initial values to obtain the equations : Substitute the initial value of the recursive equation into the general solution , obtain
k
k
k individual
k
k
k Finite element equations , adopt Solve the equations , obtain Constants in general solutions ;
( 2 ) Substitute constants to obtain the general solution : Substitute constants into the general solution , You can get the final solution of the recursive equation ;
The general solution form under the double root is listed :
1 . Number of characteristic elements :
q
1
,
q
2
,
⋯
,
q
t
q_1, q_2, \cdots , q_t
q1,q2,⋯,qt Is the characteristic root of recursive equation , Unequal characteristic roots
t
t
t ;
2 . according to Characteristic root Write the items in the general solution
H
i
(
n
)
H_i(n)
Hi(n) : Characteristic root
q
i
q_i
qi , Repeatability
e
i
e_i
ei , among
i
i
i The value is
0
0
0 To
t
t
t; The first
i
i
i The general solution term corresponding to characteristic roots , Write it down as
H
i
(
n
)
H_i(n)
Hi(n) ;
- ( 1 ) form : Coefficient term multiply
q
i
n
q_i^n
qin ;
- ( 2 ) Coefficient term :
- ① Number : Yes
e
i
e_i
ei term ; Number of coefficient terms , Is the repeatability of the characteristic root ;
- ② form : constant multiply
n
n
n
e
i
−
1
n^{e_i-1}
nei−1 , Here you are
e
i
e_i
ei It's a constant ;
- 1 > constant : The constant subscript is from
c
i
1
c_{i1}
ci1 To
c
i
e
i
c_{ie_i}
1
1
1 To
e
i
e_i
ei ;
ciei , The right part of the subscript is
- 2 >
n
n
0
0
0 To
e
i
−
1
e_i - 1
ei−1 ;
n Power of power : The value of power is from
- 3 > Suggested arrangement : constant and The next power , It's best to arrange them from small to large , Constant subscript And
n
n
n The power of Difference between
1
1
1 ;
n Power of power ; Such as :
- 1 > constant : The constant subscript is from
- ① Number : Yes
- ( 3 ) General explanation
i
i
H
i
(
n
)
=
(
c
i
1
+
c
i
2
n
+
⋯
+
c
i
e
i
n
e
i
−
1
)
q
i
n
H_i(n) = (c_{i1} + c_{i2}n + \cdots + c_{ie_i}n^{e_i - 1})q_i^n
Hi(n)=(ci1+ci2n+⋯+cieinei−1)qin
i term :
3 . Write a general solution :
- ( 1 ) Number of general solutions : Number of characteristic elements
t
t
t ;
- ( 2 ) General solution composition : The general solution term corresponding to each characteristic root , Put it all together , Is the complete general solution ;
- ( 3 ) final result :
H
(
n
)
=
∑
i
=
1
t
H
i
(
n
)
H(n) = \sum\limits_{i=1}^tH_i(n)
H(n)=i=1∑tHi(n)
Recurrence equation -> Characteristic equation -> Characteristic root -> general solution -> Substitute the initial value to find the general solution constant
边栏推荐
- What material is sa537cl2 equivalent to in China? Sa537cl2 corresponding material
- 聊聊接口优化的几个方法
- Define a structure fraction to represent a fraction, which is used to represent fractions such as 2/3 and 5/6
- Network security web penetration technology
- 关于学习Qt编程的好书精品推荐
- 2022.02.14_ Daily question leetcode five hundred and forty
- ucore概述
- MySQL Basics
- CC2530 common registers
- Redis: operation commands for list type data
猜你喜欢
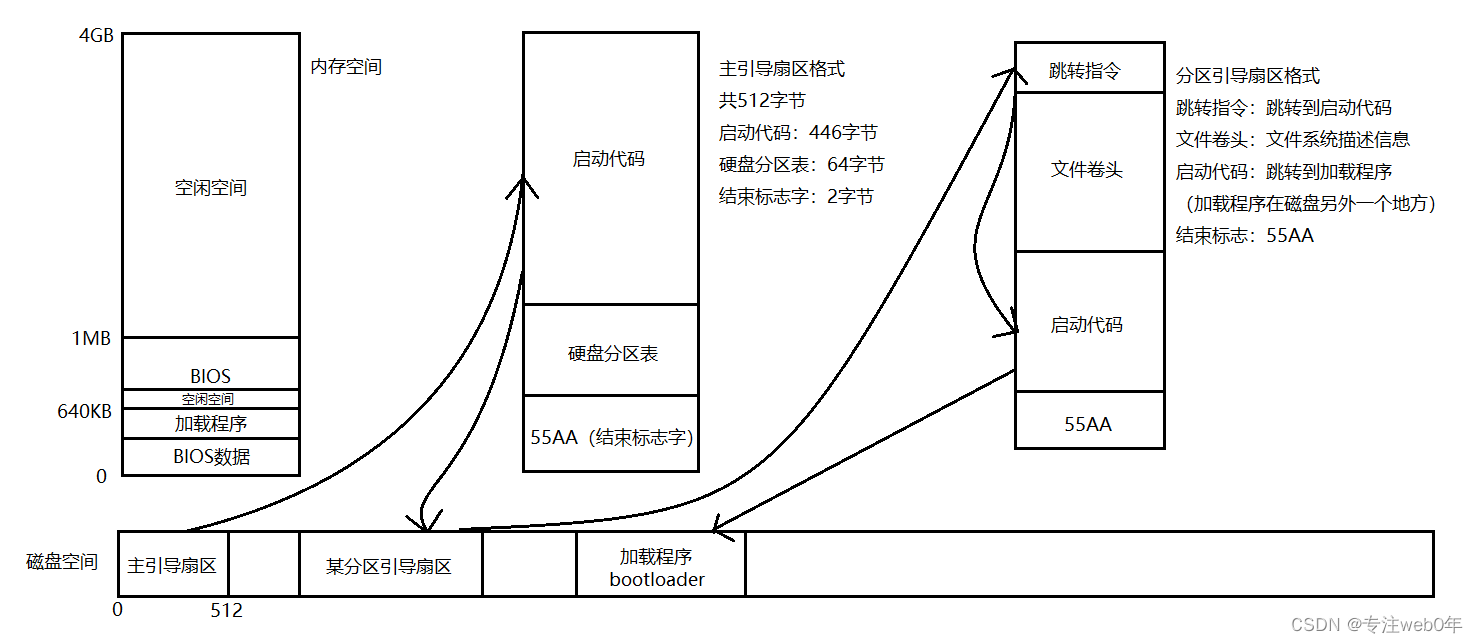
UCORE overview

What material is 13crmo4-5 equivalent to in China? 13crmo4-5 chemical composition 13crmo4-5 mechanical properties
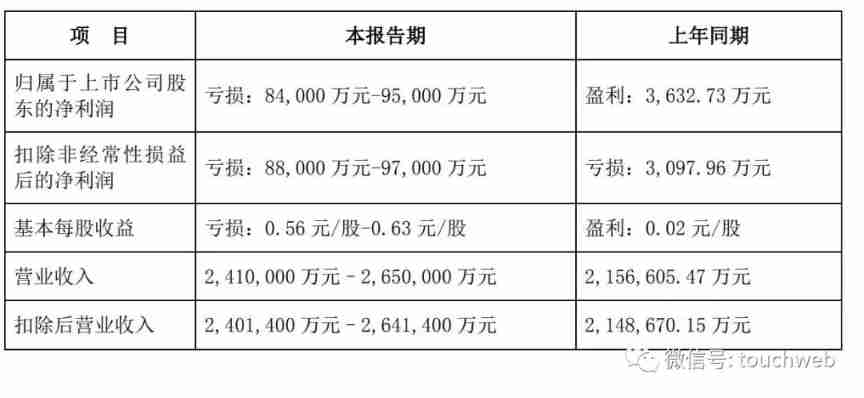
Shentong express expects an annual loss of nearly 1billion

CC2530 common registers for crystal oscillator settings
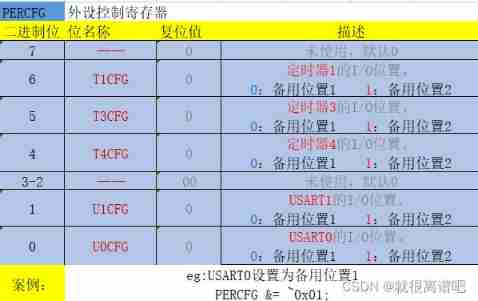
CC2530 common registers for serial communication

Leetcode: lucky number in matrix

Daily code 300 lines learning notes day 10

What material is sa537cl1? Sa537cl1 corresponds to the national standard material
![[try to hack] active detection and concealment technology](/img/43/d48f851268fec566ce0cc83bd9557e.png)
[try to hack] active detection and concealment technology
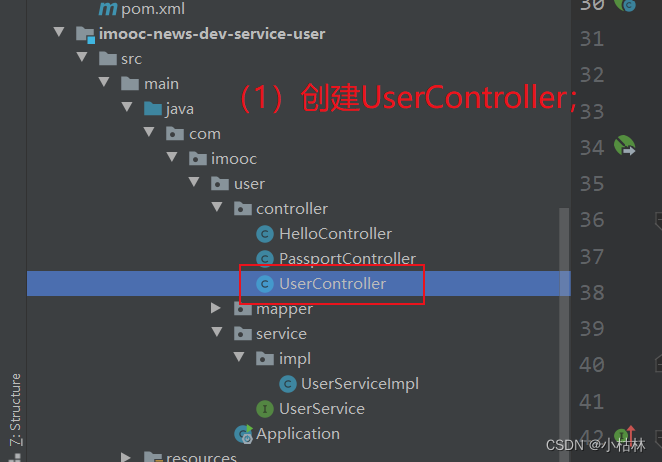
29:第三章:开发通行证服务:12:开发【获得用户账户信息,接口】;(使用VO类包装查到的数据,以符合接口对返回数据的要求)(在多处都会用到的逻辑,在Controller中可以把其抽成一个共用方法)
随机推荐
[combinatorics] polynomial theorem (polynomial theorem | polynomial theorem proof | polynomial theorem inference 1 item number is the number of non negative integer solutions | polynomial theorem infe
Capacités nécessaires à l'analyse des données
图之深度优先搜索
Thread pool: the most common and error prone component of business code
[combinatorics] recursive equation (characteristic equation and characteristic root | example of characteristic equation | root formula of monadic quadratic equation)
29: Chapter 3: develop Passport Service: 12: develop [obtain user account information, interface]; (use VO class to package the found data to meet the requirements of the interface for the returned da
CC2530 common registers for timer 1
匯編實例解析--實模式下屏幕顯示
[combinatorics] recursive equation (constant coefficient linear homogeneous recursive equation | constant coefficient, linear, homogeneous concept description | constant coefficient linear homogeneous
Fast Ethernet and Gigabit Ethernet: what's the difference?
C语言按行修改文件
One brush 142 monotone stack next larger element II (m)
13mnnimo5-4 German standard steel plate 13MnNiMo54 boiler steel 13MnNiMo54 chemical properties
Kotlin学习快速入门(7)——扩展的妙用
Great changes! National housing prices fell below the 10000 yuan mark
Necessary ability of data analysis
How to promote cross department project collaboration | community essay solicitation
LeetCode 1656. Design ordered flow
What material is sa537cl2? Analysis of mechanical properties of American standard container plate
Shentong express expects an annual loss of nearly 1billion