当前位置:网站首页>[combinatorics] recursive equation (the problem of solving recursive equation with multiple roots | the problem is raised)
[combinatorics] recursive equation (the problem of solving recursive equation with multiple roots | the problem is raised)
2022-07-03 17:13:00 【Programmer community】
List of articles
- One 、 The problem of solving recurrence equations with multiple roots
- Two 、 Examples of recurrence equations with multiple roots
One 、 The problem of solving recurrence equations with multiple roots
There are some Recurrence equation Of Characteristic equation Of Characteristic root Yes Heavy root The situation of , The characteristic equation is solved Some of the characteristic roots are equal , So that makes Constants in the general solution cannot obtain unique values ;
Reference resources : 【 Combinatorial mathematics 】 Recurrence equation ( General definition | Structure theorem of general solution of recursive equation without multiple roots ) Two 、 Structure theorem of general solution of recursive equation without multiple roots
stay “ Structure theorem of general solution of recursive equation without multiple roots ” In the chapter , General understanding requirements In the system of equations The coefficient determinant is not equal to
0
0
0 ,
∏
1
≤
i
<
j
≤
k
(
q
i
−
q
k
)
≠
0
\prod\limits_{1 \leq i < j \leq k} ( q_i - q_k ) \not= 0
1≤i<j≤k∏(qi−qk)=0 , If there are two characteristic roots
q
i
,
q
k
q_i , q_k
qi,qk equal , The top " The coefficient determinant is not equal to
0
0
0" It cannot be achieved ;
If the characteristic equation has multiple roots , You can't use it “ Formula solution of recurrence equation without multiple roots ” Solve the recursive equation ;
For recursive equations with multiple roots , Needs to be Linearly independent elements All found , Linear combination , To get a general solution ;
A linear combination : Multiply a solution by
c
1
c_1
c1 , The other solution is multiplied by
c
2
c_2
c2 , The combination after adding ;
Two 、 Examples of recurrence equations with multiple roots
Recurrence equation :
H
(
n
)
−
4
H
(
n
−
1
)
+
4
H
(
n
−
2
)
=
0
H(n) - 4H(n-1) + 4H(n-2) = 0
H(n)−4H(n−1)+4H(n−2)=0
initial value :
H
(
0
)
=
0
,
H
(
1
)
=
1
H(0) = 0 , H(1) = 1
H(0)=0,H(1)=1
Complete process of solving recursive equations without multiple roots :
- 1 . Write the characteristic equation :
- ( 1 ) The standard form of recurrence equation : Write the recurrence equation Standard form , All items are to the left of the equal sign , On the right is
0
0
0 ;
- ( 2 ) Number of terms of characteristic equation : determine Number of terms of characteristic equation , And The recurrence equation has the same number of terms ;
- ( 3 ) The characteristic equation is sub idempotent : The highest power is Number of terms of characteristic equation
−
1
-1
0
0
0 ;
−1 , Lowest power
- ( 4 ) Write There is no coefficient The characteristic equation of ;
- ( 5 ) The coefficients of the recursive equation will be deduced bit by bit Copy Into the characteristic equation ;
- 2 . Solution characteristic root : take The eigenvalue of the characteristic equation is solved ,
x
=
−
b
±
b
2
−
4
a
c
2
a
x = \cfrac{-b \pm \sqrt{b^2 - 4ac}}{2a}
x=2a−b±b2−4ac
- 3 . Construct the general solution of recurrence equation : structure
c
1
q
1
n
+
c
2
q
2
n
+
⋯
+
c
k
q
k
n
c_1q_1^n + c_2q_2^n + \cdots + c_kq_k^n
c1q1n+c2q2n+⋯+ckqkn Linear combination of forms , The linear combination is the solution of the recursive equation ;
- 4 . Find the constant in the general solution : Substitute the initial value of the recursive equation into the general solution , obtain
k
k
k individual
k
k
k Finite element equations , By solving the equations , Get the constant in the general solution ;
- ( 1 ) The constant is substituted into the general solution : Get the final solution of the recursive equation ;
Recurrence equation -> Characteristic equation -> Characteristic root -> general solution -> Substitute the initial value to find the general solution constant
Solve according to the above solution process :
1 . Characteristic equation :
( 1 ) The standard form of recurrence equation : Recursive equations are already in standard form ;
( 2 ) Number of terms of characteristic equation : And The number of terms of recurrence equation identical ,
3
3
3 term ;
( 3 ) The characteristic equation is sub idempotent : The highest power is The number of terms of the characteristic equation minus one ,
3
−
1
=
2
3-1=2
3−1=2 , Lowest power
0
0
0 ;
( 4 ) Write There is no coefficient The characteristic equation of :
x
2
+
x
+
1
=
0
x^2 + x + 1 = 0
x2+x+1=0
( 5 ) The coefficients of the recursive equation will be deduced bit by bit Copy Into the characteristic equation ;
1
x
2
+
(
−
4
)
x
+
(
4
)
1
=
0
1x^2 + (-4)x + (4)1 = 0
1x2+(−4)x+(4)1=0
x
2
−
4
x
+
4
=
0
x^2 - 4x + 4 = 0
x2−4x+4=0
2 . Solution characteristic root : take The eigenvalue of the characteristic equation is solved ,
x
=
−
b
±
b
2
−
4
a
c
2
a
x = \cfrac{-b \pm \sqrt{b^2 - 4ac}}{2a}
x=2a−b±b2−4ac
x
=
4
±
16
−
16
2
=
2
x=\cfrac{4 \pm \sqrt{16 - 16}}{2} = 2
x=24±16−16=2
Both characteristic roots are
2
2
2 ,
q
1
=
2
,
q
2
=
2
q_1=2, q_2 = 2
q1=2,q2=2 ;
3 . Construct the general solution of recurrence equation : structure
c
1
q
1
n
+
c
2
q
2
n
+
⋯
+
c
k
q
k
n
c_1q_1^n + c_2q_2^n + \cdots + c_kq_k^n
c1q1n+c2q2n+⋯+ckqkn Linear combination of forms , The linear combination is the solution of the recursive equation ;
The general solution is :
H
(
n
)
=
c
1
2
n
+
c
2
2
n
=
c
2
n
H(n) = c_12^n + c_22^n = c2^n
H(n)=c12n+c22n=c2n
4 . Find the constant in the general solution : Substitute the initial value of the recursive equation into the general solution , obtain
k
k
k individual
k
k
k Finite element equations , By solving the equations , Get the constant in the general solution ;
take
c
2
n
c2^n
c2n Substitute into
x
2
−
4
x
+
4
=
0
x^2 - 4x + 4 = 0
x2−4x+4=0 In the characteristic equation ,
c
c
c There is no solution ;
If Two characteristic roots All are
2
2
2 , Linear correlation , Right now It is impossible to determine
c
1
,
c
2
c_1, c_2
c1,c2 Undetermined constant ;
Observe
n
2
n
n2^n
n2n Is the solution , The solution and
2
n
2^n
2n Linearly independent , Linearly combine the above two solutions ,
c
1
n
2
n
+
c
2
2
n
c_1n2^n + c_22^n
c1n2n+c22n A linear combination , Is the solution of a recursive equation ,
Substitute the initial value into , You can work out
c
1
,
c
2
c_1, c_2
c1,c2 The value of the constant ;
边栏推荐
- 美团一面:为什么线程崩溃崩溃不会导致 JVM 崩溃
- One brush 142 monotone stack next larger element II (m)
- New library online | cnopendata complete data of Chinese insurance institution outlets
- Unity notes unityxr simple to use
- Why is WPA3 security of enterprise business so important?
- 在iptables防火墙下开启vsftpd的端口
- 【Try to Hack】主动侦查隐藏技术
- 2021 ICPC regional competition (Shanghai) g.edge groups (tree DP)
- 數據分析必備的能力
- Test your trained model
猜你喜欢

Build your own website (23)
Atom QT 16_ audiorecorder
Meituan side: why does thread crash not cause JVM crash

Kotlin learning quick start (7) -- wonderful use of expansion

美团一面:为什么线程崩溃崩溃不会导致 JVM 崩溃

Take you to API development by hand

Simple use of unity pen XR grab
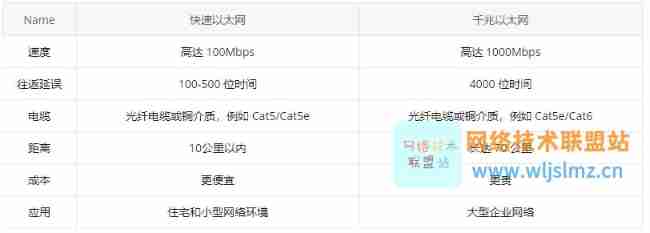
Fast Ethernet and Gigabit Ethernet: what's the difference?
Kubernetes resource object introduction and common commands (III)

線程池:業務代碼最常用也最容易犯錯的組件
随机推荐
C language string practice
Hands on in-depth learning notes (XIV) 3.7 Simple implementation of softmax regression
SSH连接远程主机等待时间过长的解决方法
The way of wisdom (unity of knowledge and action)
[combinatorics] recursive equation (general solution structure of recursive equation with multiple roots | linear independent solution | general solution with multiple roots | solution example of recu
kubernetes资源对象介绍及常用命令(三)
基于主机的入侵系统IDS
Prepare for the golden three silver four, 100+ software test interview questions (function / interface / Automation) interview questions. win victory the moment one raises one 's standard
线程池:业务代码最常用也最容易犯错的组件
Visual studio "usually, each socket address (Protocol / network address / port) can only be used once“
LeetCode 1658. Minimum operand to reduce x to 0
浅谈拉格朗日插值及其应用
One brush 144 force deduction hot question-1 sum of two numbers (E)
Luogu: p1155 [noip2008 improvement group] double stack sorting (bipartite graph, simulation)
Kubernetes resource object introduction and common commands (V) - (NFS & PV & PVC)
Squid service startup script
大变局!全国房价,跌破万元大关
When absolutely positioned, the element is horizontally and vertically centered
网络安全web渗透技术
绝对定位时元素水平垂直居中