当前位置:网站首页>[combinatorics] permutation and combination (multiple set permutation | multiple set full permutation | multiple set incomplete permutation all elements have a repetition greater than the permutation
[combinatorics] permutation and combination (multiple set permutation | multiple set full permutation | multiple set incomplete permutation all elements have a repetition greater than the permutation
2022-07-03 12:50:00 【Programmer community】
List of articles
- One 、 Multiple sets
- Two 、 Full Permutation of multiple sets
- 3、 ... and 、 Examples of Full Permutation of multiple sets
- 3、 ... and 、 Multiset incomplete permutation 1 The repetition of all elements is greater than the number of permutations (
n
i
≥
r
n_i \geq r
ni≥r )
- Four 、 Multiset incomplete permutation 2 The repetition of some elements is less than the number of permutations (
n
i
≤
r
n_i \leq r
ni≤r )
Arrange and combine reference blogs :
- 【 Combinatorial mathematics 】 Basic counting principle ( The principle of addition | Multiplication principle )
- 【 Combinatorial mathematics 】 Examples of permutation and combination of sets ( array | Combine | Circular arrangement | binomial theorem )
- 【 Combinatorial mathematics 】 Permutation and combination ( Arrange and combine content summary | Select the question | Set arrangement | Set combination )
- 【 Combinatorial mathematics 】 Permutation and combination ( Examples of permutations )
One 、 Multiple sets
Multiset representation :
S
=
{
n
1
⋅
a
1
,
n
2
⋅
a
2
,
⋯
,
n
k
⋅
a
k
}
,
0
≤
n
i
≤
+
∞
S = \{ n_1 \cdot a_1 , n_2 \cdot a_2 , \cdots , n_k \cdot a_k \} , \ \ \ 0 \leq n_i \leq +\infty
S={ n1⋅a1,n2⋅a2,⋯,nk⋅ak}, 0≤ni≤+∞
- Type of elements : Multiple sets contain
k
k
k Different elements ,
- The element represents : Each element is represented as
a
1
,
a
2
,
⋯
,
a
k
a_1 , a_2 , \cdots , a_k
a1,a2,⋯,ak ,
- Element number : The number of occurrences of each element is
n
1
,
n
2
,
⋯
,
n
k
n_1, n_2, \cdots , n_k
n1,n2,⋯,nk ,
- The value of the number of elements :
n
i
n_i
ni The value requirement of is Greater than
0
0
0 , Less than positive infinity
+
∞
+ \infty
+∞ ;
Two 、 Full Permutation of multiple sets
Multiple sets :
S
=
{
n
1
⋅
a
1
,
n
2
⋅
a
2
,
⋯
,
n
k
⋅
a
k
}
,
0
≤
n
i
≤
+
∞
S = \{ n_1 \cdot a_1 , n_2 \cdot a_2 , \cdots , n_k \cdot a_k \} , \ \ \ 0 \leq n_i \leq +\infty
S={ n1⋅a1,n2⋅a2,⋯,nk⋅ak}, 0≤ni≤+∞
* Full Permutation :
r
=
n
1
+
n
2
+
⋯
+
n
k
=
n
r = n_1 + n_2 + \cdots + n_k = n
r=n1+n2+⋯+nk=n
N
=
n
!
n
1
!
n
2
!
⋯
n
k
!
N = \cfrac{n!}{n_1! n_2! \cdots n_k!}
N=n1!n2!⋯nk!n!
* The total permutation number of a multiset is Factorial of the total number of elements , Divide Factorial of all repetitions ;
Here's the derivation process
Yes
k
k
k Elements ,
Place the elements
a
1
a_1
a1 : Put the first element in the arrangement
a
1
a_1
a1 , This element has
n
1
n_1
n1 individual ,
n
n
n Select from two positions
n
1
n_1
n1 individual Location , Yes
C
(
n
,
n
1
)
C(n, n_1)
C(n,n1) Methods ;
C
(
n
,
n
1
)
=
n
!
(
n
−
n
1
)
!
n
1
!
C(n, n_1) = \cfrac{n!}{(n-n_1) ! \ n_1!}
C(n,n1)=(n−n1)! n1!n!
Place the elements
a
2
a_2
a2 : Put it in place
n
1
n_1
n1 Then put the second element
a
2
a_2
a2 , This element has
n
2
n_2
n2 individual , And then there is
n
−
n
1
n-n_1
n−n1 Empty space , from
n
−
1
n-1
n−1 Choose among the positions
n
2
n_2
n2 There are two positions
C
(
n
−
n
1
,
n
2
)
C(n-n_1 , n_2)
C(n−n1,n2) Methods ;
C
(
n
−
n
1
,
n
2
)
=
(
n
−
n
1
)
!
(
n
−
n
1
−
n
2
)
!
n
2
!
C(n - n_1, n_2) = \cfrac{(n-n_1)!}{(n-n_1 - n_2) ! \ n_2!}
C(n−n1,n2)=(n−n1−n2)! n2!(n−n1)!
⋮
\vdots
⋮
Place the elements
a
k
a_k
ak : Place the last element
a
k
a_k
ak , This element has
n
k
n_k
nk individual , And then there is
n
−
n
1
−
⋯
−
n
k
−
1
n-n_1- \cdots -n_{k-1}
n−n1−⋯−nk−1 Empty space , from
n
−
n
1
−
⋯
−
n
k
−
1
n-n_1- \cdots -n_{k-1}
n−n1−⋯−nk−1 Choose among the positions
n
k
n_k
nk There are two positions
C
(
n
−
n
1
−
⋯
−
n
k
−
1
,
n
k
)
C(n-n_1- \cdots -n_{k-1} , n_k)
C(n−n1−⋯−nk−1,nk) Methods ;
C
(
n
−
n
1
−
⋯
−
n
k
−
1
,
n
k
)
=
(
n
−
n
1
−
⋯
−
n
k
−
1
)
!
(
n
−
n
1
−
⋯
−
n
k
−
1
−
n
k
)
!
n
k
!
C(n-n_1- \cdots -n_{k-1} , n_k) = \cfrac{(n-n_1- \cdots -n_{k-1})!}{(n-n_1- \cdots -n_{k-1} - n_k) ! \ n_k!}
C(n−n1−⋯−nk−1,nk)=(n−n1−⋯−nk−1−nk)! nk!(n−n1−⋯−nk−1)!
product rule : Finally, according to the law of multiplication , Multiply each of the above placement methods , You get the final result , Factorials look complicated , however Factorial options such as
(
n
−
n
1
−
⋯
−
n
k
−
1
)
!
(n-n_1- \cdots -n_{k-1})!
(n−n1−⋯−nk−1)! You can make an appointment , The final results are as follows :
N
=
C
(
n
,
n
1
)
C
(
n
−
n
1
,
n
2
)
C
(
n
−
n
1
−
⋯
−
n
k
−
1
,
n
k
)
=
n
!
(
n
−
n
1
)
!
n
1
!
×
(
n
−
n
1
)
!
(
n
−
n
1
−
n
2
)
!
n
2
!
×
(
n
−
n
1
−
⋯
−
n
k
−
1
)
!
(
n
−
n
1
−
⋯
−
n
k
−
1
−
n
k
)
!
n
k
!
about
fall
Ministry
branch
rank
ride
=
n
!
n
1
!
n
2
!
⋯
n
k
!
\begin{array}{lcl} N & = & C(n, n_1) C(n - n_1, n_2) C(n-n_1- \cdots -n_{k-1} , n_k) \\\\ & = & \cfrac{n!}{(n-n_1) ! \ n_1!} \times \cfrac{(n-n_1)!} {(n-n_1 - n_2) ! \ n_2!} \times \cfrac{(n-n_1- \cdots -n_{k-1})!}{(n-n_1- \cdots -n_{k-1} - n_k) ! \ n_k!} \ \ \ Omit some factorials \\\\ &=& \cfrac{n!}{n_1! n_2! \cdots n_k!} \end{array}
N===C(n,n1)C(n−n1,n2)C(n−n1−⋯−nk−1,nk)(n−n1)! n1!n!×(n−n1−n2)! n2!(n−n1)!×(n−n1−⋯−nk−1−nk)! nk!(n−n1−⋯−nk−1)! about fall Ministry branch rank ride n1!n2!⋯nk!n!
3、 ... and 、 Examples of Full Permutation of multiple sets
Finding multiple sets
S
=
{
3
⋅
a
,
2
⋅
b
,
1
⋅
c
}
S=\{ 3 \cdot a , 2 \cdot b , 1 \cdot c \}
S={ 3⋅a,2⋅b,1⋅c} The whole arrangement ?
The total number of elements in the above multiset is
n
=
3
+
2
+
1
=
6
n = 3 + 2 + 1 = 6
n=3+2+1=6 ;
The total number of permutations is :
N
=
6
!
3
!
×
2
!
×
1
!
=
6
×
5
×
4
×
3
×
2
×
1
(
3
×
2
×
1
)
×
(
2
×
1
)
×
(
1
×
1
)
=
60
N = \cfrac{6!}{3! \times 2! \times 1!} = \cfrac{6 \times 5 \times 4 \times 3 \times 2 \times 1}{( 3 \times 2 \times 1 ) \times ( 2 \times 1 ) \times (1 \times 1)} = 60
N=3!×2!×1!6!=(3×2×1)×(2×1)×(1×1)6×5×4×3×2×1=60
3、 ... and 、 Multiset incomplete permutation 1 The repetition of all elements is greater than the number of permutations ( n
i
≥
r
n_i \geq r
ni≥r )
Multiple sets :
S
=
{
n
1
⋅
a
1
,
n
2
⋅
a
2
,
⋯
,
n
k
⋅
a
k
}
,
0
≤
n
i
≤
+
∞
S = \{ n_1 \cdot a_1 , n_2 \cdot a_2 , \cdots , n_k \cdot a_k \} , \ \ \ 0 \leq n_i \leq +\infty
S={ n1⋅a1,n2⋅a2,⋯,nk⋅ak}, 0≤ni≤+∞
* Incomplete permutation
1
1
1 :
r
≤
n
i
r \leq n_i
r≤ni , Notice the
r
r
r want Less than or equal to The smallest
n
i
n_i
ni ;
N
=
k
r
N = k^r
N=kr
Derivation process :
Under the above conditions ,
r
r
r A place ,
There are elements in every position
k
k
k A choice ,
According to the law of multiplication , The total number of choices is
k
×
k
×
⋯
×
k
⏟
r
individual
k
\begin{matrix} \underbrace{ k \times k \times \cdots \times k } \\ r individual k \end{matrix}
k×k×⋯×kr individual k ,
namely
r
k
r^k
rk ;
Four 、 Multiset incomplete permutation 2 The repetition of some elements is less than the number of permutations ( n
i
≤
r
n_i \leq r
ni≤r )
The above situation only applies to the situation where the repeatability is large enough , namely The repetition of each element is greater than the number of selections ,
r
≤
n
i
r \leq n_i
r≤ni
If The repetition of one element is less than the number of selections ,
r
≥
n
i
r \geq n_i
r≥ni ,
Such as
S
=
{
3
⋅
a
,
2
⋅
b
,
1
⋅
c
}
S=\{ 3 \cdot a , 2 \cdot b , 1 \cdot c \}
S={ 3⋅a,2⋅b,1⋅c} Three permutations of multiple sets , You can't use formulas ,
There is no formula for , But it can. Use The inclusion exclusion principle , Generating function Calculate ;
边栏推荐
- 剑指Offer10- I. 斐波那契数列
- Simple use and precautions of kotlin's array array and set list
- Drop down refresh conflicts with recyclerview sliding (swiperefreshlayout conflicts with recyclerview sliding)
- Sword finger offer05 Replace spaces
- [judgment question] [short answer question] [Database Principle]
- Xctf mobile--app3 problem solving
- Quick learning 1.8 front and rear interfaces
- CNN MNIST handwriting recognition
- TOGAF认证自学宝典V2.0
- How to convert a decimal number to binary in swift
猜你喜欢

(latest version) WiFi distribution multi format + installation framework

剑指Offer03. 数组中重复的数字【简单】
公纵号发送提示信息(用户微服务--消息微服务)
【ArcGIS自定义脚本工具】矢量文件生成扩大矩形面要素

Eureka self protection

Application of ncnn neural network computing framework in orange school orangepi 3 lts development board

GaN图腾柱无桥 Boost PFC(单相)七-PFC占空比前馈

Quick learning 1.8 front and rear interfaces
Detailed explanation of the most complete constraintlayout in history

Sword finger offer10- I. Fibonacci sequence
随机推荐
最新版盲盒商城thinkphp+uniapp
Method overloading and rewriting
Powerful avatar making artifact wechat applet
Summary of error prone knowledge points: Calculation of define s (x) 3*x*x+1.
[embedded] - Introduction to four memory areas
Swift5.7 extend some to generic parameters
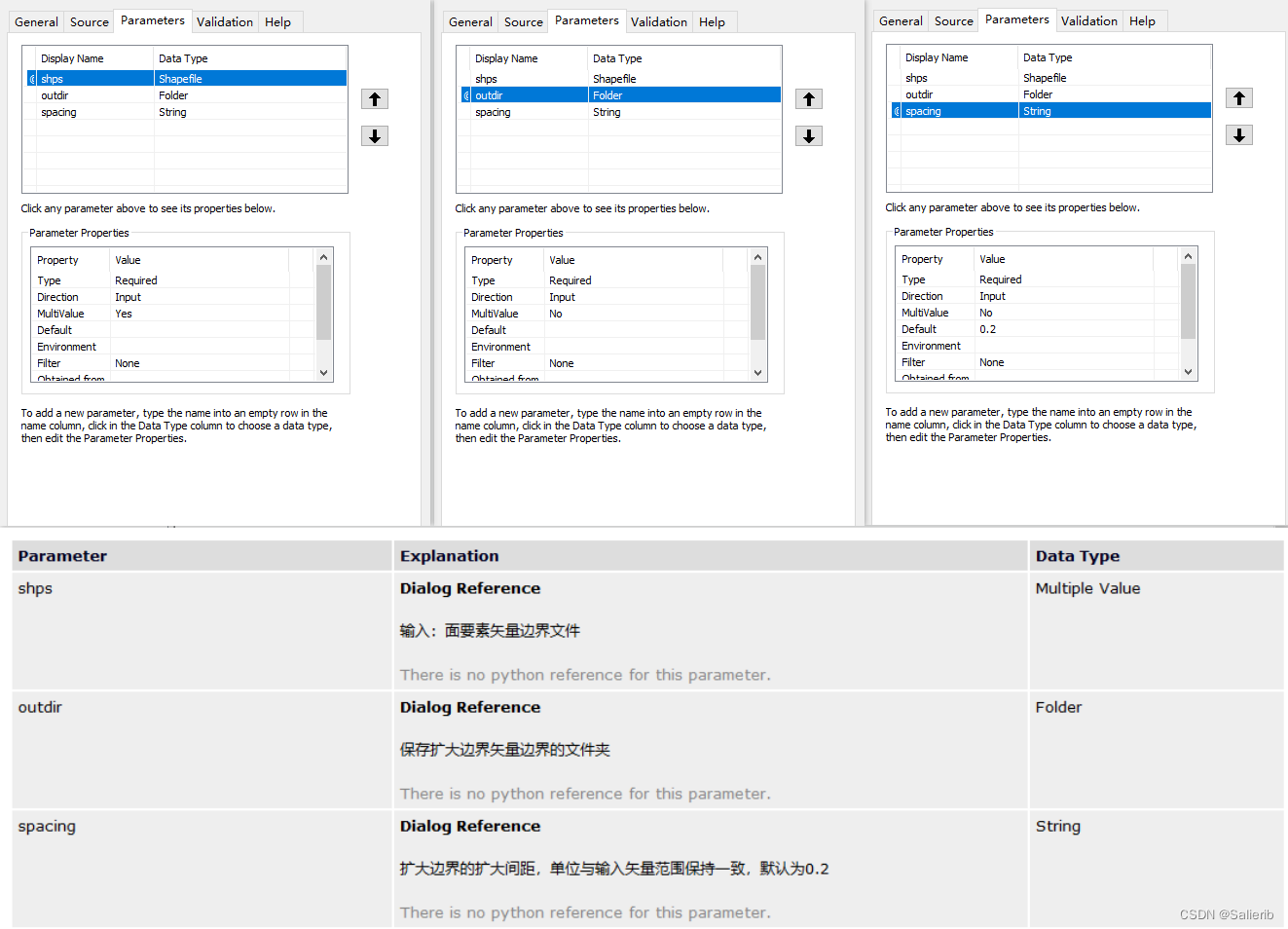
【ArcGIS自定义脚本工具】矢量文件生成扩大矩形面要素
Ali & ant self developed IDE
alright alright alright
[exercice 7] [principe de la base de données]
elastic_ L01_ summary
Enable SASL authentication for memcached
Airflow installation jump pit
Integer case study of packaging
【综合题】【数据库原理】
Kung Fu pays off, and learning is done
LeetCode 0556. Next bigger element III - end of step 4
4. 无线体内纳米网:电磁传播模型和传感器部署要点
01_ Using the concurrent tool class library, is thread safety safe
studio All flavors must now belong to a named flavor dimension. Learn more