当前位置:网站首页>[Luogu p1971] rabbit and egg game (bipartite game)
[Luogu p1971] rabbit and egg game (bipartite game)
2022-07-07 07:04:00 【SSL_ TJH】
Rabbit and egg game
Topic link :luogu P1971
The main idea of the topic
Give you a two-dimensional grid , There is only one position without chess pieces , There are white or black pieces in other positions .
Then two people take turns , The first hand can move one of the four white chess pieces next to the empty grid , The back hand moves the black chess pieces .
Then those who cannot move lose , Then give you the operation process of two people , Guarantee the backhand to win , Then I ask you what moments have changed from being the first to win to being the first to lose .
Ideas
First of all, if you know the bipartite graph game , You can find that this can be a bipartite graph game model .
( After all, the grid diagram )
But there are too many states , Let's consider optimization , Or merge duplicate States , Get rid of useless state .
Then you are watching two people alternate , And everyone applies to different situations , Then you find that this picture is not big .
Can we change the state to the level of graph point size ? Think about it and you'll find a point. Don't repeat it .
Because you must go out and come back after an even number of times , At this time, another person operates to this point , But you last went out and left your color here , The next person can't get in .
Then with this property , In fact, we don't need to care about the color of other positions , Just set the position of the space directly .
Then the edges are fixed, because the colors around you are actually fixed ( We have to go from a different color to this color , Then the different color is equivalent to being replaced with this color )
So we can do it .
But this is not an inquiry , But you can understand it as asking whether to win first after each operation ( If the situation before and after the first hand operation is that the first hand will win, the first hand will make a mistake ).
Then you run so many network flows / Hungary is not very good .
When we think about it once, how did we do it , We deleted some first , Then you can't delete it .
In fact, we can do this in reverse order , First get all deleted , Then add one by one , Get the answers respectively .
Code
#include<queue>
#include<cstdio>
#include<iostream>
#define INF 0x3f3f3f3f3f3f3f3f
using namespace std;
const int N = 45;
char s[N][N];
int n, m, K, tot, S, T;
bool col[N][N], in[N][N], win[2005];
int dx[4] = {
1, 0, -1, 0}, dy[4] = {
0, 1, 0, -1};
pair <int, int> del[2005];
int id(int x, int y) {
return (x - 1) * m + y;}
bool check(int x, int y) {
if (x < 1 || x > n) return 0; if (y < 1 || y > m) return 0; if (col[x][y]) return 0; return 1;}
bool check_(int x, int y) {
if (x < 1 || x > n) return 0; if (y < 1 || y > m) return 0; return 1;}
struct Flow {
struct node {
int x, to, nxt, op;
}e[N * N * 500];
int le[N * N], KK, dis[N * N], lee[N * N];
queue <int> q;
void add(int x, int y, int z) {
e[++KK] = (node){
z, y, le[x], KK + 1}; le[x] = KK;
e[++KK] = (node){
0, x, le[y], KK - 1}; le[y] = KK;
}
bool bfs() {
while (!q.empty()) q.pop();
for (int i = 1; i <= tot; i++) dis[i] = 0, lee[i] = le[i];
q.push(S); dis[S] = 1;
while (!q.empty()) {
int now = q.front(); q.pop();
for (int i = le[now]; i; i = e[i].nxt)
if (!dis[e[i].to] && e[i].x) {
dis[e[i].to] = dis[now] + 1;
if (e[i].to == T) return 1;
q.push(e[i].to);
}
}
return 0;
}
int dfs(int now, int sum) {
if (now == T) return sum;
int go = 0;
for (int &i = lee[now]; i; i = e[i].nxt)
if (dis[e[i].to] == dis[now] + 1 && e[i].x) {
int this_go = dfs(e[i].to, min(sum - go, e[i].x));
if (this_go) {
e[i].x -= this_go; e[e[i].op].x += this_go;
go += this_go; if (go == sum) return go;
}
}
return go;
}
int dinic() {
int re = 0;
while (bfs())
re += dfs(S, INF);
return re;
}
}W;
void Init() {
for (int i = 1; i <= n; i++)
for (int j = 1; j <= m; j++)
if (col[i][j] && !in[i][j]) {
for (int k = 0; k < 4; k++) {
int tx = i + dx[k], ty = j + dy[k];
if (!check(tx, ty) || in[tx][ty]) continue;
W.add(id(i, j), id(tx, ty), 1);
}
}
W.dinic();
}
int main() {
scanf("%d %d", &n, &m); tot = id(n, m); S = ++tot; T = ++tot;
for (int i = 1; i <= n; i++)
for (int j = 1; j <= m; j++) {
s[i][j] = getchar(); while (s[i][j] != 'X' && s[i][j] != 'O' && s[i][j] != '.') s[i][j] = getchar();
if (s[i][j] == '.') del[0] = make_pair(i, j), in[i][j] = 1;
if (s[i][j] == '.' || s[i][j] == 'X') col[i][j] = 1, W.add(S, id(i, j), 1);
else W.add(id(i, j), T, 1);
}
scanf("%d", &K);
for (int i = 1; i <= K << 1; i++) {
int x, y; scanf("%d %d", &x, &y); del[i] = make_pair(x, y); in[x][y] = 1;
}
Init();
for (int i = K << 1; i >= 0; i--) {
pair <int, int> v = del[i];
in[v.first][v.second] = 0;
for (int j = 0; j < 4; j++) {
int tx = v.first + dx[j], ty = v.second + dy[j];
if (!check_(tx, ty) || in[tx][ty] || col[v.first][v.second] == col[tx][ty]) continue;
if (col[v.first][v.second]) W.add(id(v.first, v.second), id(tx, ty), 1);
else W.add(id(tx, ty), id(v.first, v.second), 1);
}
win[i] = W.dinic();
}
int ans = 0;
for (int i = 1; i <= K << 1; i += 2) {
if (win[i - 1] && win[i]) ans++;
}
printf("%d\n", ans);
for (int i = 1; i <= K << 1; i += 2) {
if (win[i - 1] && win[i]) {
printf("%d\n", (i + 1) / 2);
}
}
return 0;
}
边栏推荐
- Take you to brush (niuke.com) C language hundred questions (the first day)
- MySQL user permissions
- impdp的transform参数的测试
- 多线程与高并发(9)——AQS其他同步组件(Semaphore、ReentrantReadWriteLock、Exchanger)
- 如何给目标机器人建模并仿真【数学/控制意义】
- DB2获取表信息异常:Caused by: com.ibm.db2.jcc.am.SqlException: [jcc][t4][1065][12306][4.25.13]
- leetcode 509. Fibonacci Number(斐波那契数字)
- A slow SQL drags the whole system down
- How to do sports training in venues?
- Advantages of using net core / why
猜你喜欢
学术报告系列(六) - Autonomous Driving on the journey to full autonomy
Take you to brush (niuke.com) C language hundred questions (the first day)
剑指offer-高质量的代码

What books can greatly improve programming ideas and abilities?
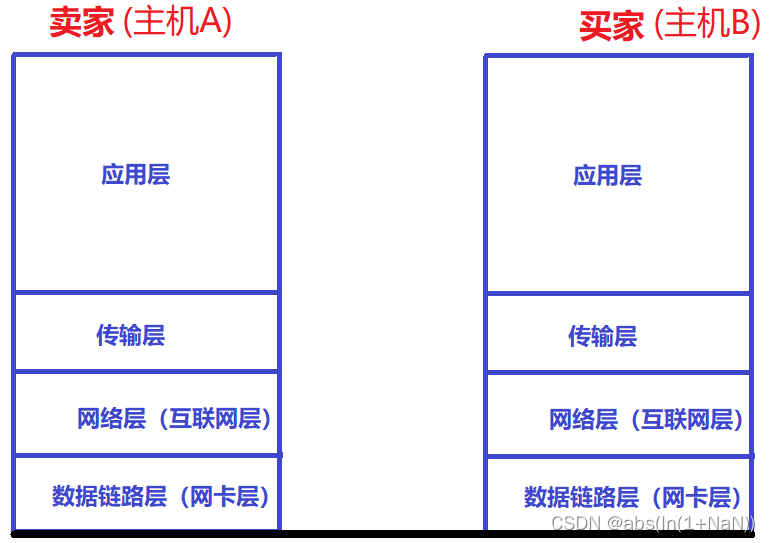
使用TCP/IP四层模型进行网络传输的基本流程
The latest trends of data asset management and data security at home and abroad
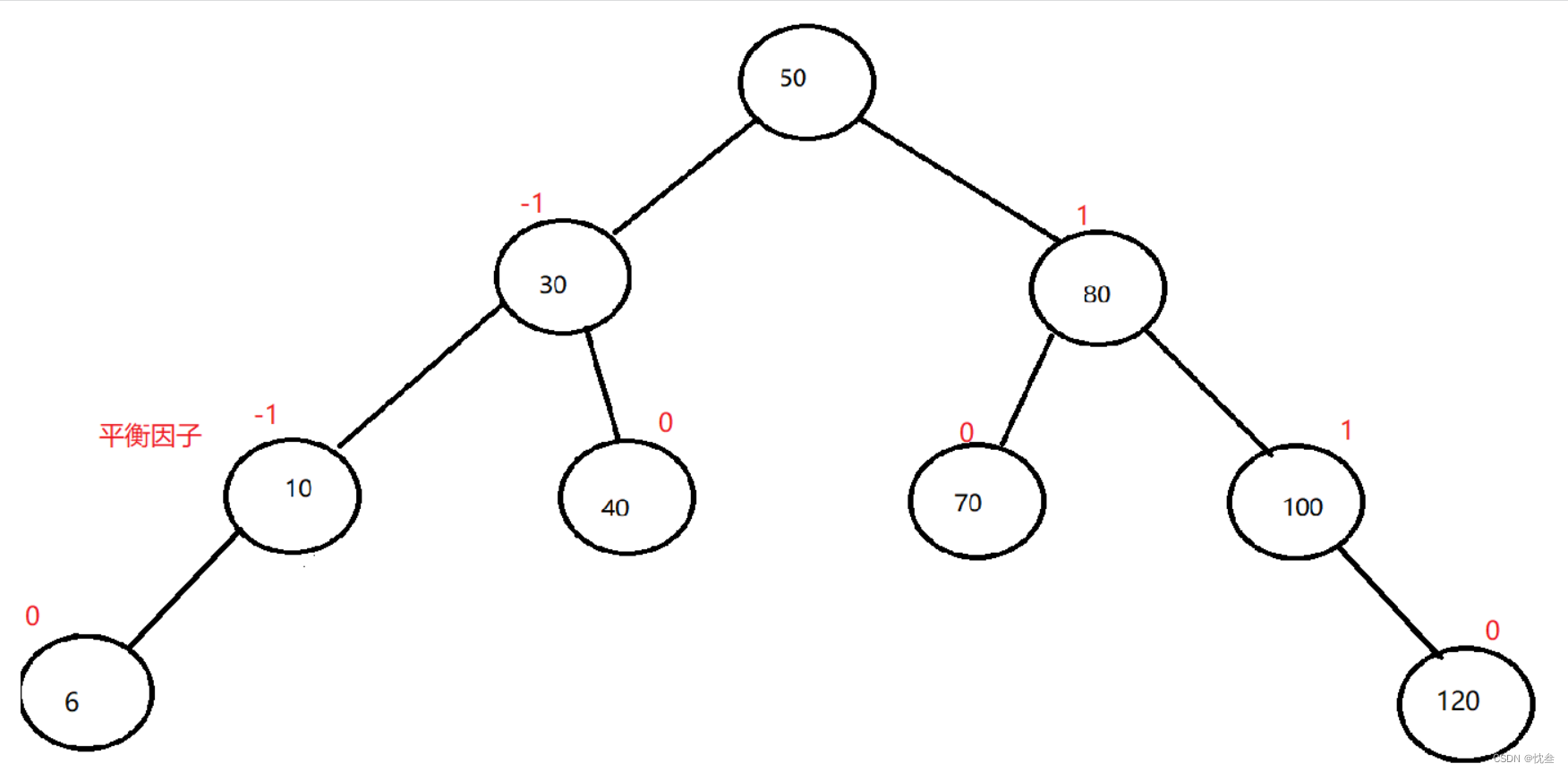
AVL树的实现
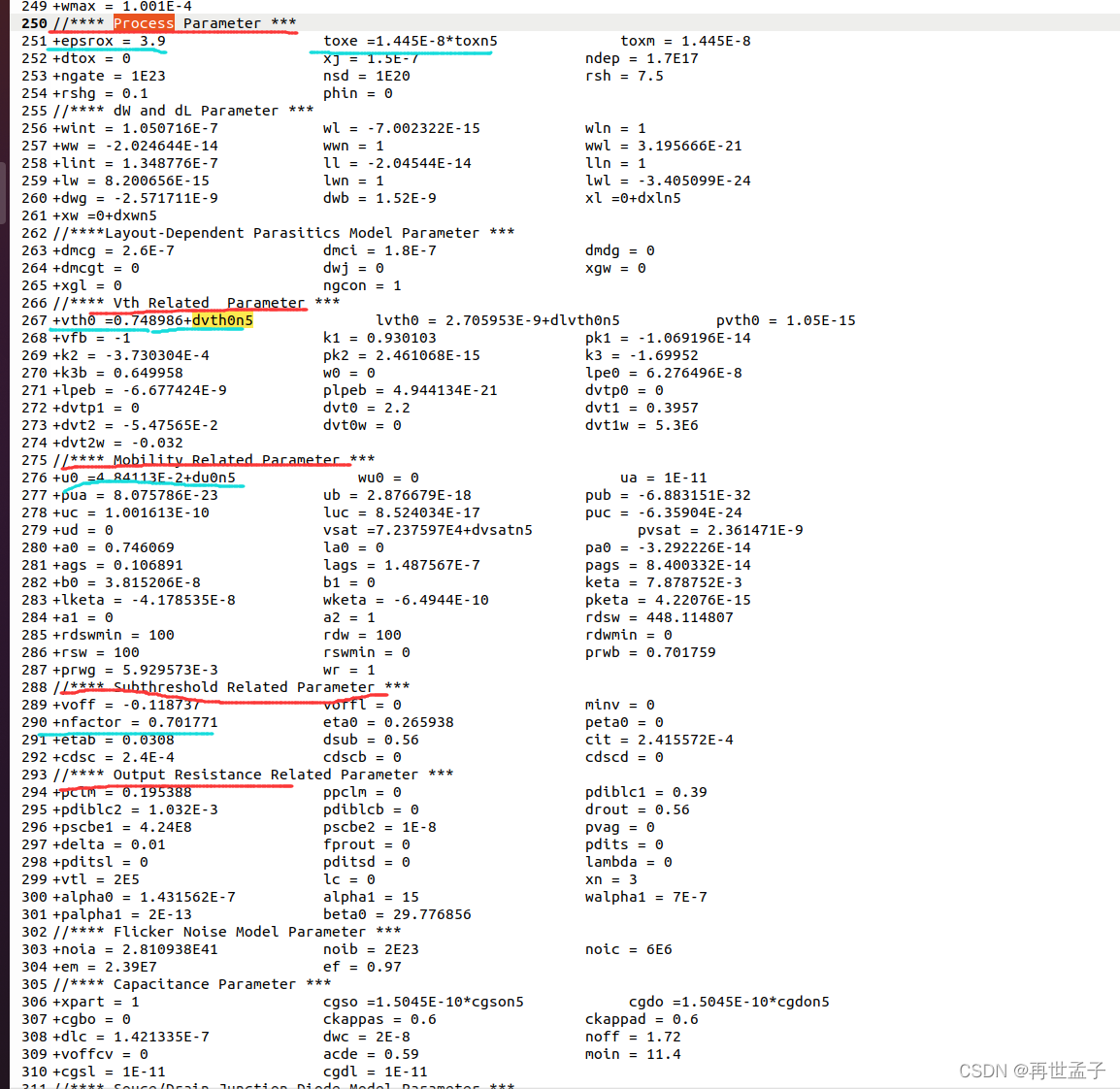
MOS tube parameters μ A method of Cox

Stack and queue-p79-9
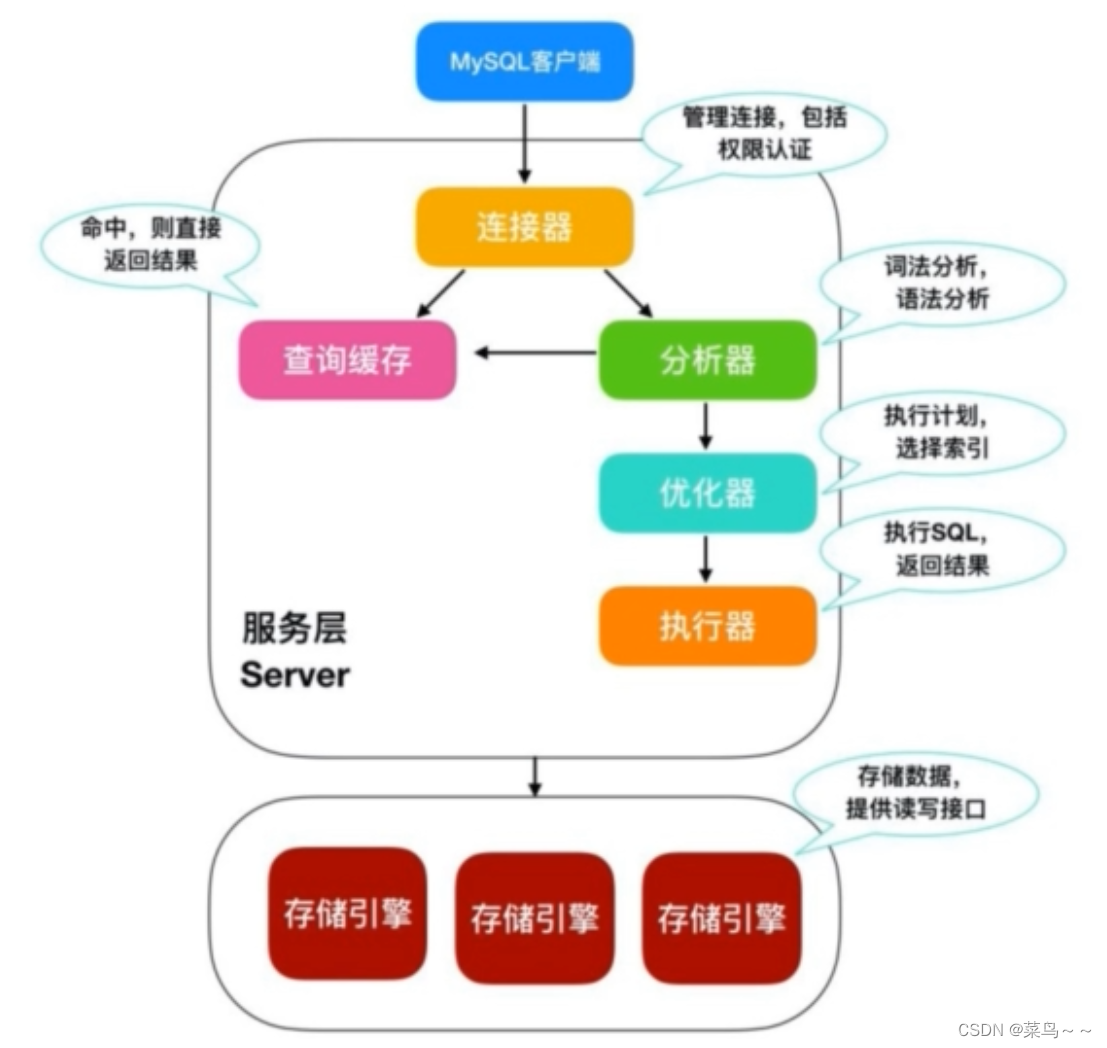
Complete process of MySQL SQL
随机推荐
Can 7-day zero foundation prove HCIA? Huawei certification system learning path sharing
Multithreading and high concurrency (9) -- other synchronization components of AQS (semaphore, reentrantreadwritelock, exchanger)
Matlab tips (29) polynomial fitting plotfit
2018年江苏省职业院校技能大赛高职组“信息安全管理与评估”赛项任务书第二阶段答案
DB2获取表信息异常:Caused by: com.ibm.db2.jcc.am.SqlException: [jcc][t4][1065][12306][4.25.13]
Sword finger offer high quality code
请问 flinksql对接cdc时 如何实现计算某个字段update前后的差异 ?
MOS tube parameters μ A method of Cox
How to model and simulate the target robot [mathematical / control significance]
场馆怎么做体育培训?
Networkx绘图和常用库函数坐标绘图
MYSQL binlog相关命令
impdp的transform参数的测试
Prime partner of Huawei machine test questions
关于数据库数据转移的问题,求各位解答下
多学科融合
请教一个问题,flink oracle cdc,读取一个没有更新操作的表,隔十几秒就重复读取全量数据
unity3d学习笔记
String (explanation)
使用TCP/IP四层模型进行网络传输的基本流程