当前位置:网站首页>Prior knowledge of machine learning in probability theory (Part 1)
Prior knowledge of machine learning in probability theory (Part 1)
2022-07-05 20:53:00 【Full stack programmer webmaster】
Hello everyone , I meet you again , I'm the king of the whole stack , I've prepared for you today Idea Registration code .
With Hadoop Such as the emergence of big data and the development of Technology , machine Study More and more people's attention .
Actually early Hadoop Before , Machine learning and data mining already exist , As a separate discipline , Why? hadoop After that , Machine learning is so compelling ? because of this hadoop The emergence of enables many people to have the technical support to deal with massive data . And then discover the importance of data , And to find valuable information from data . Choosing machine learning seems to be an inevitable trend . Of course, it does not rule out the factor of public opinion . In fact, I have always been skeptical of many people claiming to have mastered machine learning . To understand the essence of machine learning . Mathematical knowledge is indispensable , For example, linear algebra . Probability theory and calculus 、 Vector space, etc . And the assumption has no certain mathematical basis , Using machine learning can only know it but don't know why . For this reason , We will systematically summarize some mathematical knowledge used in machine learning , Of course, it's impossible to cover everything , But it will be as accurate as possible .
This article first studies probability theory , Probability theory plays a major role in machine learning . Because probability theory provides a theoretical basis for the correctness of machine learning algorithm . The design of learning algorithm often depends on the probability assumption of data and is directly used in some algorithms .
Permutation and combination
array : from n Among the different elements , Take whatever you like m(m≤n,m And n All are natural numbers ) The elements are arranged in a column in a certain order , Called from n Take out... Of the different elements m An arrangement of elements ; from n Take out... Of the different elements m(m≤n) The number of all permutations of elements , Called from n Take out... Of the different elements m Number of permutations of elements , Use symbols A(n,m) Express .A(n,m)=n(n-1)(n-2)……(n-m+1)=n!/(n-m)!. Usually what we call permutation refers to the number of all permutations , namely A(n,m).
Combine : from n Among the different elements . Take whatever you like m(m≤n) A group of elements , It's called from n Take out... Of the different elements m A combination of elements ; from n Take out... Of the different elements m(m≤n) The number of all combinations of elements , It's called from n Take out... Of the different elements m Number of combinations of elements . Use symbols C(n,m) Express .C(n,m)=A(n,m)/m!.C(n,m)=C(n,n-m).
Usually, what we call combination refers to the number of all combinations . namely C(n,m).
The difference between combination and arrangement is only seen from the formula ,C(n,m)=A(n,m)/m!, And why divide by m! Well ? Analyze from the definition . Arrangement is an ordered sequence , That is, the element x,y Put it in position 1.2 And put it on 2.1 There are two different sequences , Composition only cares about whether an element is selected . Regardless of order , That is to say x,y Put it in position 1.2 still 2.1 Are considered to be the same combination . because m The elements are m There are two positions m! Arrangement in , And this is just a combination for a combination , So you have to divide by m!.
A random variable
In probability theory . Random variables play an important role . Never confuse random variables with commonly mentioned variables , Think that random variables are variables whose values have randomness , But in fact . Random variables are functions . Map the test results to real numbers , More generally understood as , Random variables are artificially defined functions based on test results , The definition domain of this function is the value of the test result , Its value range varies according to different situations . Capital letters are usually used to represent random variables .
Suppose that random variables X Indicates that the result of rolling six sided dice is mapped to a real number , Be able to define X The result of the throw i It maps to i, For example, the result of throwing is 2, be X The result is 2.
It can also define the assumption that the throwing result is even . be X As the result of the 1. Otherwise 0. Such random variables are called indicator variables . Used to indicate whether an event has occurred .
A random variable X Value a And the probability of that is expressed as P(X = a) or P X(a), Use Val(X) Indicates the value range of random variables .
Joint distribution 、 Marginal distribution and conditional distribution
The distribution of random variables refers to the probability of taking certain values , According to the definition, the distribution is essentially probability , Use P(X) Represents a random variable X The distribution of .
When it comes to the distribution of more than one variable . This distribution is called joint distribution , At this time, the probability is determined by all the variables involved .
Consider the following example of joint distribution .X Random variable for rolling dice . The value is [1,6],Y A random variable for tossing coins , The value is [0,1], The joint distribution of the two is :
P | X=1 | X=2 | X=3 | X=4 | X=5 | X=6 |
|---|---|---|---|---|---|---|
Y=0 | 1/12 | 1/12 | 1/12 | 1/12 | 1/12 | 1/12 |
Y=1 | 1/12 | 1/12 | 1/12 | 1/12 | 1/12 | 1/12 |
Use P(X=a,Y=b) or PX,Y(a,b) Express X take a,Y take b The probability of time , Use P(X,Y) Express X.Y The joint distribution of .
Given a random variable X and Y The joint distribution of , Be able to define X perhaps Y The marginal distribution of . Marginal distribution refers to the probability distribution of a random variable itself , To calculate the marginal distribution of a random variable , You need to add other random variables in the joint distribution , Formula for :
The conditional distribution points out that when other random variables are known , The distribution of a particular random variable . And for a random variable X stay Y=b In this case, the value is a The conditional probability of can be defined as follows , The conditional distribution of the variable can be determined according to the formula :
The above formula can be extended to conditional probabilities based on multiple random variables . example , Based on two variables :
Using symbols P(X|Y=b) It means that Y=b Under the circumstances ,X The distribution of .P(X|Y)X Distributed set . Each of these elements is Y When taking different values X The distribution of .
In probability theory , Independence means that the distribution of one random variable is not affected by another random variable . Use the following mathematical formula to define random variables X Independent of Y:
According to this formula and the formula of conditional distribution, we can deduce the hypothesis X Independent of Y, that Y Also independent of X. Push to step, such as the following :
According to the push process above, we can get P(X,Y)=P(X)P(Y). That is, the formula is X and Y Mutually independent equivalent formulas .
Further, we can define conditional independence . That is, the value of one or more random variables is known , If some other variables are independent of each other, it is called conditional independence . It is known that Z.X and Y Independent mathematical definitions, such as the following :
Finally, let's look at two important theorems , They are chain rules and Bayesian rules .
The formula of chain rule is as follows :
The formula of Bayesian rule is as follows :
Bayesian formula is calculated P(Y|X) To get the value of P(X|Y) Value . This formula can be derived from the conditional formula :
The value of the denominator can be calculated from the edge distribution mentioned above :
Discrete distribution and continuous distribution
A broad sense . There are two kinds of distributions . They are discrete distribution and continuous distribution .
Discrete distribution means that random variables under this distribution can only take finite different values ( Or the result space is limited ). The discrete distribution can be defined by simply enumerating the probabilities of random variables to take each possible value , Such enumeration is called probability quality function , Because this function will unit mass ( Total probability ,1) Cut and then assign different values that random variables can take .
Continuous distribution means that random variables can take infinitely different values ( Or the result space is infinite ), Use the probability density function (probability density function,PDF) Define continuous distribution .
Probability density function f Non negative . An integrable function :
A random variable X According to the probability density function :
Special . The value of a continuously distributed random variable is whatever the probability of ordering a single value is 0, For example, random variables with continuous distribution X The value is a The probability of is 0. Because the upper and lower limits of the integral are a.
The cumulative distribution function can be derived from the probability density function . This function gives the probability that the random variable is less than a certain value , The relationship with probability density function is :
So according to the meaning of indefinite integral ,
Copyright notice : This article is an original blog article , Blog , Without consent , Shall not be reproduced .
Publisher : Full stack programmer stack length , Reprint please indicate the source :https://javaforall.cn/117652.html Link to the original text :https://javaforall.cn
边栏推荐
- 示波器探头对信号源阻抗的影响
- CareerCup它1.8 串移包括问题
- Clear app data and get Icon
- 当Steam教育进入个性化信息技术课程
- Duchefa丨S0188盐酸大观霉素五水合物中英文说明书
- The Chinese Academy of Management Sciences gathered industry experts, and Fu Qiang won the title of "top ten youth" of think tank experts
- 概率论机器学习的先验知识(上)
- Duchefa细胞分裂素丨二氢玉米素 (DHZ)说明书
- 国外LEAD美国简称对照表
- 王老吉药业“关爱烈日下最可爱的人”公益活动在南京启动
猜你喜欢
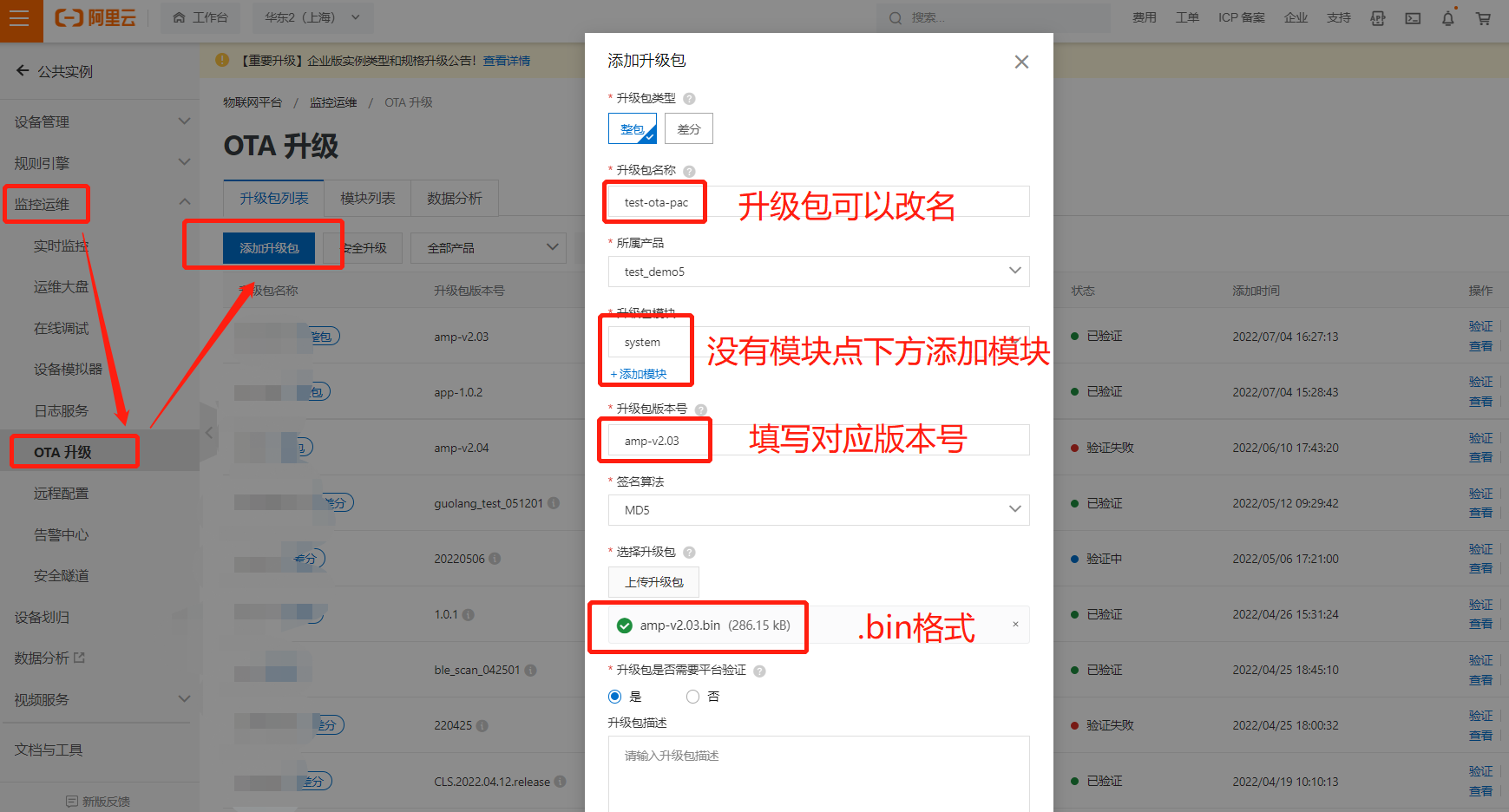
haas506 2.0开发教程 - 阿里云ota - pac 固件升级(仅支持2.2以上版本)

Duchefa丨MS培养基含维生素说明书
台风来袭!建筑工地该如何防范台风!
Make Jar, Not War
学习机器人无从下手?带你体会当下机器人热门研究方向有哪些
基於flask寫一個接口
Abnova e (diii) (WNV) recombinant protein Chinese and English instructions

Duchefa cytokinin dihydrozeatin (DHZ) instructions

产品好不好,谁说了算?Sonar提出分析的性能指标,帮助您轻松判断产品性能及表现
AI 从代码中自动生成注释文档
随机推荐
中国管理科学研究院凝聚行业专家,傅强荣获智库专家“十佳青年”称号
科普|英语不好对NPDP考试有影响吗 ?
Abnova CD81 monoclonal antibody related parameters and Applications
如何让化工企业的ERP库存账目更准确
Composition of applet code
Nprogress plug-in progress bar
MySQL fully parses json/ arrays
Applet page navigation
手机开户股票开户安全吗?我家比较偏远,有更好的开户途径么?
Redis唯一ID生成器的实现
解读协作型机器人的日常应用功能
Web Service简单入门示例
Abnova DNA marker high quality control test program
leetcode:1755. 最接近目标值的子序列和
hdu2377Bus Pass(构建更复杂的图+spfa)
Material design component - use bottomsheet to show extended content (II)
渗透创客精神文化转化的创客教育
台风来袭!建筑工地该如何防范台风!
MYSQL IFNULL使用功能
Which securities is better for securities account opening? Is online account opening safe?