当前位置:网站首页>[AGC009D]Uninity
[AGC009D]Uninity
2022-07-06 11:17:00 【OneInDark】
subject
Ideas
For me , Super big problem , No idea at all ; Yes H I D \sf HID HID Come on , Garbage water problem , Make it casually .
The topic is preliminarily transformed : Build a class point tree , Minimize the height . If you build from top to bottom , There is a conclusion a n s ∈ { k , k + 1 } ans\in\{k,\;k{\rm+}1\} ans∈{ k,k+1}, However, it is completely useless . You can also consider the adjustment method of finding the center of gravity —— Obviously not. .
I thought again Bottom up Build up trees . The leaves of the original tree , There must be leaves on the dianfen tree . therefore , Adjacent to the leaves of the original tree , It is the penultimate layer of the dot tree . But further up ? I feel , If it could be 1 1 1 That's it 1 1 1 . Or license ; What about going up ? Because it is not formalized , I finally failed .
What to do in this topic , Namely Write the above thinking into a theorem . So it's the conclusion again, right . Only from the perspective of the above visual description , It's hard to clarify something .
Theorem : set up f : V ↦ N f:V\mapsto\N f:V↦N, That is, label each dot , Satisfy for any f ( x ) = f ( y ) ( x ≠ y ) f(x)=f(y)\;(x\ne y) f(x)=f(y)(x=y), There is z ∈ path ( x , y ) z\in\text{path}(x,y) z∈path(x,y) bring f ( z ) > f ( x ) f(z)>f(x) f(z)>f(x), remember v ( f ) = max f ( x ) v(f)=\max f(x) v(f)=maxf(x), The answer is min v ( f ) \min v(f) minv(f) .
Obviously, any legal solution corresponds to such a f f f . Next, just prove , In this way f f f In turn, a set of solutions can be constructed , And the answer of this solution does not exceed v ( f ) v(f) v(f) . Using induction , A point is obviously true ; When there are multiple points , find max f ( x ) \max f(x) maxf(x), Obviously this is the only . Take it as the root of the pointwise tree , Induction is applied to each connected block , The answer is no more than v ( f ′ ) ⩽ v ( f ) − 1 v(f')\leqslant v(f)-1 v(f′)⩽v(f)−1, So the answer of this plan does not exceed v ( f ′ ) + 1 ⩽ v ( f ) v(f')+1\leqslant v(f) v(f′)+1⩽v(f), Certificate completion .
then , We can Greedy recursive processing . Its source , Is the idea of building from the bottom up , But here we can give a more concise 、 More formal proof . set up y < f ( x ) y<f(x) y<f(x), If order f ( x ) = y f(x)=y f(x)=y after x x x The subtree of is still a legal point tree ( When only the points in the subtree are considered , Satisfying the theorem f f f mapping ), set up z z z by x x x Father in the tree , Then order again f ( z ) = max [ f ( z ) , f ( x ) ] f(z)=\max[f(z),f(x)] f(z)=max[f(z),f(x)] You can get a new legal point tree ( there f ( x ) f(x) f(x) Is the original mapping value ).
It is easy to verify the legitimacy of the scheme : Across x x x The path of , If you cross z z z It is still legal , because f ( z ) f(z) f(z) No less than the original f ( x ) f(x) f(x); No crossing z z z It is still legal , This is a prerequisite . No crossing x x x The path of , It must still be legal .
and v ( f ) v(f) v(f) It won't change . therefore f ( x ) f(x) f(x) The value of is unique , It just makes the subtree become the minimum value of the legal point tree . that , On the original tree d f s \tt dfs dfs, Store which in each subtree f ( x ) f(x) f(x) It needs to be avoided ( No bigger f f f Put it “ Occlusion ” live ) that will do . in consideration of f ( x ) ⩽ log n f(x)\leqslant\log n f(x)⩽logn, It can be compressed , Time complexity O ( n ) \mathcal O(n) O(n) .
Code
#include <cstdio> // JZM yydJUNK!!!
#include <iostream> // XJX yyds!!!
#include <algorithm> // XYX yydLONELY!!!
#include <cstring> // (the STRONG long for LONELINESS)
#include <cctype> // ZXY yydSISTER!!!
#include <vector>
using namespace std;
# define rep(i,a,b) for(int i=(a); i<=(b); ++i)
# define drep(i,a,b) for(int i=(a); i>=(b); --i)
typedef long long llong;
inline int readint(){
int a = 0, c = getchar(), f = 1;
for(; !isdigit(c); c=getchar())
if(c == '-') f = -f;
for(; isdigit(c); c=getchar())
a = (a<<3)+(a<<1)+(c^48);
return a*f;
}
inline void getMin(int &x, const int &y){
if(y < x) x = y;
}
const int MAXN = 100005;
struct Edge{
int to, nxt;
Edge() = default;
Edge(int _t, int _n): to(_t), nxt(_n){
}
};
Edge e[MAXN<<1]; int head[MAXN], cntEdge;
void addEdge(int a, int b){
e[cntEdge] = Edge(b,head[a]), head[a] = cntEdge ++;
}
int ans;
int dfs(int x, int pre){
int s = 0, bad = 0;
for(int i=head[x]; ~i; i=e[i].nxt){
if((i^1) == pre) continue;
const int &&ch = dfs(e[i].to,i);
bad |= s&ch, s |= ch;
}
int chose = bad ? 32-__builtin_clz(bad) : 0;
chose += __builtin_ffs((s>>chose)+1)-1;
ans = max(ans,chose); return ((s>>chose)|1)<<chose;
}
int main(){
int n = readint();
memset(head+1,-1,n<<2);
for(int i=1,a,b; i!=n; ++i){
a = readint(), b = readint();
addEdge(a,b), addEdge(b,a);
}
dfs(1,-1); printf("%d\n",ans);
return 0;
}
边栏推荐
- QT creator runs the Valgrind tool on external applications
- Some problems in the development of unity3d upgraded 2020 VR
- 解决安装Failed building wheel for pillow
- 02 staff information management after the actual project
- 连接MySQL数据库出现错误:2059 - authentication plugin ‘caching_sha2_password‘的解决方法
- Pytorch基础
- Development of C language standard
- Swagger, Yapi interface management service_ SE
- 学习问题1:127.0.0.1拒绝了我们的访问
- 01项目需求分析 (点餐系统)
猜你喜欢
AcWing 1298.曹冲养猪 题解
CSDN question and answer tag skill tree (I) -- Construction of basic framework
图片上色项目 —— Deoldify
MySQL主从复制、读写分离
Cookie setting three-day secret free login (run tutorial)
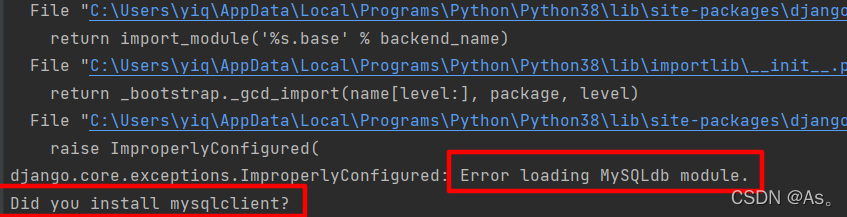
Django运行报错:Error loading MySQLdb module解决方法

MySQL master-slave replication, read-write separation

MySQL主從複制、讀寫分離
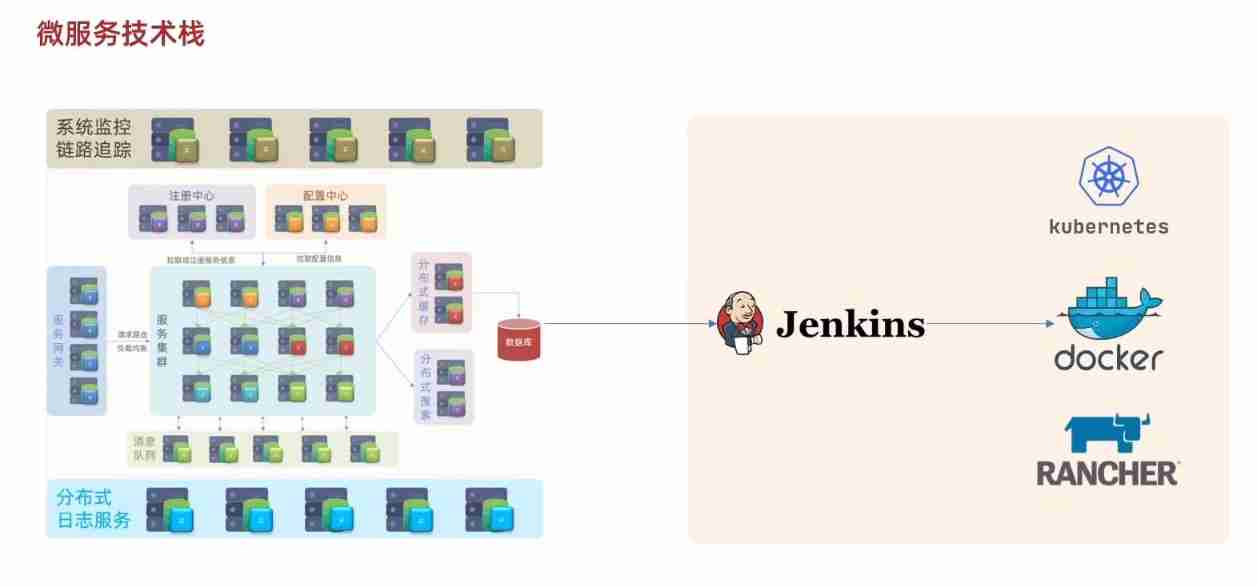
A brief introduction to the microservice technology stack, the introduction and use of Eureka and ribbon
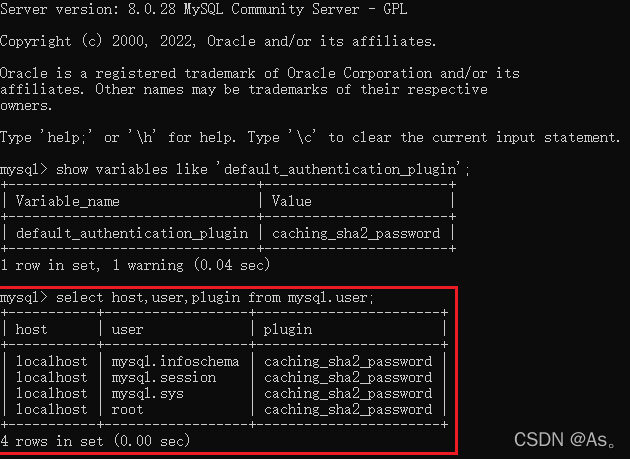
Error connecting to MySQL database: 2059 - authentication plugin 'caching_ sha2_ The solution of 'password'
随机推荐
CSDN Q & a tag skill tree (V) -- cloud native skill tree
QT creator custom build process
There are three iPhone se 2022 models in the Eurasian Economic Commission database
The virtual machine Ping is connected to the host, and the host Ping is not connected to the virtual machine
Ansible practical Series III_ Task common commands
Postman uses scripts to modify the values of environment variables
Are you monitored by the company for sending resumes and logging in to job search websites? Deeply convinced that the product of "behavior awareness system ba" has not been retrieved on the official w
Rhcsa certification exam exercise (configured on the first host)
打开浏览器的同时会在主页外同时打开芒果TV,抖音等网站
基于apache-jena的知识问答
02-项目实战之后台员工信息管理
Punctual atom stm32f103zet6 download serial port pin
MySQL主从复制、读写分离
Did you forget to register or load this tag
Swagger, Yapi interface management service_ SE
解决:log4j:WARN Please initialize the log4j system properly.
Armv8-a programming guide MMU (2)
图像识别问题 — pytesseract.TesseractNotFoundError: tesseract is not installed or it‘s not in your path
数据库高级学习笔记--SQL语句
1. Mx6u learning notes (VII): bare metal development (4) -- master frequency and clock configuration