Shortest path problem of graph theory (acwing template)

Catalog

Simple Dijkstra Algorithm ：

Dijkstra Templates ：

  Example ：Dijkstra Find the shortest path

Heap optimized dijkstra Algorithm

Example ：Dijkstra Find the shortest path Ⅱ

Bellman-Ford Algorithm （ Dealing with graphs with negative weighted edges ）

  Example ： The shortest path with limited number of edges

spfa Algorithm （Bellman-Ford Algorithm queue Optimization Algorithm ）

spfa Find the negative ring

Floyd Algorithm （ Multi source shortest path ）

---

A term is used to explain ：n： Usually represents points                          m： Usually represents the number of sides

Source ： The starting point                   Single source ： A starting point                   Multi-source ： Multiple starting points

Confluence ： End

The degree of ： How many sides point to me

The degree of ： The number of sides going out from this point

Border right ： In discrete mathematics or data structures , A numerical value of each edge band of a graph , The meaning he represents can be length and so on , This value is the edge weight

Frame diagram ：

Simple Dijkstra Algorithm ：

Because its time complexity is O（N^2） The number of edges is independent of time complexity , So it is suitable for dense graphs

A dense picture ： The number of sides is the number of points 2~3 times

ACwing Notes for the whole course of basic algorithm course （2021 year 8 month 12 Rewrite on the th + Optimize ）_hebtu_Kangweiqi The blog of -CSDN Blog _acwing note Basic algorithm Course Notes , Please support genuine https://blog.csdn.net/hebtu_Kangweiqi/article/details/109124329 Borrow the big guy's picture = =

Dijkstra Templates ：

  Example ：Dijkstra Find the shortest path

Given a n A little bit m Directed graph of strip edge , There may be double edges and self rings in the graph , All edge weights are positive .

Please find out 1 It's on the n The shortest distance of point No , If you can't get from 1 Go to No n Number point , The output -1

  Thinking analysis ： I feel greedy , Every time I choose the one with the smallest distance from me , Then most 1~j The shortest distance becomes

1~t Distance of points t~j The shortest distance

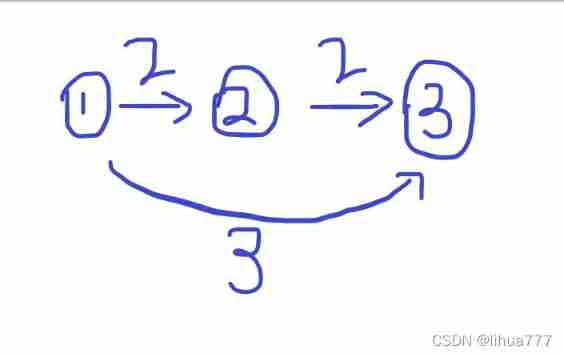
  I suggest you look at the code and simulate it on the diagram , You will feel the beauty of this algorithm ！！！

#include<iostream>
using namespace std;
const int N = 510;
int n, m;
int g[N][N];// Adjacency matrix is used to store 
int dist[N];// Distance array 
bool st[N];// Judge array 
int dijkstra() 
{
    memset(dist, 0x3f, sizeof dist);// The value of the initialization array is a large number 1e9
    dist[1] = 0;// Will be the first 1 The distance from point No. to itself is initialized to 0
    for (int i = 0; i < n; i++) 
    {//n Sub iteration 
        int t = -1;// Just a meaningless number ： The purpose is to find out 2~n Inner distance point 1 Minimum number 
        for (int j = 1; j <= n; j++)// from 1 To traverse the ： Pay attention to this detail 
        {
            if (!st[j] && (t == -1 || dist[j] < dist[t]))// If the point has not been passed 
            {
                t = j;// It is equivalent to finding the minimum value   1~1  1~2
            }
        }
        st[t] = 1;// The mark has been used at this point 
        for (int j = 1; j <= n; j++)// Traverse 1 It's on the n Number point 
        {// Update distance 
            dist[j] = min(dist[j], dist[t] + g[t][j]);//1~j The distance between points becomes ：1~t Distance of +t~j Distance of 
        }
    }
    if (dist[n] == 0x3f3f3f3f) return -1;// If n Point arrays have not been used , Then prove that you can't go n spot 
    else return dist[n];
}
int main() {
    cin >> n >> m;
    memset(g, 0x3f, sizeof g);
    while (m--)
    {
        int x, y, z;//x~y The distance to  z
        cin >> x >> y >> z;
        g[x][y] = min(g[x][y], z);
    }
    int t = dijkstra();
    printf("%d\n", t);
    return 0;
}

---

Heap optimized dijkstra Algorithm

  Through the analysis of the above figure ： We can 2 Of （1）（3） Optimize with heap to reduce time complexity , But the variable of the number of edges will be introduced , So heap optimized dijkstra Version is suitable for sparse drawings

Example ：Dijkstra Find the shortest path Ⅱ

problem ：

Given a n A little bit m Directed graph of strip edge , There may be double edges and self rings in the graph , All edge weights are nonnegative .

Please find out 1 It's on the n The shortest distance of point No , If you can't get from 1 Go to No n Number point , The output -1.

For sparse graphs , We use adjacency table

Code implementation ：

#include<iostream>
#include <queue>
using namespace std;

const int N = 1e6 + 10;
typedef pair<int, int> PII;
int h[N], e[2 * N], ne[2 * N], w[N], idx;// Adjacency list + Weight array 
int n, m;// points + Number of edges  
int dist[N];// Distance array 
bool st[N];// Judge array 

void add(int a, int b, int c) 
{
	e[idx] = b; 
	ne[idx] = h[a];
	w[idx] = c; 
	h[a] = idx++;
}
int dijkstra() 
{
	memset(dist, 0x3f, sizeof dist);// The initialization distance is a large number 
	dist[1] = 0;//1 The distance to oneself is 0
	priority_queue<PII, vector<PII>, greater<PII> >heap;// Build a little root pile （ Priority queue ）
	heap.push({ 0,1 });// Into the heap 
	while (heap.size())// When the queue is not empty 
	{
		auto t = heap.top();// Take the head 
		heap.pop();// Truncate 
		int ver = t.second, distance = t.first;//first： distance ,ver： Number of nodes 
		if (st[ver]) continue;// If the node has been marked , I will not go 
		st[ver] = 1;// Tag nodes 
		for (int i = h[ver]; i != -1; i = ne[i])// Traversal of adjacency table  
		{
			int j = e[i];// Get the number of nodes 
			if (dist[j] > distance + w[i])// Find the minimum distance 
			{
				dist[j] = distance + w[i];// node 1 To node of j The distance of is the distance of the head node + Its weight （ Side length ）
				heap.push({ dist[j],j });// Into the heap 
			}
		}
	}
	if (dist[n] == 0x3f3f3f3f) return -1;
	return dist[n];
}
int main() {
	cin >> n >> m;
	memset(h, -1, sizeof h);
	while (m--) 
	{
		int x, y, z;
		cin >> x >> y >> z;
		add(x, y, z);
	}
	cout << dijkstra();
}

---

Bellman-Ford Algorithm （ Dealing with graphs with negative weighted edges ）

1、 What is? bellman - ford Algorithm ？
Bellman - ford The algorithm is an algorithm for finding the shortest path of a single source with a negative weight graph , Low efficiency , The code is less difficult . The principle is continuous relaxation , Update each edge every time you relax , If in n-1 It can be renewed after relaxation , Then it shows that there is a negative ring in the figure , Therefore, no results can be obtained , Otherwise, complete .
( Generally speaking, it's ： hypothesis 1 It's on the n Point number is accessible , Every point starts in the same direction , Update the shortest distance between adjacent points , Through the loop n-1 operations , If there is no negative ring in the graph , be 1 Point number is bound to arrive n Number point , If there is a negative ring in a graph , It's in n-1 It's bound to be updated after a second relaxation )

2、bellman - ford The specific steps of the algorithm
for n Time
for All sides a,b,w ( Slack operation )
dist[b] = min(dist[b],back[a] + w)

Be careful ：back[] The array is after the last iteration dist[] Backup of array , Because every point starts out at the same time , So you need to dist[] Array for backup , If you don't do a backup, there will be a cascading effect , To the next point

3、 In the following code , Whether we can reach n In the judgment of No if(dist[n] > INF/2) Judge , It is not. if(dist[n] == INF) Judge , as a result of INF It's a definite value , It's not really infinity , Will be affected by other values ,dist[n] Greater than one with INF The same order of magnitude

4、bellman - ford The algorithm is good at solving the shortest path problem with limited number of edges
Time complexity O ( n m ) O(nm)O(nm)
among n For points ,m Number of sides
————————————————
Copyright notice ： This paper is about CSDN Blogger 「 Cloud algorithm 」 The original article of , follow CC 4.0 BY-SA Copyright agreement , For reprint, please attach the original source link and this statement .
Link to the original text ：https://blog.csdn.net/hebtu_Kangweiqi/article/details/109124329

  Example ： The shortest path with limited number of edges

problem ：

Given a n A little bit m Directed graph of strip edge , There may be double edges and self rings in the graph ,  The edge weight may be negative .

Please find out from 1 It's on the n The maximum passage of point No k The shortest distance of the edge , If you can't get from 1 Go to No n Number point , Output impossible         Be careful ： Possible in the figure   There is a negative weight loop

#include <iostream>
#include <cstring>

using namespace std;

const int N = 1e4 + 50;

int n, m, k;
int dis[N], backup[N];

struct edge { int a, b, w; } e[N];

void bellman()
{
    memset(dis, 0x3f, sizeof dis);
    dis[1] = 0;
    
    for (int i = 0; i < k; i ++)// At most  k  side 
    {
        memcpy(backup, dis, sizeof dis);// Update every time with the last status 
        for (int j = 1; j <= m; j ++)
        {
            int a = e[j].a, b = e[j].b, w = e[j].w;
            dis[b] = min(dis[b], backup[a] + w);// To b The distance between points is equal to a Point distance plus side length 
        }
    }
}

int main()
{
    cin >> n >> m >> k;
    
    for (int i = 1; i <= m; i ++)
        cin >> e[i].a >> e[i].b >> e[i].w;
    
    bellman();
    // Whether we can reach n In the judgment of No if(dist[n] > INF/2) Judge , It is not. if(dist[n] == INF) Judge , as a result of INF It's a definite value , It's not really infinity , Will be affected by other values ,dist[n] Greater than one with INF The same order of magnitude 
    if (dis[n] > 0x3f3f3f3f / 2) puts("impossible");
    else cout << dis[n] << endl;
    
    return 0;
}

---

spfa Algorithm （Bellman-Ford Algorithm queue Optimization Algorithm ）

spfa Algorithm steps 
queue <– 1
while queue  Not empty 
(1) t <–  Team head 
queue.pop()
(2) use  t  Update all outgoing edges  t –> b, A weight of w
queue <– b ( If the point has been updated , Use this point to update other points )

Time complexity commonly ：O ( m ) The worst ：O ( n m )
n For points ,m Number of sides

3、spfa It can also solve the shortest distance problem of graphs with positive weights , And in general, it's better than Dijkstra The algorithm is OK
————————————————
Copyright notice ： This paper is about CSDN Blogger 「 Cloud algorithm 」 The original article of , follow CC 4.0 BY-SA Copyright agreement , For reprint, please attach the original source link and this statement .
Link to the original text ：https://blog.csdn.net/hebtu_Kangweiqi/article/details/109124329

problem ：

The shortest path with limited number of edges

Given a n A little bit m Directed graph of strip edge , There may be double edges and self rings in the graph ,  The edge weight may be negative .

Please find out from 1 It's on the n The maximum passage of point No k The shortest distance of the edge , If you can't get from 1 Go to No n Number point , Output impossible.

Be careful ： Possible in the figure   There is a negative weight loop  .

#include<iostream>
#include<queue>
using namespace std;

const int N = 1e5 + 5;
int n, m, dis[N];
bool st[N];// Whether the representative point is in the queue 
int h[N], e[N], ne[N], w[N], idx;// Single chain list 
void add(int a, int b, int c)// Insert operation of single chain table 
{
	e[idx] = b; 
	ne[idx] = h[a]; 
	w[idx] = c; 
	h[a] = idx++;
}
int spfa() 
{
	queue<int> q;
	memset(dis, 0x3f, sizeof dis);
	q.push(1);// Put in node 1
	dis[1] = 0;// A distance of 1
	st[1] = 1;// Judge 
	while (!q.empty()) // The queue is not empty 
	{
		int u = q.front();// Take out the head 
		q.pop();// Truncate 
		st[u] = 0;// Mark 
		for (int i = h[u]; i != -1; i = ne[i])// Traversing the linked list 
		{
			int v = e[i];// Take out node 
			if (dis[v] > dis[u] + w[i])// For the minimum  
			{
				dis[v] = dis[u] + w[i];
				if (!st[v]) // If not 
				{
					q.push(v);// Put in 
					st[v] = 1;// Mark 
				}
			}
		}
	}
	if (dis[n] == 0x3f3f3f3f) return -1;
	else return dis[n];
}
int main() {
	memset(h, -1, sizeof h);
	scanf("%d%d", &n, &m);
	while (m--) {
		int x, y, z;
		scanf("%d%d%d", &x, &y, &z);
		add(x, y, z);
	}
	int t = spfa();
	if (t == -1) puts("impossible");
	else printf("%d", t);
}

---

spfa Find the negative ring

problem ：

Given a n A little bit m Directed graph of strip edge , There may be double edges and self rings in the graph ,  The edge weight may be negative

Please judge whether there is a negative weight circuit in the figure

#include<iostream>
#include<queue>
using namespace std;
const int N = 2005, M = 10005;
int h[N], e[M], ne[M], w[M], idx, n, m;
int dis[N], cnt[N];// Need an extra cnt Array , Record the number of sides on the shortest path at the current point 
bool st[N];
void add(int a, int b, int c) 
{
	e[idx] = b; 
	w[idx] = c; 
	ne[idx] = h[a];
	h[a] = idx++;
}
bool spfa() 
{
	/* First of all, the distance does not need to be initialized */
	queue<int> q;
	/* Join all points in the team , It can prevent some points from going to the negative ring */
	for (int i = 1; i <= n; i++) 
	{
		q.push(i);
		st[i] = 1;
	}
	while (!q.empty()) 
	{
		int u = q.front();
		q.pop();
		st[u] = 0;
		for (int i = h[u]; i != -1; i = ne[i]) 
		{
			int v = e[i];
			if (dis[v] > dis[u] + w[i]) 
			{
				dis[v] = dis[u] + w[i];
				cnt[v] = cnt[u] + 1;
				if (cnt[v] >= n) return 1;
				/* If exceeded n, According to the drawer principle , The number of points passed in the middle must be greater than n,*/
				if (!st[v]) {
					q.push(v);
					st[v] = 1;
				}
			}
		}
	}
	return 0;
}
int main() {
	scanf("%d%d", &n, &m);
	memset(h, -1, sizeof h);
	for (int i = 0; i < m; i++) {
		int x, y, z;
		scanf("%d%d%d", &x, &y, &z);
		add(x, y, z);
	}
	if (spfa()) puts("Yes");
	else puts("No");
}

---

Floyd Algorithm （ Multi source shortest path ）

problem ：

Given a n A little bit m Directed graph of strip edge , There may be double edges and self rings in the graph , The edge weight may be negative .

Re given k A asked , Each query contains two integers x and y, Indicates that the query is from point x point-to-point y The shortest distance , If the path doesn't exist , The output “impossible”.

There is no negative weight loop in the data assurance diagram

#include<iostream>
using namespace std;
const int N = 210;
const int inf = 0x3f3f3f3f;
int n, m, q;
int d[N][N];
void floyd() {
	for (int k = 1; k <= n; k++) 
	{
		for (int i = 1; i <= n; i++) 
		{
			for (int j = 1; j <= n; j++) 
			{
				d[i][j] = min(d[i][j], d[i][k] + d[k][j]);
			}
		}
	}
}
int main() {
	scanf("%d%d%d", &n, &m, &q);
	/* initialization */
	for (int i = 1; i <= n; i++) 
	{
		for (int j = 1; j <= n; j++) 
		{
			if (i == j) d[i][j] = 0;
			else d[i][j] = inf;
		}
	}

	while (m--)
	{
		int x, y, z;
		scanf("%d%d%d", &x, &y, &z);
		d[x][y] = min(d[x][y], z);// Pay attention to the double edge 
	}
	floyd();
	while (q--) //q Time to ask 
	{
		int x, y;
		scanf("%d%d", &x, &y);
		if (d[x][y] > inf / 2) puts("impossible");// The problem may appear negative weight side , So we need to apply the previous routine 
		else printf("%d\n", d[x][y]);
	}
	return 0;
}

---