Understanding rotation matrix R from the perspective of base transformation

In understanding the camera coordinate system , We will definitely touch the camera's external parameter matrix R, It converts coordinates from the world coordinate system to the camera coordinate system ：
$$P_c=R\ast P_w+t$$
This is actually a transformation between two coordinate systems , We know $R$ A matrix is an orthogonal matrix , So it's 3 Row （ Column ） The vector is 3 A set of orthonormal bases in a vector space , And a set of orthonormal bases can be used as three basis vectors of a coordinate system . So our $R$ How does a matrix relate to the basis vectors of two coordinate systems ？

Let's draw two coordinate systems first $X_w{Y}_w{Z}_w$ and $X_c{Y}_c{Z}_c$：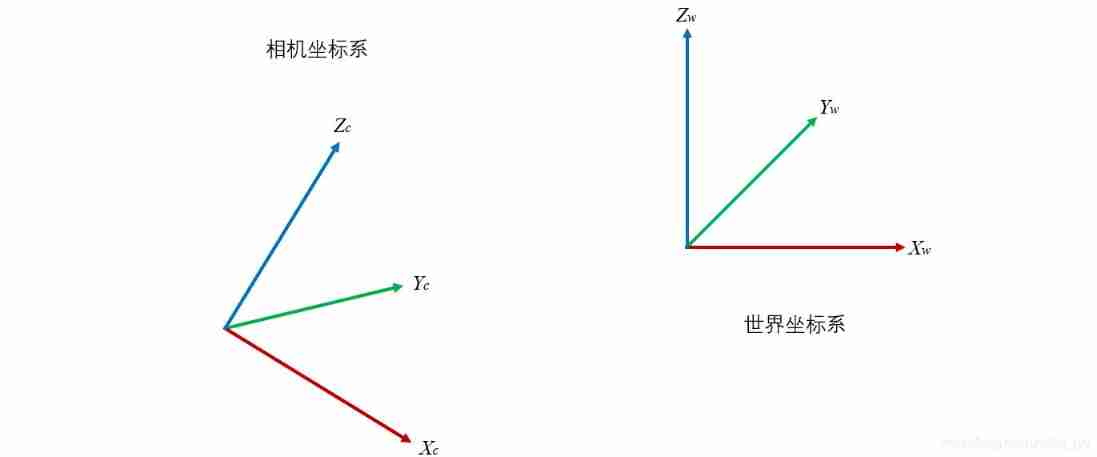

We're going to talk about how to put a certain point $P$ Coordinates in the world coordinate system are converted into coordinates in the camera coordinate system .

Let's not consider the translation between the two coordinate systems , So move the origin of the camera coordinate system to the origin of the world coordinate system , like this ：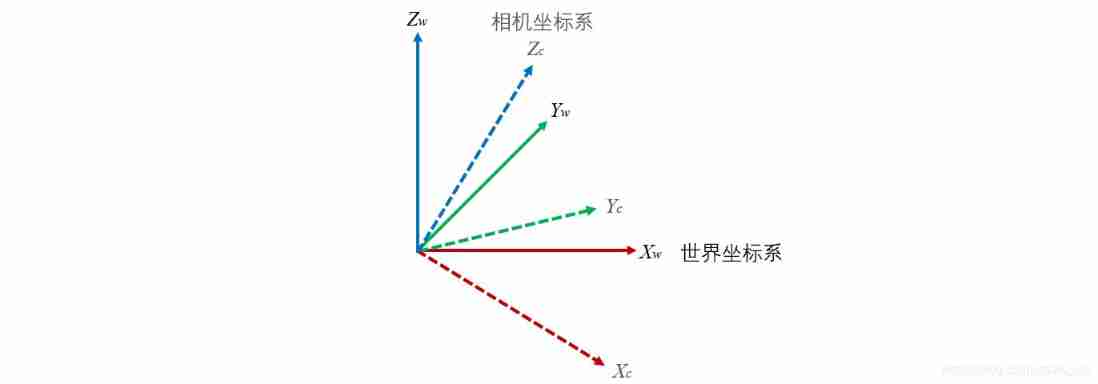

We can mark the set of basis vectors of two coordinate systems $e_w({\vec{e}}_{wx},{\vec{e}}_{wy},{\vec{e}}_{wz})$ and $e_c({\vec{e}}_{cx},{\vec{e}}_{cy},{\vec{e}}_{cz})$. They're all in the world coordinate system .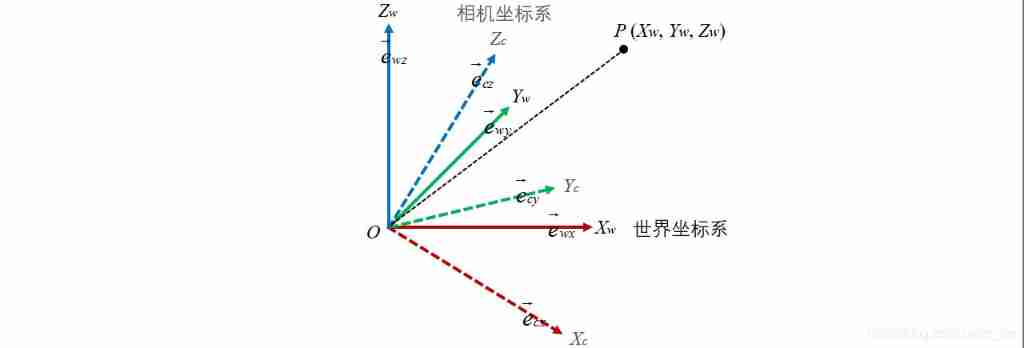

Next , And how to put a point in the world coordinate system $P(X_w,Y_w,Z_w)$ Convert to camera coordinates
$$P(X_w,Y_w,Z_w)\to P(X_c,Y_w,Z_w)$$
In the world coordinate system , Basis vector set $e_w({\vec{e}}_{wx},{\vec{e}}_{wy},{\vec{e}}_{wz})$ Is the unit matrix , That is to say

among ${\vec{e}}_{wx}=(1,0,0{)}^T$,${\vec{e}}_{wy}=(0,1,0{)}^T$,${\vec{e}}_{wz}=(0,0,1{)}^T$.

We know $P$ The coordinates in the world coordinate system are actually a linear combination of the above three sets of basis vectors , namely $P_w=X_w\ast {\vec{e}}_{wx}+Y_w\ast {\vec{e}}_{wx}+Z_w\ast {\vec{e}}_{wx}$

This is the base vector representation of coordinates .

So let's take $P$ The coordinates of the points are transformed into a set of basis vectors $e_c({\vec{e}}_{cx},{\vec{e}}_{cy},{\vec{e}}_{cz})$ Then we get the transformation in the camera coordinate system . let me put it another way , We're going to calculate $P$ The point is in the base vector set $e_c({\vec{e}}_{cx},{\vec{e}}_{cy},{\vec{e}}_{cz})$ The coordinates under $P_c=X_c\ast {\vec{e}}_{cx}+Y_c\ast {\vec{e}}_{cx}+Z_c\ast {\vec{e}}_{cx}$.

From the perspective of the rotation matrix , The formula is ：
$$P_c=R{P}_w$$

Let's forget for a moment $P$, Let's think about the set of basis vectors $e_c({\vec{e}}_{cx},{\vec{e}}_{cy},{\vec{e}}_{cz})$ adopt $R$ What does a matrix look like in camera coordinates ？

The answer is obvious , It's a unit array $E$.

That is to say, by left multiplying the rotation matrix $R$, We can set the basis vectors $e_c({\vec{e}}_{cx},{\vec{e}}_{cy},{\vec{e}}_{cz})$ Into a unit matrix $E$, The expression is as follows ：
$$R({\vec{e}}_{cx},{\vec{e}}_{cy},{\vec{e}}_{cz})=E$$

So we know
$$({\vec{e}}_{cx},{\vec{e}}_{cy},{\vec{e}}_{cz})=R^{-1}=R^T$$

This is our rotation matrix $R$ Understanding from the perspective of basis transformation ,$R$ The inverse matrix （ Or transpose matrix ） Three column vectors of , It is the coordinates of the three base vectors of the camera coordinate system in the world coordinate system .