当前位置:网站首页>Visual Studio 插件之CodeMaid自动整理代码
Visual Studio 插件之CodeMaid自动整理代码
2022-07-07 17:31:00 【执着的涛】
介绍
当我们平时 coding 时,代码的格式千奇百怪,不知道快捷键的同学,可能还在徒手敲敲空格或者缩进。知道快捷的同学,每次写完后,会按下快捷键,单有时还会忘记,使得阅读代码时不太便于理解,更重要的是也影响了我们的开发效率。CodeMaid 的出现,可以快速解放你的双手,提高你的开发效率,自动格式化你的代码、清理排序命名空间,当然他也是免费开源的。
Visual Studio神级插件之CodeMaid自动整理你的代码_菜鸟厚非的博客-CSDN博客_codemaid怎么使用
边栏推荐
- Netease Yunxin participated in the preparation of the standard "real time audio and video service (RTC) basic capability requirements and evaluation methods" issued by the Chinese Academy of Communica
- 怎么在手机上买股票开户 股票开户安全吗
- 关于ssh登录时卡顿30s左右的问题调试处理
- Kirin Xin'an cloud platform is newly upgraded!
- 9 atomic operation class 18 Rohan enhancement
- PV静态创建和动态创建
- 解决rosdep的报错问题
- 一锅乱炖,npm、yarn cnpm常用命令合集
- 凌云出海记 | 赛盒&华为云:共助跨境电商行业可持续发展
- 杰理之关于 TWS 声道配置【篇】
猜你喜欢

Kunpeng developer summit 2022 | Kirin Xin'an and Kunpeng jointly build a new ecosystem of computing industry
PV static creation and dynamic creation
让这个 CRMEB 单商户微信商城系统火起来,太好用了!

In the first half of 2022, I found 10 books that have been passed around by my circle of friends
多个kubernetes集群如何实现共享同一个存储
2022.07.04
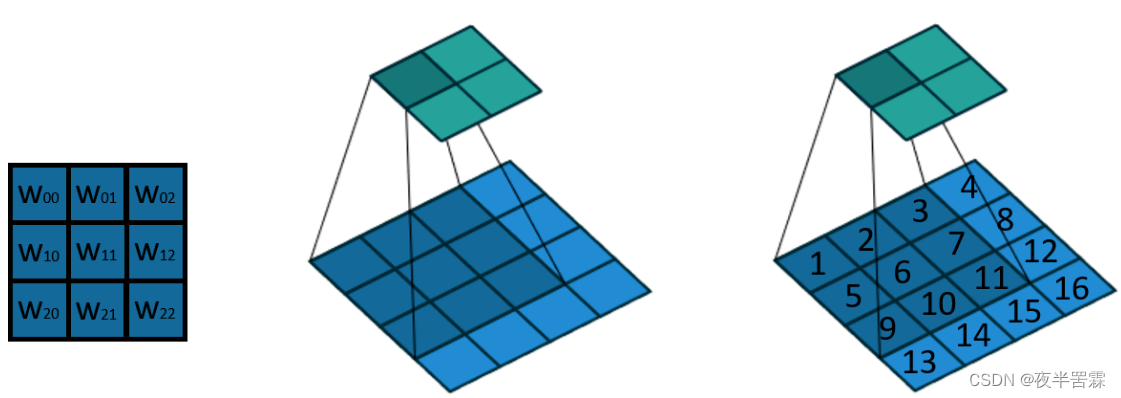
转置卷积理论解释(输入输出大小分析)
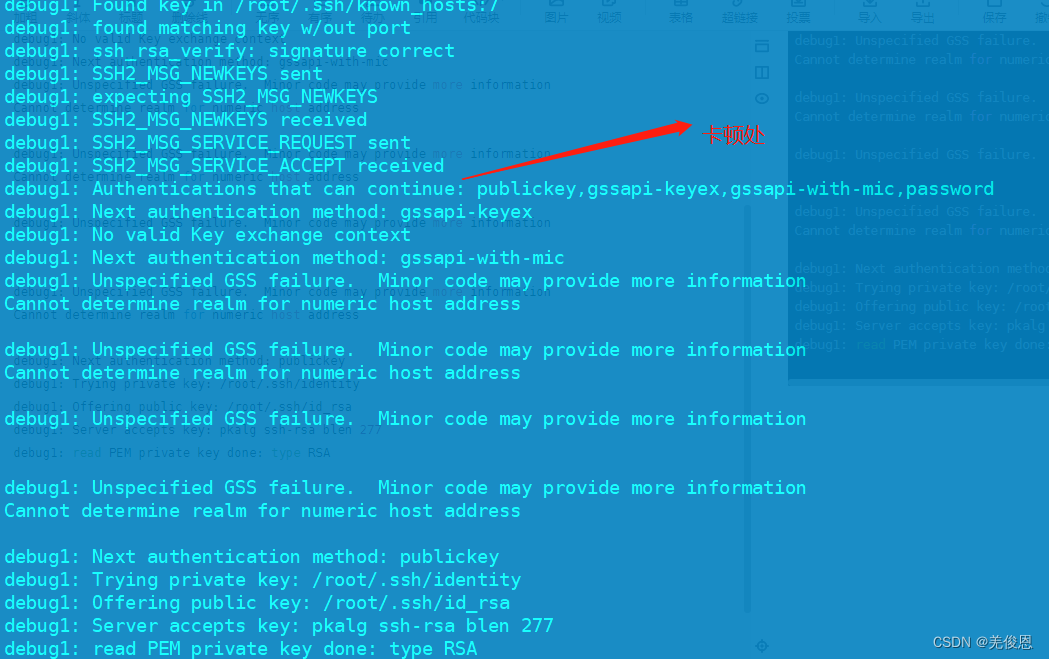
关于ssh登录时卡顿30s左右的问题调试处理
Le PGR est - il utile au travail? Comment choisir une plate - forme fiable pour économiser le cœur et la main - d'œuvre lors de la préparation de l'examen!!!

索引总结(突击版本)
随机推荐
杰理之开机自动配对【篇】
L1-019 who falls first (Lua)
2022年投资哪个理财产品收益高?
杰理之关于 TWS 声道配置【篇】
2022如何评估与选择低代码开发平台?
【Confluence】JVM内存调整
歌单11111
杰理之相同声道的耳机不允许配对【篇】
IP tools
杰理之快速配对,不支持取消配对【篇】
Solve the problem of remote rviz error reporting
PMP practice once a day | don't get lost in the exam -7.7
Where does brain hole come from? New research from the University of California: creative people's neural connections will "take shortcuts"
R语言dplyr包mutate_at函数和min_rank函数计算dataframe中指定数据列的排序序号值、名次值、将最大值的rank值赋值为1
Dynamic addition of El upload upload component; El upload dynamically uploads files; El upload distinguishes which component uploads the file.
tp6 实现佣金排行榜
Hongmeng smart home [1.0]
L1-023 output gplt (Lua)
位运算介绍
R语言使用ggplot2函数可视化需要构建泊松回归模型的计数目标变量的直方图分布并分析构建泊松回归模型的可行性