当前位置:网站首页>Binary tree high frequency question type
Binary tree high frequency question type
2022-07-07 09:24:00 【Fly upward】
Catalog
1. The number of nodes in a complete binary tree
3. For the first K The number of layer nodes
4. The maximum depth of a binary tree
5. Find binary tree value Whether there is
6. Whether it is a complete binary tree
8. The subtree of another tree
11. Sequence traversal of binary tree
12. The nearest common ancestor of a binary search tree
13. Binary search tree Convert to a sorted two-way linked list
1. The number of nodes in a complete binary tree
Here's a tree for you Perfect binary tree The root node root , Find out the number of nodes of the tree .
Perfect binary tree Is defined as follows : In a complete binary tree , Except that the lowest node may not be full , The number of nodes in each layer reaches the maximum , And the nodes of the lowest layer are concentrated in the leftmost positions of the layer . If the bottom layer is No h layer , Then the layer contains 1~ 2h Nodes .
Title source : Power button (LeetCode)
link :https://leetcode.cn/problems/count-complete-tree-nodes

class Solution {
//1. Traversal ideas
int count = 0;
public int countNodes1(TreeNode root) {
if (root == null){
return 0;
}
count++;
countNodes(root.left);
countNodes(root.right);
return count;
}
//2 Sub problem ideas
public int countNodes(TreeNode root) {
if (root == null){
return 0;
}
return countNodes(root.left) + countNodes(root.right) + 1;
}
}2. Number of leaf nodes
//1. Traversal ideas
int leafCount = 0;
int getLeafNodeCount1(TreeNode root) {
if (root == null) {
return 0;
}
if (root.left == null && root.right == null) {
leafCount++;
}
getLeafNodeCount(root.left);
getLeafNodeCount(root.right);
return leafCount;
}
//2. Sub problem ideas
int getLeafNodeCount(TreeNode root) {
if (root == null) {
return 0;
}
if (root.left == null && root.right == null) {
return 1;
}
return getLeafNodeCount(root.left) + getLeafNodeCount(root.right);
}
3. For the first K The number of layer nodes
/**
* For the first K The number of layer nodes
* Sub problem ideas
*/
int GetKLeveNodeCount(TreeNode root, int k) {
if (root == null) {
return 0;
}
if (k == 1) {
return 1;
}
return GetKLeveNodeCount(root.left, k-1) + GetKLeveNodeCount(root.right, k-1);
}4. The maximum depth of a binary tree
Given a binary tree , Find out the maximum depth .
The depth of a binary tree is the number of nodes in the longest path from the root node to the farthest leaf node .
explain : A leaf node is a node that has no children .

Title source : Power button (LeetCode)
public int maxDepth(TreeNode root) {
if (root == null) {
return 0;
}
int leftDepth = maxDepth(root.left);
int rightDepth = maxDepth(root.right);
return leftDepth > rightDepth ? leftDepth+1 : rightDepth+1;
}5. Find binary tree value Whether there is
TreeNode find(TreeNode root,char val) {
if (root == null) {
return null;
}
if (root.val == val) {
return root;
}
TreeNode ret = final (root.left, val);
if (ret != null) {
return ret;
}
ret = final (root.right, val);
if (ret != null) {
return ret;
}
return null;
}6. Whether it is a complete binary tree
boolean isCompleteTree(TreeNode root) {
if (root == null) {
return true;
}
Queue<TreeNode> queue = new LinkedList;
// In the queue
queue.offer(root);
while (!queue.isEmpty()) {
TreeNode cur = queue.poll();
if (cur != null) {
queue.offer(left);
queue.offer(right);
}else {
break;
}
}
// Outgoing queue
while (!queue.isEmpty()) {
TreeNode top = queue.peek();
if (top != null) {
return false;
}
queue.poll();
}
return true;
}7. Same tree
Here are the root nodes of two binary trees p and q , Write a function to check whether the two trees are the same .
If two trees are the same in structure , And the nodes have the same value , They are the same .
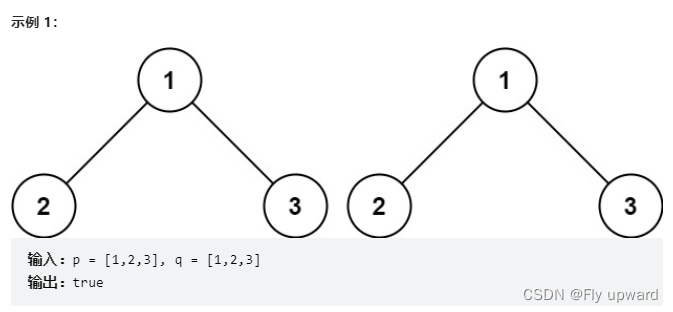
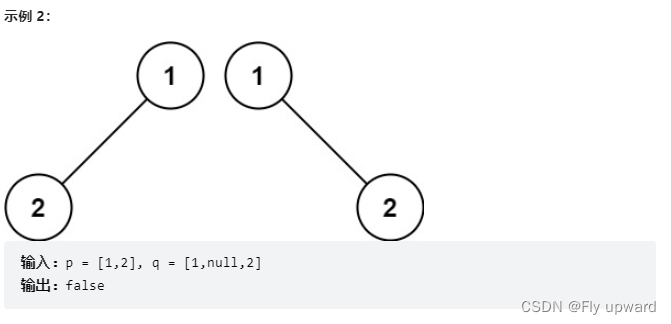
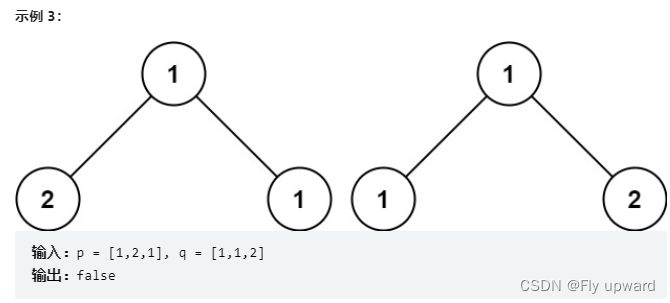
Title source : Power button (LeetCode)
public boolean isSameTree(TreeNode p, TreeNode q) {
if (p == null && q != null || p != null && q == null) {
return false;
}
if (p == null && q == null) {
return true;
}
if (q.val != p.val) {
return false;
}
return isSameTree(p.left,q.left) && isSameTree(p.right,q.right);
}8. The subtree of another tree
Here are two binary trees root and subRoot . test root Whether and subRoot Subtrees with the same structure and node values . If there is , return true ; otherwise , return false .
Binary tree tree A subtree of includes tree A node of and all descendants of this node .tree It can also be seen as a subtree of its own .
Title source : Power button (LeetCode)
link :https://leetcode.cn/problems/subtree-of-another-tree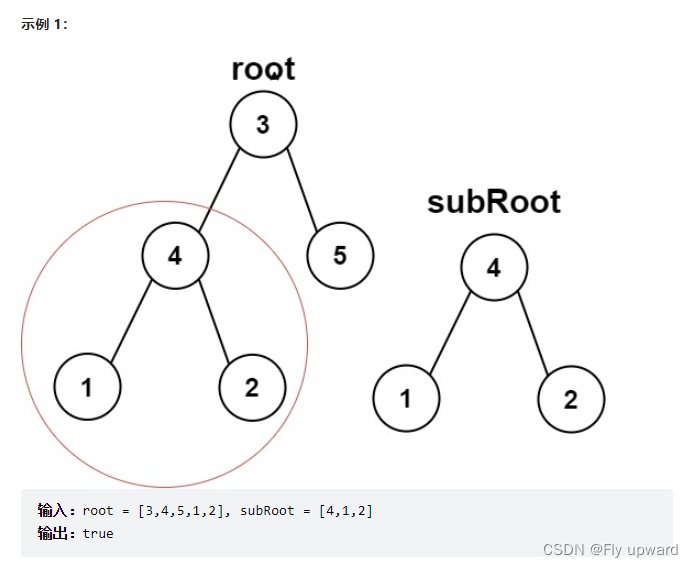
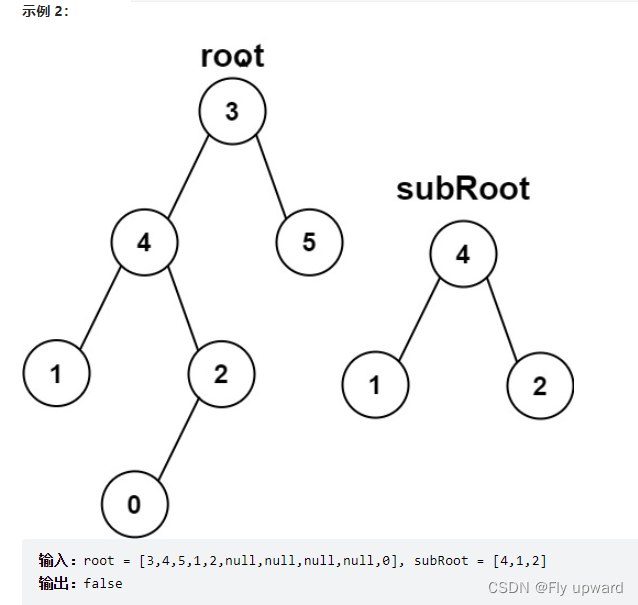
public boolean isSubtree(TreeNode root,TreeNode subRoot) {
if (root == null || subRoot == null) {
return false;
}
// Root node and subroot Are there two same trees
if (isSameTree(root,subRoot)) {
return true;
}
//subRoot Is it right? root The left subtree
if (isSubtree(root.left,subRoot)) {
return true;
}
if (isSubtree(root.right,subRoot)) {
return true;
}
return false;
}9. Balanced binary trees
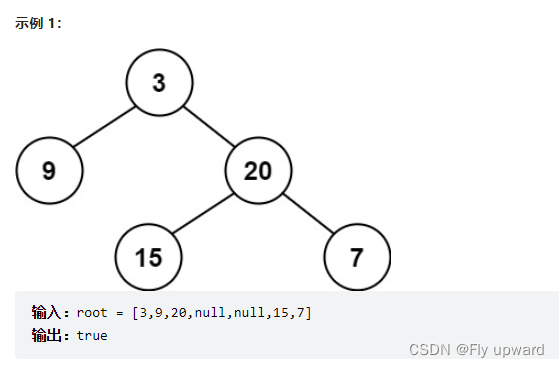
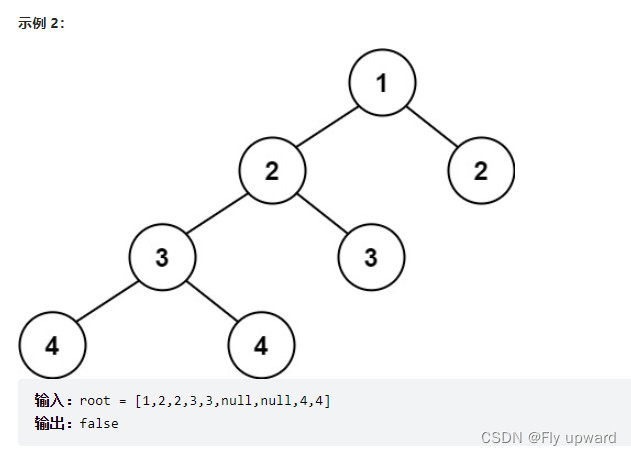
Given a binary tree , Determine if it's a highly balanced binary tree .
In this question , A height balanced binary tree is defined as :
A binary tree Every node The absolute value of the height difference between the left and right subtrees is not more than 1
Title source : Power button (LeetCode)
Solution 1
//1. First check the depth
public int height(TreeNode root) {
if (root == null) {
return 0;
}
int leftHeight = height(root.left);
int rightHeight = height(root.right);
return (leftHeight > rightHeight) ? (leftHeight + 1) : (rightHeight + 1);
}
//2. Check the depth difference between the left and right subtrees 1 Is balanced
public boolean isBalance(TreeNode root) {
if (root == null) {
return true;
}
int left = height(root.left);
int right = height(root.right);
return Math.abs(left - right) <= 1 && isBalanced(root.left) && isBalanced(root.right);
}
Solution 2
public int height(TreeNode root) {
if (root == null) {
return 0;
}
int leftHeight = height(root.left);
int rightHeight = height(root.right);
if (leftHeight >= 0 && rightHeight >= 0 && Math.abs(leftHeight-rightHeight) <= 1) {
return Math.max(leftHeight,rightHeight) + 1;
}else {
// Explain the imbalance
return -1;
}
}
public boolean isBalance(TreeNode root) {
if (root == null) {
return true;
}
return height(root) >= 0;
}
10. Symmetric binary tree
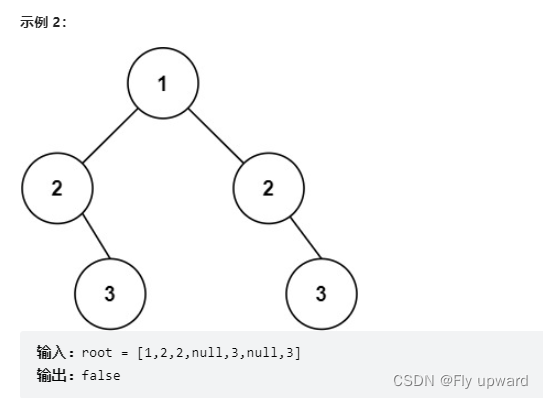
Give you the root node of a binary tree root , Check whether it is axisymmetric .

Title source : Power button (LeetCode)
public boolean isSymmetricChild(TreeNode leftTree, TreeNode rightTree) {
if (leftTree == null && rightTree != null) return false;
if (leftTree != null && rightTree == null) return false;
if (leftTree == null && rightTree == null) return true;
if (leftTree.val != rightTree.val) return false;
return isSymmetricChild(leftTree.left,rightTree.right) &&
isSymmetricChild(leftTree.right,rightTree.left);
}
public boolean isSymmetric(TreeNode root) {
if (root == null) {
return true;
}
return isSymmetricChild(root.left ,root.right);
}
11. Sequence traversal of binary tree
Give you the root node of the binary tree root , Returns the Sequence traversal . ( That is, layer by layer , Access all nodes from left to right )
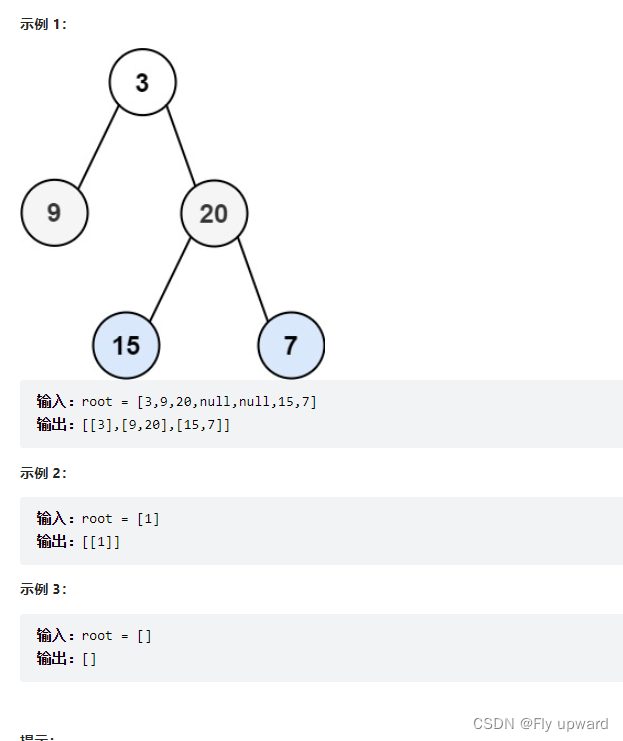
Title source : Power button (LeetCode)
public List<List<Integer>> levelOrder(TreeNode root) {
List<List<Integer>> ret = new ArrayList<>();
if (root == null) return ret;
Queue<TreeNode> queue = new LinkedList<>();
queue.offer(root);
while (!queue.isEmpty) {
int size = queue.size();// How many nodes are there in the current layer
List<Integer> lst = new ArrayList<>();
while (size != 0) {
TreeNode cur = queue.poll();
list.add(cur.val);
if (cur.left != null) {
queue.offer(cur.left);
}
if (cur.right != null) {
queue.offer(cur.right);
}
size--;
}
ret.add(list);
}
return ret;
}
12. The nearest common ancestor of a binary search tree
Given a binary tree , Find the nearest common ancestor of the two specified nodes in the tree .
In Baidu Encyclopedia, the most recent definition of public ancestor is :“ For a tree T Two nodes of p、q, Recently, the common ancestor is represented as a node x, Satisfy x yes p、q Our ancestors and x As deep as possible ( A node can also be its own ancestor ).
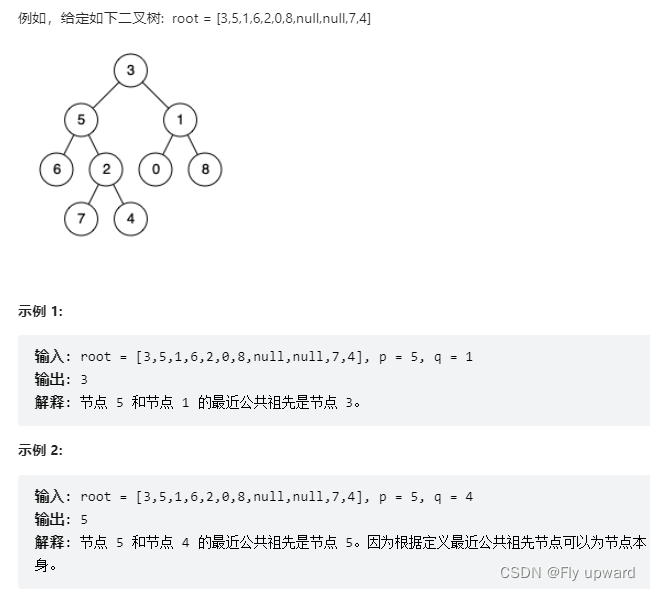
source : Power button (LeetCode)
link :https://leetcode.cn/problems/er-cha-shu-de-zui-jin-gong-gong-zu-xian-lcof
public TreeNode lowestCommonAncestor(TreeNode root, TreeNode p, TreeNode q) {
if (`root == null) {
return null;
}
if (root == p || root == q) {
return root;
}
TreeNode leftT = lowestCommonAncestor(root.left,p,q);
TreeNode rightT = lowestCommonAncestor(root.right,p,q);
if (leftT != null && rightT != null) {
return root;
}else if (leftT != null) {
return leftT;
}else {
return rightT;
}
}13. Binary search tree Convert to a sorted two-way linked list
TreeNode prev = null;
public void inorder(TreeNode pCur) {
if (pCur == null) {
return;
}
inorder(pCur.left);
pCur.left = prev;
if (prev != null) {
prev.right = pCur;
}
prev = pCur;
inorder(pCur.right);
}
public TreeNode Convert(TreeNode pRootOfTree) {
if (pRootOfTree == null) {
return null;
}
inorder(pRootOfTree);
TreeNode head = pRootOfTree;
while (head.left != null) {
head = head.left;
}
return head;
}边栏推荐
- [chaosblade: node CPU load, node network delay, node network packet loss, node domain name access exception]
- 数据库多表关联查询问题
- Unity shader (to achieve a simple material effect with adjustable color attributes only)
- Jmeters use
- JVM 内存结构 详细学习笔记(一)
- What are the conditions for applying for NPDP?
- 2020 year end summary
- Windows starts redis service
- Colorbar of using vertexehelper to customize controls (II)
- PMP certificate preparation experience sharing
猜你喜欢
Confitest of fixture py
超十万字_超详细SSM整合实践_手动实现权限管理
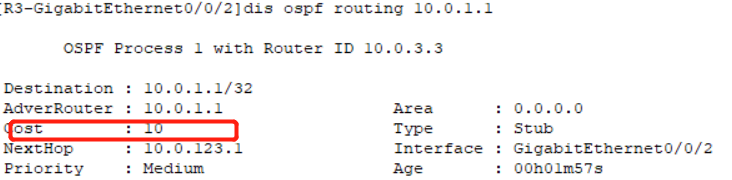
Huawei hcip datacom core_ 03day
flex弹性布局
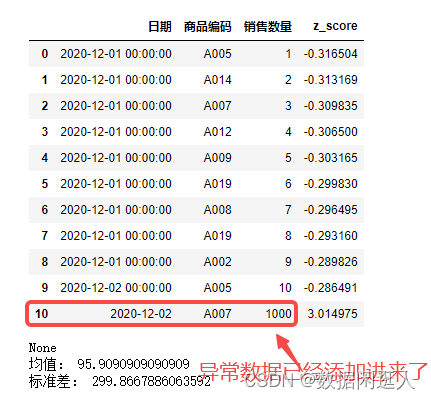
数据建模中利用3σ剔除异常值进行数据清洗
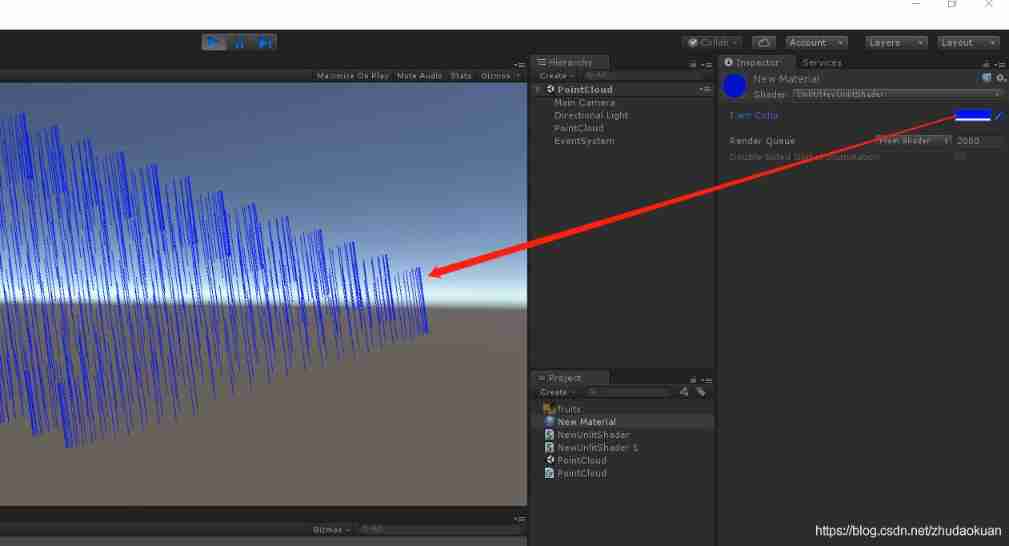
Unity shader (to achieve a simple material effect with adjustable color attributes only)
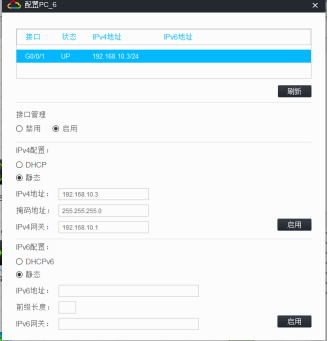
H3C vxlan configuration
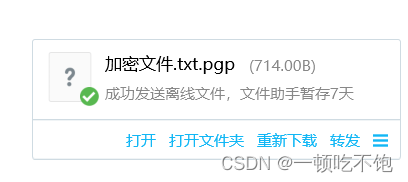
信息安全实验三 :PGP邮件加密软件的使用
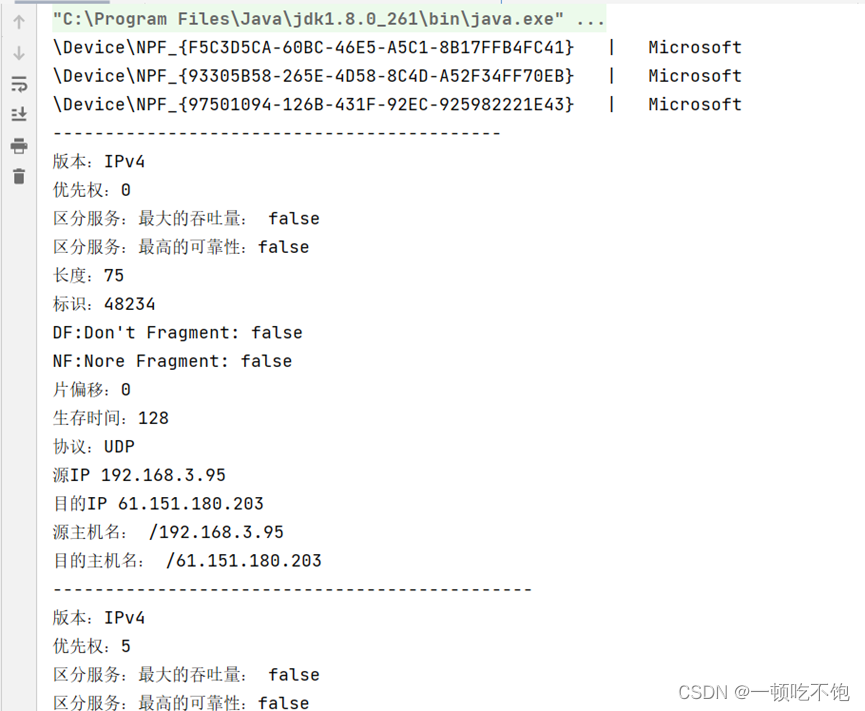
Information Security Experiment 4: implementation of IP packet monitoring program
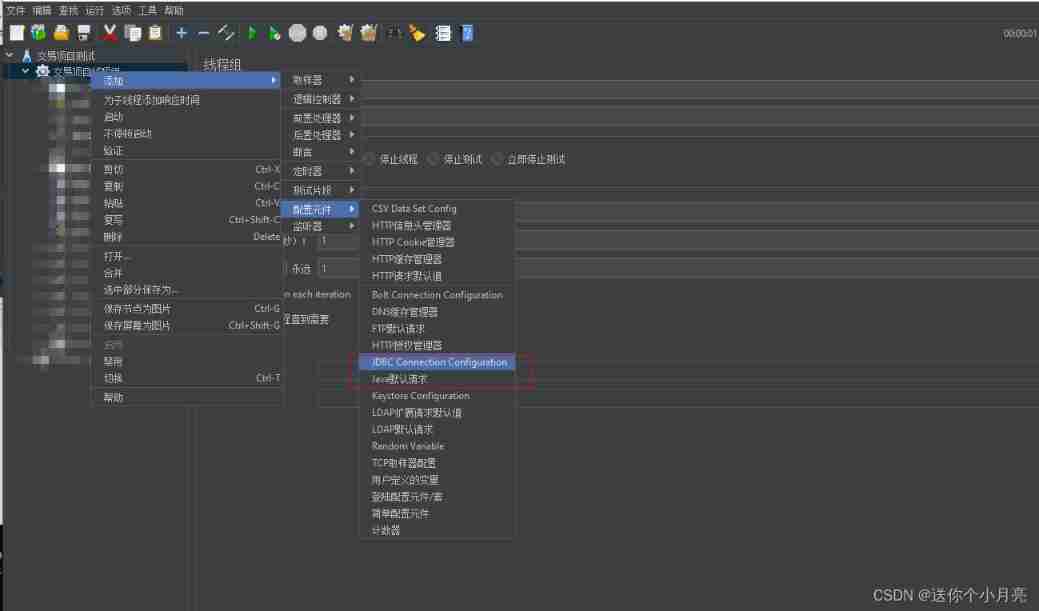
JMeter JDBC batch references data as input parameters (the simplest method for the whole website)
随机推荐
Information Security Experiment 3: the use of PGP email encryption software
Skill review of test engineer before interview
C language pointer (exercises)
5A summary: seven stages of PMP learning
Netease Cloud Wechat applet
E-commerce campaign Guide
Redis common commands
Add new item after the outbound delivery order of SAP mm sto document is created?
What are the conditions for applying for NPDP?
信息安全实验二 :使用X-SCANNER扫描工具
Install pyqt5 and Matplotlib module
正则匹配以XXX开头的,XXX结束的
[chaosblade: node CPU load, node network delay, node network packet loss, node domain name access exception]
Jemter operation
Some pit avoidance guidelines for using Huawei ECS
Locust performance test 5 (analysis)
How to speed up video playback in browser
四、机器学习基础
Huawei hcip datacom core_ 03day
PMP Exam Preparation experience systematically improve project management knowledge through learning