当前位置:网站首页>Special topic of rotor position estimation of permanent magnet synchronous motor -- Summary of position estimation of fundamental wave model
Special topic of rotor position estimation of permanent magnet synchronous motor -- Summary of position estimation of fundamental wave model
2022-07-06 20:07:00 【Explain the motor in simple terms】
introduction
This paper classifies the methods of estimating the rotor position through the fundamental wave model of permanent magnet synchronous motor , Summarize the angle estimated by back EMF 、 Estimate the angle through the rotor flux 、 Closed loop scheme , Three different ideas , Break it down one by one , Its core principles are described respectively , It also outlines the different ideas of different schemes , Give relevant papers for reference .
List of articles
1、 Back EMF method
The last article deduced α β \alpha \beta αβ Voltage equation in coordinate system
[ v α v β ] = [ i α i β ] R s + d d t [ L s i α L s i β ] + [ − ω e ϕ f s i n θ ω e ϕ f c o s θ ] (1) \left[\begin{array}{c} v_{\alpha }\\ v_{\beta } \end{array}\right] = \left[\begin{array}{c} i_{\alpha }\\ i_{\beta } \end{array}\right] R_s + \frac{d}{dt} \left[\begin{array}{c}L_s i_{\alpha }\\L_s i_{\beta } \end{array}\right] + \left[\begin{array}{c}- \omega_e \phi_{f } sin\theta \\ \omega_e\phi_{f}cos\theta \end{array}\right] \tag{1} [vαvβ]=[iαiβ]Rs+dtd[LsiαLsiβ]+[−ωeϕfsinθωeϕfcosθ](1)
among ω e \omega_e ωe Is the electrical angular velocity .
Easy to see , α β \alpha \beta αβ The voltage consists of three parts , Resistance voltage drop , The voltage produced by the change of inductive current , The back electromotive force produced by the change of the flux linkage of the equivalent permanent magnet . As mentioned above , The rotor position of permanent magnet synchronous motor can be extracted from the back EMF . The key point of various position estimation methods of back EMF is to separate the back EMF term from the voltage equation . We are running senseless foc when , Motor stator resistance , Motor stator inductance , The flux linkage constant of the permanent magnet is a known constant , Voltage and current are measurable parameters , speed 、 The rotor position is the parameter to be estimated . For the three components of the voltage equation , The resistance voltage drop part is easy to calculate , The inductance voltage cannot be accurately calculated because it involves differentiation , Although the voltage is known , However, the direct calculation error of inductance voltage is large , It is difficult to directly separate the components of back EMF .
For this kind of content that is difficult to calculate directly , It is often estimated by designing observers .
d d t [ i α i β ] = [ v α v β ] 1 L s − [ i α i β ] R s L s + [ − ω e ϕ f s i n θ ω e ϕ f c o s θ ] 1 L s (2) \frac{d}{dt} \left[\begin{array}{c} i_{\alpha }\\ i_{\beta } \end{array}\right] = \left[\begin{array}{c} v_{\alpha }\\ v_{\beta } \end{array}\right] \frac{1}{L_s} - \left[\begin{array}{c} i_{\alpha }\\ i_{\beta } \end{array}\right] \frac{R_s}{L_s} + \left[\begin{array}{c}- \omega_e \phi_{f } sin\theta \\ \omega_e\phi_{f}cos\theta \end{array}\right]\frac{1}{L_s} \tag{2} dtd[iαiβ]=[vαvβ]Ls1−[iαiβ]LsRs+[−ωeϕfsinθωeϕfcosθ]Ls1(2)
The written voltage equation is in the above form , For the rightmost back EMF term , Not easy to calculate , As an unknown part , Current equals formula 2 The integral on the right . There may be an error between the current obtained by integration and the real current sampled , use e r r α , e r r β err_{\alpha} ,err_{\beta} errα,errβ Express .
[ i ^ α i ^ β ] = [ v α v β ] 1 L s − [ i ^ α i ^ β ] R s L s + [ x α + e r r α x β + e r r β ] 1 L s (3) \left[\begin{array}{c}\hat i_{\alpha }\\ \hat i_{\beta } \end{array}\right] = \left[\begin{array}{c} v_{\alpha }\\ v_{\beta } \end{array}\right] \frac{1}{L_s} - \left[\begin{array}{c} \hat i_{\alpha }\\ \hat i_{\beta } \end{array}\right] \frac{R_s}{L_s} + \left[\begin{array}{c}x_{\alpha} + err_{\alpha}\\ x_{\beta} +err_{\beta}\end{array}\right]\frac{1}{L_s} \tag{3} [i^αi^β]=[vαvβ]Ls1−[i^αi^β]LsRs+[xα+errαxβ+errβ]Ls1(3)
among
e r r α = i α − i ^ α ; e r r β = i β − i ^ β err_{\alpha} = i_{\alpha} - \hat i_{\alpha};err_{\beta}= i_{\beta} - \hat i_{\beta} errα=iα−i^α;errβ=iβ−i^β
Use some form of correction , Such as synovium , send e r r α = 0 , e r r β = 0 err_{\alpha} = 0,err_{\beta} = 0 errα=0,errβ=0, be
[ x α x β ] = [ − ω e ϕ f s i n θ ω e ϕ f c o s θ ] \left[\begin{array}{c}x_{\alpha}\\ x_{\beta} \end{array}\right] = \left[\begin{array}{c}- \omega_e \phi_{f } sin\theta \\ \omega_e\phi_{f}cos\theta \end{array}\right] [xαxβ]=[−ωeϕfsinθωeϕfcosθ]
Here we are , Complete the separation of the back EMF term , adopt arctan Or phase locked loop calculation can obtain the rotor position , For speed , It can be obtained by phase-locked loop , It can also be obtained by positional differentiation , in addition , x α 2 + x β 2 / ϕ f \sqrt{ x_{\alpha}^2 + \\ x_{\beta}^2}/ \phi_f xα2+xβ2/ϕf You can also get the rotor speed .
Related articles :
Kim H, Son J, Lee J. A high-speed sliding-mode observer for the sensorless speed control of a PMSM[J]. IEEE Transactions on Industrial Electronics, 2010, 58(9): 4069-4077.
observation i α , i β i_{\alpha},i_{\beta} iα,iβ, Use the traditional synovial observer Signum Function Do the switch function , This article USES the sigmoid function Do the switch function , Low pass filtering can be avoided .
Qiao Z, Shi T, Wang Y, et al. New sliding-mode observer for position sensorless control of permanent-magnet synchronous motor[J]. IEEE Transactions on Industrial electronics, 2012, 60(2): 710-719.
This article is optimized on the basis of the previous article , A speed observer is designed , Instead of calculating speed by differentiating angles .
2、 Flux linkage method
The last article mentioned α β \alpha \beta αβ The following expression of the voltage equation in the coordinate system .
[ v α v β ] = [ i α i β ] R s + d d t [ ϕ α ϕ β ] (4) \left[\begin{array}{c} v_{\alpha }\\ v_{\beta } \end{array}\right] = \left[\begin{array}{c} i_{\alpha }\\ i_{\beta } \end{array}\right] R_s + \frac{d}{dt} \left[\begin{array}{c} \phi_{\alpha }\\ \phi_{\beta } \end{array}\right] \tag{4} [vαvβ]=[iαiβ]Rs+dtd[ϕαϕβ](4)
among ϕ α ϕ β \phi_{\alpha} \phi_{\beta} ϕαϕβ Express α β \alpha \beta αβ Axial flux linkage
[ ϕ α ϕ β ] = ∫ ( [ v α v β ] − [ i α i β ] R s ) d t (5) \left[\begin{array}{c} \phi_{\alpha }\\ \phi_{\beta } \end{array}\right] = \int \left( {\left[\begin{array}{c} v_{\alpha }\\ v_{\beta } \end{array}\right] - \left[\begin{array}{c} i_{\alpha }\\ i_{\beta } \end{array}\right] R_s }\right) dt \tag{5} [ϕαϕβ]=∫([vαvβ]−[iαiβ]Rs)dt(5)
Finishing type 4, Get the formula 5, The flux linkage can be written in addition to the resistance voltage drop , Integration of other voltages . Imagine a coil , Placed in a magnetic field , When the magnetic field changes , Voltage will be generated at both ends of the first coil . Easy to understand formula 4, Understand the formula 4, It is easy to deduce the formula 5, type 5 Calculate the flux linkage through the integration of voltage , This formula can be called the voltage model of permanent magnet synchronous motor flux linkage model ..
Above α β \alpha \beta αβ The flux linkage is the total flux linkage on two axes , The flux linkage of each shaft can be written as the combination of rotor part flux linkage and stator part flux linkage . stay d q dq dq Coordinate system , d d d Shaft is defined as rotor permanent magnet N The direction of the pole , So the rotor permanent magnet flux linkage ϕ f \phi_f ϕf All in d d d Axis , meanwhile d q dq dq The shaft also has stator flux .
As mentioned earlier, the change of magnetic field will generate voltage at both ends of the coil , The stator inductance of permanent magnet synchronous motor is such a coil .
from ϕ = ∫ v d t \phi = \int{v}dt ϕ=∫vdt; And L d i d t = v L\frac{di}{dt} = v Ldtdi=v; Available ϕ = L i \phi = Li ϕ=Li; That is, the magnetic chain generated by the current flowing through the coil is the product of inductance and current , if d q dq dq The shaft current is not 0, d q dq dq The shaft flux linkage will contain stator flux linkage components , adopt ipark transformation α β \alpha \beta αβ Axial flux linkage , As follows .
[ ϕ α ϕ β ] = [ c o s θ s i n θ − s i n θ c o s θ ] [ L s i d + ϕ f L s i q ] (6) \left[\begin{array}{c} \phi_{\alpha }\\ \phi_{\beta } \end{array}\right] = \left[\begin{array}{c} cos\theta&sin\theta\\ -sin\theta & cos\theta \end{array}\right] \left[\begin{array}{c} L_s i_{d } + \phi_f\\ L_s i_{q } \end{array}\right] \tag{6} [ϕαϕβ]=[cosθ−sinθsinθcosθ][Lsid+ϕfLsiq](6)
This formula is calculated by the current flowing through the inductance α β \alpha \beta αβ Axial flux linkage , It can be called the current model of permanent magnet synchronous motor flux model .
Because the rotor position information is contained in the rotor flux linkage component , And this part is easy to pass α β \alpha \beta αβ Separation of flux linkage equations in coordinate system , The position estimation methods of flux linkage are mostly α β \alpha \beta αβ Calculate the total flux linkage in the coordinate system , Then subtract the stator flux , Get the rotor flux , Then the rotor position and speed are obtained by using phase-locked loop , Or obtain the rotor position by arctangent , Then the differential gives the velocity .
It is worth mentioning that the rotor flux linkage has nothing to do with the motor speed , Using the flux linkage method to estimate the rotor position of the motor is easy to obtain better low-speed performance .
To accurately separate the rotor flux components is a core problem of the flux method , The common way is to calculate the total flux linkage through the voltage and current model , Then take a reference value that is not easy to change to correct the error . Or the famous reference model adaptive method , Take the current model as a reference , Voltage model adaptation ; The two models can also be fused directly , Trust the current model at low speed , Trust the voltage model at high speed .
The way of magnetic linkage is 2010 The more popular method after years , There are many points worth discussing in detail , Relevant content will be analyzed in a subsequent meeting .
Related articles :
Lee J, Hong J, Nam K, et al. Sensorless control of surface-mount permanent-magnet synchronous motors based on a nonlinear observer[J]. IEEE Transactions on power electronics, 2009, 25(2): 290-297.
Famous nonlinear observer , Use the rotor flux to correct the voltage model .
An L, Franck D, Hameyer K. Sensorless control for surface mounted permanent magnet synchronous machines at low speed[C]//2013 International Conference on Electrical Machines and Systems (ICEMS). IEEE, 2013: 77-82.
Use current model to correct voltage model , Or use the sampling current to correct the voltage model .
3、 Closed loop method
First look at d q dq dq Coordinate system voltage equation
u d = R s i d + L d d i d d t − ω e L q i q u q = R s i q + L q d i q d t + ω e L d i d + ω e ϕ f (7) u_d = R_s i_d + L_d \frac{di_d}{dt}- \omega_eL_qi_q\\ u_q = R_s i_q + L_q \frac{di_q}{dt} + \omega_e L_d i_d + \omega_e \phi_f \tag{7} ud=Rsid+Lddtdid−ωeLqiquq=Rsiq+Lqdtdiq+ωeLdid+ωeϕf(7)
d q dq dq The idea of position estimation in coordinate system is essentially different from that mentioned above , The two methods mentioned above belong to open-loop schemes , The input of the position observer is i α , i β ; u α , u β i_{\alpha},i_{\beta};u_{\alpha},u_{\beta} iα,iβ;uα,uβ, The output of the observer is angle and speed . The output of the observer will not affect the input in turn .
however d q dq dq The scheme under the coordinate system is different , This kind of scheme requires that the input is calculated by using the angle of the observer output i d , i q ; u d , u q i_d,i_q;u_d,u_q id,iq;ud,uq, And then use i d , i q i_d,i_ q id,iq Calculated by voltage equation u ^ d , u ^ q \hat u_d,\hat u_q u^d,u^q, Or use u d , u q u_d,u_ q ud,uq Calculated by voltage equation i ^ d , i ^ q \hat i_d,\hat i_q i^d,i^q, The estimated value is compared with the sampled value , If the angle is more accurate , The error is relatively small , Or on the contrary, if the estimation error tends to 0, Then the output angle of the observer converges near the real angle .
To look back d q dq dq Voltage equation in coordinate system , Back EMF component at rated speed ω e ϕ f \omega_e\phi_f ωeϕf Generally, it can occupy q q q Shaft voltage 70% above , If the angle is accurate , The back EMF components will all lead out at q q q Axis , If there is an error in the angle , d d d The shaft will have a back EMF component , Calculated using the voltage equation d d d The shaft voltage will be significantly less than park Transformed d d d Shaft voltage . Closed loop methods often take similar errors as inputs , Design regulator , The output of the regulator is speed or angle , Adjustment makes the error approach 0, The angle is the exact angle .
There are also many places worth discussing in this kind of scheme , The following articles will be analyzed in detail .
Related articles :
Lascu C, Andreescu G D. PLL position and speed observer with integrated current observer for sensorless PMSM drives[J]. IEEE Transactions on Industrial Electronics, 2020, 67(7): 5990-5999.
2020 New paper in , Use a current observer to estimate d q dq dq electric current , The angle and speed errors are reflected by current errors .
Harnefors L, Ottersten R. Regenerating-Mode Stabilization of the “Statically Compensated Voltage Model”[J]. IEEE Transactions on Industrial Electronics, 2007, 54(2): 818-824.
Good low-speed performance is achieved
4、 Summary
It says 3 The specific principle of class method , According to my understanding and observation of the industry , Back EMF method was the mainstream method in the early years , So far, the scheme of high-speed motor control is also used , This kind of method is difficult to achieve good low-speed performance . The method of magnetic linkage is the mainstream in recent years , Many common platforms such as ti fast The scheme uses the magnetic linkage method , This kind of method is easy to achieve better low-speed performance . The closed-loop method is a new scheme , It can also achieve better low-speed performance , Online transmission Huichuan has turned to this kind of scheme , According to my judgment of industry trends , This kind of method will be applied more in the future .
边栏推荐
- AsyncHandler
- JS get browser system language
- RT-Thread 组件 FinSH 使用时遇到的问题
- rt-thread i2c 使用教程
- Finally, there is no need to change a line of code! Shardingsphere native driver comes out
- 深入浅出,面试突击版
- 121. The best time to buy and sell stocks
- VMware virtual machine cannot open the kernel device "\.\global\vmx86"
- Guangzhou's first data security summit will open in Baiyun District
- Node.js: express + MySQL实现注册登录,身份认证
猜你喜欢
Tencent T2 Daniel explained in person and doubled his job hopping salary
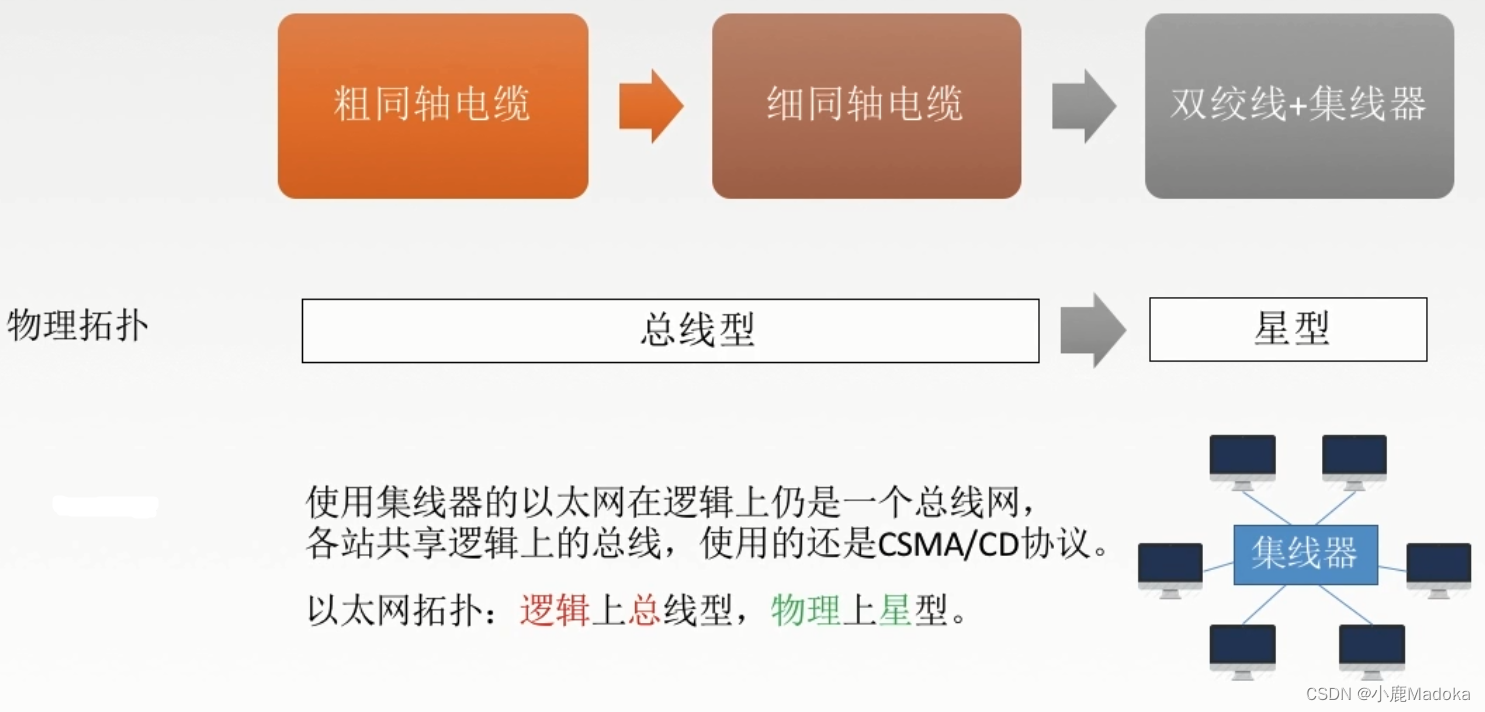
【计网】第三章 数据链路层(4)局域网、以太网、无线局域网、VLAN
爬虫(14) - Scrapy-Redis分布式爬虫(1) | 详解
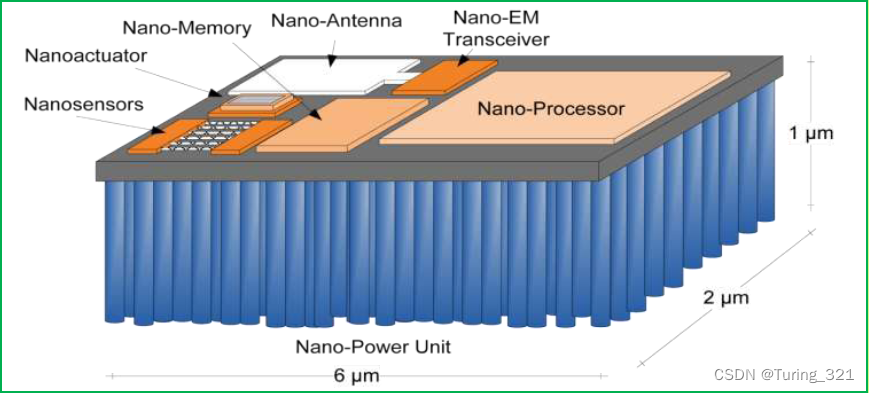
5. 無線體內納米網:十大“可行嗎?”問題

5. 无线体内纳米网:十大“可行吗?”问题
腾讯T3大牛手把手教你,大厂内部资料
Vscode debug run fluent message: there is no extension for debugging yaml. Should we find yaml extensions in the market?
HMS Core 机器学习服务打造同传翻译新“声”态,AI让国际交流更顺畅

数字三角形模型 AcWing 1018. 最低通行费

Enumeration gets values based on parameters
随机推荐
Crawler (14) - scrape redis distributed crawler (1) | detailed explanation
POJ3617 Best Cow Line 馋
Introduction to enterprise lean management system
Linear distance between two points of cesium
句号压缩过滤器
5. Nano - Net in wireless body: Top 10 "is it possible?" Questions
Technology sharing | packet capturing analysis TCP protocol
范式的数据库具体解释
rt-thread i2c 使用教程
[Yann Lecun likes the red stone neural network made by minecraft]
深度学习分类网络 -- ZFNet
PHP and excel phpexcel
Is it difficult for small and micro enterprises to make accounts? Smart accounting gadget quick to use
beegfs高可用模式探讨
String长度限制?
系统与应用监控的思路和方法
How to handle the timeout of golang
Ideas and methods of system and application monitoring
SSH connection denied
数字三角形模型 AcWing 1018. 最低通行费