当前位置:网站首页>Array cyclic shift problem
Array cyclic shift problem
2022-07-05 12:21:00 【A finger leaf next to the giant】
About array cyclic shift , stay Draw lessons from articles I learned three solutions in .
First, the first circular transposition algorithm , Not much .
Let's start with the second algorithm
Triple inversion algorithm :
The triple inversion algorithm completes the cyclic shift through triple inversion , For example, array [1,2,3,4,5], Then move three steps to the left , This can be done through the following functions :
reverse(0,2) ->[3,2,1,4,5]
reverse(2,4) ->[3,2,1,5,4]
reverse(0,4) ->[4,5,1,2,3]
If the number of steps is recorded as p, Array has n Number , Then there are :
reverse(0,p-1)
reverse(p,n-1)
reverse(0,n-1)
Indeed, before we look at the shift , The result after shift , You will find that the number of steps divides the array into two parts , Then the position of the two parts changed , The principle of position exchange achieved after three reversals can be understood in conjunction with the palm turning Algorithm :
Three reverses –> Palm turning algorithm :
At first, the palm was on our face , The left hand is on the right , First turn your left hand upside down ( Palm outward , Palm back to our face ), Turn your right hand over ( Same as left hand ), Finally, turn both hands at the same time , At this time, you will find , The palms of both hands are still facing us , But the stacking order of hands has changed .
// Using three inversions to realize cyclic shift
public class CircleGo_1 {
public static void reverse(int nums[],int a, int b) {
int middle;
// Note the reversal , It is the inversion from the first to the last number of the interval , The middle number does not change
for(int i=0;i<(b-a+1)/2;i++) {
middle=nums[a+i];
nums[a+i]=nums[b-i];
nums[b-i]=middle;
}
}
public static void main(String[] args) {
int nums[]= {
1,2,3,4,5};
int p =3,n=nums.length;
reverse( nums, 0,p-1);
reverse(nums, p,n-1);
reverse(nums,0,n-1);
System.out.println(Arrays.toString(nums));
}
}
Permutation loop algorithm :
In ordinary circular transposition algorithm , Need to move a few steps , It needs to be cycled several times , Move one step at a time , Such spatial complexity is O(1), The time complexity is O(nk). If k Less than n, be O(n^2).
that , Is it possible to directly move the number to be moved to the position after the cycle , such , Each number only needs to be moved once to complete the cyclic shift , So if possible , How to determine the specific location to move .
For example, the sequence subscript is [i] Number of numbers , Its cyclic shift k Step , Then it should be in [i+k] The location of ,[i+k] The number of positions should be [i+2k] The location of , Because each element moves to the right n After that, he returned to his original position , So move right k Bit equals shift right k mod n position . So there is such a sequence of positions :0,k,2k,…,(t-1)k. Each of them is in the mold n Position in the sense of .tk mod n The result is 0.t Is making tk mod n As the result of the 0 The smallest positive integer of . And t Is inevitable , The biggest is in t be equal to n When , In this way, a ring is formed .
To be exact, it forms a cyclic group .
This sequence of positions is essentially a module n The additive group consists of elements k Generate a cyclic subgroup . From the conclusion of group theory ( The proof of this conclusion is shown at the end ) know , Cyclic subgroup (k) The period of is n / gcd(k,n), The number of elements is n / gcd(k,n), The number of cosets is gcd(k,n). let me put it another way ,A[0…n-1] Cycle moves to the right k The result of bit is cyclic subgroup (k) And all its coset cycles are shifted one bit to the right . for example , take A[0…5] = {1,2,3,4,5,6} Cycle moves to the right 4 position , here n = 6, k = 4, gcd(k, n) = 2.A[0] The final position is 4,A[4] The final position is 2,A[2] The final position is 0, such , Location 0,4,2 It is from k=4 Generated cyclic group , The period is 6 / gcd(4,6) = 6 / 2 = 3, Such cyclic subgroups share gcd(4,6) = 2 individual .
So the complete code has , Make sure the gcd(k,n) Number of cyclic subgroups , Then shift the elements in the cyclic group one by one .
// Cyclic shift of array
#include <cstdio>
//gcd(x,y) Express x and y Maximum common factor of -- Euclidean algorithm
int gcd(int m, int n) {
int r;
//r Record the remainder , Divide the divisor by the remainder , Until the remainder is 0, At this point, the divisor is the greatest common factor
while (r = m % n) {
m = n;
n = r;
}
return n;
}
void shiftArray(int A[], int n, int k) {
// Because the code shifted to the left is much easier to implement than the code shifted to the right , And move right k position
// Equivalent to moving left -k position , Generally speaking, shift right k position , Equal to shift left n-k position . The following code is moved left n-k Bit to shift right k position
k = n - (k % n);
for (int i = 0, cnt = gcd(n, k); i < cnt; i++) {
int t = A[i], p = i, j = (k + i) % n;
while (j != i) {
A[p] = A[j]; p = j; j = (k + p) % n;
}
A[p] = t;
}
}
void printArray(int A[], int n) {
for (int i = 0; i < n; i++) {
printf("%-3d", A[i]);
}
}
int main() {
int A[] = {
1,2,3,4,5,6,7,8,9,10,11,12,13,14,15 };
printf(" Cycle to the right 12 Step \n");
shiftArray(A, 15, 12);
printArray(A, 15);
return 0;
}
边栏推荐
- Halcon template matching actual code (I)
- PXE startup configuration and principle
- Solve the error 1045 of Navicat creating local connection -access denied for user [email protected] (using password
- Redis highly available sentinel mechanism
- Course design of compilation principle --- formula calculator (a simple calculator with interface developed based on QT)
- 自动化测试生命周期
- Master-slave mode of redis cluster
- byte2String、string2Byte
- Understand redis persistence mechanism in one article
- Matlab label2idx function (convert the label matrix into a cell array with linear index)
猜你喜欢
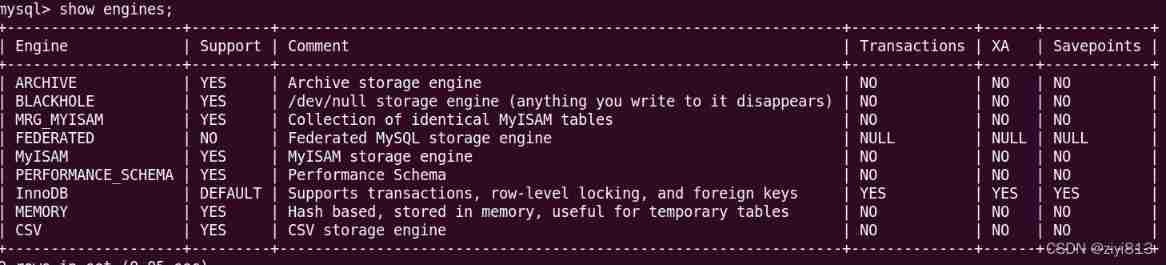
MySQL storage engine
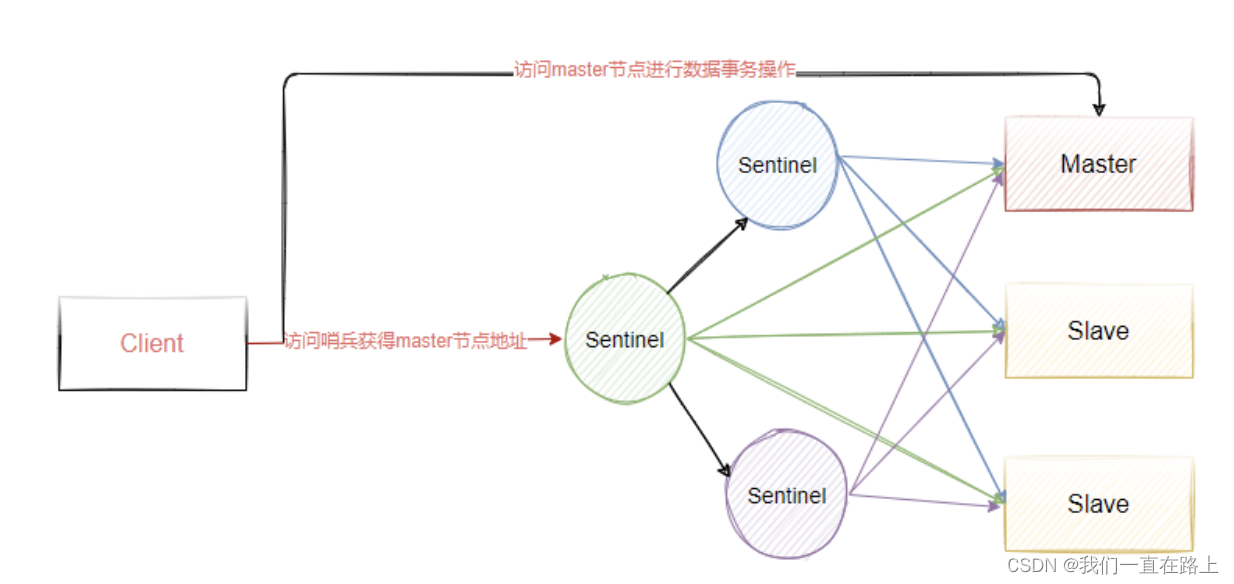
Sentinel sentinel mechanism of master automatic election in redis master-slave
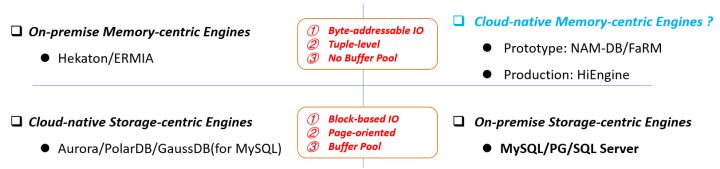
HiEngine:可媲美本地的云原生内存数据库引擎

Thoughts and suggestions on the construction of intelligent management and control system platform for safe production in petrochemical enterprises
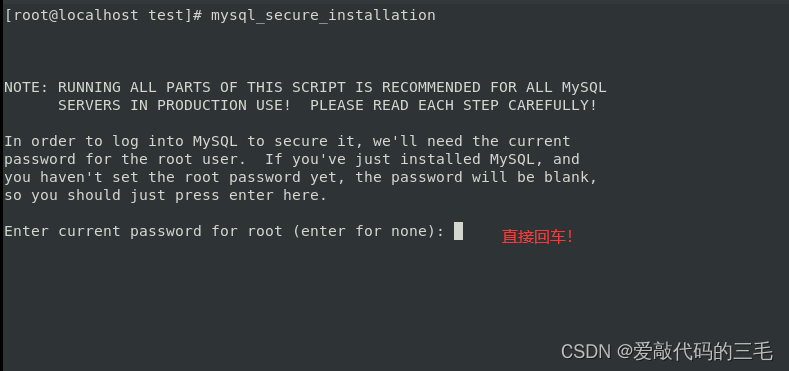
Linux Installation and deployment lamp (apache+mysql+php)
Select drop-down box realizes three-level linkage of provinces and cities in China

MySQL splits strings for conditional queries
查看rancher中debug端口信息,并做IDEA Remote Jvm Debug
Multi table operation - Auto Association query

The evolution of mobile cross platform technology
随机推荐
Error modulenotfounderror: no module named 'cv2 aruco‘
Read and understand the rendering mechanism and principle of flutter's three trees
mysql拆分字符串做条件查询
II. Data type
How does MySQL execute an SQL statement?
Learn the memory management of JVM 02 - memory allocation of JVM
[cloud native | kubernetes] actual battle of ingress case (13)
Learn JVM garbage collection 02 - a brief introduction to the reference and recycling method area
GPS数据格式转换[通俗易懂]
Halcon template matching actual code (I)
Codeforces Round #804 (Div. 2)
POJ-2499 Binary Tree
Take you hand in hand to develop a service monitoring component
mmclassification 训练自定义数据
SENT协议译码的深入探讨
一类恒等式的应用(范德蒙德卷积与超几何函数)
Linux Installation and deployment lamp (apache+mysql+php)
Course design of compilation principle --- formula calculator (a simple calculator with interface developed based on QT)
1. Laravel creation project of PHP
JS for循环 循环次数异常