leetcode算法(1)

想让APP和微信一样，可以流畅运行小程序？ | 体验即送大疆、华为、樱桃键盘！>>>

这是leetcode最简单的题了吧!!!

本次博主给大家推荐几个究极简单的算法, 简单归简单,但无论难易与否,万变不离其宗. 就由博主带大家从易到难,慢慢更新,积累. 如有更好的算法,还望大家分享.

---

---

题目需求1:

小A 和 小B 在玩猜数字。小B 每次从 1, 2, 3 中随机选择一个，小A 每次也从 1, 2, 3 中选择一个猜。他们一共进行三次这个游戏，请返回 小A 猜对了几次？

输入的guess数组为 小A 每次的猜测，answer数组为 小B 每次的选择。guess和answer的长度都等于3。

代码实现

public class Solution {
   
      
    public int game(int[] guess, int[] answer) {
   
      
        int count = 0;
        for (int i = 0; i < 3; i++) {
   
      
            if (guess[i] == answer[i]){
   
      
                count+=1;
            }
        }
        return count;
    }

    public static void main(String[] args) {
   
      
        Solution n2 = new Solution();
        System.out.println(n2.game(new int[]{
   
      1,2,3}, new int[]{
   
      1, 2, 2}));
    }
}

题目需求2:

求 1+2+...+n ，要求不能使用乘除法、for、while、if、else、switch、case等关键字及条件判断语句（A?B:C）。

代码实现

public class Solution3 {
   
      
    public int sumNums(int n) {
   
      
        //等差数列求和
        return (1+n)*n/2;
    }

    public static void main(String[] args) {
   
      
        Solution3 q = new Solution3();
        System.out.println(q.sumNums(3));
    }
}

题目需求3:

给你一个正方形矩阵 mat，请你返回矩阵对角线元素的和。

请你返回在矩阵主对角线上的元素和副对角线上且不在主对角线上元素的和。

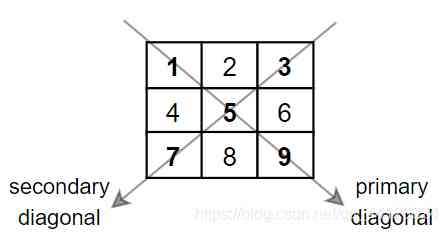
输出:1 + 5 + 9 + 3 + 7 = 25
注意,元素 mat[1][1] = 5 只会被计算一次。

代码实现

public class Solution4 {
   
      

    int diagonal= 0;
    public int diagonalSum(int[][] mat) {
   
      
        for (int i=0;i<mat.length;i++){
   
      
            for (int j = 0; j < mat[i].length; j++) {
   
      
                if ( i==j || i+j == mat.length-1){
   
      
                    diagonal += mat[i][j];
                }
            }
        }
        return diagonal;
    }

    public static void main(String[] args) {
   
      
        Solution4 s = new Solution4();
        System.out.println(s.diagonalSum(new int[][]{
   
      {
   
      1,2,3},{
   
      4,5,6},{
   
      7,8,9}}));
    }
}

题目需求4:

桌上有 n 堆力扣币，每堆的数量保存在数组 coins 中。我们每次可以选择任意一堆，拿走其中的一枚或者两枚，求拿完所有力扣币的最少次数。

简化前代码实现

public class Solution5 {
   
      

    public int minCount(int[] coins) {
   
      

        int count1 = 0;
        int count2 = 0;

        for (int i = 0; i < coins.length; i++) {
   
      

            if (coins[i] % 2 ==0){
   
      
                count1 += coins[i]/2;
            }
            if (coins[i] % 2 !=0){
   
      
                count2 += coins[i]/2+1;
            }
        }
        return count1+count2;
    }

    public static void main(String[] args) {
   
      
        Solution5 s = new Solution5();
        System.out.println(s.minCount(new int[]{
   
      1,1,1}));
    }
}

简化后代码实现

public class Solution5 {
   
      

   public int minCount1(int[] coins) {
   
      
        int c = 0;
        for (int i = 0; i < coins.length; i++) {
   
      
            c += coins[i] / 2;
            c += coins[i] % 2;
        }
        return c;
    }
    
     public static void main(String[] args) {
   
      
        Solution5 s = new Solution5();
        System.out.println(s.minCount1(new int[]{
   
      2,5,7}));
    }