当前位置:网站首页>Commonly used probability distributions: Bernoulli distribution, binomial distribution, polynomial distribution, Gaussian distribution, exponential distribution, Laplace distribution and Dirac delta d
Commonly used probability distributions: Bernoulli distribution, binomial distribution, polynomial distribution, Gaussian distribution, exponential distribution, Laplace distribution and Dirac delta d
2022-07-05 22:56:00 【YaoHa_】
Bernoulli distribution (Bernoulli distribution)
** Bernoulli distribution :** Distribution of single binary random variable . By a single parameter φ∈[0,1] control .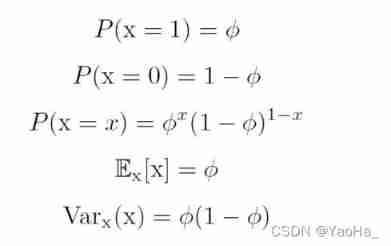
example : Flip a coin , The probability of facing up .
Binomial distribution (binomial distrubution)
Binomial distribution : stay n Events in this test A It happens k The probability of time .
Probability calculation :
In style k=0,1,2,…,n,
Expect to calculate :E(x)=np
Variance calculation :D(x)=np(1-p)
example : throw n The second coin , Face up m The probability of time .
Polynomial distribution (multinoulli distribution)
Polynomial distribution : have k Distribution on a single discrete random variable in different states , among k It's a finite value . Expansion of binomial distribution .
Probability calculation :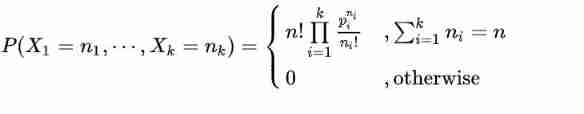
example : Multiple screen throwing , Count the probability of the number of times each side is thrown .
Gaussian distribution (Gaussian dis-tribution)
Gaussian distribution (Gaussian dis-tribution), Also known as normal distribution (normal distribution). namely :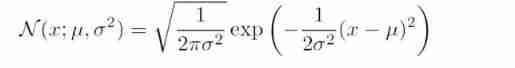
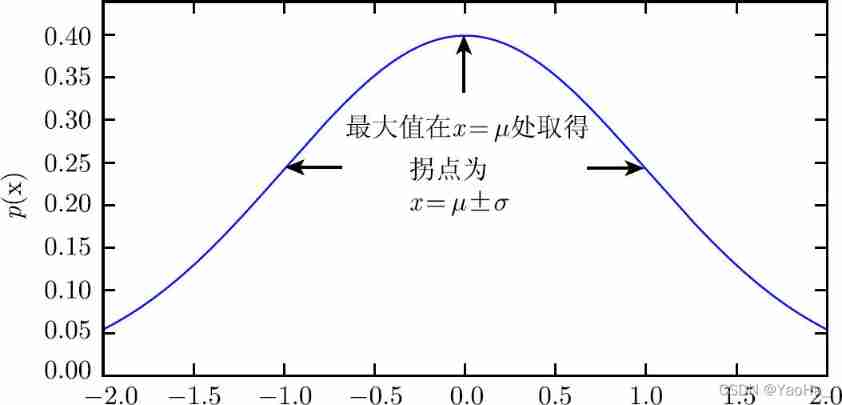
notes : The normal distribution presents a classic “ A bell curve ” The shape of the , Of which the central peak x Coordinates from µ give , The width of the peak is affected by σ control . In this example , What we're showing is Standard normal distribution (standard normaldistribution), among µ=0,σ=1.
Expect to calculate :E(x)=µ
Variance calculation :D(x)=σ^2
An index distribution (exponential distribution)
An index distribution (exponential distribution): stay x=0 Get the boundary point (sharp point) The distribution of . namely :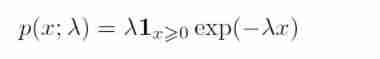
Indicator function (indicator function):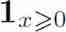
The exponential distribution is made proper by the indicator function x The probability of taking a negative value is zero .
Laplacian distribution (Laplace distribution)
Laplacian distribution (Laplace distribution): Probability density function distribution of random variables . namely :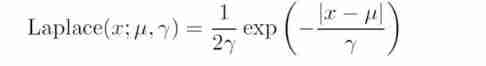
Dirac Distribution
Dirac delta function (Dirac delta function): Defined as in addition to 0 Values for all points except 0, But the integral is 1.
Dirac Distribution is often used as an empirical distribution (empirical distribution) A component of :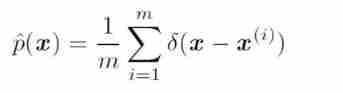
边栏推荐
- Roman numeral to integer
- How to create a thread
- All expansion and collapse of a-tree
- Element operation and element waiting in Web Automation
- 【无标题】
- The introduction to go language is very simple: String
- Nangou Gili hard Kai font TTF Download with installation tutorial
- 一文搞定垃圾回收器
- Ultrasonic sensor flash | LEGO eV3 Teaching
- Distributed solution selection
猜你喜欢
Common model making instructions
Paddy serving v0.9.0 heavy release multi machine multi card distributed reasoning framework
![[error record] file search strategy in groovy project (src/main/groovy/script.groovy needs to be used in the main function | groovy script directly uses the relative path of code)](/img/b6/b2036444255b7cd42b34eaed74c5ed.jpg)
[error record] file search strategy in groovy project (src/main/groovy/script.groovy needs to be used in the main function | groovy script directly uses the relative path of code)

Tensor attribute statistics
TCC of distributed solutions

Nanjing: full use of electronic contracts for commercial housing sales
Arduino 测量交流电流
基于STM32的ADC采样序列频谱分析
查看网页最后修改时间方法以及原理简介
Finally understand what dynamic planning is
随机推荐
使用rewrite规则实现将所有到a域名的访问rewrite到b域名
The difference between MVVM and MVC
Distance entre les points et les lignes
Overview of Fourier analysis
Thinkphp5.1 cross domain problem solving
Masked Autoencoders Are Scalable Vision Learners (MAE)
Usage Summary of scriptable object in unity
我把开源项目alinesno-cloud-service关闭了
Nacos installation and service registration
南京:全面启用商品房买卖电子合同
二叉树(二)——堆的代码实现
Metasploit (MSF) uses MS17_ 010 (eternal blue) encoding:: undefined conversionerror problem
How to create a thread
【Note17】PECI(Platform Environment Control Interface)
3 find the greatest common divisor and the least common multiple
Global and Chinese market of water treatment technology 2022-2028: Research Report on technology, participants, trends, market size and share
Binary tree (II) -- code implementation of heap
请求二进制数据和base64格式数据的预览显示
How can easycvr cluster deployment solve the massive video access and concurrency requirements in the project?
TypeError: this. getOptions is not a function