Detailed introduction to drawing complex surfaces using the plot_surface command

一、引言
The 3 d geometry in,Often need to draw the complex curved surface,For example, the closed space curved surface.Based on the drawing by planez=1,旋转抛物面z=x^2 + y^2And parabolicy=2xIn the closed surface, for example,In detail usingPython命令plot_surfaceThe process of drawing complex curved surface.
二、Draw a closed surface
1、First draw rotating parabolicz=x^2 + y^2
Consider rotating parabolic function domain is a circle domain,So using polar coordinates to indicate the graphic abscissa and ordinate,Let get some graphics look better on the vision.
具体的步骤为：
1）First of all make graphics window,And with the provisions of the three-dimensional coordinate system
2）Determine the extremely diameter and Angle of the grid coordinates
3）According to the relationship of rectangular coordinates and polar coordinates gets the abscissa of rotating paraboloid、Axis and the vertical coordinates of
4）利用plot_surfaceFunction mapping rotating parabolic
5）Increase each coordinate axis labels,Determine the Angle of 3 d graphics
参考代码如下：

import matplotlib.pyplot as plt
import numpy as np
import math

fig = plt.figure()             #建立图形窗口
ax = fig.gca( projection = '3d' )  #Using 3 d coordinate
theta = np.arange( 0, 2 * math.pi, 0.05 )
rou  = np.arange( 0, 1, 0.005 )
T, R = np.meshgrid( theta, rou ) #Polar Angle and diameter of the grid coordinates
Xp = R * np.cos(T)   #Abscissa based on polar coordinates calculation
Yp = R * np.sin(T)    #Based on polar coordinates to calculate the vertical
Zp = Xp**2 + Yp**2  #The abscissa and ordinate generation into the rotating parabolic function to get the vertical coordinates
ax.plot_surface( Xp, Yp, Zp, cstride = 1, rstride = 2, edgecolor = 'b' )  #Draw the rotating parabolic 
ax.set_xlabel( 'x' )
ax.set_ylabel( 'y' )
ax.set_zlabel( 'z' )
ax.view_init( 20, 45 )

上述代码运行结果为：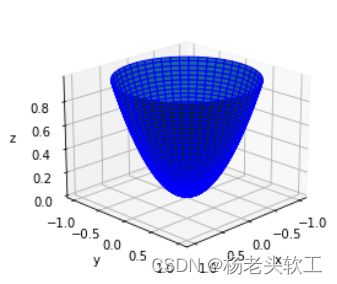

2、Draw the parabolicy=x^2
With the above drawing of rotating paraboloid experience,此处直接上代码.

fig = plt.figure()   #建立图形窗口
ax = fig.gca( projection = '3d' )  #三维坐标系
Xc, Zc = np.meshgrid( np.arange(-1, 1, 0.005), np.arange(0, 1, 0.005 ) )#Independent variable grid coordinates
Yc = Xc**2
ax.plot_surface( Xc, Yc, Zc, cstride = 1, rstride = 2, edgecolor = 'y' )#绘图
ax.set_xlabel( 'x' )
ax.set_ylabel( 'y' )
ax.set_zlabel( 'z' )
ax.view_init( 20, 45 )

上述代码运行结果为：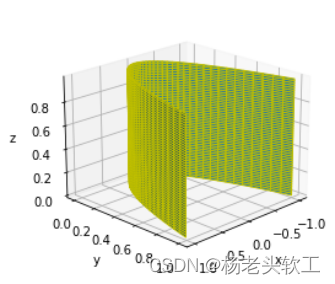

3、Draw with rotating parabolic plane with the same domainz=1
With the above drawing of rotating paraboloid experience,此处直接上代码.

fig = plt.figure()              #建立图形窗口
ax = fig.gca( projection = '3d' )  #三维坐标系
[ m, n ] = np.shape( Xp )
Zf = np.ones( Xp.shape )
ax.plot_surface( Xp, Yp, Zf, cstride = 1, rstride = 2, edgecolor = 'r' ) 
ax.set_xlabel( 'x' )
ax.set_ylabel( 'y' )
ax.set_zlabel( 'z' )
ax.view_init( 20, 45 )

上述代码运行结果为：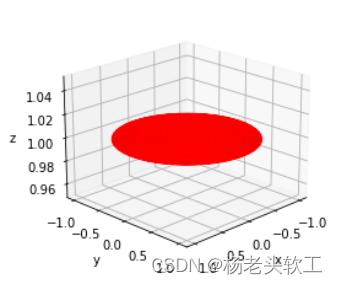

4、The above code into a running can get the graphic below：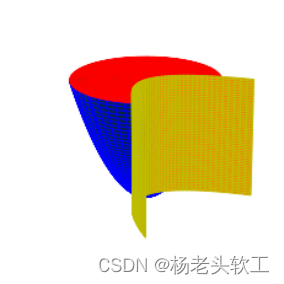

注意：Need to draw the parabolic and code in the following two statements to delete

fig = plt.figure()              #建立图形窗口
ax = fig.gca( projection = '3d' )  #三维坐标系

5、Delete redundant on each graphic primitives
从上图可以看出,结果不尽人意,If you can remove the surface spare parts,You can get better closed surface.So speak the following code is used to delete respectively select paraboloid、On the surface of the parabolic peace redundant data,Just need to make extra the vertical coordinate components of the primitive value ofNone即可.
1）Delete the parabolic redundant data（This method is of low efficiency）

[ mc, nc ] = np.shape( Xc )
for i in range( mc ):
    for j in range( nc ):
        if Zc[ i, j ] < Xc[ i, j ] * Xc[ i, j ] + Yc[ i, j ] * Yc[ i, j ]:
            Zc[ i, j ] = None
      ```
2）Delete selected paraboloid and redundant data（This method is of low efficiency）
```python
[ m, n ] = np.shape( Xp )
Zf = np.ones( Xp.shape )
for i in range( m ):
    for j in range( n ):
        if Yp[ i, j ] < Xp[ i, j ] * Xp[ i, j ]:
            Zf[ i, j ] = None
            Zp[ i, j ] = None

So you can get the following visual effect in good shape：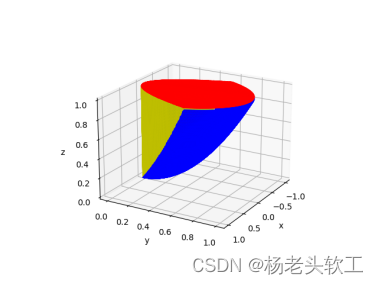

6、The complete drawing closed surface code

import matplotlib.pyplot as plt
import numpy as np
import math
#建立图形窗口
fig = plt.figure()
ax = fig.gca( projection = '3d' )#Axes3D( fig )

#旋转抛物面 Paraboloid 的图形数据
theta = np.arange( 0, math.pi, 0.01 )
rou   = np.arange( 0, 1, 0.01 )
T, R = np.meshgrid( theta, rou )
Xp = R * np.cos(T) 
Yp = R * np.sin(T)
Zp = Xp**2 + Yp**2

#抛物柱面 Parabolic Cylinder 的图形数据
Xc, Zc = np.meshgrid( np.arange(-1, 1, 0.01), np.arange(0, 1, 0.01 ) )
Yc = Xc**2
[ mc, nc ] = np.shape( Xc )
#Delete the parabolic redundant data
for i in range( mc ):
    for j in range( nc ):
        if Zc[ i, j ] < Xc[ i, j ] * Xc[ i, j ] + Yc[ i, j ] * Yc[ i, j ]:
            Zc[ i, j ] = None

#平面 flat surface 的图形数据
Zf = np.ones( Xp.shape )
[ m, n ] = np.shape( Xp )
#Deletes data plane and rotating parabolic redundant
for i in range( m ):
    for j in range( n ):
        if Yp[ i, j ] < Xp[ i, j ] * Xp[ i, j ]:
            Zf[ i, j ] = None
            Zp[ i, j ] = None
#绘图
ax.plot_surface( Xc, Yc, Zc, cstride = 1, rstride = 1, edgecolor = 'y' )
ax.plot_surface( Xp, Yp, Zp, cstride = 1, rstride = 1, edgecolor = 'b' )           
ax.plot_surface( Xp, Yp, Zf, cstride = 1, rstride = 1, edgecolor = 'r' )
ax.set_xlabel( 'x' )
ax.set_ylabel( 'y' )
ax.set_zlabel( 'z' )
ax.view_init( 20, 30 )