R language [logic control] [mathematical operation]

Catalog

One 、 Logical operators

  Two 、 Boolean operator

  Index and related contents

  3、 ... and 、 Mathematical operators

  Quadratic function

Trigonometric functions

  Polar coordinates

  With e The exponential function at the bottom

  Logarithmic function

  Four 、 Matrix correlation operations

1. The inner product of a vector

 2. The outer product of a vector

3. The transpose of the matrix  

 4. The addition and subtraction of matrices

 5. The multiplication of a matrix

 6. Matrix multiplication

 7. Operations related to the diagonal elements of a matrix

 8. Matrix inversion

 9. Eigenvalues and eigenvectors of matrices

 10. Matrix Choleskey decompose

 11. Calculate determinant

12. Extract the dimension of the matrix

 13. Row sum of matrix 、 Column sum 、 Row average and column average

  5、 ... and 、 Integral operations

6、 ... and 、 Negative number operation  

  7、 ... and 、 Mathematical functions

  8、 ... and 、 Other utility functions

Apply functions to data frames and matrices  

---

One 、 Logical operators

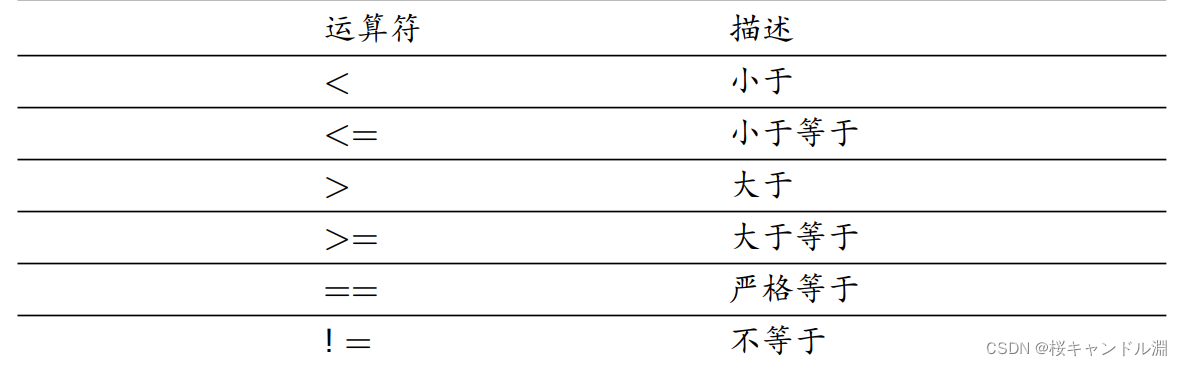
x <- c(3,0,-2,-5,7,2,-1)
y <- c(2,4,-1,0,5,1,-4)
x <= y
compare.xy <- (x<=y)
compare.xy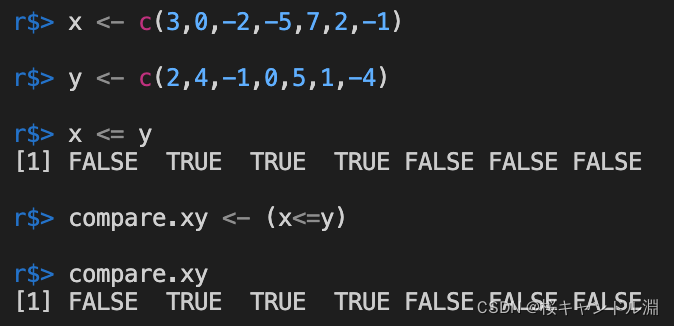

 

  Strings are compared in character order

a <- c("ann","gretchen","maria","ruth","wendy")
b <- c("bruce","ed","robert","seth","thomas")
a <= b
a >= b

  Two 、 Boolean operator

Operator	describe
！x	Not x
x|y	x or y
x&y	x and y
isTRUE(x)	Judge x Is it true

We can see from the following test code that if the element of the corresponding position meets the judgment of Boolean operator, it will return TRUE, If not, return to FALSE 

x <- c(3,0,-2,-5,7,2,-1)
y <- c(2,4,-1,0,5,1,-4)
(x-y > -2)&(x-y < 2)
x[(x-y> -2)&(x-y < 2)]
(x-y >= -2)|(x-y <= 2)
x[(x-y>-2)&(x-y<2)]
(x-y > -2)
!(x-y > -2)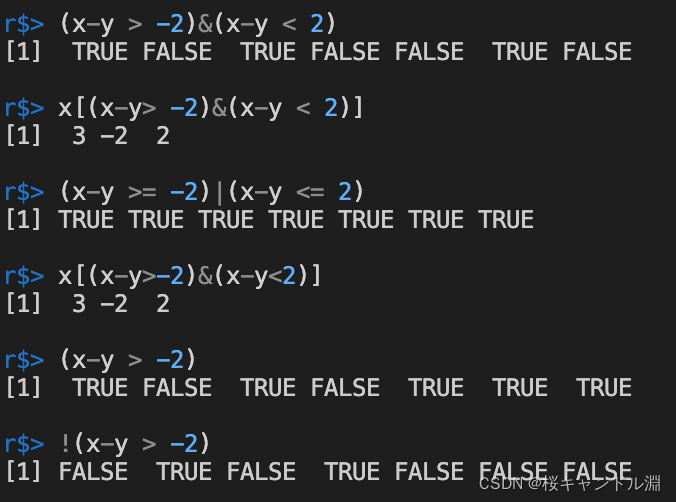

  Index and related contents

x <- c(3,7,5,-2,0,-8)
x[3]
x[1:3]
x[c(2,4,5)]
x[1+3]
i =4
x[i]
x[-2]
x[-c(2,4,5)]
y <- c(2,7,-5,4,-1,6)
(x+y)[1:3]
z <- c("jan","feb","mar","apr","may","jun")
monthly.numbers <- data.frame(x,y,z)
monthly.numbers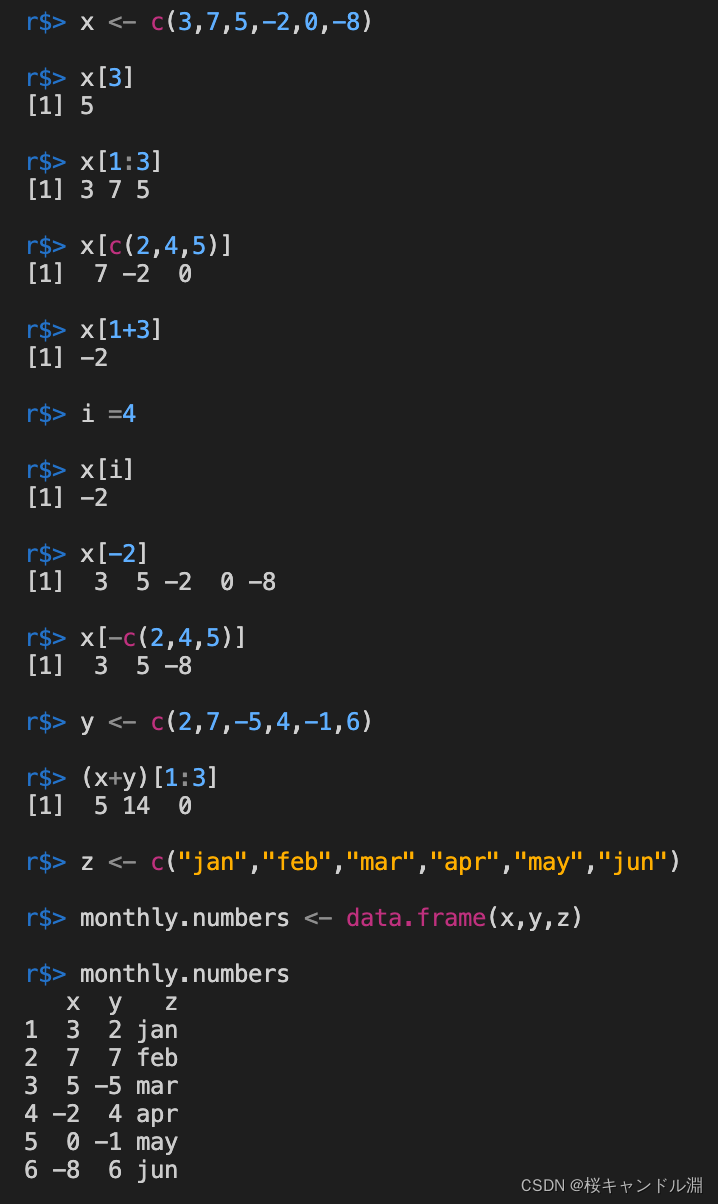

  3、 ... and 、 Mathematical operators

3+4 # 7
3-4 # -1
3*4 # 12
3/4 # 0.75
3^4 # 81
16**(1/4) # 2
13%%4 # 1
13%/%4 # 3
x <- c(-1,0,1)
exp(x)
y <- c(1,2,3,4)
sqrt(y)

 

  Quadratic function

xlow <- -1
xup <- 2
x <- xlow + (xup-xlow)*(0:100)/100
y <- x^2-x-1
plot(x,y,type="l")
y2 <- numeric(length(x))
# Draw a dot , Respectively by x,y2 The parameters of the corresponding position in the form of coordinate points , The type of line drawn is l, The straight line is a dotted line 
#lty Parameters of can also be input 1,2,3 And other parameters to draw different styles of dimension lines 
points(x,y2,type="l",lty="dashed")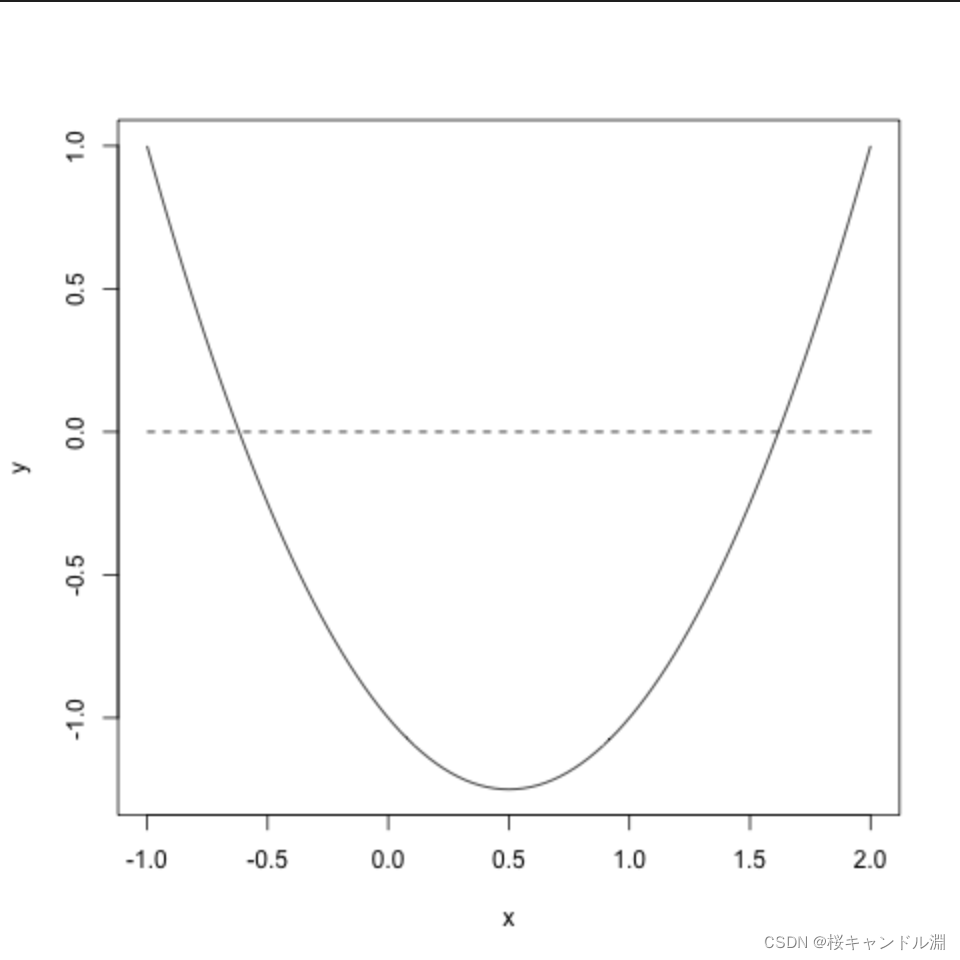

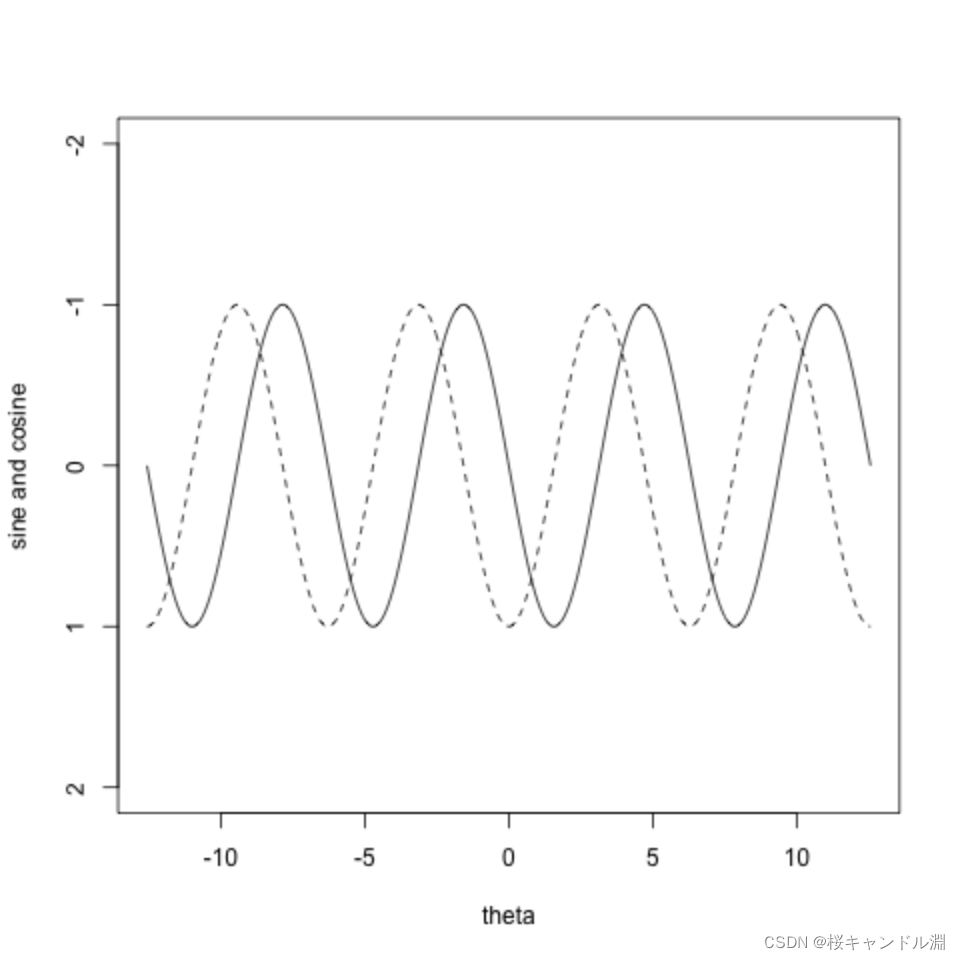
Trigonometric functions

# Set the upper and lower limits 
th.low <- -4*pi
th.up <- 4*pi
theta <- th.low + (th.up-th.low)*(0:1000)/1000
y1 <- sin(theta)
y2 <- cos(theta)
plot(theta,y1,type="l",lty=1,ylim=c(2,-2),xlab="theta",
ylab="sine and cosine")
points(theta,y2,type="l",lty=2)

  Polar coordinates

# Set the angle to 0-360 degree 
theta <- 2*pi*(0:100)/100
r <- 1
x <- r*cos(theta)
y <- r*sin(theta)
# Set the size, length and width of our canvas to 4 Inch .
par(pin=c(4,4))
plot(x,y,type="l",lty=1)

  With e The exponential function at the bottom

Use exp Function can be set to e Exponential function with base .

x <- 2
y <- exp(x)
z <- exp(0:10)
y
z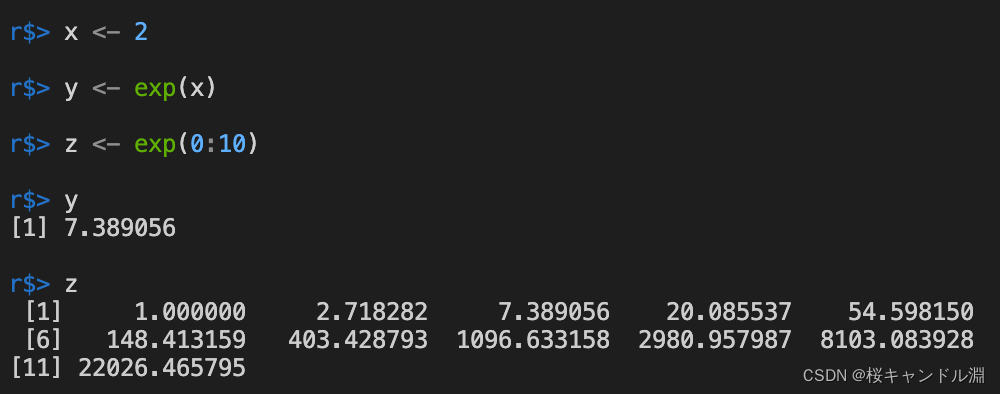

  Logarithmic function

y = loga x, R Is represented by log(x,a)
y = ln x, R Is represented by log(x)
y = lg x, R Is represented by log10(x)
y = log2 x, R Is represented by log2(x)

If direct log(X) The default is e Bottom , That is to say ln(X)

 

w <- 0
log(w)
w <- 1:10
log(w)
w <- 10000
log10(w)
w <- 16
log2(w)
log(9,3)
log(64,8)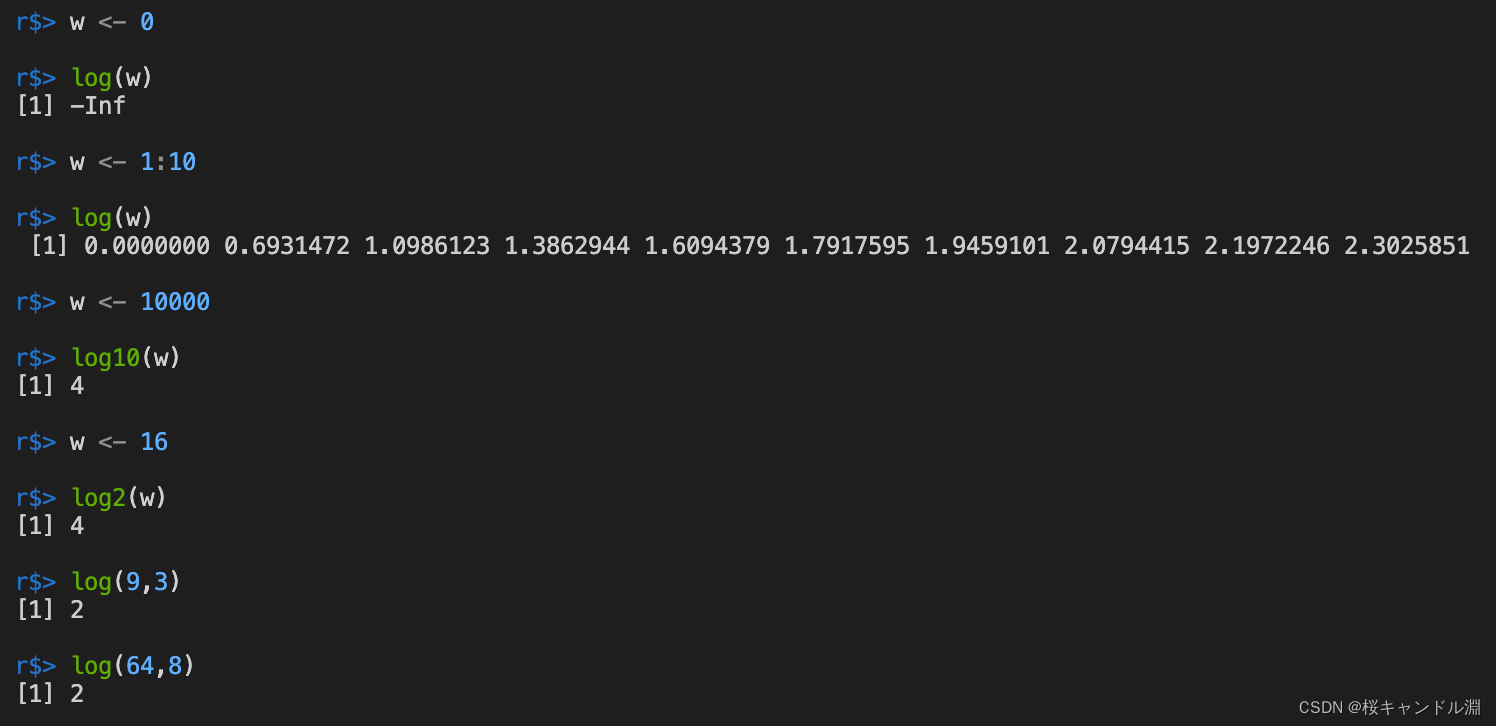

  Four 、 Matrix correlation operations

1. The inner product of a vector

Use %*% perhaps crossprod Can generate the result of the inner product of vectors .

x <- 1:5 
x
y <- 2*1:5
y
x %*% y 
crossprod(x,y)

 2. The outer product of a vector

Use %o% perhaps tcrossprod perhaps outer Can get the outer product of the vector , The outer product is the multiplication of elements in the same position

x %o% y 
tcrossprod(x,y)
outer(x,y)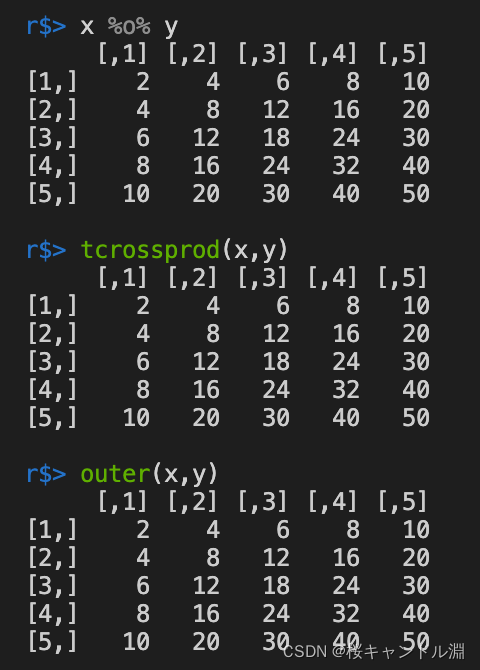

3. The transpose of the matrix  

Use the transpose command t(x) We can realize the transpose of our matrix

x <- matrix(1:12,nrow=3)
x
y = matrix(1:12,ncol=3)
y
z = matrix(1:12,ncol=3, byrow=T)
z
X = t(x)
X
Y <- t(t(y))
Y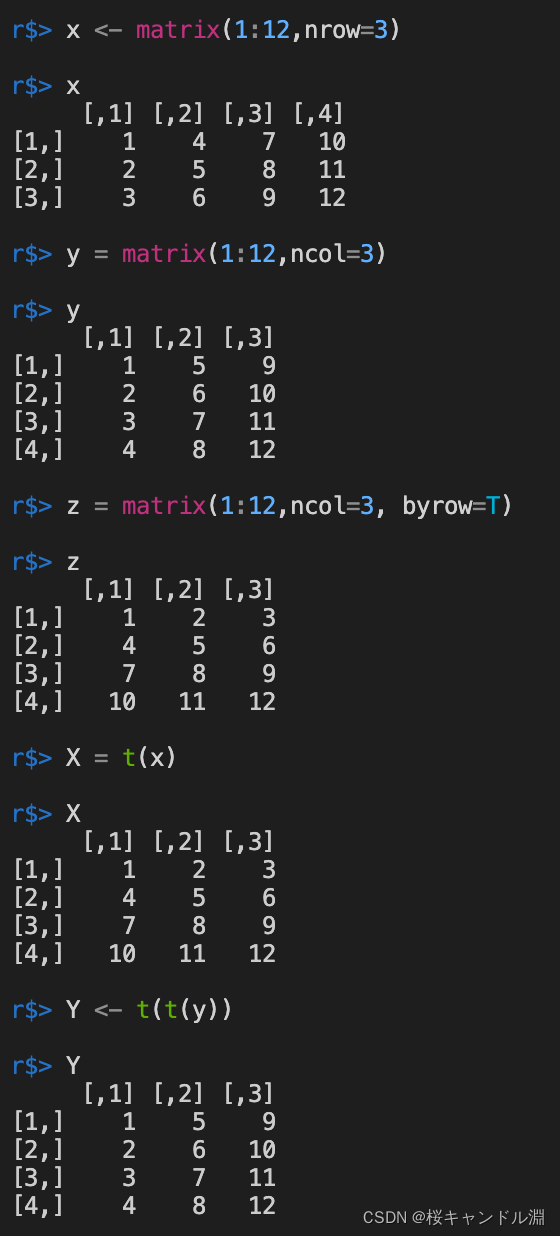

 4. The addition and subtraction of matrices

x+t(z)
x-t(z)
x + 1
y <- 1:12
x + y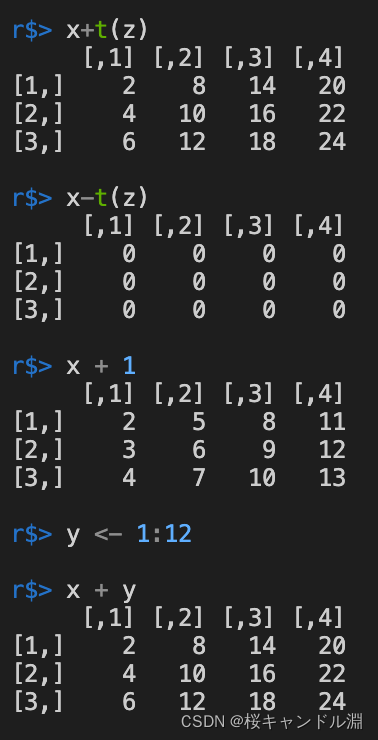

 5. The multiplication of a matrix

Matrix multiplication will multiply every element in the matrix

a <- 3
M <- a*x
M

 6. Matrix multiplication

Use %*% perhaps crossprod Can realize matrix multiplication

A = matrix(1:12,ncol=3)
B = matrix(1:18, nrow=3)
A
B
C = A%*%B
C
D = matrix(1:12, nrow=4, byrow=T)
D
t(A) %*% D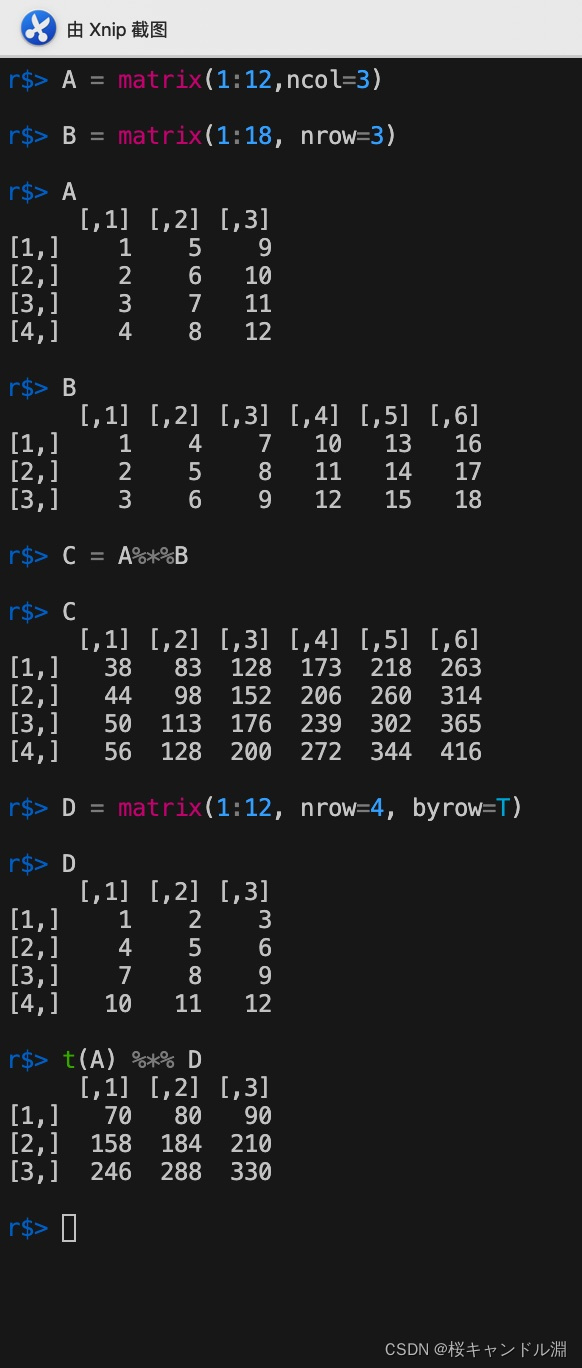

 

  Used crossprod after , We don't need to transpose , It can also realize our A And D Multiplication of matrices . by comparison crossprod More efficient , in other words crossprod The matrix of the first parameter will be transposed and then multiplied by the matrix of the second parameter .

crossprod(A,D)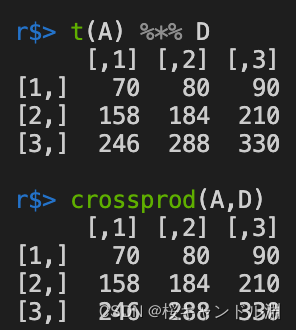

 7. Operations related to the diagonal elements of a matrix

Use diag Function can get the elements on the diagonal of the current matrix , And splice it into a vector .

If diag If the parameter of is a vector , It will help us generate a diagonal matrix .

A = matrix(1:16,nrow=4)
A
diag(A)
diag(diag(A))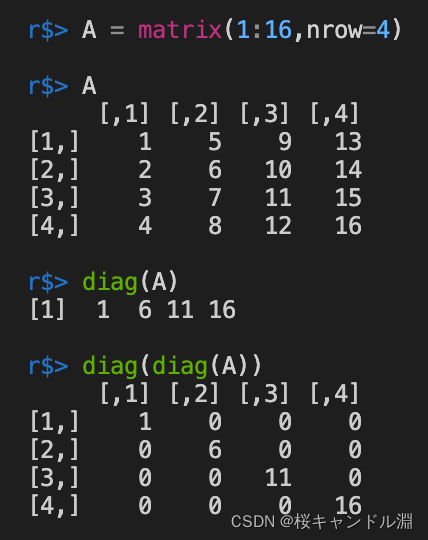 

Generate a n The unit matrix of dimension

diag(n)
n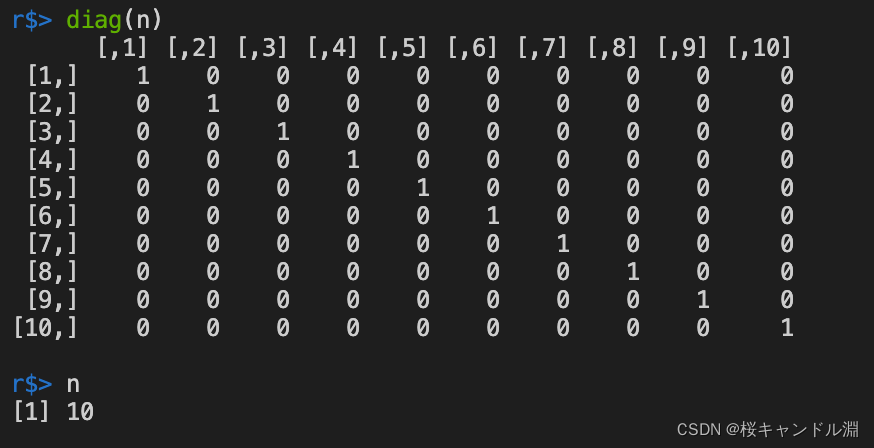

When diag When the parameter of is a vector , It will return a matrix with this vector as the main diagonal element .

x<-c(1,2,3,4,5)
diag(x)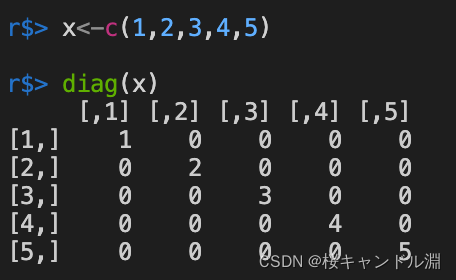

 

 8. Matrix inversion

Use solve Function can find the inverse of our current matrix

AX=b,X=solve(A,b)

A = matrix(c(1,2,3,4,7,5,2,1,3,1,3,2,3,2,3,2),ncol=4)
A
solve(A) 
b <- c(1,2,3,4)
# Calculation equations AX=b Solution 
solve(A,b)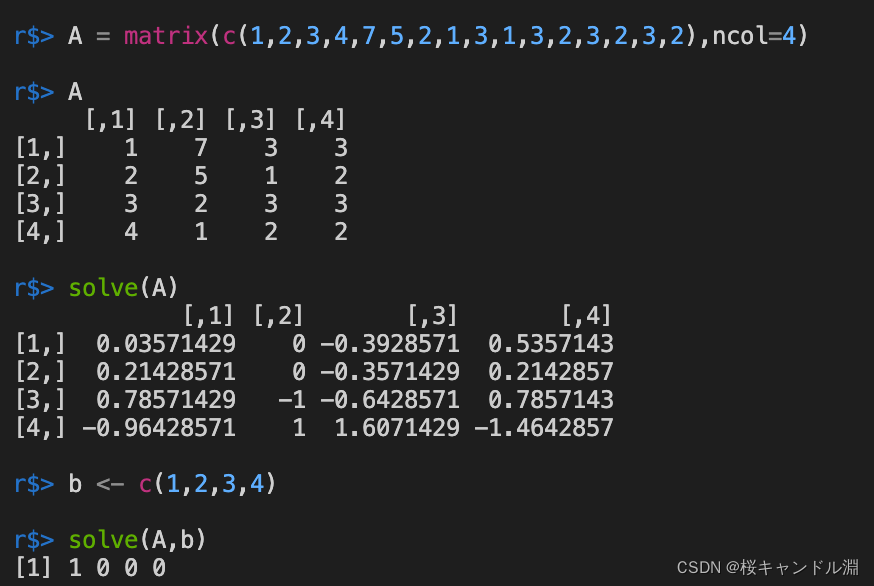

 9. Eigenvalues and eigenvectors of matrices

Use eigen This method can calculate the eigenvalue of our current matrix and the corresponding eigenvector

values Represents the eigenvalue

vectors Represents the corresponding eigenvector

A = diag(4)+1
A
A.eigen = eigen(A,symmetric=T) 
A.eigen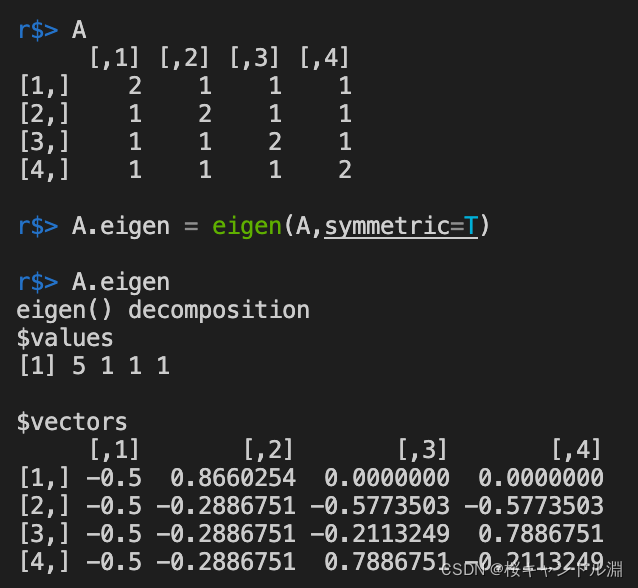

 10. Matrix Choleskey decompose

Use our chol Method , It can realize the decomposition of our current matrix .

That is to say, Qiujiang A Decompose into P'P Of P Matrix

A = diag(4)+1
A
P <- chol(A)
P

 11. Calculate determinant

Use det Function can calculate the determinant of our current matrix .

det(A)

12. Extract the dimension of the matrix

dim(A) Calculation of matrix A The number of rows and columns

ncol(A) Return matrix A Columns of

nrow(A) Return matrix A The number of rows

dim(A) 
ncol(A)
nrow(A) 
A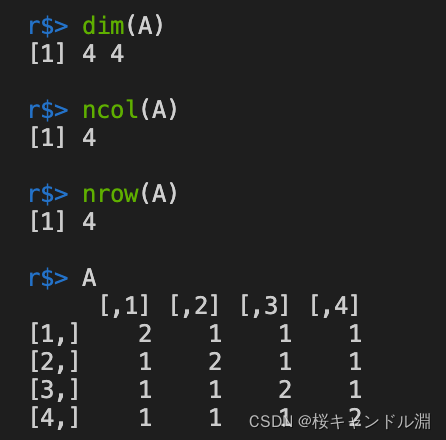

 13. Row sum of matrix 、 Column sum 、 Row average and column average

A=matrix(1:16,ncol=4)
A
# Row sum 
rowSums(A)
# Row average 
rowMeans(A)
# Column sum 
colSums(A)
# Column average 
colMeans(A)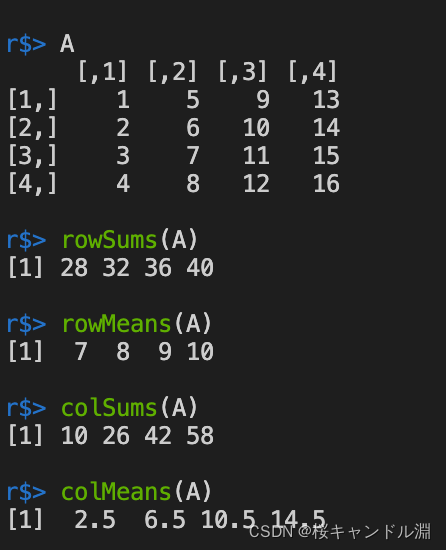

  5、 ... and 、 Integral operations

integrate()

The first parameter is our formula for calculating the integral , The second parameter is our lower bound , The third parameter is our upper limit . In the following code Inf Infinity

integrand <- function(x) {1/((x+1)*sqrt(x))}
integrate(integrand, lower=0, upper=Inf)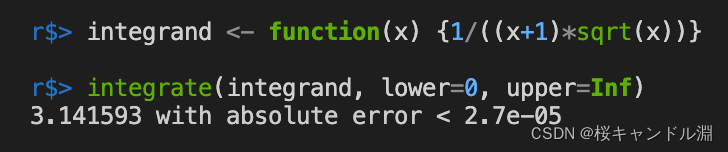

 

6、 ... and 、 Negative number operation  

Generate complex vector .

complex(length.out,real=numeric(0),imaginary=numeric(0))

x <- 1+2i
y <- complex(1,1,1)
# Take the real part 
Re(y)
# Take imaginary part  
Im(y)
# Find module length  
Mod(y)
# Find conjugate complex number 
Conj(y) 
# Judge whether it is plural 
is.complex(y)
# Generate complex vector group 
complex(re=1:4,im=2:3) 
# Yes -2 Square root is not feasible 
sqrt(-2) 
# Yes -2+0i The square root is ok , Because this is a complex number operation 
sqrt(-2+0i)
# If you want to be direct to -2 Find the square root , Or you could just say -2 Convert to plural form and open square root .
sqrt(as.complex(-2))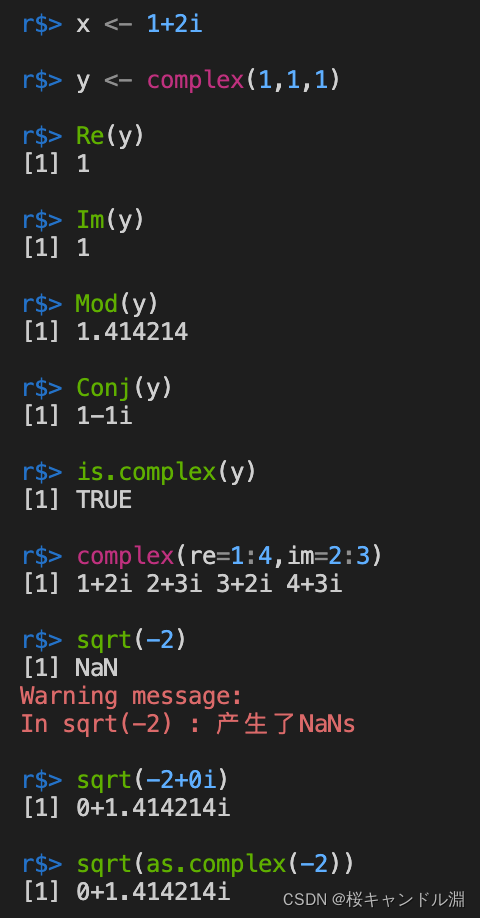

 

  7、 ... and 、 Mathematical functions

 

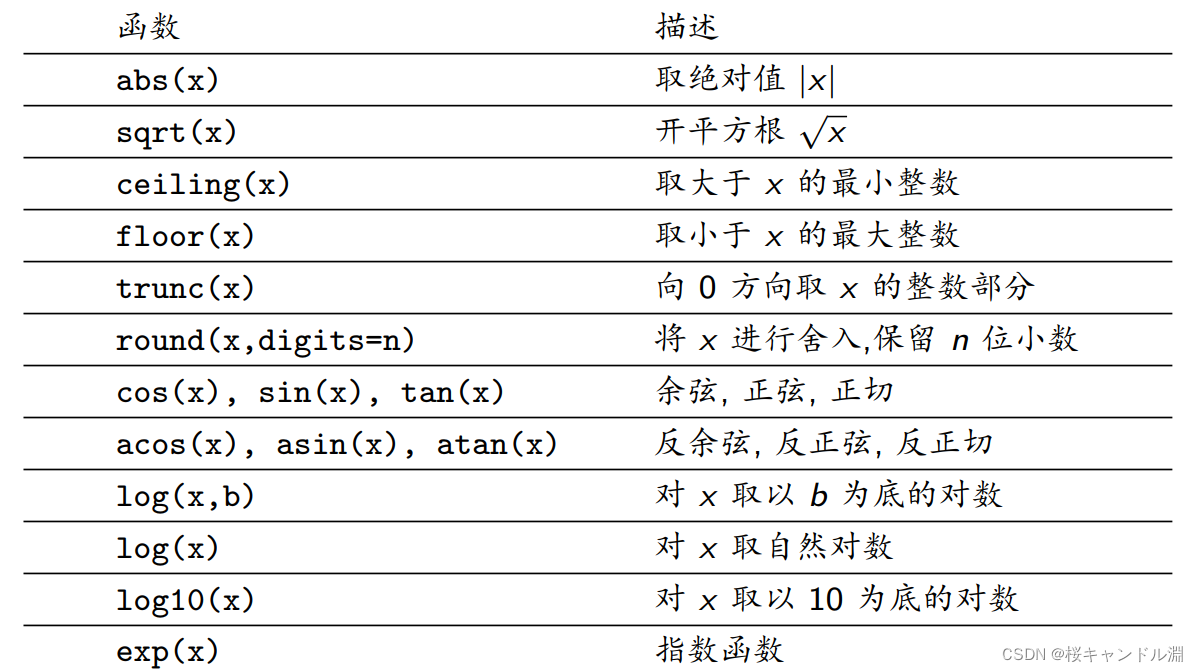
abs(-4.21) # 4.21
sqrt(16) # 4
ceiling(3.78) # 4
floor(3.78) # 3
floor(-3.78) # -4
trunc(-3.78) # -3
trunc(3.78) # 3
round(3.1415926, digits=4) # 3.1415
log(10,2) # 3.321928
log(10) # 2.302585
log10(10) # 1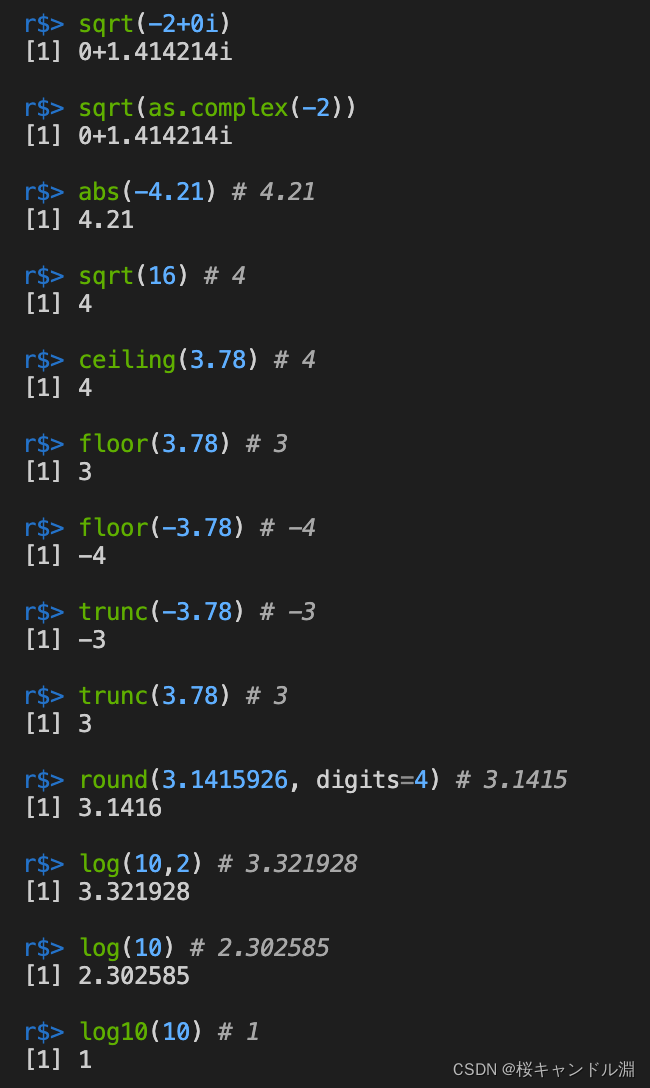

  8、 ... and 、 Other utility functions

 

x<- c(1,2,3,4)
length(x) 
rep(x,2) 
rep(1,10) 
seq(1,2,by=0.2) 

Apply functions to data frames and matrices  

b <- c(1.23, 4.271, 9.2727)
# towards 0 Round the direction 
trunc(b)
#runif(n, min, max) function , This function generates uniformly distributed values ,n Number of ,min and max Are the minimum and maximum values respectively , The default parameter is 0 and 1.
d <- matrix(runif(12),nrow=3)
# Take the logarithm of each element 
log(d)