当前位置:网站首页>Application of Lie group in gtsam
Application of Lie group in gtsam
2022-07-06 06:01:00 【Zhan Miao】
1. AdjointMap Definition
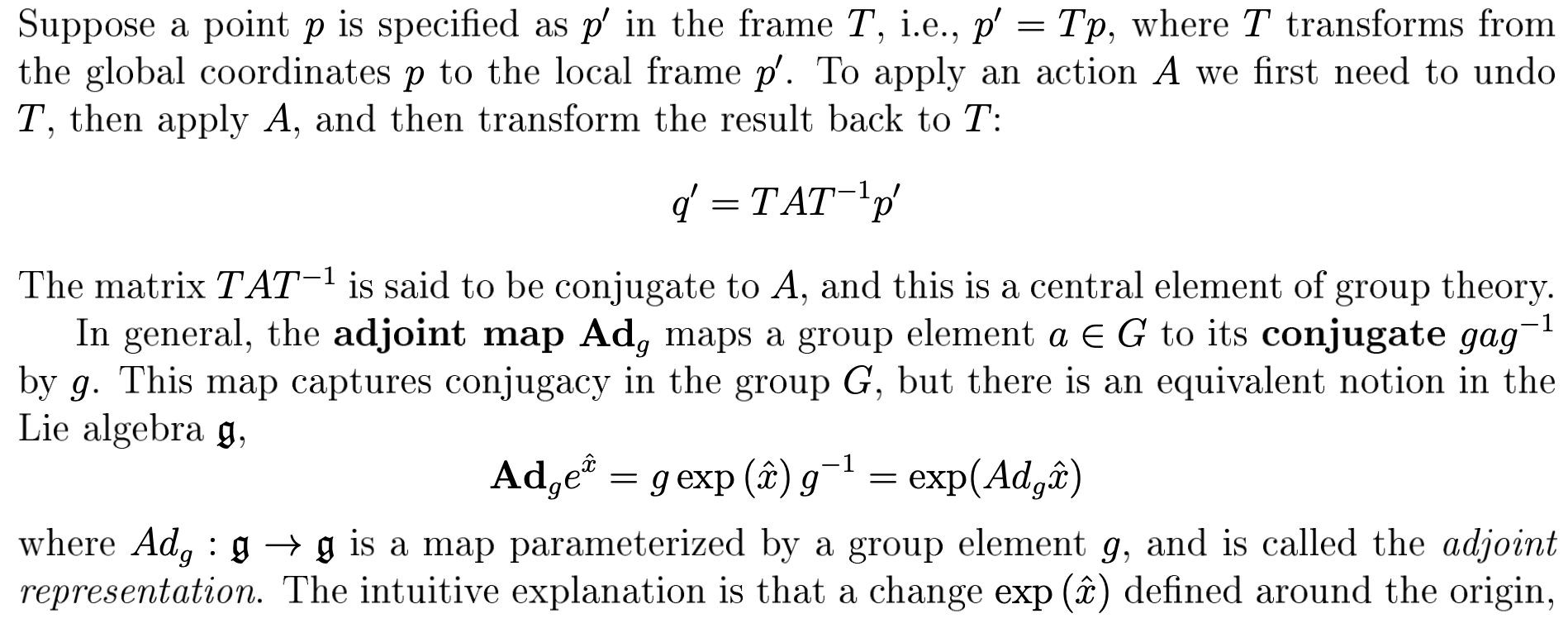
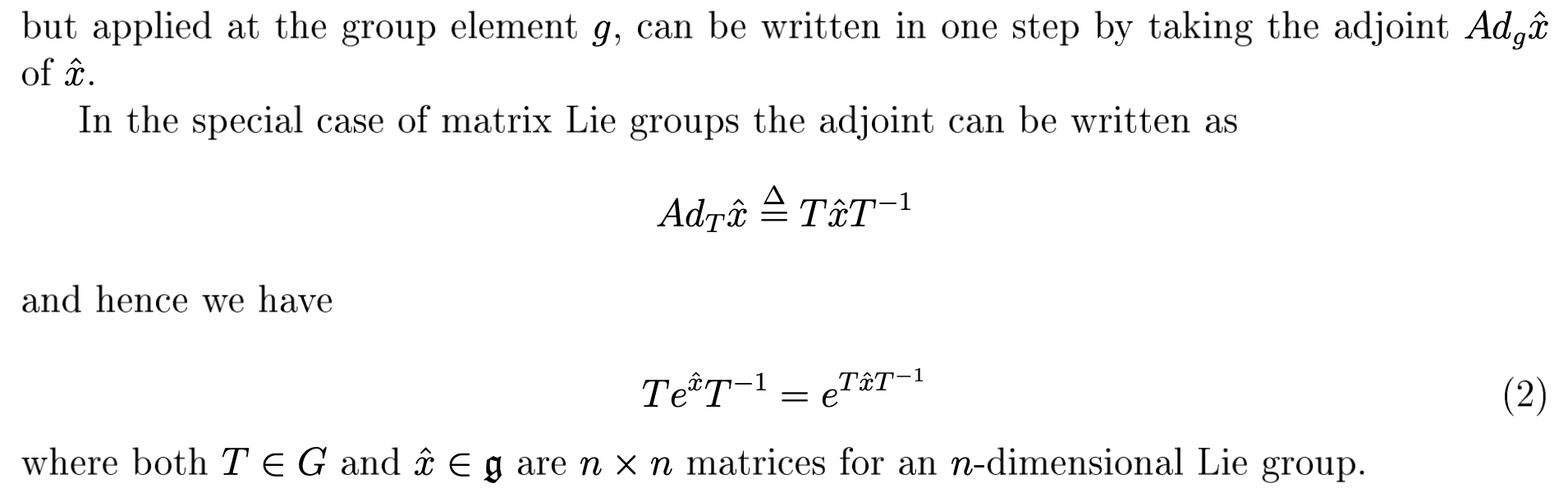
Why in matrix Lie group , The above two definitions can be mixed ? Prove the following

2. Li Qun's AdjointMap
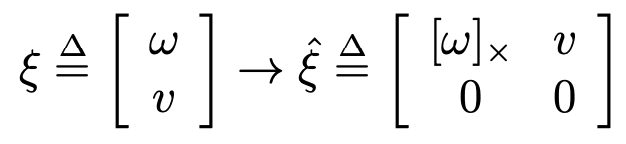
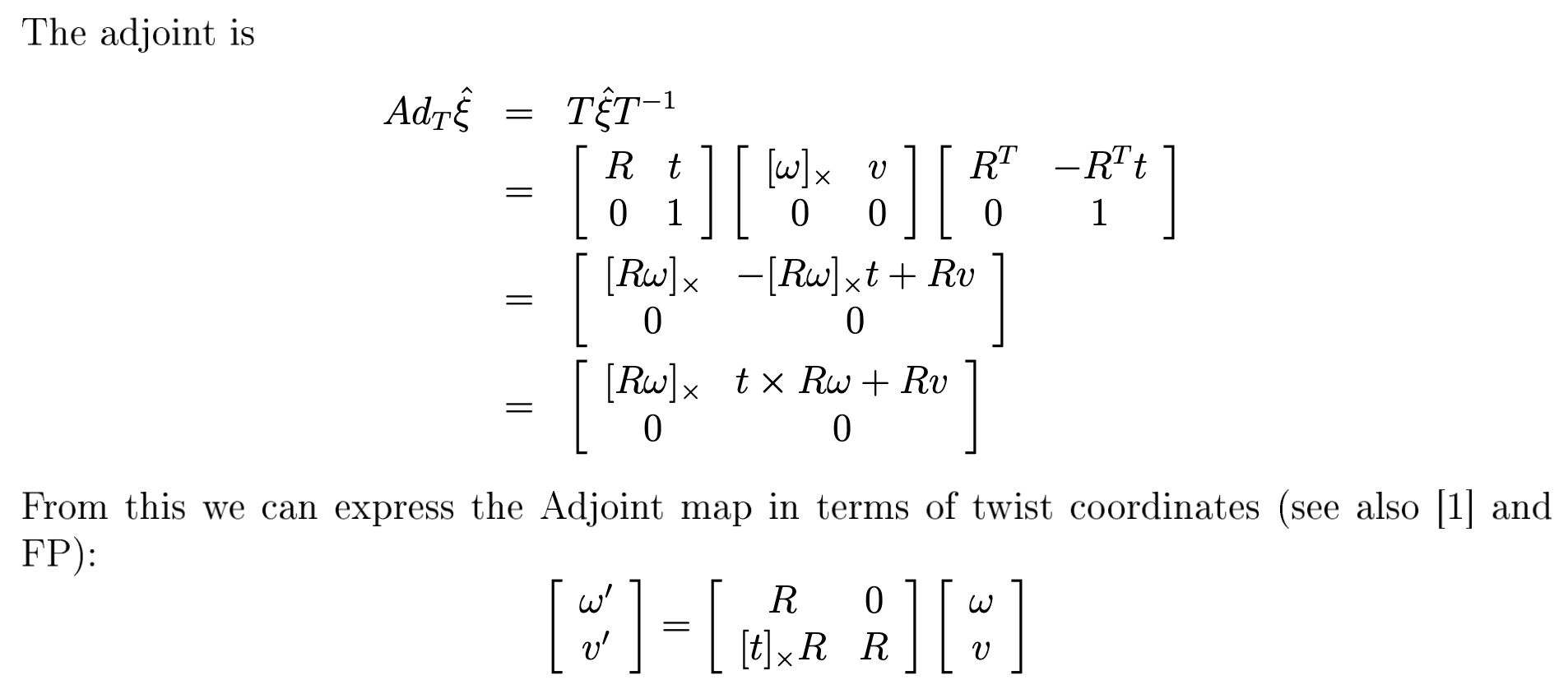
How can the above formula lead to the following conclusion
AdjointMap Is the mapping from antisymmetric matrix to antisymmetric matrix
Make

Then the above formula can be written as the following formula
![\begin{bmatrix} [\omega']_{\times} & v' \\ 0 & 0 \end{bmatrix} = \begin{bmatrix} [R\omega]_{\times} & t \times R\omega + Rv \\ 0 & 0 \end{bmatrix} \Rightarrow \begin{cases} \omega' = R \omega \\ v' = t \times R\omega + Rv \end{cases}](http://img.inotgo.com/imagesLocal/202207/06/202207060557471698_2.gif)
Then we get the following formula
3. Local Coordinates

There is such a formula

Prove the following

4. ImuFactor
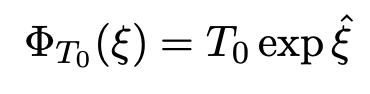
![]()
![\frac{\partial R_k}{\partial \theta_k} = H(\theta_k) = \sum_{k=0}^{\infty} \frac{(-1)^k}{(k+1)!}[\theta]_{\times}^k](http://img.inotgo.com/imagesLocal/202207/06/202207060557471698_9.gif)
prove
from
![]() have to
have to
![\frac{\partial R_k}{\partial \theta_k} \\ = \lim_{\delta \rightarrow0} \frac{ exp([\theta + \delta]_{\times}) \ominus exp([\theta]_{\times}) }{\delta} \\ = \lim_{\delta \rightarrow0} \frac{Log \left( exp([-\theta]_{\times}) exp([\theta + \delta]_{\times}) \right) }{\delta} \\ = \lim_{\delta \rightarrow0} \frac{Log \left( exp([-\theta]_{\times}) exp([\theta]_{\times}) exp([H(\theta)\delta]_{\times}) \right) }{\delta} \\ = \lim_{\delta \rightarrow0} \frac{Log \left( exp([H(\theta)\delta]_{\times}) \right) }{\delta} \\ = H(\theta)](http://img.inotgo.com/imagesLocal/202207/06/202207060557471698_7.gif)
边栏推荐
- Li Chuang EDA learning notes 12: common PCB board layout constraint principles
- Analysis of grammar elements in turtle Library
- [email protected] raspberry pie
- Interface test: what are the components of the URL in fiddler
- Garbage collector with serial, throughput priority and response time priority
- IDEA 新UI使用
- 假设检验学习笔记
- About PHP startup, mongodb cannot find the specified module
- Yygh-11-timing statistics
- 华为路由器忘记密码怎么恢复
猜你喜欢

Report on market depth analysis and future trend prediction of China's arsenic trioxide industry from 2022 to 2028
Sqlmap tutorial (III) practical skills II
![[Baiwen smart home] first day of the course_ Learn Embedded and understand the development mode of bare metal and RTOS](/img/ed/8d112054f31bd7e593050d1278b9f1.jpg)
[Baiwen smart home] first day of the course_ Learn Embedded and understand the development mode of bare metal and RTOS
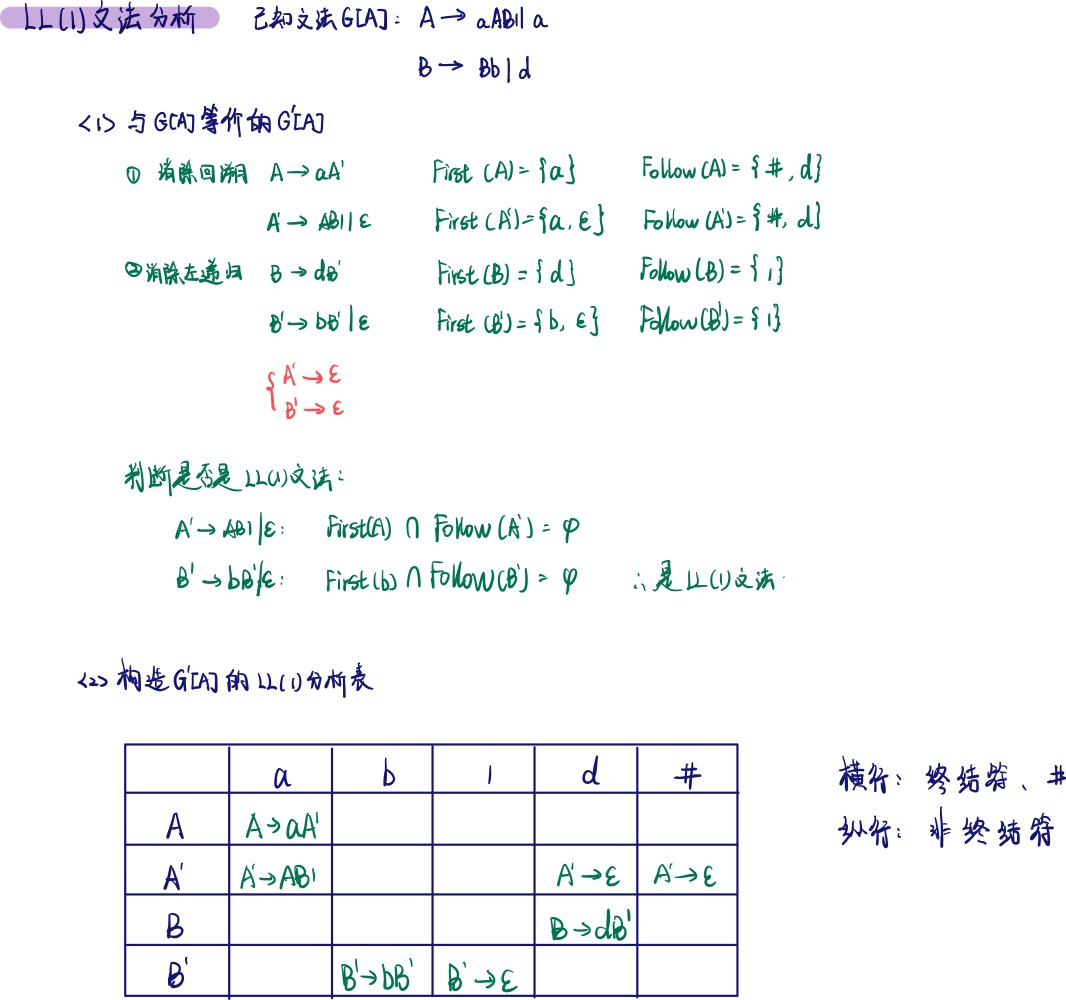
【课程笔记】编译原理

Raised a kitten
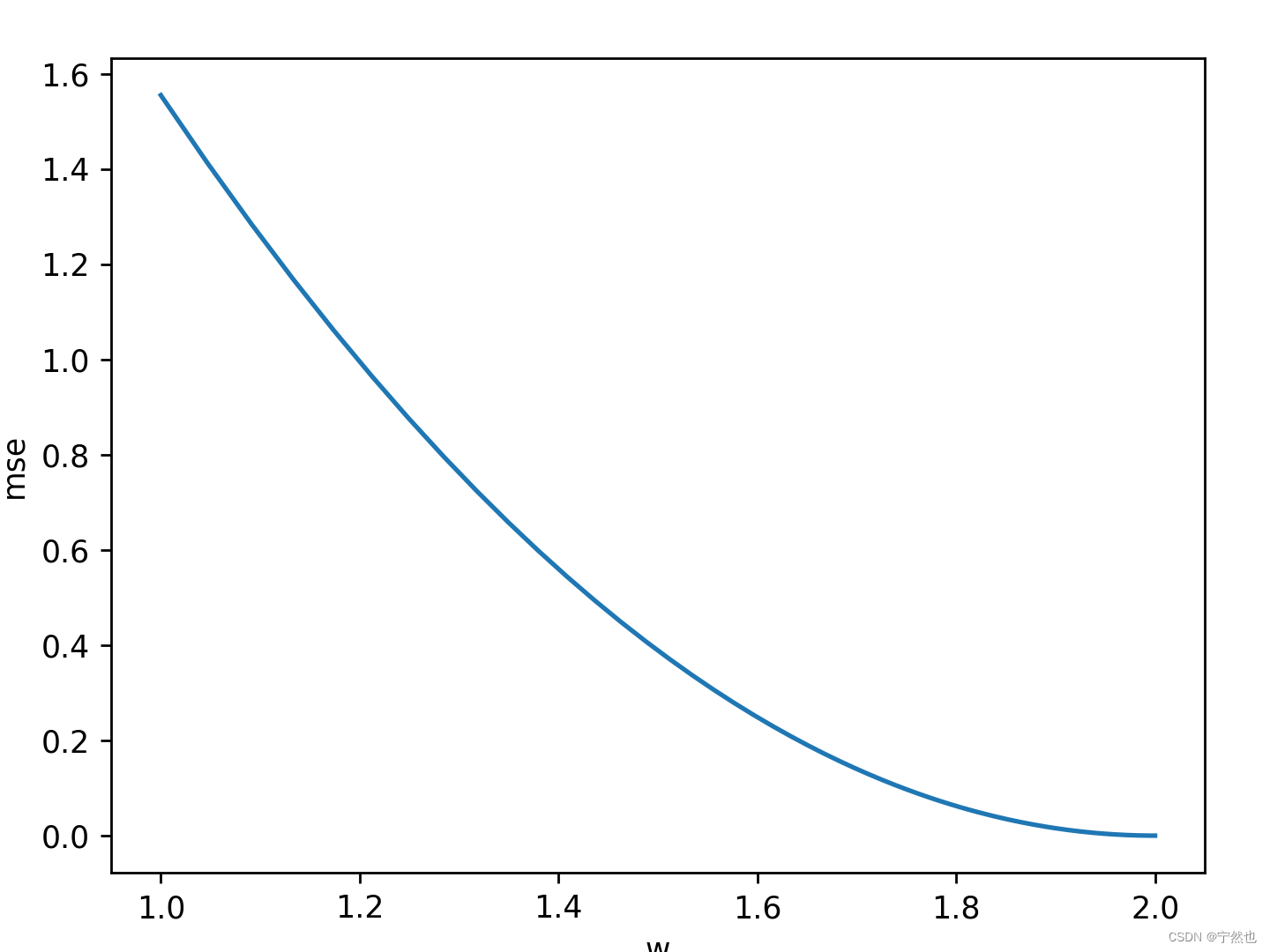
Station B Liu Erden - linear regression and gradient descent
Request forwarding and redirection

Embedded interview questions (IV. common algorithms)

A master in the field of software architecture -- Reading Notes of the beauty of Architecture
关于 PHP 启动 MongoDb 找不到指定模块问题
随机推荐
Winter 2021 pat class B problem solution (C language)
Zoom through the mouse wheel
多线程应用的测试与调试
[untitled]
Linux regularly backs up MySQL database
Go language -- language constants
H3C V7版本交换机配置IRF
[course notes] Compilation Principle
Detailed explanation of BF and KMP
[leetcode] day96 - the first unique character & ransom letter & letter ectopic word
Arrays and collections
nodejs实现微博第三方登录
Station B, Master Liu Er - dataset and data loading
Hongliao Technology: how to quickly improve Tiktok store
Buuctf-[gxyctf2019] no dolls (xiaoyute detailed explanation)
误差的基本知识
Baidu online AI competition - image processing challenge: the 8th program of handwriting erasure
Request forwarding and redirection
Memory and stack related concepts
【课程笔记】编译原理