当前位置:网站首页>Codeforces Round #771 (Div. 2)
Codeforces Round #771 (Div. 2)
2022-07-06 16:42:00 【Dog egg L】
A. Reverse
You are given a permutation p1,p2,…,pn of length n. You have to choose two integers l,r (1≤l≤r≤n) and reverse the subsegment [l,r] of the permutation. The permutation will become p1,p2,…,pl−1,pr,pr−1,…,pl,pr+1,pr+2,…,pn.
Find the lexicographically smallest permutation that can be obtained by performing exactly one reverse operation on the initial permutation.
Note that for two distinct permutations of equal length a and b, a is lexicographically smaller than b if at the first position they differ, a has the smaller element.
A permutation is an array consisting of n distinct integers from 1 to n in arbitrary order. For example, [2,3,1,5,4] is a permutation, but [1,2,2] is not a permutation (2 appears twice in the array) and [1,3,4] is also not a permutation (n=3 but there is 4 in the array).
Input
Each test contains multiple test cases. The first line contains a single integer t (1≤t≤500) — the number of test cases. Description of the test cases follows.
The first line of each test case contains a single integer n
(1≤n≤500) — the length of the permutation.
The second line of each test case contains n
integers p1,p2,…,pn (1≤pi≤n) — the elements of the permutation.
Output
For each test case print the lexicographically smallest permutation you can obtain.
Example
Input
4
1
1
3
2 1 3
4
1 4 2 3
5
1 2 3 4 5
Output
1
1 2 3
1 2 4 3
1 2 3 4 5
Note
In the first test case, the permutation has length 1, so the only possible segment is [1,1]. The resulting permutation is [1].
In the second test case, we can obtain the identity permutation by reversing the segment [1,2]. The resulting permutation is [1,2,3].
In the third test case, the best possible segment is [2,3]. The resulting permutation is [1,2,4,3].
In the fourth test case, there is no lexicographically smaller permutation, so we can leave it unchanged by choosing the segment [1,1]. The resulting permutation is [1,2,3,4,5].
#include <bits/stdc++.h>
using namespace std;
int n,t,p[510];
int main()
{
cin>>t;
while(t--){
cin>>n;
for(int i=1;i<=n;i++)cin>>p[i];
for(int i=1;i<=n;i++){
if(p[i]!=i){
int j=i+1;
for(;j<=n;j++)
if(p[j]==i)break;
reverse(p+i,p+j+1);
break;
}
}
for(int i=1;i<=n;i++)cout<<p[i]<<' ';
cout<<'\n';
}
return 0;
}
B. Odd Swap Sort
You are given an array a1,a2,…,an. You can perform operations on the array. In each operation you can choose an integer i (1≤i<n), and swap elements ai and ai+1 of the array, if ai+ai+1is odd.
Determine whether it can be sorted in non-decreasing order using this operation any number of times.
Input
Each test contains multiple test cases. The first line contains a single integer t
(1≤t≤105) — the number of test cases. Description of the test cases follows.
The first line of each test case contains a single integer n
(1≤n≤105) — the length of the array.
The second line of each test case contains n
integers a1,a2,…,an (1≤ai≤109) — the elements of the array.
It is guaranteed that the sum of n
over all test cases does not exceed 2⋅105.
Output
For each test case, print “Yes” or “No” depending on whether you can or can not sort the given array.
You may print each letter in any case (for example, “YES”, “Yes”, “yes”,“yEs” will all be recognized as positive answer).
Example
Input
4
4
1 6 31 14
2
4 2
5
2 9 6 7 10
3
6 6 6
Output
Yes
No
No
Yes
Note
In the first test case, we can simply swap 31
and 14 (31+14=45 which is odd) and obtain the non-decreasing array [1,6,14,31].
In the second test case, the only way we could sort the array is by swapping 4and 2, but this is impossible, since their sum 4+2=6is even.
In the third test case, there is no way to make the array non-decreasing.
In the fourth test case, the array is already non-decreasing.
#include <bits/stdc++.h>
using namespace std;
typedef long long ll;
int main()
{
int t;
cin>>t;
while(t--){
ll n, x;
cin >> n;
ll even = 0 , odd = 0;
bool flag = true;
for (int i = 0; i < n; i++)
{
cin >> x;
if (x & 1)
{
if (x < odd)flag = false;
odd = max(odd, x);
}
else
{
if (x < even)flag = false;
even = max(x, even);
}
}
if (flag)cout << "YES" << endl;
else cout << "NO" << endl;
}
return 0;
}
C. Inversion Graph
You are given a permutation p1,p2,…,pn. Then, an undirected graph is constructed in the following way: add an edge between vertices i, j such that ipj. Your task is to count the number of connected components in this graph.
Two vertices uand v belong to the same connected component if and only if there is at least one path along edges connecting u and v.
A permutation is an array consisting of n distinct integers from 1 to n in arbitrary order. For example, [2,3,1,5,4] is a permutation, but [1,2,2] is not a permutation (2 appears twice in the array) and [1,3,4] is also not a permutation (n=3 but there is 4in the array).
Input
Each test contains multiple test cases. The first line contains a single integer t (1≤t≤105) — the number of test cases. Description of the test cases follows.
The first line of each test case contains a single integer n (1≤n≤105) — the length of the permutation.
The second line of each test case contains n
integers p1,p2,…,pn (1≤pi≤n) — the elements of the permutation.
It is guaranteed that the sum of n
over all test cases does not exceed 2⋅105.
Output
For each test case, print one integer k — the number of connected components.
Example
Input
6
3
1 2 3
5
2 1 4 3 5
6
6 1 4 2 5 3
1
1
6
3 2 1 6 5 4
5
3 1 5 2 4
Output
3
3
1
1
2
1
Note
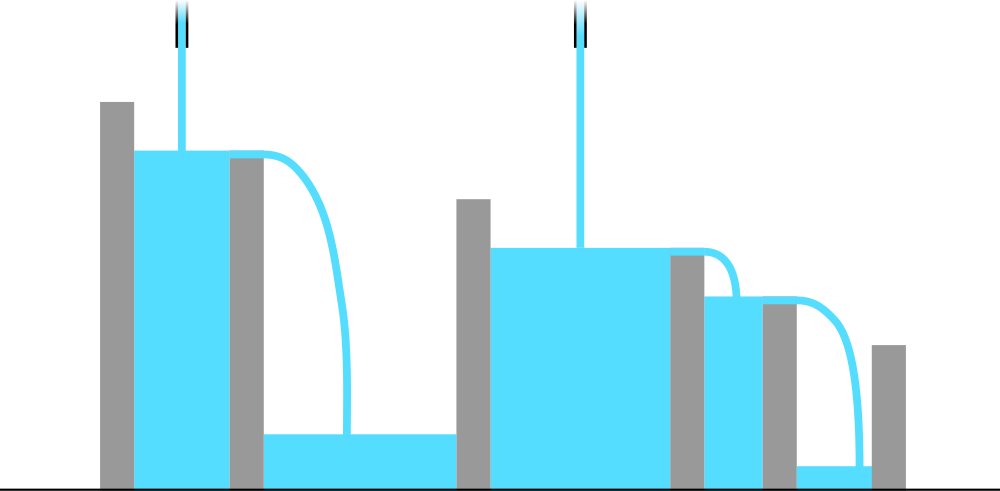
Each separate test case is depicted in the image below. The colored squares represent the elements of the permutation. For one permutation, each color represents some connected component. The number of distinct colors is the answer.
#include <bits/stdc++.h>
using namespace std;
int main(){
int t ;
cin>>t;
while(t -- ) {
int n ;
cin>> n ;
map<int , int> p ;
int res= 0 , maxx = 1 ;
for(int i = 1 ; i <= n ; i ++ ) {
int k ;
cin>>k ;
p[k] = 1 ;
while(p[maxx]) maxx ++ ;
if(maxx > i ) res ++ ;
}
cout<<res <<"\n" ;
}
return 0 ;
}
边栏推荐
- (lightoj - 1323) billiard balls (thinking)
- Codeforces Round #803 (Div. 2)A~C
- Simple records of business system migration from Oracle to opengauss database
- 图像处理一百题(1-10)
- Installation and configuration of MariaDB
- 我在字节跳动「修电影」
- Research Report on market supply and demand and strategy of China's tetraacetylethylenediamine (TAED) industry
- Classic application of stack -- bracket matching problem
- MP4格式详解
- Research Report on market supply and demand and strategy of double drum magnetic separator industry in China
猜你喜欢

Codeforces Round #799 (Div. 4)A~H
Li Kou - 298th weekly match
Pull branch failed, fatal: 'origin/xxx' is not a commit and a branch 'xxx' cannot be created from it
sublime text 代码格式化操作

< li> dot style list style type
300th weekly match - leetcode
Codeforces Round #802(Div. 2)A~D
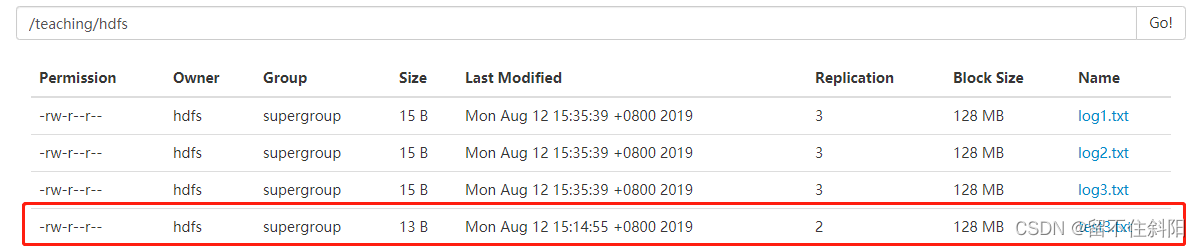
Chapter 2 shell operation of hfds
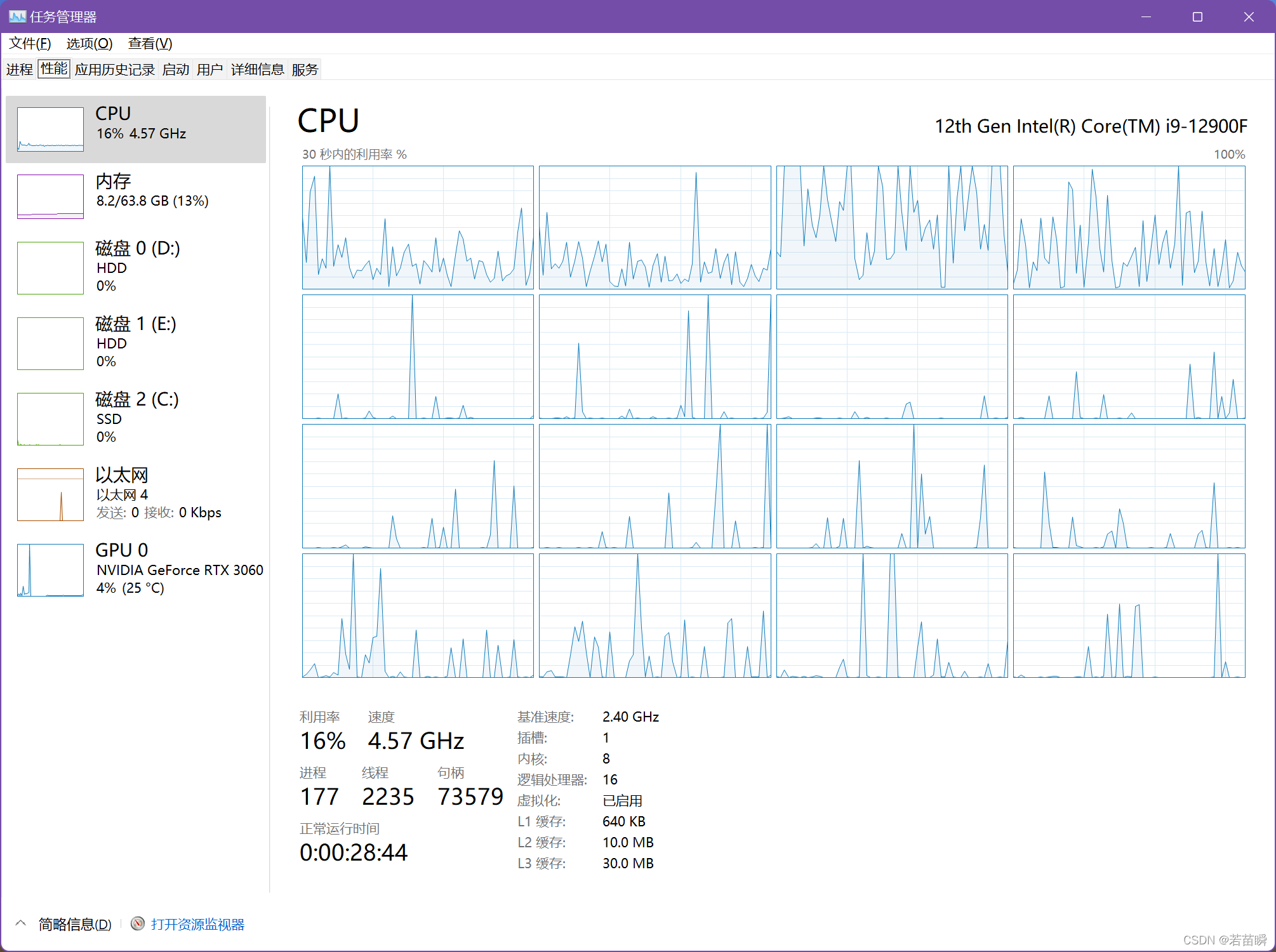
Solve the problem that intel12 generation core CPU single thread only runs on small cores
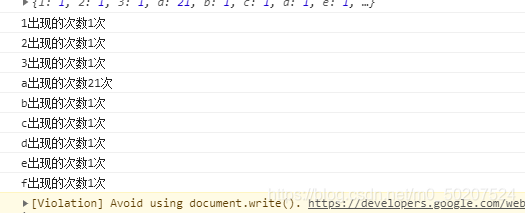
Soft music -js find the number of times that character appears in the string - Feng Hao's blog
随机推荐
Research Report on market supply and demand and strategy of Chinese table lamp industry
Oneforall installation and use
How to insert mathematical formulas in CSDN blog
MariaDB的安装与配置
Research Report on market supply and demand and strategy of China's four seasons tent industry
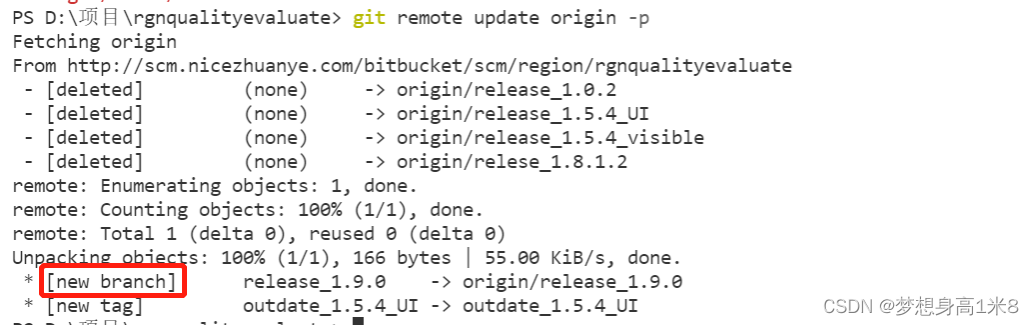
拉取分支失败,fatal: ‘origin/xxx‘ is not a commit and a branch ‘xxx‘ cannot be created from it
Hbuilder x format shortcut key settings
Chapter 1 overview of MapReduce
第7章 __consumer_offsets topic
(lightoj - 1370) Bi shoe and phi shoe (Euler function tabulation)
Spark independent cluster dynamic online and offline worker node
input 只能输入数字,限定输入
顺丰科技智慧物流校园技术挑战赛(无t4)
第5章 NameNode和SecondaryNameNode
ByteDance new programmer's growth secret: those glittering treasures mentors
力扣leetcode第 280 场周赛
Browser print margin, default / borderless, full 1 page A4
【锟斤拷】的故事:谈谈汉字编码和常用字符集
Simple records of business system migration from Oracle to opengauss database
第5章 消费者组详解