当前位置:网站首页>20220701 barbarat lemma proof
20220701 barbarat lemma proof
2022-07-04 08:48:00 【Can you eat spicy food】
(Barbalat lemma )
If differentiable function f ( t ) f(t) f(t), When t → ∞ t \rightarrow \infty t→∞ There is a finite limit , And f ˙ ( t ) \dot{f}(t) f˙(t) Uniformly continuous , So when t → ∞ t \rightarrow \infty t→∞ when , f ˙ ( t ) → 0 \dot{f}(t) \rightarrow 0 f˙(t)→0.
prove ( Reduction to absurdity ):
Suppose that t → ∞ t \rightarrow \infty t→∞ when , f ˙ ( t ) → 0 \dot{f}(t) \rightarrow 0 f˙(t)→0 Don't set up , Then there is an increasing infinite sequence { t n } n ∈ N \{t_n\}_{n\in\mathbb{N}} { tn}n∈N bring (1) When n → ∞ n \rightarrow \infty n→∞ Yes t n → ∞ t_n \rightarrow \infty tn→∞ ;(2) ∣ f ˙ ( t n ) ∣ ⩾ ϵ > 0 |\dot{f}(t_n) | \geqslant \epsilon>0 ∣f˙(tn)∣⩾ϵ>0 For all { t n } \{t_n\} { tn}.
consider f ˙ ( t ) \dot{f}(t) f˙(t) Consistent continuity of , according to ϵ − δ \epsilon-\delta ϵ−δ theory , There is a certain ϵ > 0 \epsilon>0 ϵ>0 , Such that for all n ∈ N n\in\mathbb{N} n∈N and all t ∈ R t \in \mathbb{R} t∈R, When
∣ t n − t ∣ ⩽ δ |t_n -t|\leqslant\delta ∣tn−t∣⩽δ Then there are ∣ f ˙ ( t n ) − f ˙ ( t ) ∣ ≤ ε 2 \left|\dot{f}\left(t_{n}\right)-\dot{f}(t)\right| \leq \frac{\varepsilon}{2} ∣∣∣f˙(tn)−f˙(t)∣∣∣≤2ε
therefore , For all t ∈ [ t n , t n + δ ] t\in[t_n,t_n+\delta] t∈[tn,tn+δ], And all n ∈ N n\in\mathbb{N} n∈N, Yes ∣ f ˙ ( t ) ∣ = ∣ f ˙ ( t n ) − ( f ˙ ( t n ) − f ˙ ( t ) ) ∣ ⩾ ∣ f ˙ ( t n ) ∣ − ∣ f ˙ ( t n ) − f ˙ ( t ) ∣ ⩾ ε − ε 2 = ε 2 \begin{aligned} |\dot{f}(t)| =\left|\dot{f}\left(t_{n}\right)-\left(\dot{f}\left(t_{n}\right)-\dot{f}(t)\right)\right| \geqslant \left|\dot{f}\left(t_{n}\right)\right|-\left|\dot{f}\left(t_{n}\right)-\dot{f}(t)\right| \geqslant \varepsilon-\frac{\varepsilon}{2}=\frac{\varepsilon}{2} \end{aligned} ∣f˙(t)∣=∣∣∣f˙(tn)−(f˙(tn)−f˙(t))∣∣∣⩾∣∣∣f˙(tn)∣∣∣−∣∣∣f˙(tn)−f˙(t)∣∣∣⩾ε−2ε=2ε therefore , For all n ∈ N n\in\mathbb{N} n∈N, Yes ∣ ∫ 0 t n + δ f ˙ ( t ) d t − ∫ 0 t n f ˙ ( t ) d t ∣ = ∣ ∫ t n t n + δ f ˙ ( t ) d t ∣ = ∫ t n t n + δ ∣ f ˙ ( t ) ∣ d t ≥ ε δ 2 > 0 \left|\int_{0}^{t_{n}+\delta} \dot{f}(t) d t-\int_{0}^{t_{n}} \dot{f}(t) d t\right|=\left|\int_{t_{n}}^{t_{n}+\delta} \dot{f}(t) d t\right|=\int_{t_{n}}^{t_{n}+\delta}|\dot{f}(t)| d t \geq \frac{\varepsilon \delta}{2}>0 ∣∣∣∣∣∫0tn+δf˙(t)dt−∫0tnf˙(t)dt∣∣∣∣∣=∣∣∣∣∣∫tntn+δf˙(t)dt∣∣∣∣∣=∫tntn+δ∣f˙(t)∣dt≥2εδ>0
According to the hypothesis , ∫ 0 ∞ f ˙ ( t ) d t < β \int_0^\infty \dot f(t) dt<\beta ∫0∞f˙(t)dt<β There is , therefore , When n → ∞ n\rightarrow \infty n→∞, ∣ ∫ 0 t n + δ f ˙ ( t ) d t − ∫ 0 t n f ˙ ( t ) d t ∣ → 0 \left|\int_{0}^{t_{n}+\delta} \dot{f}(t) d t-\int_{0}^{t_{n}} \dot{f}(t) d t\right| \rightarrow 0 ∣∣∣∣∣∫0tn+δf˙(t)dt−∫0tnf˙(t)dt∣∣∣∣∣→0, Conflict with the above formula , Therefore, the counter evidence method can prove , When t → ∞ t \rightarrow \infty t→∞ when , f ˙ ( t ) → 0 \dot{f}(t) \rightarrow 0 f˙(t)→0.
边栏推荐
- AcWing 244. Enigmatic cow (tree array + binary search)
- manjaro安装微信
- Flutter integrated amap_ flutter_ location
- awk从入门到入土(5)简单条件匹配
- Awk from digging into the ground to getting started (10) awk built-in functions
- How to choose solid state hard disk and mechanical hard disk in computer
- swatch
- [BSP video tutorial] stm32h7 video tutorial phase 5: MDK topic, system introduction to MDK debugging, AC5, AC6 compilers, RTE development environment and the role of various configuration items (2022-
- ES6 summary
- Technology sharing | MySQL parallel DDL
猜你喜欢
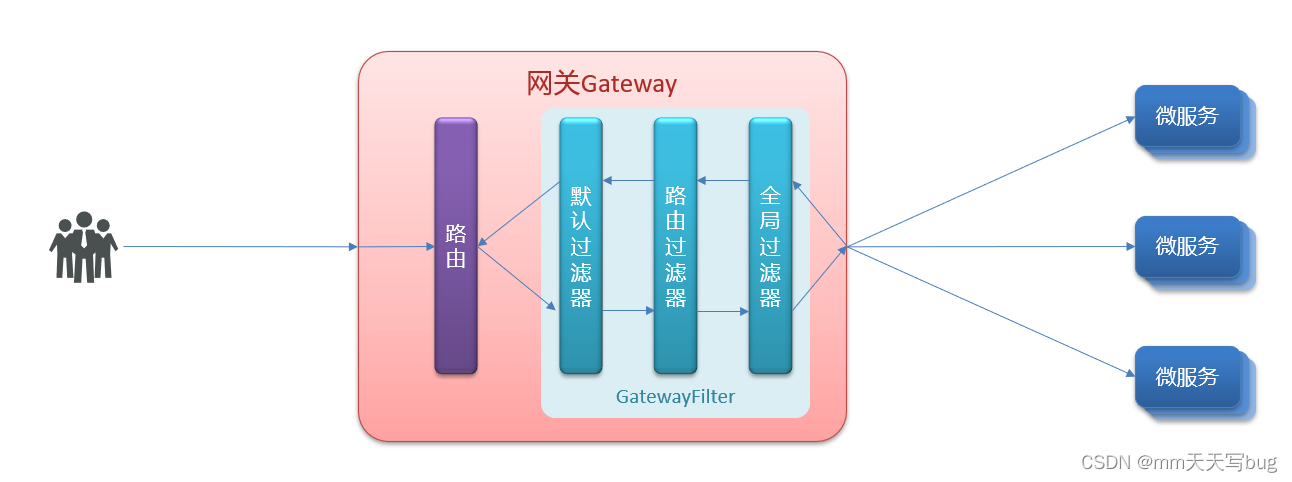
微服务入门:Gateway网关

Relationship and operation of random events
DM8 tablespace backup and recovery
根据数字显示中文汉字
What if the wireless network connection of the laptop is unavailable
Snipaste convenient screenshot software, which can be copied on the screen

How can we make a monthly income of more than 10000? We media people with low income come and have a look

HMS core helps baby bus show high-quality children's digital content to global developers

ES6 summary
Sequence model
随机推荐
C语言-入门-基础-语法-[变量,常亮,作用域](五)
Display Chinese characters according to numbers
What is inner connection and outer connection? What are the uses and benefits
Horizon sunrise X3 PI (I) first boot details
学习Nuxt.js
【无标题】转发最小二乘法
Educational Codeforces Round 119 (Rated for Div. 2)
@Role of pathvariable annotation
Awk from entry to soil (5) simple condition matching
swatch
ctfshow web255 web 256 web257
Four essential material websites for we media people to help you easily create popular models
没有Kubernetes怎么玩Dapr?
C, Numerical Recipes in C, solution of linear algebraic equations, Gauss Jordan elimination method, source code
Internal learning
From scratch, use Jenkins to build and publish pipeline pipeline project
C语言-入门-基础-语法-[主函数,头文件](二)
Awk from getting started to digging in (4) user defined variables
Newh3c - routing protocol (RIP, OSPF)
Bishi blog (13) -- oral arithmetic test app