当前位置:网站首页>Cartoon: looking for the k-th element of an unordered array (Revised)
Cartoon: looking for the k-th element of an unordered array (Revised)
2022-07-05 17:28:00 【Small ash】
This article modifies two details :
1. Method 2 , Insert the array A The condition is to traverse to the element “ Greater than ” Array A The smallest element of , Instead of ” Less than ”.
2. Method 3 , node 24 When the little top pile sinks , It should be connected to nodes 17 In exchange for , Not with nodes 20 In exchange for .
Thank you for your correction .
————— the second day —————
What does the title mean ? For example, given an unordered array as follows :
If k=6, That is to look for the second 6 Big elements , Which element is this ?
obviously , The largest element in the array is 24, The second largest element is 20, The third element is 17 ...... The first 6 The big element is 9.
Method 1 : Sequencing
This is the easiest way to think of , First, sort the unordered array from large to small , After the order k Elements , Nature is the number one in the array k Big element .
Method 2 : Insertion method
Maintain a length of k Array of A Ordered array of , Used to store known k A larger element .
Next, traverse the original array , Every time you traverse to an element , And an array A Compared with the smallest element in , If it is less than or equal to the array A The smallest element of , Continue traversing ; If it's larger than the array A The smallest element of , Then insert it into the array A in , And put the smallest element ever “ Squeeze out ”.
such as k=3, Let's start with the leftmost 7,5,15 Put three numbers in an array in order A among , Represents the current three largest numbers .
Now , Traversing 3, because 3<5, Continue traversing .
Next we go through to 17, because 17>5, Insert into array A The right place , Similar to insert sort , And put the smallest element 5“ Squeeze out ”.
Continue traversing the original array , All the way to the last element of the array ......
Final , Array A The elements stored in are 24,20,17, Represents the largest... In the entire array 3 Elements . Now the array A The smallest element in 17 That's what we're looking for k Big element .
————————————
What is a binary heap ? If you don't know much about it, you can read this article first : comic : What is a binary heap ?( Revised version )
In short , A binary heap is a special kind of complete binary tree , It consists of two forms: large top pile and small top pile .
Among them, the characteristics of small top reactor , Every parent node is less than or equal to its own child node . To solve this algorithmic problem , We can use Small cap pile Characteristics of .
Method 3 : Small top pile method
Maintain a capacity of k The little top pile , Heap k Nodes represent The biggest... At present k Elements , And the top of the pile is obviously this k Of the elements minimum value .
Iterate over the original array , One element per iteration , Compared to the top of the heap , If the current element is less than or equal to the top of the heap , Then continue to traverse ; If the element is larger than the top of the heap , Put the current element at the top of the heap , And adjust the fork stack ( Sinking operation ).
After the traversal is over , The top of the heap is an array Maximum k The minimum of the elements , That is to say The first k Big element .
hypothesis k=5, The specific implementation steps are as follows :
1. Put the front of the array k Elements are built into a heap .
2. Continue traversing the array , Compared to the top of the heap , If it is less than or equal to the top of the heap , Then continue to traverse ; If it's larger than the top of the pile , Replace the top element and adjust the heap .
Traverse to element 2, because 2<3, So continue traversing .
Traverse to element 20, because 20>3,20 Instead of the top position , And adjust the pile .
Traverse to element 24, because 24>5,24 Instead of the top position , And adjust the pile .
And so on , We traverse the elements one by one , When traversing to the last element 8 When , The situation of small top reactor is as follows :
3. The top of the heap at this time , It's the minimum in the heap , That is, the first in the array k Big element .
What is the time complexity of this method ?
1. The time complexity of building a heap is O(k)
2. The time complexity of traversing the remaining array is O(n-k)
3. The time complexity of each heap adjustment is O(logk)
among 2 and 3 It's a nested relationship ,1 and 2,3 It's juxtaposition , So the total worst-case time complexity is O((n-k)logk + k). When k Far less than n Under the circumstances , It can also be approximately regarded as O(nlogk).
What is the spatial complexity of this method ?
Just now, we showed the binary heap separately in the detailed steps , To make it easy to understand . But if it's allowed to change the original array , We can put the front of the array k Elements “ In situ exchange ” To build a binary reactor , This eliminates the need to open up additional storage space .
therefore , The spatial complexity of the method is O(1).
/**
* Search for the first k Big elements
* @param array The heap to be adjusted
* @param k What is the largest
*/
public static int findNumberK(int[] array, int k){
//1. Before use k Elements to build a small top heap
buildHeap(array, k);
//2. Continue traversing the array , Compared to the top of the heap
for(int i=k; i<array.length;i++){
if(array[i] > array[0]){
array[0] = array[i];
downAdjust(array, 0, k);
}
}
//3. Return to the top of heap element
return array[0];
}
/**
* Build heap
* @param array The heap to be adjusted
* @param length The effective size of the heap
*/
private static void buildHeap(int[] array, int length) {
// Start with the last non leaf node , Adjust in turn
for (int i = (length-2)/2; i >= 0; i--) {
downAdjust(array, i, length);
}
}
/**
* Sinking adjustment
* @param array The heap to be adjusted
* @param index The node to sink
* @param length The effective size of the heap
*/
private static void downAdjust(int[] array, int index, int length) {
// temp Save the parent node value , For the final assignment
int temp = array[index];
int childIndex = 2 * index + 1;
while (childIndex < length) {
// If there is a right child , And the right child is less than the left child , Then locate the right child
if (childIndex + 1 < length && array[childIndex + 1] < array[childIndex]) {
childIndex++;
}
// If the parent node is less than the value of any child , Direct jump out
if (temp <= array[childIndex])
break;
// There's no real exchange , One way assignment is enough
array[index] = array[childIndex];
index = childIndex;
childIndex = 2 * childIndex + 1;
}
array[index] = temp;
}
public static void main(String[] args) {
int[] array = new int[] {7,5,15,3,17,2,20,24,1,9,12,8};
System.out.println(findNumberK(array, 5));
}Method four : Divide and conquer method
We all know about quick sort , Quicksort uses divide and conquer , Divide the array into larger and smaller parts each time .
We're looking for number one k When it comes to big elements , You can also use this idea , With a certain element A Benchmarking , Make greater than A Are swapped to the left of the array , Less than A All of the elements are swapped to the right of the array .
For example, we choose elements 7 As a benchmark , Divide the array into larger ones on the left , Two smaller areas on the right , The exchange results are as follows :
Including elements 7 The larger elements inside are 8 individual , But our k=5, Obviously there are too many larger elements . So we continue to divide and conquer the larger element regions , This time with elements 12 Bit reference :
thus , Including elements 12 The larger elements inside are 5 individual , Coincides with k equal . therefore , Reference elements 12 That's what we're looking for .
This is the general idea of divide and conquer , The time complexity of this method is even better than that of the small top pile method , You can achieve O(n). Interested partners can try to use the code to achieve it .
Found this article useful , Please give it a good look , I think it's very useful , Please forward it to your friends .
边栏推荐
- MYSQL group by 有哪些注意事项
- Understand the usage of functions and methods in go language
- 【Web攻防】WAF检测技术图谱
- Judge whether a string is a full letter sentence
- Use JDBC technology and MySQL database management system to realize the function of course management, including adding, modifying, querying and deleting course information.
- 哈趣K1和哈趣H1哪个性价比更高?谁更值得入手?
- 张平安:加快云上数字创新,共建产业智慧生态
- How MySQL uses JSON_ Extract() takes JSON value
- Thoughtworks 全球CTO:按需求构建架构,过度工程只会“劳民伤财”
- CMake教程Step6(添加自定义命令和生成文件)
猜你喜欢
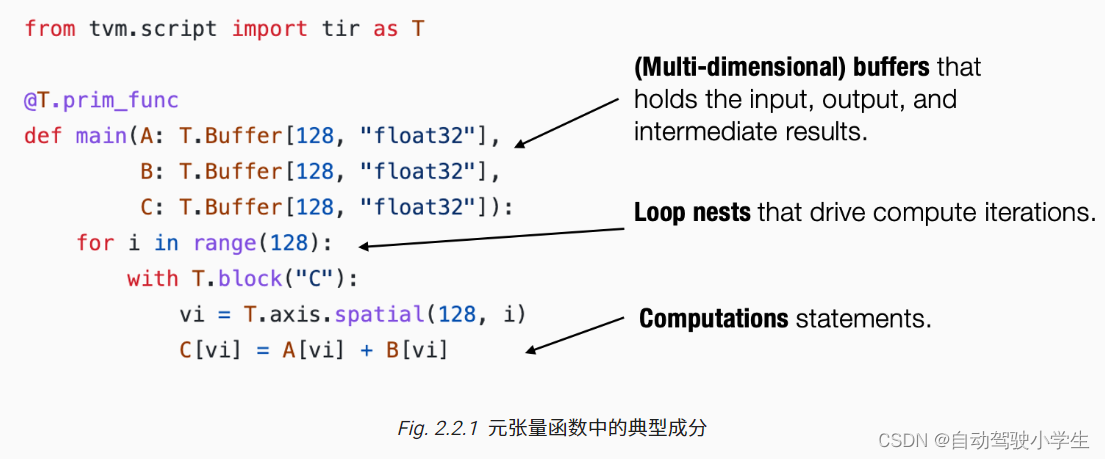
Machine learning compilation lesson 2: tensor program abstraction
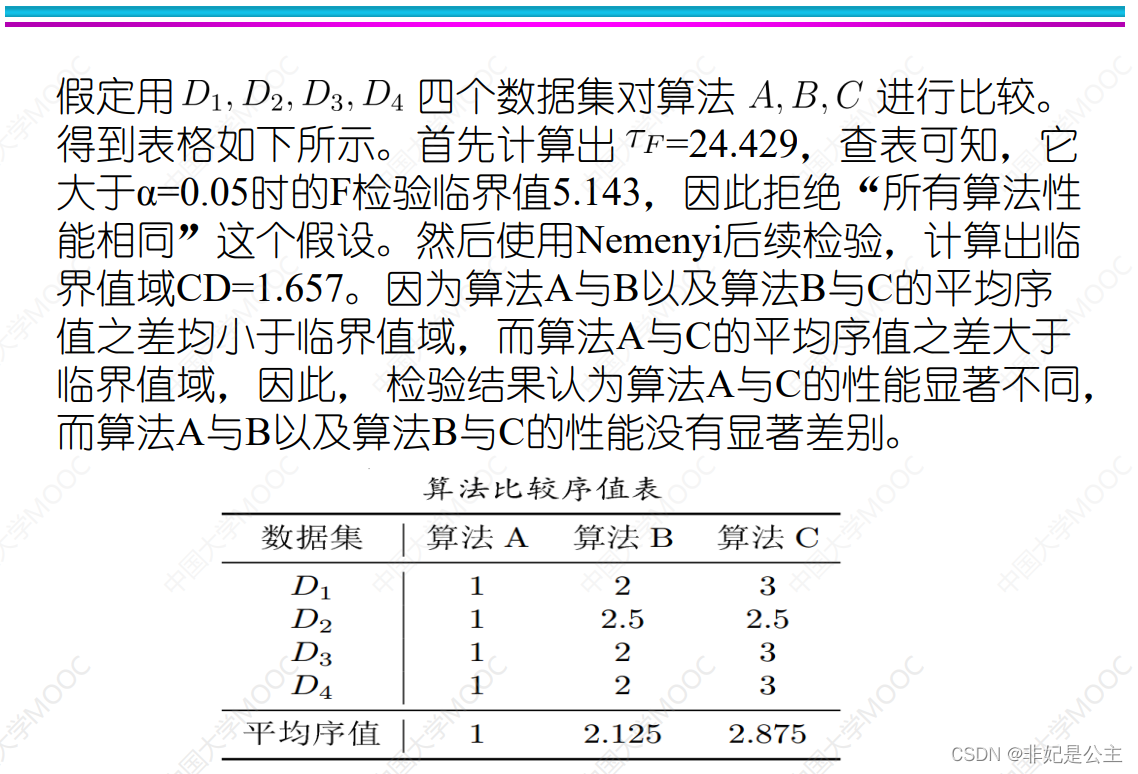
机器学习02:模型评估
First day of learning C language
![[Web attack and Defense] WAF detection technology map](/img/7c/60a25764950668ae454b2bc08fe57e.png)
[Web attack and Defense] WAF detection technology map
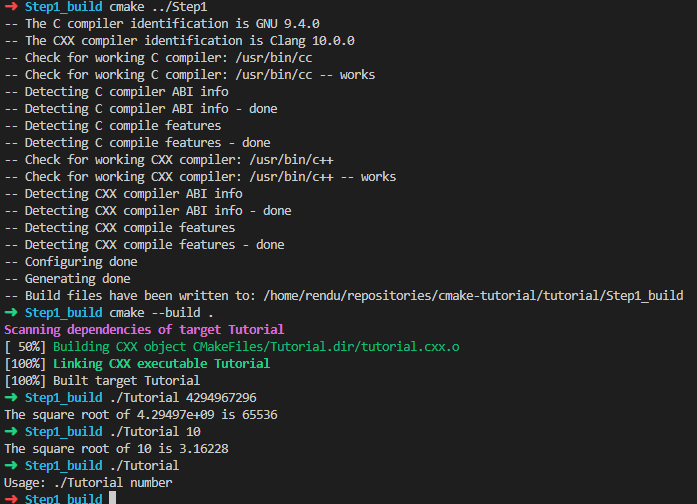
CMake教程Step1(基本起点)
【jmeter】jmeter脚本高级写法:接口自动化脚本内全部为变量,参数(参数可jenkins配置),函数等实现完整业务流测试
Wsl2.0 installation
CVPR 2022最佳学生论文:单张图像估计物体在3D空间中的位姿估计
Judge whether a string is a full letter sentence

Embedded UC (UNIX System Advanced Programming) -1
随机推荐
Design of electronic clock based on 51 single chip microcomputer
Little knowledge about C language (array and string)
普通程序员看代码,顶级程序员看趋势
VBA驱动SAP GUI实现办公自动化(二):判断元素是否存在
CMake教程Step3(添加库的使用要求)
项目引入jar从私服Nexus 拉去遇到的一个问题
Machine learning 02: model evaluation
Precision epidemic prevention has a "sharp weapon" | smart core helps digital sentinels escort the resumption of the city
Kafaka技术第一课
Force deduction solution summary 1200 minimum absolute difference
Example tutorial of SQL deduplication
Tita performance treasure: how to prepare for the mid year examination?
深入理解Redis内存淘汰策略
easyNmon使用汇总
漫画:有趣的【海盗】问题
一口气读懂 IT发展史
[Web attack and Defense] WAF detection technology map
Allusions of King Xuan of Qi Dynasty
CVPR 2022最佳学生论文:单张图像估计物体在3D空间中的位姿估计
About JSON parsing function JSON in MySQL_ EXTRACT