当前位置:网站首页>In this indifferent world, light crying
In this indifferent world, light crying
2022-07-05 05:31:00 【solemntee】
For a substring a b c abc abc, It is easy to find the front i i i Number of bit sequences , Write it down as d p [ i ] [ a ] [ b ] [ c ] dp[i][a][b][c] dp[i][a][b][c]
For a substring a b ab ab, It is easy to find the front i i i Number of bit sequences , Write it down as d p [ i ] [ a ] [ b ] dp[i][a][b] dp[i][a][b]
For a substring a a a, It is easy to find the front i i i Number of bit sequences , Write it down as d p [ i ] [ a ] dp[i][a] dp[i][a]
First consider d p [ r ] [ a ] [ b ] [ c ] − d p [ l − 1 ] [ a ] [ b ] [ c ] dp[r][a][b][c]-dp[l-1][a][b][c] dp[r][a][b][c]−dp[l−1][a][b][c], The number of sequences obtained in this way is two more .
The first category is b c bc bc stay [ l , r ] [l,r] [l,r] Inside , a a a stay [ 1 , l − 1 ] [1,l-1] [1,l−1] Inside
[ l , r ] [l,r] [l,r] Internal b c bc bc The number of sequences is d p [ r ] [ a ] [ b ] − d p [ l − 1 ] [ a ] [ b ] − ( d p [ r ] [ b ] − d p [ l − 1 ] [ b ] ) × d p [ l − 1 ] [ a ] dp[r][a][b]-dp[l-1][a][b]-(dp[r][b]-dp[l-1][b])\times dp[l-1][a] dp[r][a][b]−dp[l−1][a][b]−(dp[r][b]−dp[l−1][b])×dp[l−1][a]
The number of the first category is
( d p [ r ] [ a ] [ b ] − d p [ l − 1 ] [ a ] [ b ] − ( d p [ r ] [ b ] − d p [ l − 1 ] [ b ] ) × d p [ l − 1 ] [ a ] ) × d p [ l − 1 ] [ a ] (dp[r][a][b]-dp[l-1][a][b]-(dp[r][b]-dp[l-1][b])\times dp[l-1][a])\times dp[l-1][a] (dp[r][a][b]−dp[l−1][a][b]−(dp[r][b]−dp[l−1][b])×dp[l−1][a])×dp[l−1][a]
The second type is c c c stay [ l , r ] [l,r] [l,r] Inside , a b ab ab stay [ 1 , l − 1 ] [1,l-1] [1,l−1] Inside
The number of
( d p [ c ] [ r ] − d p [ c ] [ l − 1 ] ) × d p [ a ] [ b ] [ l − 1 ] (dp[c][r]-dp[c][l-1])\times dp[a][b][l-1] (dp[c][r]−dp[c][l−1])×dp[a][b][l−1]
The final answer is
d p [ r ] [ a ] [ b ] [ c ] − d p [ l − 1 ] [ a ] [ b ] [ c ] − ( ( d p [ r ] [ a ] [ b ] − d p [ l − 1 ] [ a ] [ b ] − ( d p [ r ] [ b ] − d p [ l − 1 ] [ b ] ) × d p [ l − 1 ] [ a ] ) × d p [ l − 1 ] [ a ] ) − ( ( d p [ c ] [ r ] − d p [ c ] [ l − 1 ] ) × d p [ a ] [ b ] [ l − 1 ] ) dp[r][a][b][c]-dp[l-1][a][b][c]-((dp[r][a][b]-dp[l-1][a][b]-(dp[r][b]-dp[l-1][b])\times dp[l-1][a])\times dp[l-1][a])-((dp[c][r]-dp[c][l-1])\times dp[a][b][l-1]) dp[r][a][b][c]−dp[l−1][a][b][c]−((dp[r][a][b]−dp[l−1][a][b]−(dp[r][b]−dp[l−1][b])×dp[l−1][a])×dp[l−1][a])−((dp[c][r]−dp[c][l−1])×dp[a][b][l−1])
be aware d p [ n ] [ a ] [ b ] [ c ] dp[n][a][b][c] dp[n][a][b][c] It can be used O ( n × 2 6 2 ) O(n\times26^2) O(n×262) Complexity of complete ( Use the end point to optimize one dimension ), We can ask each group of answers offline O ( n × 2 6 2 + q × C ) O(n\times26^2+q\times C) O(n×262+q×C) complete
#include<bits/stdc++.h>
#define ll long long
using namespace std;
struct node
{
int pos;
ll val;
};
struct query
{
int len,pos,id,f,a,b,c;
};
vector<query>v[80005];
ll Q[500005][8];
ll presum[26][26][26];
ll dp[26][26][3];
char ss[4];
char s[80005];
int main()
{
int n,q;
scanf("%d%d",&n,&q);
scanf("%s",s+1);
for(int i=1;i<=q;i++)
{
// printf("q=%d\n",q);
int l,r;
scanf("%d%d",&l,&r);
scanf("%s",ss+1);
// printf("q=%d\n",q);
/// The length is 3 And
v[l-1].push_back({
3,5,i,-1,ss[1]-'a',ss[2]-'a',ss[3]-'a'});
v[r].push_back({
3,5,i,1,ss[1]-'a',ss[2]-'a',ss[3]-'a'});
/// front 2 Back 1
v[l-1].push_back({
2,1,i,1,ss[1]-'a',ss[2]-'a',ss[3]-'a'});
v[r].push_back({
1,2,i,1,ss[3]-'a',0,0});
v[l-1].push_back({
1,2,i,-1,ss[3]-'a',0,0});
/// front 1 Back 2
v[l-1].push_back({
1,3,i,1,ss[1]-'a',ss[2]-'a',ss[3]-'a'});
v[r].push_back({
2,4,i,1,ss[2]-'a',ss[3]-'a',0});
v[l-1].push_back({
2,4,i,-1,ss[2]-'a',ss[3]-'a',0});
v[r].push_back({
1,6,i,1,ss[3]-'a',0,0});
v[l-1].push_back({
1,6,i,-1,ss[3]-'a',0,0});
v[l-1].push_back({
1,7,i,1,ss[2]-'a',0,0});
// printf("q=%d\n",q);
}
for(int j=0;j<=25;j++)
for(int k=0;k<=25;k++)dp[j][k][0]=1;
for(int i=1;i<=n;i++)
{
for(int j=0;j<=25;j++)
for(int k=0;k<=25;k++)
{
presum[j][k][s[i]-'a']+=dp[j][k][2];
if(s[i]-'a'==k)dp[j][k][2]+=dp[j][k][1];
if(s[i]-'a'==j)dp[j][k][1]+=dp[j][k][0];
}
for(auto [len,pos,id,f,a,b,c]:v[i])
{
// printf("len=%d pos=%d id=%d f=%d a=%d b=%d c=%d \n",len,pos,id,f,a,b,c);
if(len==1)Q[id][pos]+=f*dp[a][0][1];
else if(len==2)Q[id][pos]+=f*dp[a][b][2];
else if(len==3)Q[id][pos]+=f*presum[a][b][c];
}
}
for(int i=1;i<=q;i++)printf("%lld\n",Q[i][5]-Q[i][1]*Q[i][2]-Q[i][3]*(Q[i][4]-Q[i][6]*Q[i][7]));
return 0;
}
边栏推荐
- A misunderstanding about the console window
- kubeadm系列-01-preflight究竟有多少check
- Remote upgrade afraid of cutting beard? Explain FOTA safety upgrade in detail
- Sword finger offer 05 Replace spaces
- 每日一题-无重复字符的最长子串
- 挂起等待锁 vs 自旋锁(两者的使用场合)
- Zheng Qing 21 ACM is fun. (3) part of the problem solution and summary
- 剑指 Offer 09. 用两个栈实现队列
- [to be continued] [depth first search] 547 Number of provinces
- 软件测试 -- 0 序
猜你喜欢
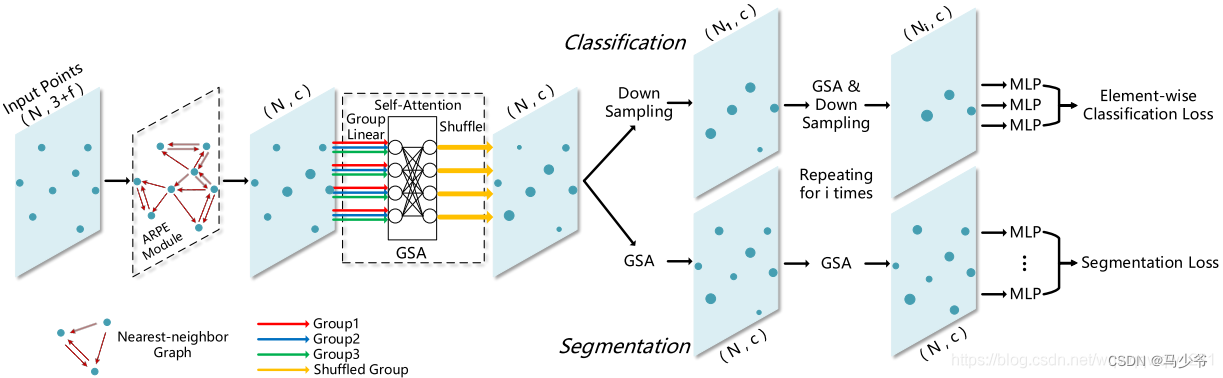
Improvement of pointnet++

The present is a gift from heaven -- a film review of the journey of the soul

第六章 数据流建模—课后习题
挂起等待锁 vs 自旋锁(两者的使用场合)

【实战技能】非技术背景经理的技术管理
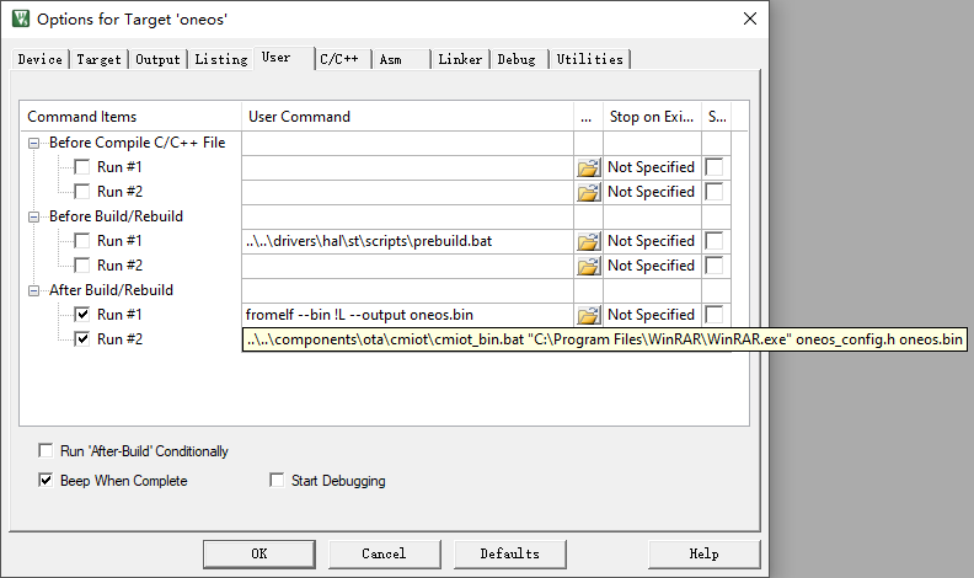
Remote upgrade afraid of cutting beard? Explain FOTA safety upgrade in detail
Sword finger offer 53 - I. find the number I in the sorted array
剑指 Offer 05. 替换空格

Sword finger offer 53 - ii Missing numbers from 0 to n-1
YOLOv5-Shufflenetv2
随机推荐
Warning using room database: schema export directory is not provided to the annotation processor so we cannot export
Yolov5 adds attention mechanism
Maximum number of "balloons"
Download xftp7 and xshell7 (official website)
Find a good teaching video for Solon framework test (Solon, lightweight application development framework)
SSH password free login settings and use scripts to SSH login and execute instructions
[allocation problem] 455 Distribute cookies
[to be continued] [UE4 notes] L2 interface introduction
服务熔断 Hystrix
After setting up the database and website When you open the app for testing, it shows that the server is being maintained
发现一个很好的 Solon 框架试手的教学视频(Solon,轻量级应用开发框架)
Pointnet++的改进
Solon Logging 插件的添加器级别控制和日志器的级别控制
搭建完数据库和网站后.打开app测试时候显示服务器正在维护.
Improvement of pointnet++
C language Essay 1
Corridor and bridge distribution (csp-s-2021-t1) popular problem solution
Csp-j-2020-excellent split multiple solutions
kubeadm系列-00-overview
Talking about JVM (frequent interview)