当前位置:网站首页>LeetCode_哈希表_困难_149. 直线上最多的点数
LeetCode_哈希表_困难_149. 直线上最多的点数
2022-07-05 20:57:00 【小城老街】
1.题目
给你一个数组 points ,其中 points[i] = [xi, yi] 表示 X-Y 平面上的一个点。求最多有多少个点在同一条直线上。
示例 1: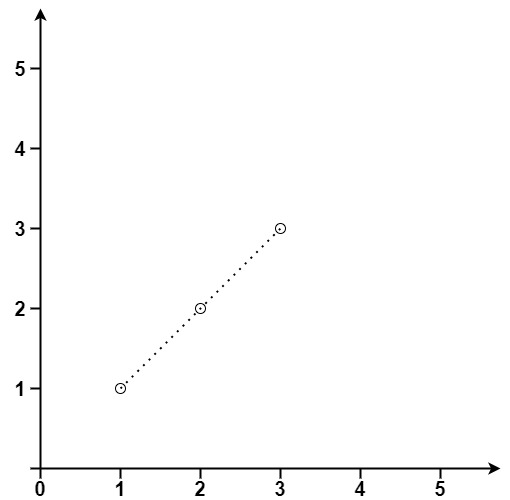
输入:points = [[1,1],[2,2],[3,3]]
输出:3
示例 2: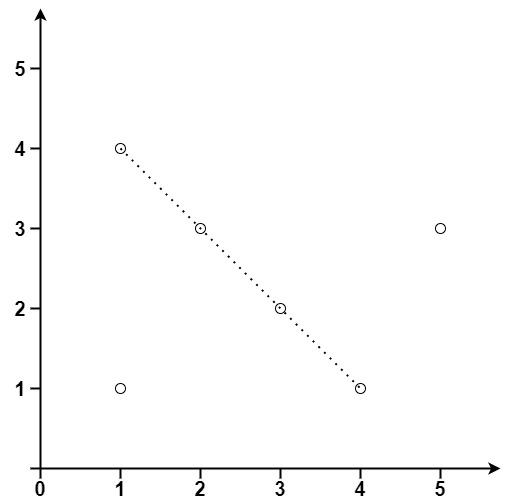
输入:points = [[1,1],[3,2],[5,3],[4,1],[2,3],[1,4]]
输出:4
提示:
1 <= points.length <= 300
points[i].length == 2
-104 <= xi, yi <= 104
points 中的所有点互不相同
来源:力扣(LeetCode)
链接:https://leetcode.cn/problems/max-points-on-a-line
2.思路
(1)暴力穷举法
暴力穷举法比较容易想到,由于在平面中两个不同的点可以确定一条直线,所以可以先穷举两个点的组合(即确定一条直线),然后再检查其余的点是否在该直线上。
(2)暴力穷举法改进_哈希表
思路参考该用户题解。
由于两点确定一条直线,所以可以先穷举所有可能出现的直线斜率(即枚举所有的点对),然后使用哈希表统计所有斜率对应的直线上点的数量(即键/值 = 斜率/点的数量),那么所有值中的最大值即为答案。
3.代码实现(Java)
//思路1————暴力穷举法
class Solution {
public int maxPoints(int[][] points) {
//最多有 cnt 个点在同一条直线上,由于 1 <= points.length <= 300,所以 cnt 的初始值为 1
int cnt = 1;
int n = points.length;
for (int i = 0; i < n; i++) {
int[] p1 = points[i];
for (int j = i + 1; j < n; j++) {
int[] p2 = points[j];
//p1 与 p2 一定在一条直线上,故至少有 2 个点在同一条直线上
int tmpCnt = 2;
for (int k = j + 1; k < n; k++) {
int[] p3 = points[k];
/* (1) 如果 (p1[1] - p2[1])/(p1[0] - p2[0]) == (p2[1] - p3[1])/(p2[0] - p3[0]) 那么 p1、p2、p3 这 3 个点在同一条直线上 (2) 为了防止整数相除时有精度损失,所以将上面的等式改为: (p1[0] - p2[0]) * (p2[1] - p3[1]) = (p1[1] - p2[1]) * (p2[0] - p3[0]) */
if ((p1[0] - p2[0]) * (p2[1] - p3[1]) == (p1[1] - p2[1]) * (p2[0] - p3[0])) {
tmpCnt++;
}
}
cnt = Math.max(tmpCnt, cnt);
}
}
return cnt;
}
}
//思路2————暴力穷举法改进_哈希表
class Solution {
public int maxPoints(int[][] ps) {
int n = ps.length;
int cnt = 1;
for (int i = 0; i < n; i++) {
Map<String, Integer> hashMap = new HashMap<>();
// 由当前点 i 发出的直线所经过的最多点数量
int max = 0;
for (int j = i + 1; j < n; j++) {
int x1 = ps[i][0], y1 = ps[i][1], x2 = ps[j][0], y2 = ps[j][1];
int a = x1 - x2, b = y1 - y2;
int k = gcd(a, b);
String key = (a / k) + "_" + (b / k);
hashMap.put(key, hashMap.getOrDefault(key, 0) + 1);
max = Math.max(max, hashMap.get(key));
}
cnt = Math.max(cnt, max + 1);
}
return cnt;
}
//求 a 和 b 的最大公约数
public int gcd(int a, int b) {
return b == 0 ? a : gcd(b, a % b);
}
}
边栏推荐
- haas506 2.0开发教程 - 阿里云ota - pac 固件升级(仅支持2.2以上版本)
- MySQL ifnull usage function
- ViewRootImpl和WindowManagerService笔记
- 当Steam教育进入个性化信息技术课程
- 挖财商学院给的证券账户安全吗?可以开户吗?
- Interpreting the daily application functions of cooperative robots
- Chemical properties and application instructions of prosci Lag3 antibody
- Hdu2377bus pass (build more complex diagram +spfa)
- Pytorch实战——MNIST数据集手写数字识别
- Abnova CRISPR spcas9 polyclonal antibody protocol
猜你喜欢
Abnova maxpab mouse derived polyclonal antibody solution
教你自己训练的pytorch模型转caffe(一)
Abnova CRISPR spcas9 polyclonal antibody protocol
解读协作型机器人的日常应用功能
![最长摆动序列[贪心练习]](/img/e1/70dc21b924232c7e5e3da023a4bed2.png)
最长摆动序列[贪心练习]

Abnova丨CRISPR SpCas9 多克隆抗体方案
Norgen AAV提取剂盒说明书(含特色)
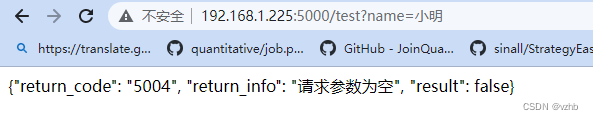
Écrire une interface basée sur flask
显示器要申请BS 476-7 怎么送样?跟显示屏一样吗??

Duchefa MS medium contains vitamin instructions
随机推荐
解读协作型机器人的日常应用功能
shell编程100例
Clear app data and get Icon
Material Design组件 - 使用BottomSheet展现扩展内容(二)
Interpreting the daily application functions of cooperative robots
Mathematical analysis_ Notes_ Chapter 9: curve integral and surface integral
手机开户股票开户安全吗?我家比较偏远,有更好的开户途径么?
Abnova丨DNA 标记高质量控制测试方案
Comparison table of foreign lead American abbreviations
判断横竖屏的最佳实现
Duchefa p1001 plant agar Chinese and English instructions
ClickHouse 复制粘贴多行sql语句报错
当用户登录,经常会有实时的下拉框,例如,输入邮箱,将会@qq.com,@163.com,@sohu.com
Matplotlib drawing retouching (how to form high-quality drawings, such as how to set fonts, etc.)
基于flask写一个接口
Abnova丨 MaxPab 小鼠源多克隆抗体解决方案
Which is the best online collaboration product? Microsoft loop, notion, flowus
phpstudy小皮的mysql点击启动后迅速闪退,已解决
中国管理科学研究院凝聚行业专家,傅强荣获智库专家“十佳青年”称号
Duchefa丨低熔点琼脂糖 PPC中英文说明书