当前位置:网站首页>二叉搜索树(基操篇)
二叉搜索树(基操篇)
2022-07-07 14:17:00 【Joey Liao】
二叉搜索树(特性篇)介绍了 BST 的基本特性,还利用二叉搜索树「中序遍历有序」的特性来解决了几道题目,本文来实现 BST 的基础操作:判断 BST 的合法性、增、删、查。其中「删」和「判断合法性」略微复杂。
BST 的基础操作主要依赖「左小右大」的特性,可以在二叉树中做类似二分搜索的操作,寻找一个元素的效率很高。比如下面这就是一棵合法的二叉树: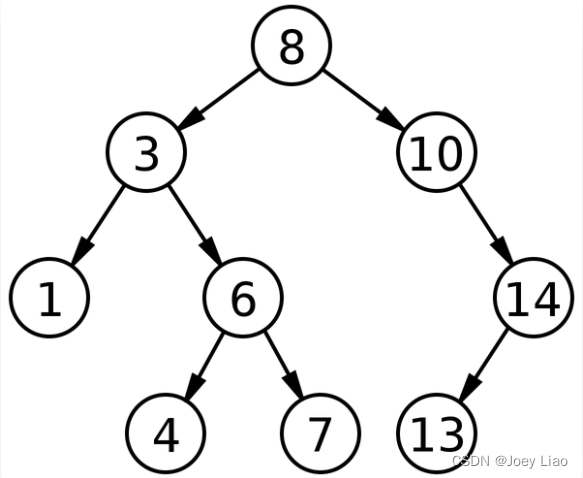
对于 BST 相关的问题,你可能会经常看到类似下面这样的代码逻辑:
void BST(TreeNode root, int target) {
if (root.val == target)
// 找到目标,做点什么
if (root.val < target)
BST(root.right, target);
if (root.val > target)
BST(root.left, target);
}
一、判断 BST 的合法性
力扣第 98 题「 验证二叉搜索树」就是让你判断输入的 BST 是否合法。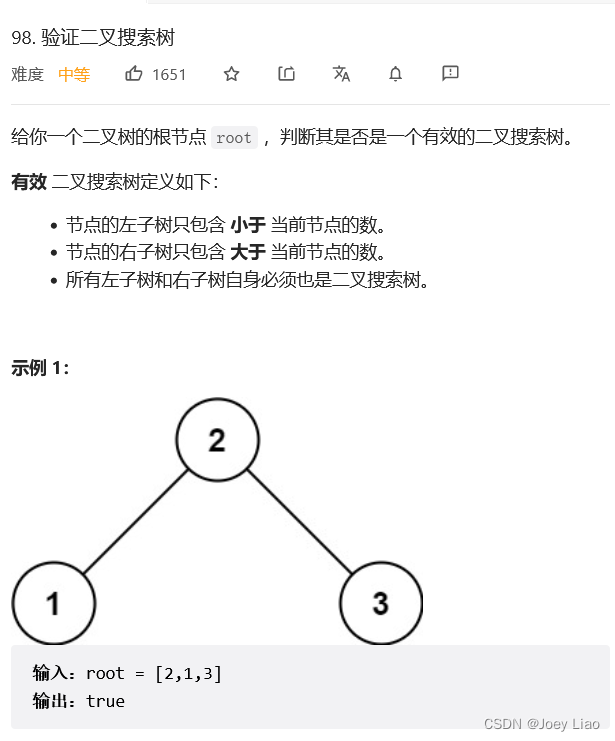 需要注意的一点是不能直接比较自己和左右孩子,但这种想法是错误的,BST 的每个节点应该要小于右边子树的所有节点,正确代码如下:
需要注意的一点是不能直接比较自己和左右孩子,但这种想法是错误的,BST 的每个节点应该要小于右边子树的所有节点,正确代码如下:
class Solution {
public boolean isValidBST(TreeNode root) {
return traverse(root,null,null);
}
public boolean traverse(TreeNode root,TreeNode minNode,TreeNode maxNode){
if(root==null) return true;
if(minNode!=null&&minNode.val>=root.val) return false;
if(maxNode!=null&&maxNode.val<=root.val) return false;
return traverse(root.left,minNode,root)&&traverse(root.right,root,maxNode);
}
}
在 BST 中搜索元素
力扣第 700 题「 二叉搜索树中的搜索」 很简单的二分思想:
很简单的二分思想:
/** * Definition for a binary tree node. * public class TreeNode { * int val; * TreeNode left; * TreeNode right; * TreeNode() {} * TreeNode(int val) { this.val = val; } * TreeNode(int val, TreeNode left, TreeNode right) { * this.val = val; * this.left = left; * this.right = right; * } * } */
class Solution {
public TreeNode searchBST(TreeNode root, int val) {
if(root==null) return null;
if(val<root.val){
return searchBST(root.left,val);
}else if(val>root.val){
return searchBST(root.right,val);
}
return root;
}
}
在 BST 中插入一个数
对数据结构的操作无非遍历 + 访问,遍历就是「找」,访问就是「改」。具体到这个问题,插入一个数,就是先找到插入位置,然后进行插入操作。
上一个问题,我们总结了 BST 中的遍历框架,就是「找」的问题。直接套框架,加上「改」的操作即可。一旦涉及「改」,就类似二叉树的构造问题,函数要返回 TreeNode 类型,并且要对递归调用的返回值进行接收。
TreeNode insertIntoBST(TreeNode root, int val) {
// 找到空位置插入新节点
if (root == null) return new TreeNode(val);
// if (root.val == val)
// BST 中一般不会插入已存在元素
if (root.val < val)
root.right = insertIntoBST(root.right, val);
if (root.val > val)
root.left = insertIntoBST(root.left, val);
return root;
}
在 BST 中删除一个数
这个问题稍微复杂,跟插入操作类似,先「找」再「改」,先把框架写出来再说
TreeNode deleteNode(TreeNode root, int key) {
if (root.val == key) {
// 找到啦,进行删除
} else if (root.val > key) {
// 去左子树找
root.left = deleteNode(root.left, key);
} else if (root.val < key) {
// 去右子树找
root.right = deleteNode(root.right, key);
}
return root;
}
找到目标节点了,比方说是节点 A,如何删除这个节点,这是难点。因为删除节点的同时不能破坏 BST 的性质。有三种情况,用图片来说明。
情况1: A 恰好是末端节点,两个子节点都为空,那么它可以当场去世了。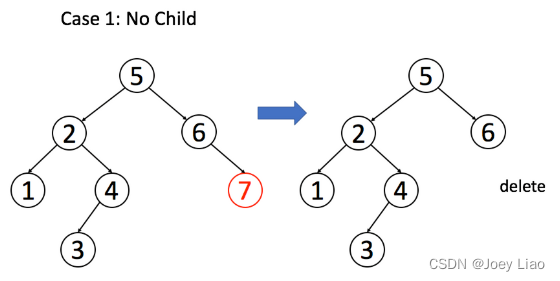
if (root.left == null && root.right == null)
return null;
情况 2:A 只有一个非空子节点,那么它要让这个孩子接替自己的位置。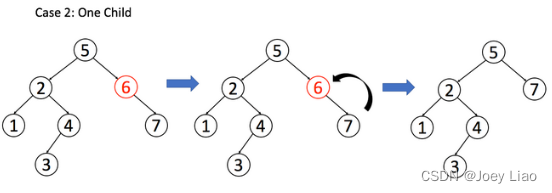
if(root.left==null) return root.right;
if(root.right==null) return root.left;
情况 3:A 有两个子节点,麻烦了,为了不破坏 BST 的性质,A 必须找到左子树中最大的那个节点,或者右子树中最小的那个节点来接替自己。我们以第二种方式讲解。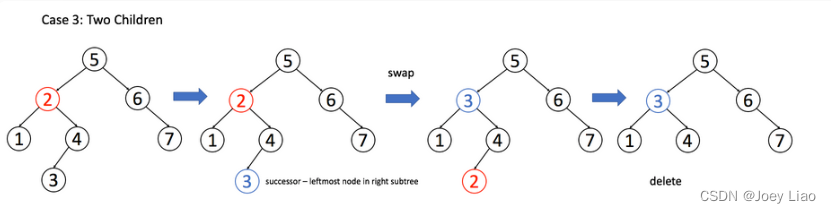
if(root.left!=null&&root.right!=null){
TreeNode minNode=getMin(root.right);
root.val=minNode.val;
root.right=deleteNode(root.right,minNode.val);
}
三种情况分析完毕,填入框架,简化一下代码
TreeNode deleteNode(TreeNode root, int key) {
if (root == null) return null;
if (root.val == key) {
// 这两个 if 把情况 1 和 2 都正确处理了
if (root.left == null) return root.right;
if (root.right == null) return root.left;
// 处理情况 3
// 获得右子树最小的节点
TreeNode minNode = getMin(root.right);
// 删除右子树最小的节点
root.right = deleteNode(root.right, minNode.val);
// 用右子树最小的节点替换 root 节点
minNode.left = root.left;
minNode.right = root.right;
root = minNode;
} else if (root.val > key) {
root.left = deleteNode(root.left, key);
} else if (root.val < key) {
root.right = deleteNode(root.right, key);
}
return root;
}
TreeNode getMin(TreeNode node) {
// BST 最左边的就是最小的
while (node.left != null) node = node.left;
return node;
}
这样,删除操作就完成了。注意一下,上述代码在处理情况 3 时通过一系列略微复杂的链表操作交换 root 和 minNode 两个节点:
// 处理情况 3
// 获得右子树最小的节点
TreeNode minNode = getMin(root.right);
// 删除右子树最小的节点
root.right = deleteNode(root.right, minNode.val);
// 用右子树最小的节点替换 root 节点
minNode.left = root.left;
minNode.right = root.right;
root = minNode;
有的读者可能会疑惑,替换 root 节点为什么这么麻烦,直接改 val 字段不就行了?看起来还更简洁易懂:
仅对于这道算法题来说是可以的,但这样操作并不完美,我们一般不会通过修改节点内部的值来交换节点。因为在实际应用中,BST 节点内部的数据域是用户自定义的,可以非常复杂,而 BST 作为数据结构(一个工具人),其操作应该和内部存储的数据域解耦,所以我们更倾向于使用指针操作来交换节点,根本没必要关心内部数据.
最后总结一下吧,通过这篇文章,我们总结出了如下几个技巧:
- 如果当前节点会对下面的子节点有整体影响,可以通过辅助函数增长参数列表,借助参数传递信息。
- 在二叉树递归框架之上,扩展出一套 BST 代码框架:
void BST(TreeNode root, int target) {
if (root.val == target)
// 找到目标,做点什么
if (root.val < target)
BST(root.right, target);
if (root.val > target)
BST(root.left, target);
}
- 根据代码框架掌握了 BST 的增删查改操作。
边栏推荐
猜你喜欢
pycharm 终端部启用虚拟环境
Leetcode-231-2的幂
![[Android -- data storage] use SQLite to store data](/img/f6/a4930276b3da25aad3ab1ae6f1cf49.png)
[Android -- data storage] use SQLite to store data

Enterprise log analysis system elk
Imitate the choice of enterprise wechat conference room
Record the migration process of a project

Eye of depth (VII) -- Elementary Transformation of matrix (attachment: explanation of some mathematical models)
Logback日志框架第三方jar包 免费获取
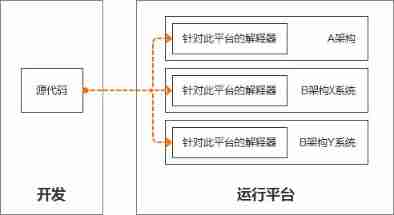
The difference and working principle between compiler and interpreter

Power of leetcode-231-2
随机推荐
Eye of depth (VI) -- inverse of matrix (attachment: some ideas of logistic model)
【C 语言】 题集 of Ⅹ
Leetcode-136-只出现一次的数(用异或来解答)
Excessive dependence on subsidies, difficult collection of key customers, and how strong is the potential to reach the dream of "the first share of domestic databases"?
SysOM 案例解析:消失的内存都去哪了 !| 龙蜥技术
Odoo集成Plausible埋码监控平台
【HCSD大咖直播】亲授大厂面试秘诀-简要笔记
The unity vector rotates at a point
three. JS create cool snow effect
3000 words speak through HTTP cache
Cesium (4): the reason why gltf model is very dark after loading
95.(cesium篇)cesium动态单体化-3D建筑物(楼栋)
Strengthen real-time data management, and the British software helps the security construction of the medical insurance platform
应用程序和matlab的通信方式
laravel怎么获取到public路径
Balanced binary tree (AVL)
Three. JS series (2): API structure diagram-2
Imitate the choice of enterprise wechat conference room
面试题 01.02. 判定是否互为字符重排-辅助数组算法
Apache Doris just "graduated": why should we pay attention to this kind of SQL data warehouse?