当前位置:网站首页>2-3 lookup tree
2-3 lookup tree
2022-07-07 08:26:00 【Perkinl】
2-3 Search tree (2-3-Search-Tree)
- 2- node : The nodes in a standard binary tree are called 2- node ( It has a key and two links ).
- 3- node : Contains two keys and three links .
Definition : One 2-3 Find a tree or an empty tree , Or composed of the following nodes :
2- node , It has a key ( And its corresponding value ) And two links , The left link points to 2-3 All the keys in the tree are smaller than this node , The right link points to 2-3 All the keys in the tree are larger than this node .
3- node , It has two keys ( And its corresponding value ) And three links , The left link points to 2-3 All the keys in the tree are smaller than this node , Link to 2-3 The keys in the tree are located in the Between two keys , The right link points to 2-3 All the keys in the tree are larger than this node .
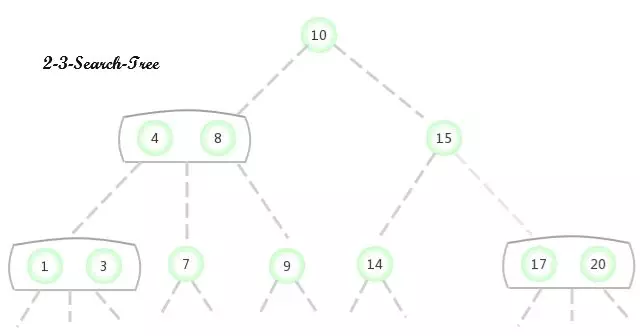
2-3 Search tree (2-3-Search-Tree) operation
A perfectly balanced 2-3 The distance between all empty links in the search tree and the root node should be the same .
lookup
2-3 The search in the search tree is similar to the search in the binary search tree . To determine whether a key is in the tree , First compare it with the key in the root node . If it and any one of them equal , Find hits . otherwise , Find the link to the corresponding interval according to the comparison results , And recursively continue to search in the subtree it points to . If it is an empty link , Find misses .
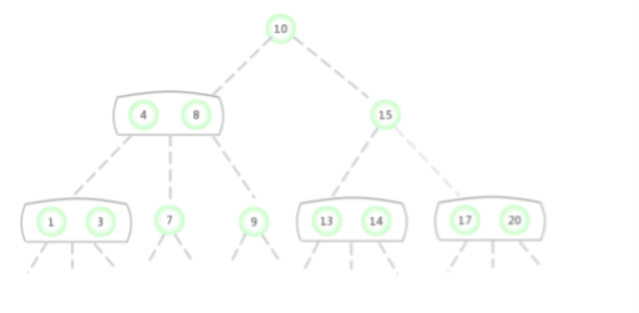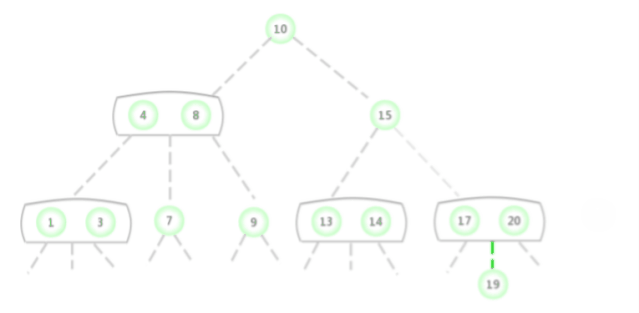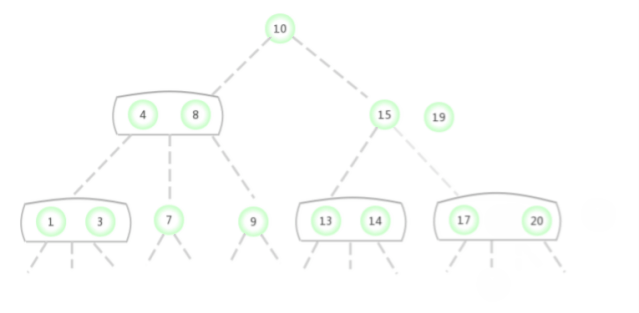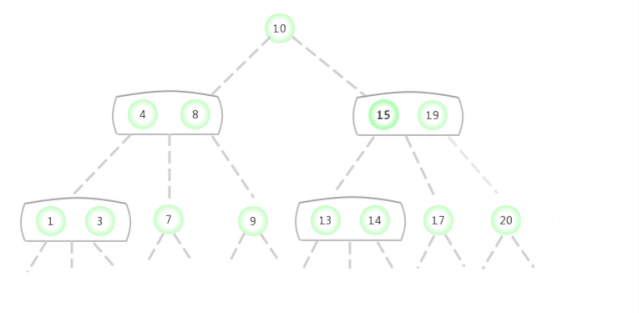
In the picture above 2-3 The search key in the tree is 2 Whether the node exists , The process is as follows :
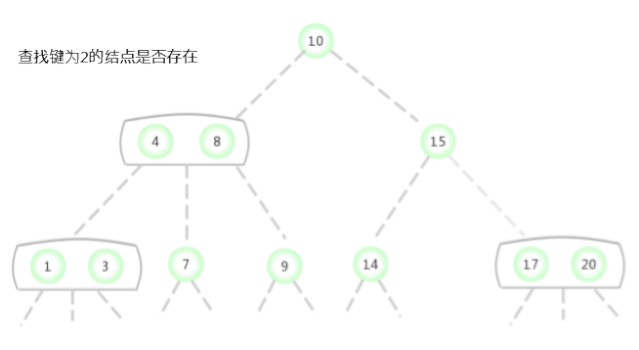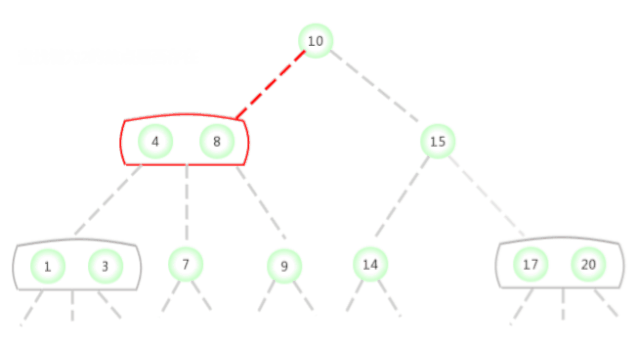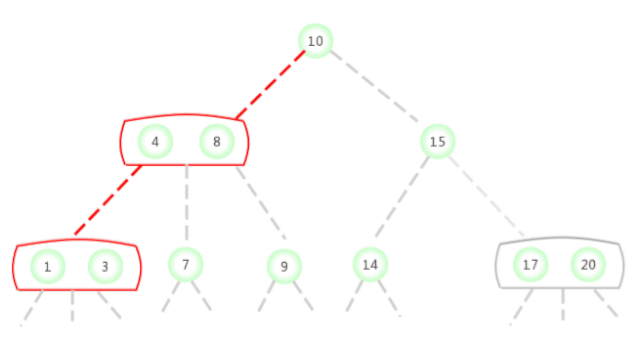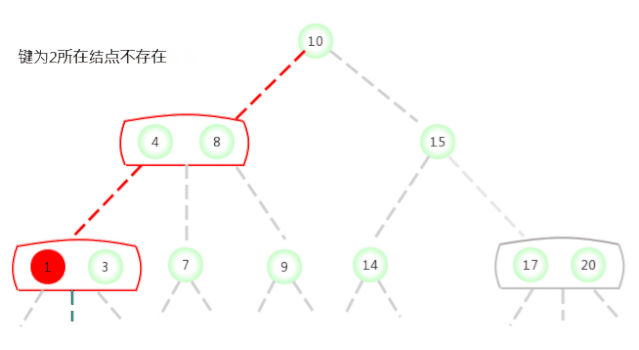
In the picture above 2-3 The search key in the tree is 17 Whether the node exists , The process is as follows :
Insert
towards 2- Insert a new key in the node
To be in 2-3 Insert a new node into the tree , Similar to the insertion of binary search tree , First perform a miss search , Find the location of the node to be inserted , Hang it at the bottom of the tree . But in this way, the tree cannot guarantee perfect balance . Use 2-3 The main reason is : After inserting new nodes, you can continue to maintain balance .
If the missed lookup ends with a 2- node , Will this 2- node Replace with 3- node , Save the key to be inserted in it .
If the missed lookup ends with a 3- node , The analysis process is as follows .
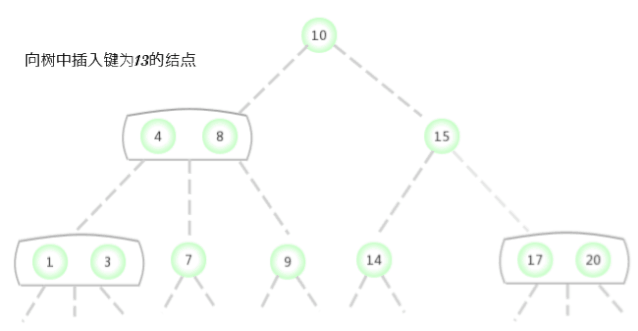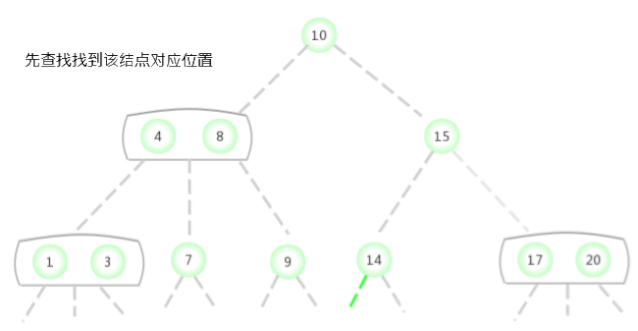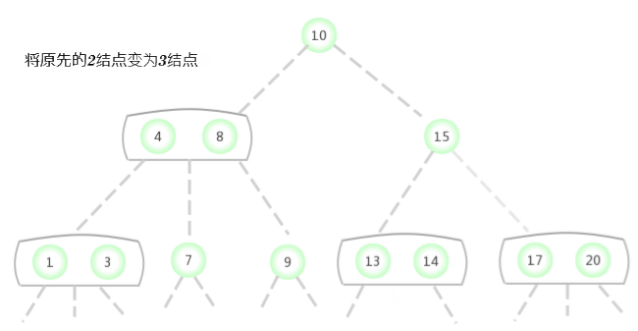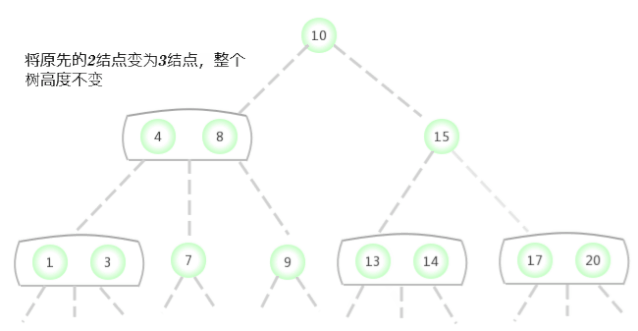
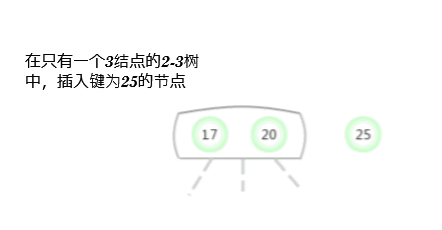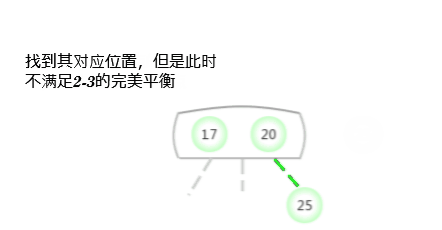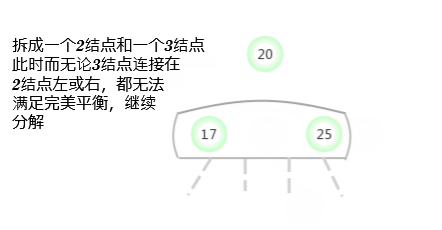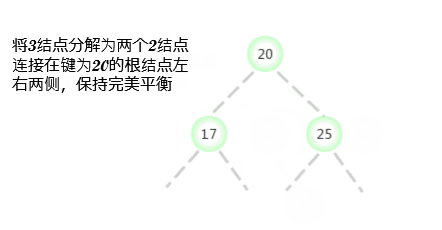
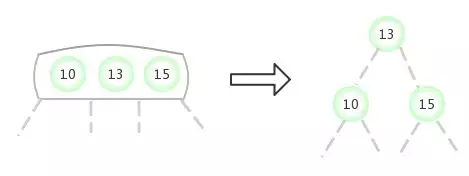
To a tree that contains only one 3- Insert a new key into the tree of the node
Before considering the general situation , Let's assume that we need a tree that contains only one 3- Insert a new key into the tree of the node . There are two keys in this tree , So in its only node There is no room to insert new keys . To insert a new key , First, temporarily store the new key in this node , To form a 4- node ( contain 3 Keys and 4 link ). Create a 4- node Very convenient , Because it is easy to transform it into a tree made of 3 individual 2- node Composed of 2-3 Trees , One of the nodes ( root ) Contains the middle key , A node contains 3 The smallest of the keys ( Connected to the left link of the root node ), A node contains 3 The largest of the keys ( Connect with the right link of the root node ). This tree is a tree that contains 3 Binary search tree of nodes , It is also a perfectly balanced tree 2-3 Trees , Because all of them The distance between empty links and nodes is equal . The height of the tree before insertion is 0, The height of the inserted tree is 1.
To a parent node is 2- Nodal 3- Insert a new key in the node
Suppose that a miss lookup ends in a 3- node , And its parent node is a 2- node . In this case, it's necessary : Under the premise of maintaining the perfect balance of the tree, it is the new key Make room . You need to construct a temporary 4- node And decompose it , But at this time, a new node will not be created for the middle key , Instead, move it to the original parent node .
Regard this transformation as pointing to the original 3- node Replace one link of the new parent node with two links on the left and right sides of the original middle key , And point to two new 2- node . Based on assumptions , Of the oil space in the parent node : The parent node is a 2- node , After inserting, it becomes a 3- node . in addition , This conversion does not affect ( Perfectly balanced )2-3 Trees Of The main properties . The trees are still in order , Because the middle key is moved to the parent node ; The tree is still perfectly balanced , After insertion, the distance from all leaf nodes to the root node is still the same .
The above process is 2-3 The core of tree dynamic change , The diagram shows as follows :
To a parent node is 3- Nodal 3- Insert a new key in the node
Assume that the Miss lookup ends with a parent node of 3- node Of 3- node . Still need to construct a temporary 4- node And decompose it , Then insert its middle key into its parent node . Because the parent node is also a 3- node , After inserting into the middle, a new temporary 4- node , Then do the same transformation on this node , Set decomposes this parent node and inserts its middle key into its parent node . Generalize to the general situation , That's how we keep breaking up the temporary 4- node And insert the middle key into the parent node at a higher level , Until I met a 2- node And replace it with one that does not need further decomposition 3- node , Or to arrive 3- node The root of the .
The process is as follows :
Decompose the root node
If the path from the inserted node to the root node is 3- node , The root node eventually becomes a temporary 4- node . At this time, there is only one tree *3- node The method of inserting new keys into the tree of . Will be temporary 4- node Break it down into three 2- node **, Tree height plus 1. This last change still maintains the perfect balance of the tree , Because it transforms the root node .
The process is as follows :
4- Decomposition of nodes
stay 2-3 Trees in 4- node There are the following situations in the location .
- 1. Root node
- 2. The parent node is 2- node Left child node or right child node of
- 3. Parent node is 3- node The left child of 、 Middle child node or right child node
Now let's take a detailed look at 2-3 Trees How to decompose when the above positions appear in .
situation :
Be temporary 4- node Appears at the root node :
Be temporary 4- node Appear in the 2- node The left side of the :
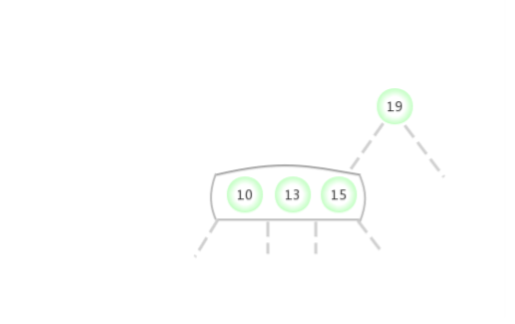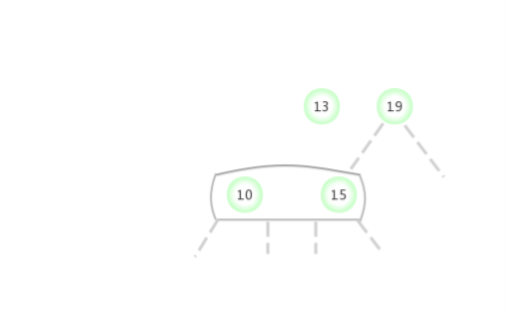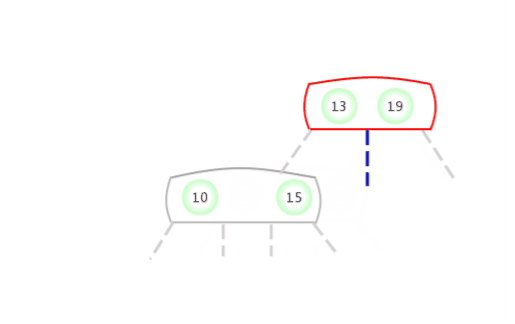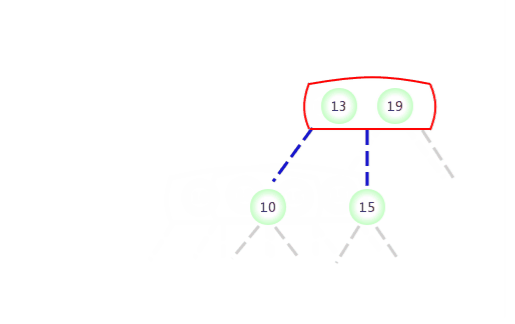
Be temporary 4- node Appear in the 2- node The right side of the :
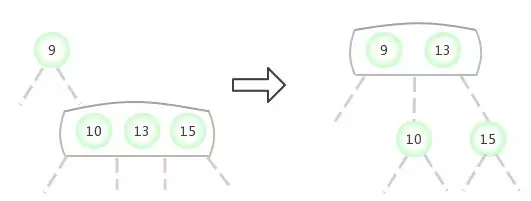
Be temporary 4- node Appear in the 3- node The left side of the :
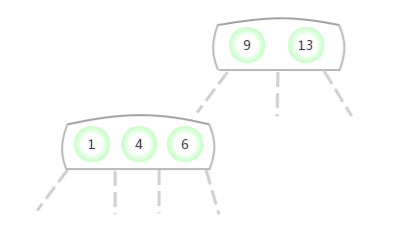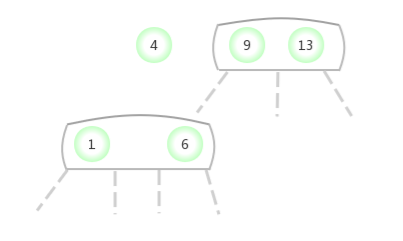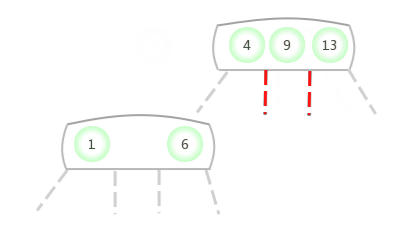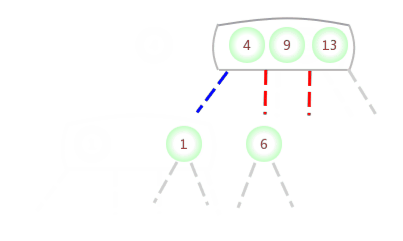
Be temporary 4- node Appear in the 3- node In the middle of the :
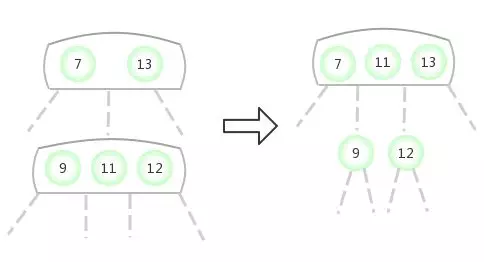
Be temporary 4- node Appear in the 3- node The right side of the :
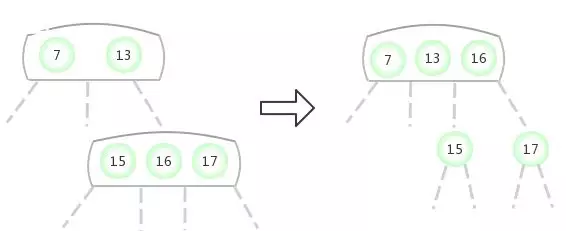
In the above case , In the process of change , Only when the root node is finally temporary 4- node , At this time, it is decomposed into 3 individual 2- node when , The height of the whole tree will increase 1. besides , Insert a node 2-3 The height of the tree is still the original height . In the above change process : The path length of any empty link to the root node is equal .
Conclusion
In a tree the size is N Of 2-3 In the tree , The nodes accessed by the search and insert operations will not exceed lgN.
- prove : A tree contains N Of nodes 2-3 The height of the tree is log2 N and log3 N Between .
边栏推荐
- The single value view in Splunk uses to replace numeric values with text
- One click deployment of highly available emqx clusters in rainbow
- Interface as a parameter (interface callback)
- 国标GB28181协议视频平台EasyGBS新增拉流超时配置
- Opencv learning notes 1 -- several methods of reading images
- The largest 3 same digits in the string of leetcode simple question
- Explore creativity in steam art design
- Standard function let and generic extension function in kotlin
- 在Rainbond中实现数据库结构自动化升级
- Rsync remote synchronization
猜你喜欢

Opencv learning notes 1 -- several methods of reading images
漏洞复现-Fastjson 反序列化

Leetcode medium question my schedule I

漏洞複現-Fastjson 反序列化
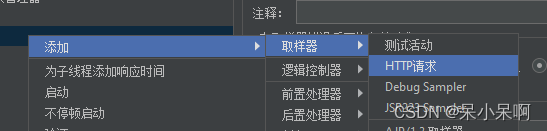
Use of JMeter
![[quick start of Digital IC Verification] 10. Verilog RTL design must know FIFO](/img/56/82f4533b5bded73df222ef65101a72.png)
[quick start of Digital IC Verification] 10. Verilog RTL design must know FIFO

解析创新教育体系中的创客教育
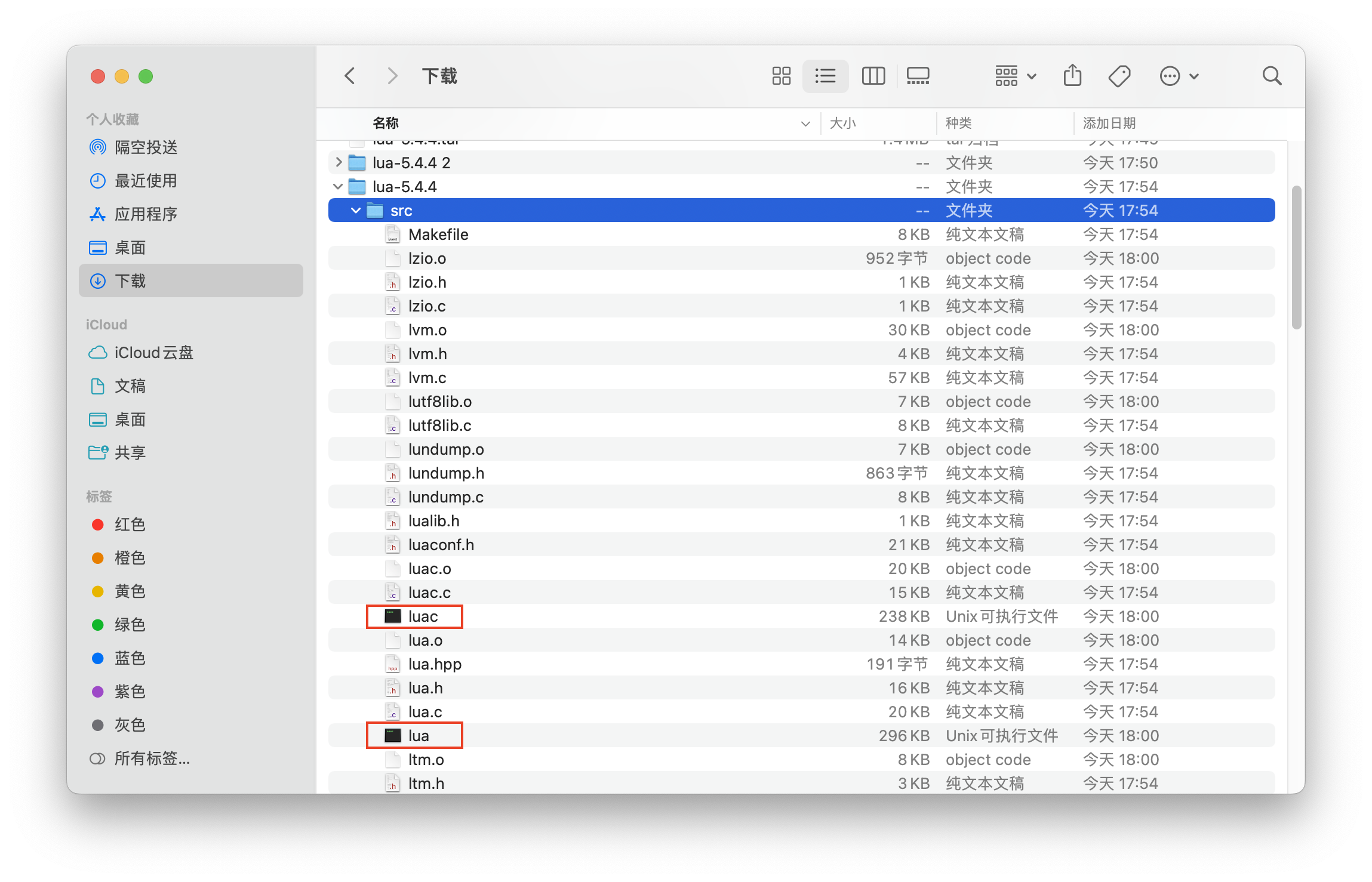
Lua programming learning notes
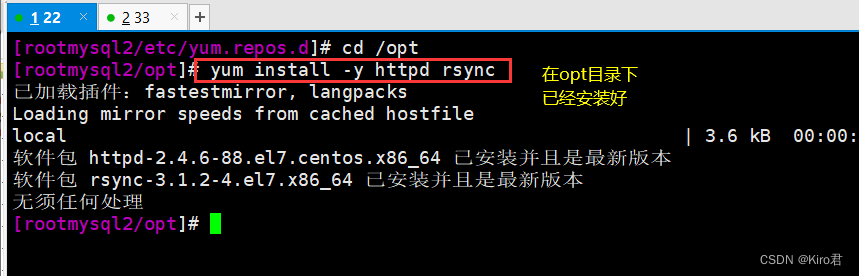
rsync远程同步

opencv学习笔记四——膨胀/腐蚀/开运算/闭运算
随机推荐
The single value view in Splunk uses to replace numeric values with text
XCiT学习笔记
DeiT学习笔记
Snyk dependency security vulnerability scanning tool
Vulnerability recurrence easy_ tornado
单场带货涨粉10万,农村主播竟将男装卖爆单?
[IELTS speaking] Anna's oral learning records Part3
It's too true. There's a reason why I haven't been rich
饥荒云服管理脚本
Improve the delivery efficiency of enterprise products (1) -- one click installation and upgrade of enterprise applications
Snyk 依赖性安全漏洞扫描工具
Automatic upgrading of database structure in rainbow
opencv学习笔记二——图像基本操作
柯基数据通过Rainbond完成云原生改造,实现离线持续交付客户
Using helm to install rainbow in various kubernetes
[IELTS speaking] Anna's oral learning records part2
eBPF Cilium实战(1) - 基于团队的网络隔离
The reified keyword in kotlin is used for generics
Battery and motor technology have received great attention, but electric control technology is rarely mentioned?
Application of slip ring of shipborne radar antenna