当前位置:网站首页>C#绘制带控制点的Bezier曲线,用于点阵图像及矢量图形
C#绘制带控制点的Bezier曲线,用于点阵图像及矢量图形
2022-07-05 08:45:00 【大可山人】
【摘要】不借助第三方, 使用c# + GDI+进行SVG等绘图,绘制带控制点的Bezier曲线。可用于点阵图像及矢量图形(如SVG)绘图。
先看效果:
(不知为何,已两次上传图片,无法显示,求助csdn)
图注:使用方法二绘制。
方法一:
/// <summary>
/// Bezier样条曲线
/// </summary>
public static class BezierSpline
{
/// <summary>
/// Get open-ended Bezier Spline Control Points.
/// </summary>
/// <param name="knots">Input Knot Bezier spline points.</param>
/// <param name="firstControlPoints">Output First Control points
/// array of knots.Length - 1 length.</param>
/// <param name="secondControlPoints">Output Second Control points
/// array of knots.Length - 1 length.</param>
/// <exception cref="ArgumentNullException"><paramref name="knots"/>
/// parameter must be not null.</exception>
/// <exception cref="ArgumentException"><paramref name="knots"/>
/// array must contain at least two points.</exception>
public static void GetCurveControlPoints(Point[] knots,
out Point[] firstControlPoints, out Point[] secondControlPoints)
{
if (knots == null)
throw new ArgumentNullException("knots");
int n = knots.Length - 1;
if (n < 1)
throw new ArgumentException
("At least two knot points required", "knots");
if (n == 1)
{ // Special case: Bezier curve should be a straight line.
firstControlPoints = new Point[1];
// 3P1 = 2P0 + P3
firstControlPoints[0].X = (2 * knots[0].X + knots[1].X) / 3;
firstControlPoints[0].Y = (2 * knots[0].Y + knots[1].Y) / 3;
secondControlPoints = new Point[1];
// P2 = 2P1 – P0
secondControlPoints[0].X = 2 *
firstControlPoints[0].X - knots[0].X;
secondControlPoints[0].Y = 2 *
firstControlPoints[0].Y - knots[0].Y;
return;
}
// Calculate first Bezier control points
// Right hand side vector
double[] rhs = new double[n];
// Set right hand side X values
for (int i = 1; i < n - 1; ++i)
rhs[i] = 4 * knots[i].X + 2 * knots[i + 1].X;
rhs[0] = knots[0].X + 2 * knots[1].X;
rhs[n - 1] = (8 * knots[n - 1].X + knots[n].X) / 2.0;
// Get first control points X-values
double[] x = GetFirstControlPoints(rhs);
// Set right hand side Y values
for (int i = 1; i < n - 1; ++i)
rhs[i] = 4 * knots[i].Y + 2 * knots[i + 1].Y;
rhs[0] = knots[0].Y + 2 * knots[1].Y;
rhs[n - 1] = (8 * knots[n - 1].Y + knots[n].Y) / 2.0;
// Get first control points Y-values
double[] y = GetFirstControlPoints(rhs);
// Fill output arrays.
firstControlPoints = new Point[n];
secondControlPoints = new Point[n];
for (int i = 0; i < n; ++i)
{
// First control point
firstControlPoints[i] = new Point(x[i], y[i]);
// Second control point
if (i < n - 1)
secondControlPoints[i] = new Point(2 * knots
[i + 1].X - x[i + 1], 2 *
knots[i + 1].Y - y[i + 1]);
else
secondControlPoints[i] = new Point((knots
[n].X + x[n - 1]) / 2,
(knots[n].Y + y[n - 1]) / 2);
}
}
/// <summary>
/// Solves a tridiagonal system for one of coordinates (x or y)
/// of first Bezier control points.
/// </summary>
/// <param name="rhs">Right hand side vector.</param>
/// <returns>Solution vector.</returns>
private static double[] GetFirstControlPoints(double[] rhs)
{
int n = rhs.Length;
double[] x = new double[n]; // Solution vector.
double[] tmp = new double[n]; // Temp workspace.
double b = 2.0;
x[0] = rhs[0] / b;
for (int i = 1; i < n; i++) // Decomposition and forward substitution.
{
tmp[i] = 1 / b;
b = (i < n - 1 ? 4.0 : 3.5) - tmp[i];
x[i] = (rhs[i] - x[i - 1]) / b;
}
for (int i = 1; i < n; i++)
x[n - i - 1] -= tmp[n - i] * x[n - i]; // Backsubstitution.
return x;
}
}
方法二:
private void DrawCurve(Graphics g, PointF[] points, float tension)
{
int n=points.Length;
Pen rPen = new Pen(Color.Red, 2f);
Pen blPen= new Pen(Color.Blue, 1f);
Pen bzPen = new Pen(Color.DarkGoldenrod, 2f);
for (int i = 0; i < n; ++i)
{
// draw segment points[i] - points[(i + 1) % n]
var pPrev1 = points[(i - 1 + n) % n];
var p1 = points[i];
var p2 = points[(i + 1) % n];
var pAfter2 = points[(i + 2) % n];
// tangents 切线控制点
var t1 = new PointF(tension * (p2.X - pPrev1.X), tension * (p2.Y - pPrev1.Y));
var t2 = new PointF(tension * (pAfter2.X - p1.X), tension * (pAfter2.Y - p1.Y));
// interior Bezier control points
var c1 = new PointF(p1.X + t1.X / 3.0f, p1.Y + t1.Y / 3.0f);
var c2 = new PointF(p2.X - t2.X / 3.0f, p2.Y - t2.Y / 3.0f);
//画贝塞尔曲线
g.DrawBezier(bzPen, p1, c1, c2, p2);
//画关键点到切线控制点的直线
g.DrawLine(blPen, p1, c1);
g.DrawEllipse(rPen, p1.X - 2, p1.Y - 2, 4, 4);
g.DrawEllipse(rPen, c1.X - 2, c1.Y - 2, 4, 4);
g.DrawLine(blPen, p2, c2);
g.DrawEllipse(rPen, p2.X - 2, p2.Y - 2, 4, 4);
g.DrawEllipse(rPen, c2.X - 2, c2.Y - 2, 4, 4);
g.FillEllipse(new SolidBrush(Color.Green), new RectangleF(p1.X-2, p1.Y-2, 4, 4));
}
}
方法二的调用方法:
//这里使用的是Panel上绘图,其他控件(如PictureBox)道理一样。
Graphics g = pnlWorkArea.CreateGraphics();
g.Clear(Color.White);
g.CompositingQuality = CompositingQuality.HighQuality;
g.InterpolationMode = InterpolationMode.HighQualityBicubic;
g.SmoothingMode = SmoothingMode.HighQuality;
g.TextRenderingHint = TextRenderingHint.AntiAliasGridFit;
PointF[] points = { new PointF(568,200),new PointF(168,110),new PointF(60,186),new PointF(300,191),new PointF(600,300),new PointF(800,431),new PointF(300,650), new PointF(568, 200) };
float tension=0.68f;
DrawCurve(g, points, tension);
这里有一个不错的链接:
C# GraphicsPath AddBeziers(params System.Drawing.Point[] points)
C# GraphicsPath AddBeziers(System.Drawing.PointF[] points)
源码也可以在此下载:C#带控制点的贝塞尔Bezier曲线算法(源码)-C#文档类资源-CSDN下载
边栏推荐
- Halcon Chinese character recognition
- Lori remote control LEGO motor
- Count of C # LINQ source code analysis
- ECMAScript6介绍及环境搭建
- Yolov4 target detection backbone
- Programming implementation of subscriber node of ROS learning 3 subscriber
- 资源变现小程序添加折扣充值和折扣影票插件
- kubeadm系列-02-kubelet的配置和启动
- Arrangement of some library files
- Dynamic dimensions required for input: input, but no shapes were provided. Automatically overriding
猜你喜欢
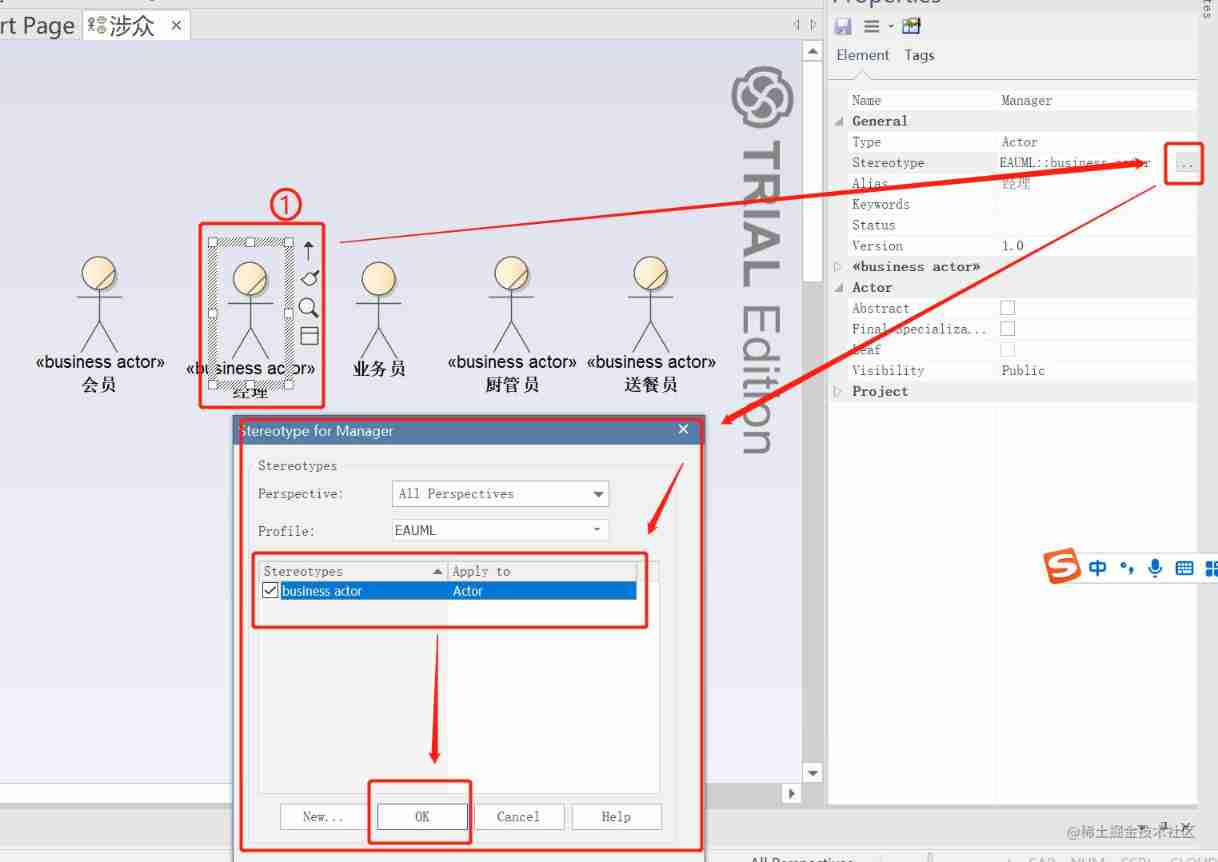
Business modeling of software model | stakeholders
Run菜单解析
EA introduction notes

My university
Use and programming method of ros-8 parameters

Beautiful soup parsing and extracting data
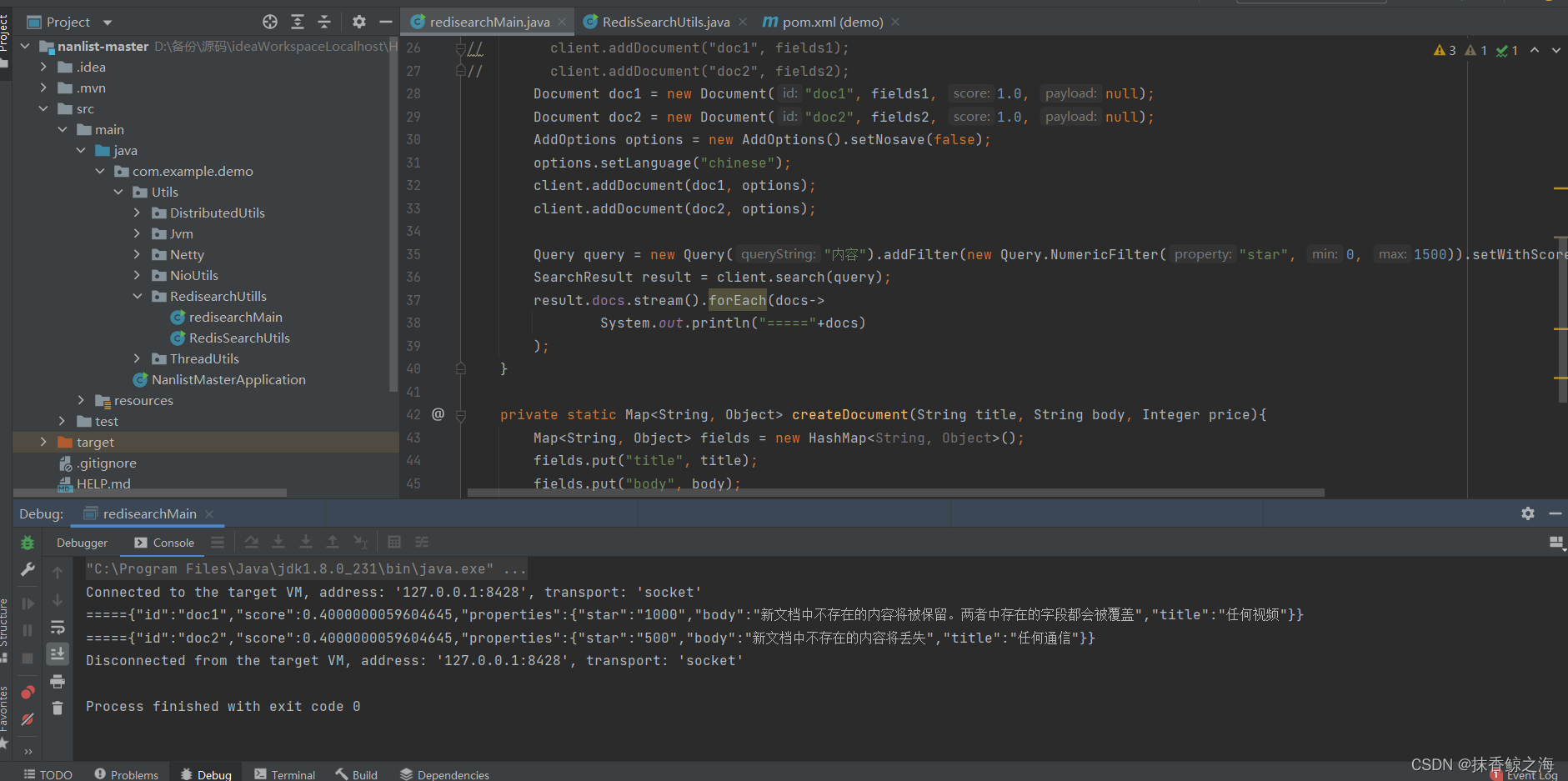
Redis implements a high-performance full-text search engine -- redisearch
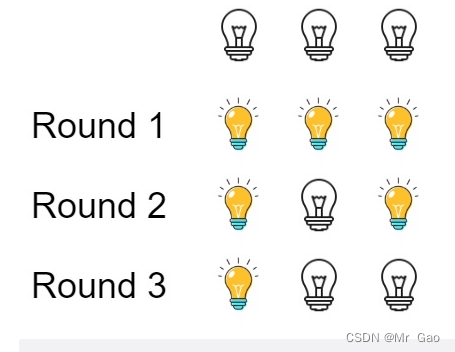
319. 灯泡开关

Guess riddles (142)
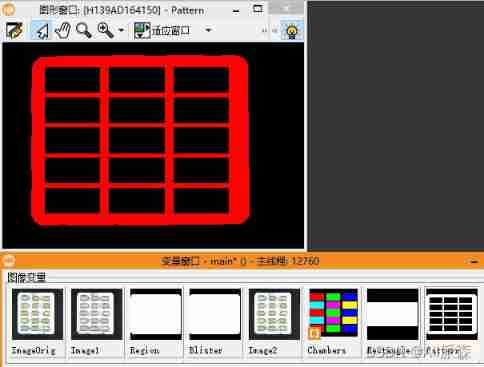
Halcon: check of blob analysis_ Blister capsule detection
随机推荐
Numpy 小坑:维度 (n, 1) 和 维度 (n, ) 数组相加运算后维度变为 (n, n)
696. Count binary substring
How can fresh students write resumes to attract HR and interviewers
Array, date, string object method
Ecmascript6 introduction and environment construction
The first week of summer vacation
Reasons for the insecurity of C language standard function scanf
Illustration of eight classic pointer written test questions
Tips 1: Web video playback code
Run menu analysis
暑假第一周
Lori remote control LEGO motor
皮尔森相关系数
My university
Speech recognition learning summary
Guess riddles (9)
location search 属性获取登录用户名
One dimensional vector transpose point multiplication np dot
我从技术到产品经理的几点体会
Halcon Chinese character recognition