Special topic of rotor position estimation of permanent magnet synchronous motor -- fundamental wave model and rotor position angle

Preface

The topic of rotor position estimation of permanent magnet synchronous motor will be written as a series , The commonly used motor position estimation method of permanent magnet synchronous motor is decomposed into several subcategories , Then write the specific principles one by one . The style of the article is consistent with other articles , Keep it easy to understand , Without losing depth .

This is the opening work of this topic , First understand what angle the rotor position angle refers to , And connect it with the mathematical model .

One 、 Rotor position angle of permanent magnet synchronous motor

This article wants to explain how to estimate the rotor position angle , This is a big topic , In order to make this problem clear , First of all, understand what is the rotor position angle .

First, I want to add the basic knowledge of permanent magnet synchronous motor , When doing permanent magnet synchronous motor control , We are concerned with the interaction between the stator and the rotor , This force is generated by the interaction of stator magnetic field and rotor magnetic field . The rotor of permanent magnet synchronous motor is a permanent magnet , After being produced , Its magnetic field is fixed , Its direction is rotor N The direction of the pole . The stator magnetic field is generated by current , The greater the current , The stronger the magnetic field generated , The magnitude of the magnetic field generated by each phase current is proportional to the current at the current moment , The direction is the direction of the phase in space . The total stator magnetic field is the vector sum of the magnetic field generated by the three-phase current .

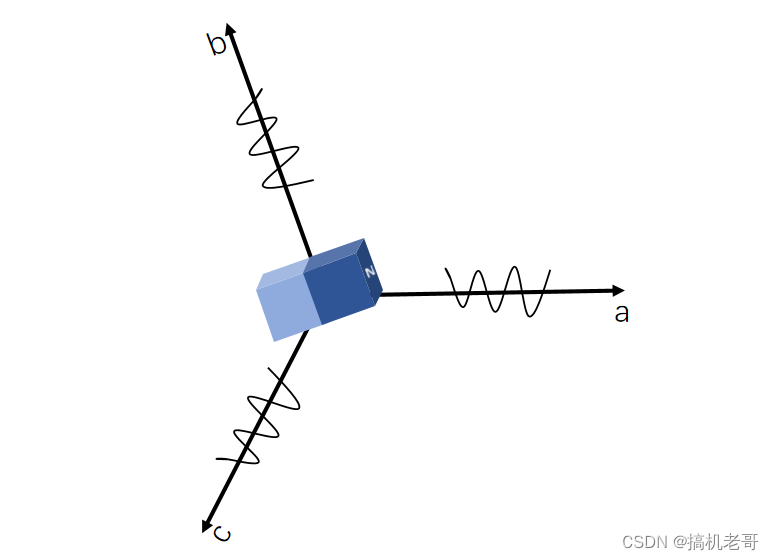
Take a pair of pole permanent magnet synchronous motor as an example , The simplified model is shown in the figure above .

In order to illustrate the spatial relationship of rotor position angle , Here, it is analyzed to use the pre positioning method to drag the rotor to 0° The process of .

Make $id=10A,iq=0,focAngle=0;$

Carry out counter-measures park Transformation and inversion clark Transformation

$$\left[\begin{array}{c}{i}_{\alpha }\\ i_{\beta }\end{array}\right]=\left[\begin{array}{cc}cos\theta & sin\theta \\ -sin\theta & cos\theta \end{array}\right]\left[\begin{array}{c}{i}_d\\ i_q\end{array}\right]\text{\hspace{1em}(1)}$$

back park Transformation

$$\left[\begin{array}{c}{i}_a\\ i_b\\ ic\end{array}\right]=\frac{2}{3}\left[\begin{array}{cc}1& 0\\ -\frac{1}{2}& \frac{\sqrt{3}}{2}\\ -\frac{1}{2}& -\frac{\sqrt{3}}{2}\end{array}\right]\left[\begin{array}{c}{i}_{\alpha }\\ i_{\beta }\end{array}\right]\text{\hspace{1em}(2)}$$

back clark Transformation

Into the Make $id=10A,iq=0,focAngle=0$, Yes $i_{\alpha }=10A,i_{\beta }=0;i_a=6.67A,i_b=-3.3A,i_c=-3.3A$

Put it in the picture above , Vector synthesis , No matter in $\alpha \beta$ Coordinate system , Still $abc$ Coordinate system , The same conclusion can be reached , The direction and of the composite vector a Axis coincidence , The size is 10A;

So when the position angle of the pre positioned rotor is 0 When the degree of , Get one Direction and a Stator magnetic field with coincident shafts , Pull the rotor to a Axis direction . It can also be said that the rotor position angle is 0 When the degree of , rotor N Pole position and a Phase stator coils coincide , Here we are , The following statement has been verified ： The rotor position angle refers to the rotor in space N Pole and stator a Electrical included angle of phase winding .

Rotor position angle here , Also called vector angle , Pole position angle , Different industries have different customary names .

Two 、 Where does the rotor position angle react

To estimate the rotor position , You must know when the rotor position is in different positions , How will you react . stay abc Coordinate system , Three phase voltage 、 electric current 、 flux linkage , Coupled with each other , The mathematical model is complex , Common rotor position estimation methods are $\alpha \beta$ Coordinate system .

2.1 $\alpha \beta$ Mathematical model in coordinate system

Let's first look at the voltage equation
$$\left[\begin{array}{c}{v}_{\alpha }\\ v_{\beta }\end{array}\right]=\left[\begin{array}{c}{i}_{\alpha }\\ i_{\beta }\end{array}\right]R_s+\frac{d}{dt}\left[\begin{array}{c}{\varphi }_{\alpha }\\ {\varphi }_{\beta }\end{array}\right]\text{\hspace{1em}(3)}$$
among ${\varphi }_{\alpha }{\varphi }_{\beta }$ Express $\alpha \beta$ Axial flux linkage

$$\left[\begin{array}{c}{\varphi }_{\alpha }\\ {\varphi }_{\beta }\end{array}\right]=\left[\begin{array}{cc}cos\theta & sin\theta \\ -sin\theta & cos\theta \end{array}\right]\left[\begin{array}{c}{L}_s{i}_d+{\varphi }_f\\ L_s{i}_q\end{array}\right]\text{\hspace{1em}(4)}$$
hold $dq$ The axis flux linkage changes to $\alpha \beta$ Axis
$$\left[\begin{array}{c}{\varphi }_{\alpha }\\ {\varphi }_{\beta }\end{array}\right]=\left[\begin{array}{c}{i}_{\alpha }\\ i_{\beta }\end{array}\right]L_s+\left[\begin{array}{c}{\varphi }_{f}cos\theta \\ {\varphi }_{f}sin\theta \end{array}\right]\text{\hspace{1em}(5)}$$
among ${\varphi }_f$ Indicates permanent magnet flux linkage .

Relative to the above form of voltage equation , I think more friends are more familiar with the following forms

$$\left[\begin{array}{c}{v}_{\alpha }\\ v_{\beta }\end{array}\right]=\left[\begin{array}{c}{i}_{\alpha }\\ i_{\beta }\end{array}\right]R_s+\frac{d}{dt}\left[\begin{array}{c}{L}_s{i}_{\alpha }\\ L_s{i}_{\beta }\end{array}\right]+\left[\begin{array}{c}-{\omega }_e{\varphi }_{f}sin\theta \\ {\omega }_e{\varphi }_{f}cos\theta \end{array}\right]\text{\hspace{1em}(6)}$$
among ${\omega }_e$ Is the electrical angular velocity .
But compared to (6),(4) and (5) Easier to explain

2.2 $\alpha \beta$ The rotor flux linkage component of the shaft contains position information

(4) in ,$\alpha \beta$ The flux linkage of the shaft is composed of $dq$ Axial flux linkage ipark transformation , among ,$d$ The axis flux linkage is composed of $L_s{i}_d$ and ${\varphi }_f$ Two parts , I said before. ,$d$ The position of the shaft is defined as a permanent magnet $N$ The direction of the pole , So permanent magnet flux linkage ${\varphi }_f$ Is only found in $d$ Axis , The other part is the current flowing $d$ Stator flux linkage generated by shaft inductance $L_s{i}_d$ ;$q$ The shaft has only stator flux $L_s{i}_q$.

take (4) Medium ipark Transform expansion , Get the formula (5)
You can see ,** The flux linkage in the air gap of permanent magnet synchronous motor is composed of two parts , One part is stator flux $\left[\begin{array}{c}{i}_{\alpha }\\ i_{\beta }\end{array}\right]L_s$, It is generated by the current flowing through the stator coil , The other part is rotor flux $\left[\begin{array}{c}{\varphi }_{f}cos\theta \\ {\varphi }_{f}sin\theta \end{array}\right]$, Produced by the rotor permanent magnet .

$\alpha \beta$ The rotor flux linkage component in the coordinate system contains the rotor angle information .

2.3 $\alpha \beta$ The back EMF voltage component of the shaft contains position information

take (5) Plug in (3) obtain (6), This formula is common $\alpha \beta$ Expression of voltage equation in coordinate system . We know that changes in the magnetic field produce an electric field , In permanent magnet synchronous motor , The stator coil of each phase is wound around the stator core to form a closed space , In this space , Flux linkage changes , Voltage is formed at both ends of the coil , The faster the flux linkage changes , The greater the voltage generated .$\left[\begin{array}{c}-{\omega }_e{\varphi }_{f}sin\theta \\ {\omega }_e{\varphi }_{f}cos\theta \end{array}\right]$ Describes the voltage generated by the rotation of the rotor magnetic field , Relationship with rotor speed and current rotor angle , This part of voltage is also called back EMF ;$\left[\begin{array}{c}{L}_s{i}_{\alpha }\\ L_s{i}_{\beta }\end{array}\right]$ The relationship between voltage and current caused by the change of stator magnetic field is described .

$\alpha \beta$ The back EMF voltage component in the coordinate system contains the rotor angle information .

3、 ... and 、 Summary

This paper is the first article on the topic of rotor position estimation of permanent magnet synchronous motor , To ensure that the length of each article is not too long , End here . The common methods of rotor position estimation are decomposed into ,$\alpha \beta$ Location estimation of fundamental wave model in coordinate system 、$dq$ Location estimation of fundamental wave model in coordinate system 、 Position estimation of injection signal class , Wait for multiple directions , Specific decomposition .

Like my article , Remember to pay attention .