当前位置:网站首页>【NOI模拟赛】汁树(树形DP)
【NOI模拟赛】汁树(树形DP)
2022-07-05 08:23:00 【DD(XYX)】
题面
原题:「牛客 31454H」Permutation on Tree


题解
我们把绝对值符号拆开
∑ i = 1 n − 1 ∣ P i + 1 − P i ∣ = ∑ i = 1 n − 1 ( P i + 1 − P i ) [ P i + 1 > P i ] + ( P i − P i + 1 ) [ P i + 1 < P i ] \sum_{i=1}^{n-1}|P_{i+1}-P_i|=\sum_{i=1}^{n-1}(P_{i+1}-P_i)[P_{i+1}>P_i]+(P_{i}-P_{i+1})[P_{i+1}<P_i] i=1∑n−1∣Pi+1−Pi∣=i=1∑n−1(Pi+1−Pi)[Pi+1>Pi]+(Pi−Pi+1)[Pi+1<Pi]
然后对于每个数 x x x,计算它系数为 1 的方案数和系数为 -1 的方案数。
以系数为 1 的方案数为例。
原本全局方案数是通过一个简单的 DP( d p [ x ] = ( s i z [ x ] − 1 ) ! ∏ x → y d p [ y ] s i z [ y ] ! dp[x]=(siz[x]-1)!\prod_{x\rightarrow y}\frac{dp[y]}{siz[y]!} dp[x]=(siz[x]−1)!∏x→ysiz[y]!dp[y])来计算的,我们要专门考虑 x x x ,就把整个排列砍成两半,在树上表现为砍掉 x x x 的子树(单独计算子树内部方案数),然后在剩余的树中挑选一些放到排列中 x x x 的后面。同时,我们还得给 x x x找个学伴找个邻居来配对。于是设计状态:
- d p 0 [ i ] [ j ] dp0[i][j] dp0[i][j] :仅考虑 i i i 的子树内部,放了 j j j 个到 x x x 的后面,没有给 x x x 配对的方案数。
- d p 1 [ i ] [ j ] dp1[i][j] dp1[i][j] :仅考虑 i i i 的子树内部,放了 j j j 个到 x x x 的后面,子树内已经给 x x x 配对的方案数。
当我们合并两个子树 A , B A,B A,B 时,
d p 0 ′ [ r o o t ] [ j + k ] ← d p 0 [ A ] [ j ] ⋅ d p 0 [ B ] [ k ] ⋅ ( s i z [ A ] + s i z [ B ] s i z [ A ] ) ⋅ ( i + k i ) d p 1 ′ [ r o o t ] [ j + k ] ← d p 0 [ A ] [ j ] ⋅ d p 1 [ B ] [ k ] ⋅ ( s i z [ A ] + s i z [ B ] − 1 s i z [ B ] − 1 ) ⋅ ( i + k i ) ← d p 1 [ A ] [ j ] ⋅ d p 0 [ B ] [ k ] ⋅ ( s i z [ A ] + s i z [ B ] − 1 s i z [ A ] − 1 ) ⋅ ( i + k i ) dp0'[root][j+k]\leftarrow dp0[A][j]\cdot dp0[B][k]\cdot {siz[A]+siz[B]\choose siz[A]}\cdot {i+k\choose i}\\ dp1'[root][j+k]\leftarrow dp0[A][j]\cdot dp1[B][k]\cdot {siz[A]+siz[B]-1\choose siz[B]-1}\cdot {i+k\choose i}\\ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ ~ \,\leftarrow dp1[A][j]\cdot dp0[B][k]\cdot {siz[A]+siz[B]-1\choose siz[A]-1}\cdot {i+k\choose i} dp0′[root][j+k]←dp0[A][j]⋅dp0[B][k]⋅(siz[A]siz[A]+siz[B])⋅(ii+k)dp1′[root][j+k]←dp0[A][j]⋅dp1[B][k]⋅(siz[B]−1siz[A]+siz[B]−1)⋅(ii+k) ←dp1[A][j]⋅dp0[B][k]⋅(siz[A]−1siz[A]+siz[B]−1)⋅(ii+k)
然后,若 i i i 不是 x x x 的祖先,可以有
d p 0 [ i ] [ s i z [ i ] ] ← d p 0 [ i ] [ 0 ] dp0[i][siz[i]]\leftarrow dp0[i][0] dp0[i][siz[i]]←dp0[i][0]
若 i i i 比 x x x 小(与 x x x 配对可使之系数为 1),有
d p 1 [ i ] [ s i z [ i ] − 1 ] ← d p 0 [ i ] [ 0 ] ⋅ ( [ i = f a [ x ] ∨ l c a ( i , x ) ≠ i ] + [ l c a ( i , x ) ≠ i ] ) dp1[i][siz[i]-1]\leftarrow dp0[i][0]\cdot([i=fa[x]\lor lca(i,x)\not=i]+[lca(i,x)\not=i]) dp1[i][siz[i]−1]←dp0[i][0]⋅([i=fa[x]∨lca(i,x)=i]+[lca(i,x)=i])
最后再考虑一下 x x x 的儿子与之配对的情况。
总状态数 O ( ∑ s i z [ i ] ) O(\sum siz[i]) O(∑siz[i]) ,转移是经典树上背包,所以总时间复杂度 O ( n 3 ) O(n^3) O(n3) 。
CODE
没怎么卡常,甚至有大量废用代码
#include<map>
#include<set>
#include<cmath>
#include<ctime>
#include<queue>
#include<stack>
#include<random>
#include<bitset>
#include<vector>
#include<cstdio>
#include<cstring>
#include<iostream>
#include<algorithm>
#include<unordered_map>
#pragma GCC optimize(2)
using namespace std;
#define MAXN 205
#define LL long long
#define ULL unsigned long long
#define ENDL putchar('\n')
#define DB double
#define lowbit(x) (-(x) & (x))
#define FI first
#define SE second
#define PR pair<int,int>
#define UIN unsigned int
int xchar() {
static const int maxn = 1000000;
static char b[maxn];
static int pos = 0,len = 0;
if(pos == len) pos = 0,len = fread(b,1,maxn,stdin);
if(pos == len) return -1;
return b[pos ++];
}
// #define getchar() xchar()
inline LL read() {
LL f = 1,x = 0;int s = getchar();
while(s < '0' || s > '9') {
if(s<0)return -1;if(s=='-')f=-f;s = getchar();}
while(s >= '0' && s <= '9') {
x = (x<<1) + (x<<3) + (s^48);s = getchar();}
return f*x;
}
void putpos(LL x) {
if(!x)return ;putpos(x/10);putchar((x%10)^48);}
inline void putnum(LL x) {
if(!x) {
putchar('0');return ;}
if(x<0) putchar('-'),x = -x;
return putpos(x);
}
inline void AIput(LL x,int c) {
putnum(x);putchar(c);}
const int MOD = 1000000007;
int n,m,s,o,k;
int rt,C[MAXN<<1][MAXN<<1];
int hd[MAXN],nx[MAXN<<1],v[MAXN<<1],cne;
void ins(int x,int y) {
nx[++ cne] = hd[x]; v[cne] = y; hd[x] = cne;
}
int d[MAXN],fa[MAXN];
void dfs0(int x,int ff) {
d[x] = d[fa[x] = ff] + 1;
for(int i = hd[x];i;i = nx[i]) {
if(v[i] != ff) {
dfs0(v[i],x);
}
}return ;
}
int lca(int a,int b) {
if(d[a] < d[b]) swap(a,b);
while(d[a] > d[b]) a = fa[a];
if(a == b) return a;
while(a != b) a = fa[a],b = fa[b];
return a;
}
int Ft;
int dp[MAXN][MAXN],dp1[MAXN][MAXN],siz[MAXN];
bool ifa[MAXN],mg[MAXN];
void dfsi(int x,int ff) {
dp[x][0] = 1; siz[x] = 0;
for(int i = hd[x];i;i = nx[i]) {
int y = v[i]; if(y == ff) continue;
dfsi(y,x); siz[x] += siz[y];
dp[x][0] = dp[x][0] *1ll* dp[y][0] % MOD * C[siz[x]][siz[y]] % MOD;
} siz[x] ++; return ;
}
void dfs(int x,int ff) {
for(int i = 0;i <= n;i ++) dp[x][i] = dp1[x][i] = 0;
dp[x][0] = 1; siz[x] = 0;
for(int i = hd[x];i;i = nx[i]) {
int y = v[i]; if(y==ff || y==Ft) continue;
dfs(y,x); siz[x] += siz[y];
for(int i = siz[x];i >= 0;i --) {
int dpp = 0,dpp1 = 0;
for(int j = max(0,siz[y]-(siz[x]-i));j <= siz[y] && j <= i;j ++) {
(dpp += dp[x][i-j]*1ll*dp[y][j]%MOD * C[siz[x]-i][siz[y]-j] % MOD * C[i][j] % MOD) %= MOD;
if(i<siz[x]) (dpp1 += dp1[x][i-j]*1ll*dp[y][j]%MOD * C[siz[x]-i-1][siz[y]-j] % MOD * C[i][j] % MOD) %= MOD;
if(i<siz[x] && j<siz[y]) (dpp1 += dp[x][i-j]*1ll*dp1[y][j]%MOD * C[siz[x]-i-1][siz[y]-j-1] % MOD * C[i][j] % MOD) %= MOD;
}
dp[x][i] = dpp; dp1[x][i] = dpp1;
}
}
siz[x] ++;
if(!ifa[x]) dp[x][siz[x]] = dp[x][0];
if(mg[x] && (!ifa[x] || x == fa[Ft])) (dp1[x][siz[x]-1] += dp[x][0]) %= MOD;
if(mg[x] && !ifa[x]) (dp1[x][siz[x]-1] += dp[x][0]) %= MOD;
return ;
}
int solve(int s,int op) {
Ft = s;
for(int i = 1;i <= n;i ++) {
ifa[i] = mg[i] = 0;
if(op > 0) mg[i] = (i > s);
else mg[i] = (i < s);
}
int as = 0;
int p = s; while(p) ifa[p] = 1,p = fa[p];
dfsi(s,fa[s]);
int le = siz[s];
int as0 = 1,as1 = 0,sz = 0;
for(int i = hd[s];i;i = nx[i]) {
int y = v[i]; if(y == fa[s]) continue;
sz += siz[y];
as1 = as1 *1ll* dp[y][0] % MOD * C[sz-1][siz[y]] % MOD;
if(mg[y]) (as1 += as0 *1ll* dp[y][0] % MOD * C[sz-1][siz[y]-1] % MOD) %= MOD;
as0 = as0 *1ll* dp[y][0] % MOD * C[sz][siz[y]] % MOD;
}
if(rt != s) {
dfs(rt,0);
for(int i = 0;i <= siz[rt];i ++) {
(as += dp1[rt][i] *1ll* dp[s][0] % MOD * C[le-1+i][i] % MOD) %= MOD;
if(le>1) (as += dp[rt][i] *1ll* as1 % MOD * C[le-2+i][i] % MOD) %= MOD;
}
}
else as = as1;
return as;
}
int main() {
freopen("tree.in","r",stdin);
freopen("tree.out","w",stdout);
n = read(); rt = read();
for(int i = 1;i < n;i ++) {
s = read();o = read();
ins(s,o); ins(o,s);
}
dfs0(rt,0);
C[0][0] = 1;
for(int i = 1;i <= n;i ++) {
C[i][0] = C[i][i] = 1;
for(int j = 1;j < i;j ++) {
C[i][j] = (C[i-1][j-1] + C[i-1][j]) % MOD;
}
}
int ans = 0;
for(int i = 1;i <= n;i ++) {
int A = solve(i,-1),B = solve(i,1);
ans = (ans + (A +MOD- B) *1ll* i) % MOD;
}
AIput(ans,'\n');
return 0;
}
边栏推荐
- 实例010:给人看的时间
- Halcon's practice based on shape template matching [2]
- Arduino uses nrf24l01+ communication
- leetcode - 445. 两数相加 II
- 动力电池UL2580测试项目包括哪些
- [tutorial 15 of trio basic from introduction to proficiency] trio free serial communication
- Shell script realizes the reading of serial port and the parsing of message
- Simple design description of MIC circuit of ECM mobile phone
- 【三层架构】
- C, Numerical Recipes in C, solution of linear algebraic equations, LU decomposition source program
猜你喜欢
![[three tier architecture]](/img/73/c4c75a453f03830e83cabb0762eb9b.png)
[three tier architecture]
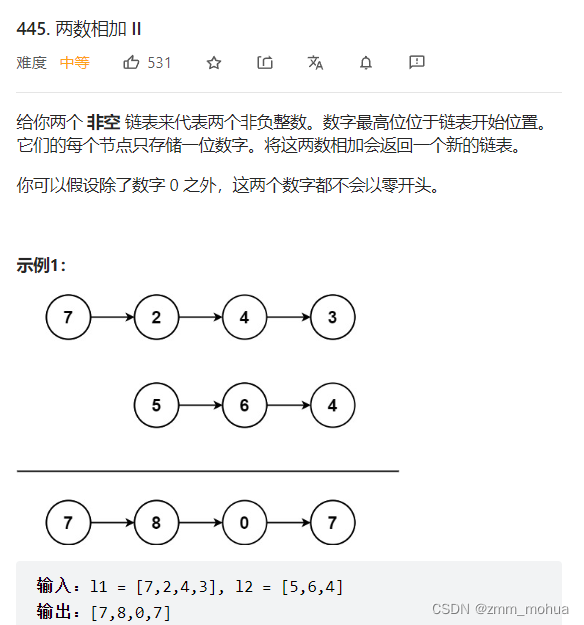
leetcode - 445. 两数相加 II

Compilation warning solution sorting in Quartus II
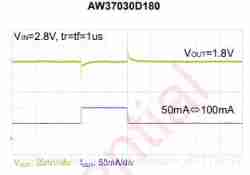
Several important parameters of LDO circuit design and type selection

Volatile of C language

Example 007: copy data from one list to another list.
实例009:暂停一秒输出

剑指 Offer 05. 替换空格
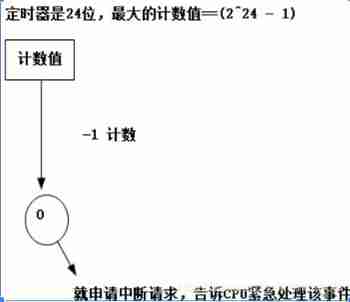
Stm32--- systick timer
![Measurement fitting based on Halcon learning [III] PM_ measure_ board. Hdev routine](/img/f9/fc4f0bbce36b3c1368d838d723b027.jpg)
Measurement fitting based on Halcon learning [III] PM_ measure_ board. Hdev routine
随机推荐
【三层架构】
Circleq of linked list
STM32 --- serial port communication
STM32 single chip microcomputer -- volatile keyword
NTC thermistor application - temperature measurement
Basic information commands and functions of kernel development
Summary -st2.0 Hall angle estimation
matlab timeserise
STM32---IIC
Installation and use of libjpeg and ligpng
实例003:完全平方数 一个整数,它加上100后是一个完全平方数,再加上168又是一个完全平方数,请问该数是多少?
Simple design description of MIC circuit of ECM mobile phone
剑指 Offer 06. 从尾到头打印链表
Imx6ull bare metal development learning 1-assembly lit LED
Various types of questions judged by prime numbers within 100 (C language)
Example 003: a complete square is an integer. It is a complete square after adding 100, and it is a complete square after adding 168. What is the number?
Naming rules for FreeRTOS
【云原生 | 从零开始学Kubernetes】三、Kubernetes集群管理工具kubectl
实例002:“个税计算” 企业发放的奖金根据利润提成。利润(I)低于或等于10万元时,奖金可提10%;利润高于10万元,低于20万元时,低于10万元的部分按10%提成,高于10万元的部分,可提成7.
Detailed explanation of SQL server stored procedures