Quick introduction to automatic control principle + understanding

Know the whole picture in the simplest words .
PS： The default is linear system , That is, the input and output are linear （ By default, you know what is linear ）.

Preliminary understanding of control

Suppose you are pushing a box , Your pushing force is $f$, Case displacement is $x$, Quality is $1$, No friction , Then according to simple physics ：

$$f=ma=\ddot{x}$$
One thing that needs special attention , Whether it's f still x It's all about time t Function of , This formula actually looks like this , This is it. Model of the system ：
$$f(t)=ma=\ddot{x}(t)$$
hypothesis $f(t)=1$, The solution is $x(t)=\frac{1}{2}{t}^2$, Get a very important output function about time .

Let's think about a problem , because F It won't be the same every time , The force we push can be any function of time , Should we bring it in every time F To solve a complex differential equation , Is there a better way ？ The answer is Laplace transform .
Laplace transform a lot , Remember a few key , The rest is to check the table . But the differential property of Laplace transform must be known A top priority . Just remember the following two , The same is true for higher-order forms , However, the topic generally does not have . If it is really high-level, it must be solved by software .
$$L\left[\frac{df(t)}{dt}\right]=sF(s)-f(0)$$,
$$L\left[\frac{d^{2}f(t)}{dt}\right]=s^{2}F(s)-sf(0)-f^{\prime }(0)$$

Let's solve it with Laplace transform ( Unified lowercase represents time domain , Capital stands for s Domain ), We assume that the initial values are 0：
$$F(t)=s^{2}X(s)-sx(0)-\dot{x}(0)=s^{2}X(s)$$
$$G(s)=\frac{X(s)}{F(s)}=\frac{1}{s^2}$$

Now you know s The ratio of output to input in the field , When your output $f(t)$ After changing , You just need to find the Laplace transform $F(s)$ Bring it in to find $X(s)$, Then pull the inverse transformation to get the result . Whatever you are $f(t)$ How to change , Not right $G(s)$ An impact , This is in this system An unchanging formula , That is, it represents the internal nature of the system .

Let's try $f(t)=1$, be $F(s)=\frac{1}{s}$, be $X(s)=G(s)F(s)=\frac{1}{s^3}$, Find the pull inverse transformation $x(t)=\frac{1}{2}{t}^2$

For the control system , Our task is to stabilize the output at a certain value , For example, we push an object in the hope that it will just stop somewhere , But now $f(t)$ Obviously divergent , That is to say, the system is Unstable , We will talk more about stability later .

Control what needs to be done ：
Of course you want to push the box gently, and finally your strength will return 0, But this system has no friction , therefore x Never stable , This system is simply not stable . So you need Make changes to the system , For example, add friction . And you should also change the way you push boxes , You may need to push quickly, but the box will be unstable , Push slowly for a long time . And these are the things that control has to do .

Amplitude frequency response and phase frequency response

Think about how our robots are controlled ？ Circuit, of course . Generally speaking, the circuit must be AC , That is, the combination of sinusoidal signals with different frequencies （ Of course, any wave can become a combination of sinusoidal signals through Fourier transform , so much the better ）. Experiments and theories tell us that for linear systems , Input sine wave , The output is also a sine wave of the same frequency , What has changed is only Amplitude and phase （ It's easy to understand the second superposition of linear systems ）. that , If we know the response of the system to different frequency signals, we can easily analyze the relationship between input and output ？

Let's change to a more complex system ：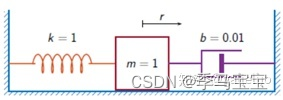

System equations ：
$$m\cdot \frac{d^{2}r}{d{t}^2}+b\cdot \frac{dr}{dt}+k\cdot r=kF(t)$$
Transfer function ：
$$Y(s)=\frac{R(s)}{F(s)}=\frac{k}{m{s}^2+bs+k}=\frac{{\omega }_n^2}{s^2+2{\xi }_{{\omega }_n}s+{\omega }_n^2}$$
among ${\omega }_n=\sqrt{\frac{k}{m}},\xi =\frac{b}{2\sqrt{km}}$
Into the $s=j\omega$, be ：( It's very important here , The Laplace transform after the change is actually The Fourier transform , That is, it is expanded according to the frequency )
$$|Y(j\omega )|=\frac{1}{\sqrt{{\left(1-{\left(\frac{\omega }{{\omega }_n}\right)}^2\right)}^2+4{\xi }^2{\left(\frac{\omega }{{\omega }_n}\right)}^2}},\angle Y(j\omega )=-\mathrm{artan}\frac{2\xi \frac{\omega }{{\omega }_n}}{1-{\left(\frac{\omega }{{\omega }_n}\right)}^2}$$

One of the above two formulas is amplitude , One is phase , For an input frequency , These are two fixed values . Let's draw these two functions .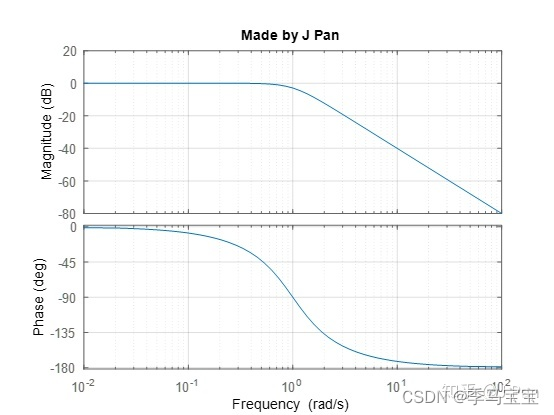

It's on it Amplitude response Set the output ratio input to K, The amplitude is taken 20logK, Because the range of values is very large , This is Porto . When input equals output , Namely 0.
Here is Frequency response , Because it's a causal signal , Always phase lag （ The phase lag in frequency domain is represented by the time delay in time domain , Your state starts with 0 You can't turn into 1 Well , There must be a time difference ）.
We can find that this is a low-pass system , The input signal with low frequency can pass , Those with high frequency basically have no attenuation .
Suppose the input is a step signal ：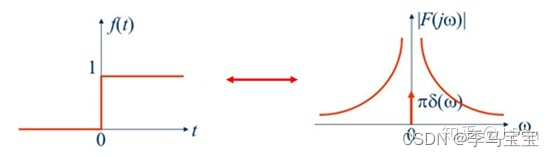

The output looks like this , The low-frequency signal is retained , You can change the system delay time by modifying the system parameters , But overshoot （ Spit out part ） Will increase , How to balance is also what you need to consider when designing the control system ：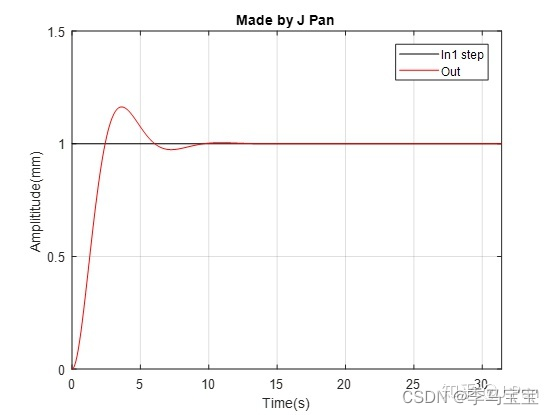

system stability

Pole-Zero ：
$G(s)=\frac{(s-b_1)(s-b_2{)}^2}{(s-a_1)(s-a_2{)}^2}$
$s=b_1$ Is the first order zero ,$s=b_2$ Is the second order zero ,$s=a_1$ Is the pole of the first order ,$s=a_2$ Is the second-order pole
If there is $a_i=b_j$, Then there is zero pole cancellation

Generally speaking , If the poles of the transfer function are all in the left half plane, it is stable , One is unstable in the right half plane . If there are poles at the origin, there will be steady-state components （ unstable ）, There will be a sinusoidal signal on the vertical axis outside the origin ( Critical stability ). Well understood. , The following is the translation property of Laplace transform ：
$$L\left[f(t)e^{-at}\right]=F(s+a)$$
once a<0, The pole of the right half will appear , There will also be instability $e^{|a|t}$ component .

PS： If you study the principle of automatic control , You will learn many ways to judge stability , Because solving equations of very high degree manually （ But now we all use computers , So it's useless to actually use ）. I don't want to spend a lot here , There are many contents in this part, which is of course very important for the exam , But don't go into detail , Just have an understanding first .

System stability and zero pole position ：
The poles of the transfer function represent the mode of the system , in other words , When the frequency of the excitation is close to or the same as the frequency represented by the pole , The system will resonate , The response of the system reaches the maximum .
What does zero mean ？—— Contrary to the pole , Represents the mode that the system can shield , That is, the anti resonance point , let me put it another way , When the frequency of excitation is the same as that represented by zero , The system will have no response or very little response .
Taken together ：** The transient response of the system depends on the mode represented by the pole ; The steady-state response depends on the distance between the excitation frequency and the zero and pole , The closer to the pole , The larger the steady-state response , The closer to zero , The smaller the steady-state response .** This obviously contradicts the idea that the closer we are to the pole, the more unstable we are （ Opposition ）, So we should also make a balance .

So we assign the position of zero and pole , Satisfactory frequency response characteristics can be obtained . For example, we want to amplify a certain frequency （ Bandpass ） Put a pole at that frequency , We want a certain frequency to be suppressed （ Notch filter ） You can put a zero there , See the details later , Need to use feedback .

in addition , There are two points about simplification .
One is to delete too small zeros and poles , It's easy to think of , For example, your output function about time is $x(t)=e^{-x}+e^{-10x}+e^{-100x}$, Obviously your x It has the biggest relationship with the first item , The latter two items can be basically ignored . Generally speaking, small ones can be ignored if there is an order of magnitude difference .
Second, when the zero pole is close enough , It can be cancelled .

In addition, I will not go into detail about the knowledge of minimum phase system , You can see Minimum phase system understanding

feedback

More content , I'll add later , You can look at Refer to Zhihu JPAN
Here I add a little understanding of the examples in the article ：
Suppose the transfer function of a system is ：$G(s)=\frac{1}{s+1}$
The step response of this system is ：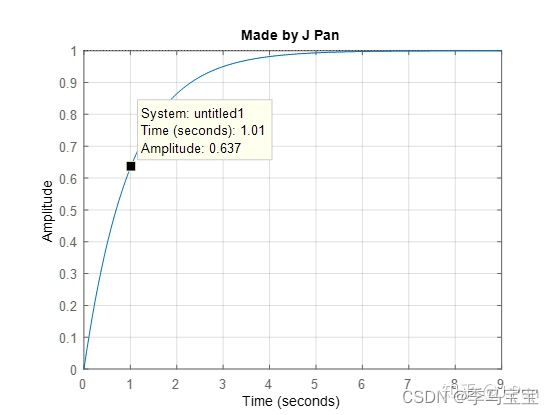

Response to stable value 63.2% The time required is about 1s, Very slowly , The reason is that the cut-off frequency is too low , Its Byrd picture is

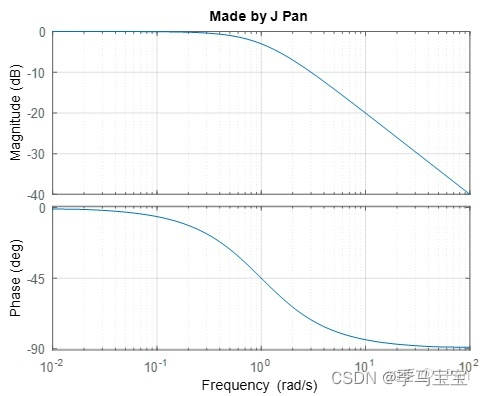
To solve this problem , We use the following results , This is a proportional control . If K It's big , It's equivalent to slamming on the accelerator , It will definitely reach the value we want very soon . But without control , In the end, we will exceed the expected speed , Something is needed to suppress , Negative feedback plays this role , If the output exceeds the input value , Then the excess part will become a reaction force and make your accelerator unable to step down .

When kp take 100 When , The cut-off frequency has increased significantly , Follow the characteristics to get better .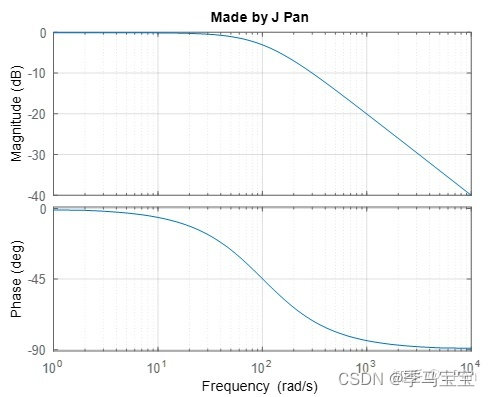

We said earlier , As long as the cut-off frequency is high enough , The output can follow the input very well , This statement is not very rigorous , Because the article said at the beginning , When each frequency component passes through the system , It's not just the amplitude that has attenuation , The phase is also delayed , If the phase delay is too much , After the signal is superimposed again, it may be quite different from the input . Therefore, the rigorous statement is ： When the phase delay is within a certain range , The higher the cut-off frequency , The closer the output is to the input . How to quantify this matter ？—— Phase margin .

Mentioned earlier , Join in PI Behind the controller , The closed-loop transfer function of the system becomes very complex , Existing poles , There is zero again , It's not easy to analyze , therefore , Predecessors in the field of automatic control have come up with an idea , That is to design the cut-off frequency and phase margin of the open-loop front channel first , Then analyze the response of the closed-loop system .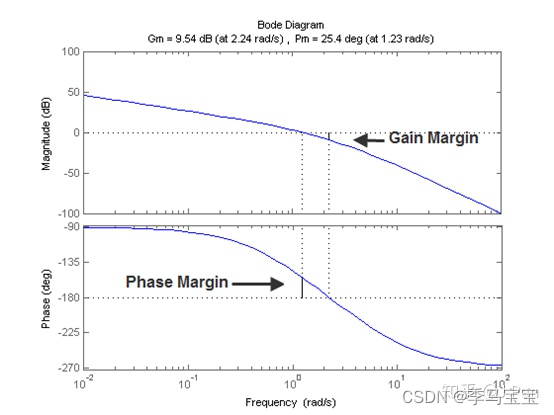

When the corrected system （ Controller and G(s) Open loop forward channel ） When the amplitude response of begins to decay （ The output amplitude is smaller than the input , That is to say 0dB）, The phase delay of the signal is related to -180° The distance is the phase margin . so , The larger the phase margin, the better , Theoretically, if the phase margin reaches 180°, Then there is no phase delay , Of course, in fact, it can't be achieved , Generally speaking, the phase margin is 40° above , Of course, it will be different according to different applications . To sum up ：

The amplitude response of the system can be constrained by the cut-off frequency , Phase response should be constrained by phase margin .