当前位置:网站首页>[technical school] spatial accuracy of binocular stereo vision system: accurate quantitative analysis
[technical school] spatial accuracy of binocular stereo vision system: accurate quantitative analysis
2022-07-05 08:54:00 【Li Yingsong~】
In previous articles 【 Technical genre 】 Teach you to improve the accuracy of binocular stereo vision system ! in , Bloggers made a more specific analysis about the accuracy of stereo vision system , But on the whole, it is qualitative , This article once again makes a quantitative analysis of accuracy , After all, accuracy is always the first factor in most scenes , Quantitative analysis is more conducive to system design and accuracy optimization . Hope to finish this article , Readers can further understand the determinants of accuracy and their respective scales of influence on accuracy , And be able to answer the following three questions :
1. The accuracy of binocular stereo vision system depends on those factors ?
2. Do all factors have the same degree of determination of accuracy ?
3. X/Y/Z Is the accuracy of the three directions the same ? If it's not the same , Which direction has better accuracy ?
List of articles
Most often , The final output of binocular stereo vision is in the left camera coordinate system XYZ coordinate , This paper takes these three components as the object of accuracy analysis .
First , Define some variables :
b b b: Baseline length
f f f: The focal length
x , y x,y x,y : Like point coordinates ( Take the main point of the image as the origin )
X , Y , Z X,Y,Z X,Y,Z: Coordinates in camera coordinate system
d d d: parallax
Let's first look at the solution formula of these three components :
Z = b f d , X = Z f x , Y = Z f y Z=\frac {bf} d,X=\frac Z f x,Y=\frac Z f y Z=dbf,X=fZx,Y=fZy
From the formula , In hardware parameters B , f B,f B,f Fixed case , X Y Z XYZ XYZ The value of is directly related to the coordinate value of the image point , X Y Z XYZ XYZ The accuracy of is actually the spatial deviation value under the image point accuracy , Therefore, we take the accuracy of image points as the basic ( Minimum ) Precision unit .
Suppose the image point x , y x,y x,y The accuracy of is s x , s y s_x,s_y sx,sy, parallax d d d The accuracy of is s d s_d sd. It is commonly believed x , y x,y x,y The accuracy of is the same , namely s x = s y = s s_x=s_y=s sx=sy=s, Generally speaking, the accuracy of parallax can be calculated by formula s d = s x 2 s_d = \frac {s_x} {\sqrt 2} sd=2sx To estimate 1.
Z Directional accuracy
First of all, for Z = b f d Z=\frac {bf} d Z=dbf, The independent variable is d d d, We are right. d d d Finding partial derivatives , Available
∂ Z ∂ d = − b f d 2 = − Z 2 b f \frac {\partial Z} {\partial d}=-\frac {bf} {d^2}=-\frac {Z^2} {bf} ∂d∂Z=−d2bf=−bfZ2
therefore , Z Z Z The accuracy of the direction can be expressed as
s Z = Z 2 b f s d = Z 2 2 b f s s_Z=\frac {Z^2} {bf}s_d=\frac {Z^2} {\sqrt 2bf}s sZ=bfZ2sd=2bfZ2s
You know Z Z Z Direction accuracy and Z Z Z Square positive correlation of , That is, it is positively correlated with the square of the distance between the object and the camera ( Strictly speaking Z Z Z Direction distance ).
meanwhile , The above formula can change one form :
s Z = Z b Z f s d s_Z=\frac {Z} {b}\frac {Z} {f}s_d sZ=bZfZsd
hypothesis q = Z b , m = Z f q=\frac {Z} {b},m=\frac {Z} {f} q=bZ,m=fZ, be q q q Is what we are familiar with Base height ratio Reciprocal , m m m Is the scale of the image ( namely GSD, The space size represented by a pixel ), This formula shows , Base height ratio and GSD Yes Z Z Z Direction accuracy plays a key role , Larger base height ratio and smaller GSD Contribute to Z Z Z Directional accuracy . This can be used to guide the design of binocular stereo vision system .
XY Directional accuracy
Empathy , about X X X, The independent variable is Z Z Z and x x x, We are right. Z Z Z and x x x Finding partial derivatives , Available
∂ X ∂ Z = x f , ∂ X ∂ x = = Z f \frac {\partial X} {\partial Z}=\frac x f,\frac {\partial X} {\partial x}==\frac Z f ∂Z∂X=fx,∂x∂X==fZ
X X X The directional accuracy can be expressed as
s X = ( x f s Z ) 2 + ( Z f s x ) 2 s_X=\sqrt { {(\frac x f s_Z)}^2+{(\frac Z f s_x)}^2} sX=(fxsZ)2+(fZsx)2
Empathy , Y Y Y The directional accuracy can be expressed as
s Y = ( y f s Z ) 2 + ( Z f s y ) 2 s_Y=\sqrt { {(\frac y f s_Z)}^2+{(\frac Z f s_y)}^2} sY=(fysZ)2+(fZsy)2
hold s Z = Z 2 2 b f s , s x = s y = s s_Z=\frac {Z^2} {\sqrt 2bf}s,s_x=s_y=s sZ=2bfZ2s,sx=sy=s Generation into the formula , Available
s X = 1 + ( x Z 2 f b ) 2 Z f s = 1 + ( X 2 b ) 2 Z f s s_X=\sqrt {1+{(\frac {xZ} {\sqrt 2 fb})}^2}\frac Z f s=\sqrt {1+{(\frac X {\sqrt 2 b})}^2}\frac Z f s sX=1+(2fbxZ)2fZs=1+(2bX)2fZs
s Y = 1 + ( y Z 2 f b ) 2 Z f s = 1 + ( Y 2 b ) 2 Z f s s_Y=\sqrt {1+{(\frac {yZ} {\sqrt 2 fb})}^2}\frac Z f s=\sqrt {1+{(\frac Y {\sqrt 2 b})}^2}\frac Z f s sY=1+(2fbyZ)2fZs=1+(2bY)2fZs
It can be seen that , s X s_X sX and s Y s_Y sY It is of equal scale , Combine the previous formula ,
s Z = Z 2 2 b f s s_Z=\frac {Z^2} {\sqrt 2bf}s sZ=2bfZ2s
Get the precision expression of three directions .
X/Z Accuracy curve
In order to observe the three directions more clearly, the accuracy increases with Z Z Z Trend of value change , Let's do a simulation , Suppose the accuracy of the image point is 10 μ m \mu m μm, Calculate and plot Z Z Z and X X X Direction ( Y Y Y Direction and X X X Direction and other scales , The trend is the same ) Accuracy curve .
Simulation data of other parameters :
f = 1100 f = 1100 f=1100 pix
x = 640 x = 640 x=640 pix
b = 200 b = 200 b=200 mm
The accuracy curve drawn is as follows :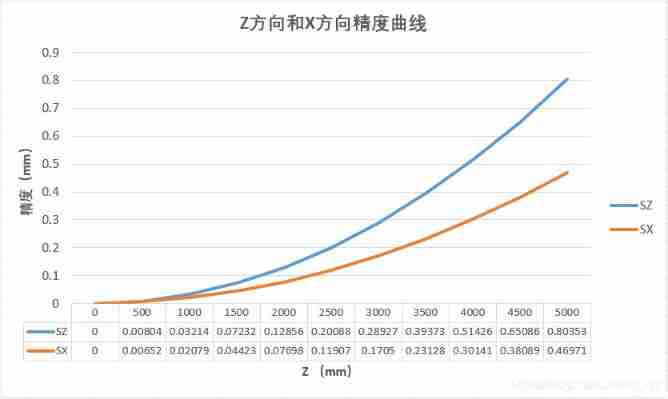
It can be seen from the results of precision calculation and trend chart , The accuracy value increases with Z Z Z It becomes bigger with the increase of ( It means that the accuracy is getting worse ), And when Z Z Z When it's worth more , Z Z Z Directional accuracy ratio X ( Y ) X(Y) X(Y) The direction accuracy is poor , With Z An increase in , The gap is more obvious .
And when Z Z Z It's worth less , Z Z Z On the contrary, the direction accuracy will be better than X Y XY XY The direction is better , We will Z The range of is reduced to 0~500, Draw another curve ,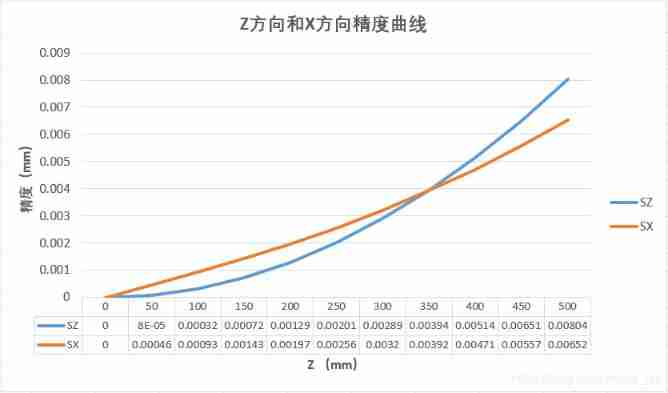
You can see , stay Z Z Z Less than 350mm Left and right , Z Z Z The direction accuracy is better than X X X Direction , This critical point Z Z Z The value is calculated according to the hardware parameters and precision formula , And it is related to the coordinates of image points .
reference
- [1] Walker, Stewart. Close-Range Photogrammetry and 3D Imaging, 2nd Edition[J]. Photogrammetric Engineering & Remote Sensing, 2015.About bloggers :
Ethan Li Li Yingsong ( You know : Li Yingsong )
Wuhan University Doctor of photogrammetry and remote sensing
Main direction Stereo matching 、 Three dimensional reconstruction
2019 Won the first prize of scientific and technological progress in surveying and mapping in ( Provincial and ministerial level )
Love 3D , Love sharing , Love open source
GitHub: https://github.com/ethan-li-coding
Personal wechat :
Welcome to exchange !
Bloggers focus on , More 3D reconstruction knowledge popular science , thank !
Blog home page :https://ethanli.blog.csdn.net
边栏推荐
- Confusing basic concepts member variables local variables global variables
- MPSoC QSPI flash upgrade method
- [daily training] 1200 Minimum absolute difference
- OpenFeign
- 皮尔森相关系数
- Golang foundation - the time data inserted by golang into MySQL is inconsistent with the local time
- My experience from technology to product manager
- Guess riddles (8)
- Ros-11 common visualization tools
- [code practice] [stereo matching series] Classic ad census: (4) cross domain cost aggregation
猜你喜欢
C# LINQ源码分析之Count
Shift operation of complement
![[daiy4] copy of JZ35 complex linked list](/img/bc/ce90bb3cb6f52605255f1d6d6894b0.png)
[daiy4] copy of JZ35 complex linked list

My university
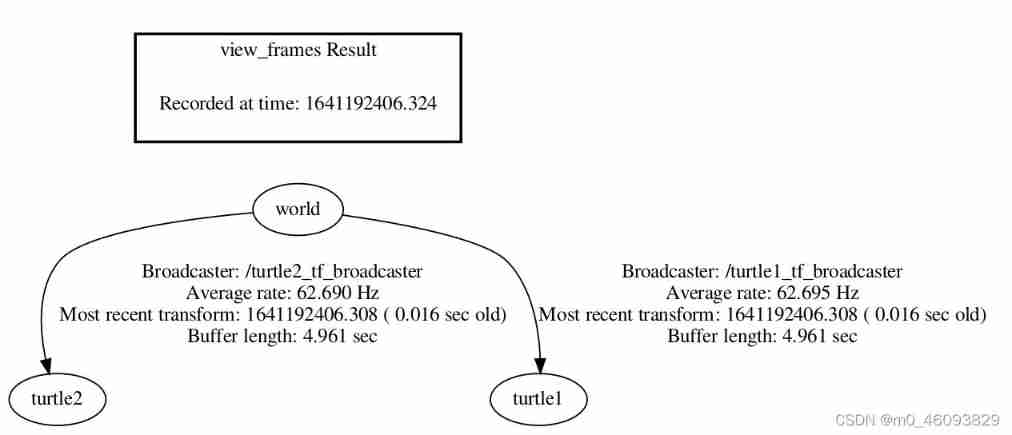
TF coordinate transformation of common components of ros-9 ROS

Guess riddles (8)

AUTOSAR从入门到精通100讲(103)-dbc文件的格式以及创建详解
![[Niuke brush questions day4] jz55 depth of binary tree](/img/f7/ca8ad43b8d9bf13df949b2f00f6d6c.png)
[Niuke brush questions day4] jz55 depth of binary tree
Install the CPU version of tensorflow+cuda+cudnn (ultra detailed)
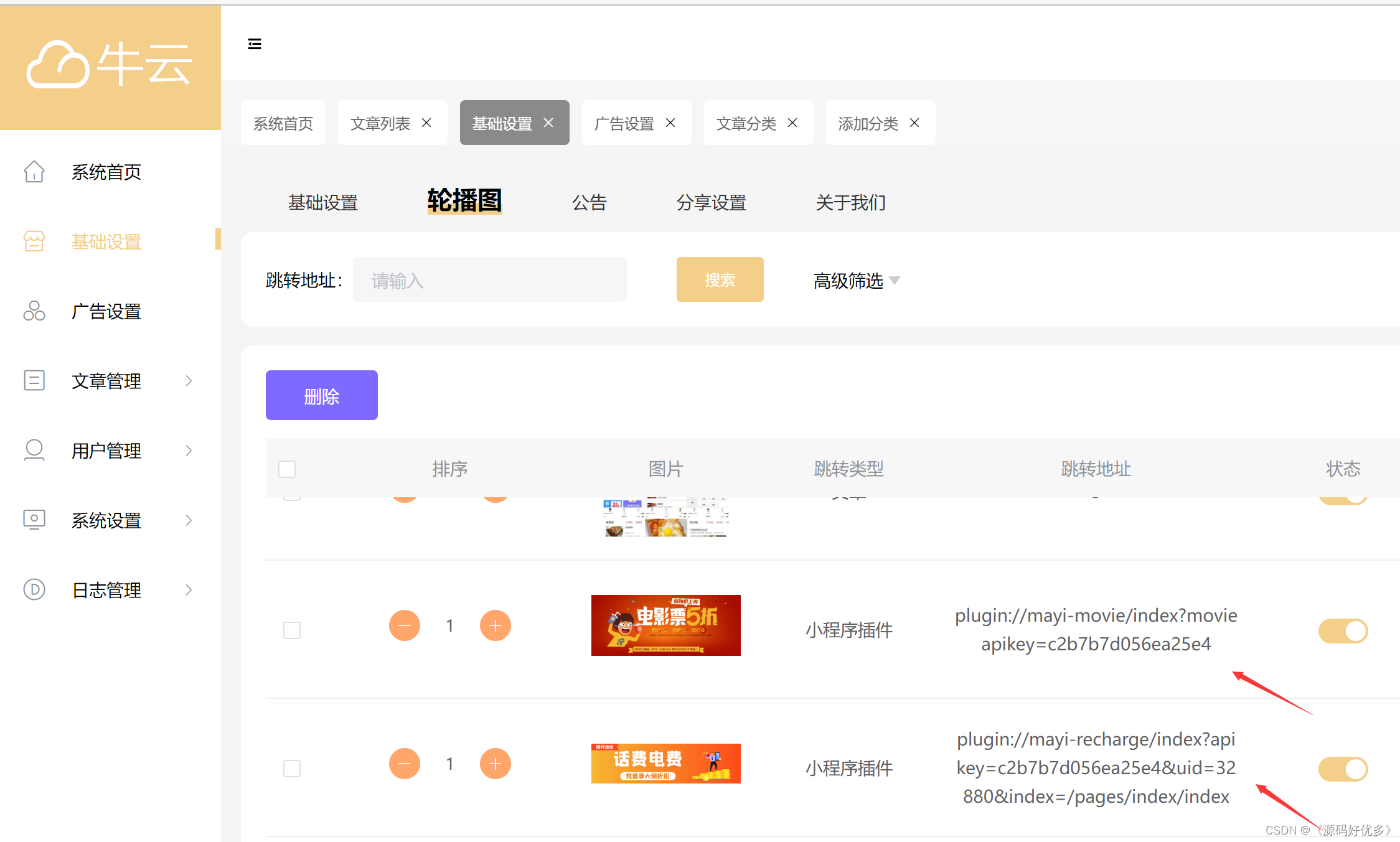
Add discount recharge and discount shadow ticket plug-ins to the resource realization applet
随机推荐
Bit operation related operations
Yolov4 target detection backbone
Digital analog 1: linear programming
容易混淆的基本概念 成员变量 局部变量 全局变量
Use and programming method of ros-8 parameters
File server migration scheme of a company
12、动态链接库,dll
Ros-11 common visualization tools
优先级队列(堆)
My university
Business modeling of software model | overview
Guess riddles (7)
Ecmascript6 introduction and environment construction
Understanding rotation matrix R from the perspective of base transformation
Redis implements a high-performance full-text search engine -- redisearch
Halcon color recognition_ fuses. hdev:classify fuses by color
kubeadm系列-01-preflight究竟有多少check
Golang foundation -- map, array and slice store different types of data
我从技术到产品经理的几点体会
The first week of summer vacation
