当前位置:网站首页>数论函数及其求和 待更新
数论函数及其求和 待更新
2022-07-05 04:43:00 【Strezia】
主要记录一下相关的题目,略过知识讲解。
例题1 数论分块
题目描述
若 x x x 分解质因数结果为 x = p 1 k 1 p 2 k 2 ⋯ p n k n x=p_1^{k_1}p_2^{k_2}\cdots p_n^{k_n} x=p1k1p2k2⋯pnkn,令 f ( x ) = ( k 1 + 1 ) ( k 2 + 1 ) ⋯ ( k n + 1 ) f(x)=(k_1+1)(k_2+1)\cdots (k_n+1) f(x)=(k1+1)(k2+1)⋯(kn+1),求 ∑ i = l r f ( i ) \sum_{i=l}^rf(i) ∑i=lrf(i) 对 998 244 353 998\,244\,353 998244353 取模的结果。
时间复杂度 O ( n ) O(\sqrt n) O(n)。
思路
首先要知道 f ( x ) f(x) f(x) 其实就是 x x x 的约数个数。(每种素因子都有 k i k_i ki 个那么可以取 0 , 1 , . . . , k i 0,1,...,k_i 0,1,...,ki 一共 k i + 1 k_i+1 ki+1 种取法排列组合,由唯一分解定理显然乘积都是不同的)因为题目要求的是区间和,于是正常地转化为前缀和形式 a n s = ∑ i = 1 r f ( x ) − ∑ i = 1 l − 1 f ( x ) ans=\sum_{i=1}^rf(x)-\sum_{i=1}^{l-1}f(x) ans=∑i=1rf(x)−∑i=1l−1f(x) ,设 S ( n ) = ∑ i = 1 n f ( x ) S(n) = \sum_{i=1}^nf(x) S(n)=∑i=1nf(x),问题转化为了求 S ( n ) S(n) S(n) ,也就是求 1 − n 1-n 1−n 这 n n n 个数的因数个数之和。
1 − n 1-n 1−n 中含有因子 i i i 的数一共有 ⌊ n i ⌋ \lfloor\frac ni\rfloor ⌊in⌋ ,直接用数论分块即可。
代码
int ll, rr, n;
int cal(int l, int r) {
int ansr = 0, ansl = 0;
n = rr;
for(int l=1,r;l<=n;l=r+1){
r=n/(n/l);
ansr+=(n/l)*(r-l+1) % P;
ansr %= P;
}
n = ll;
for(int l=1,r;l<=n;l=r+1){
r=n/(n/l);
ansl+=(n/l)*(r-l+1) % P;
ansl %= P;
}
ansr = (ansr - ansl + P) % P;
return ansr;
}
void solve() {
cin >> ll >> rr;
ll--;
cout << cal(ll, rr) << endl;
}
例题2 数论分块
题目描述
给定一个整数 n n n,你需要求出 ∑ i = 1 n gcd ( i , n ) \sum\limits_{i=1}^n \gcd(i, n) i=1∑ngcd(i,n), n < 1 0 9 n<10^9 n<109
思路
注意到 g c d ( i , n ) gcd(i,n) gcd(i,n) 的取值最多只有 n n n 的约数个,所以考虑按取值分类,而且出现 g c d gcd gcd 的题往往都需要想办法凑出 g c d ( i , j ) = 1 gcd(i,j)=1 gcd(i,j)=1,于是我们把原式化为 ∑ d ∣ n d ∑ i = 1 n [ g c d ( i , n ) = d ] = ∑ d ∣ n d ∑ i = 1 n d [ g c d ( i , n d ) = 1 ] = ∑ d ∣ n d ϕ ( n d ) \sum_{d|n}d\sum_{i=1}^n[gcd(i,n)=d ]=\sum_{d|n}d\sum_{i=1}^{\frac nd}[gcd(i,\frac nd)=1]=\sum_{d|n}d\phi(\frac nd) d∣n∑di=1∑n[gcd(i,n)=d]=d∣n∑di=1∑dn[gcd(i,dn)=1]=d∣n∑dϕ(dn)
可以通过枚举 n n n 的约数直接求,时间复杂度 O ( n ) O(\sqrt n) O(n)。
代码
void solve() {
cin >> n;
ll ans = 0;
for(ll i = 1; i * i <= n; i++) {
if(n % i == 0) {
ans += i * phi(n/i);
if(n/i!=i) {
ans += (n/i) * phi(i);
}
}
}
cout << ans << endl;
}
莫比乌斯函数
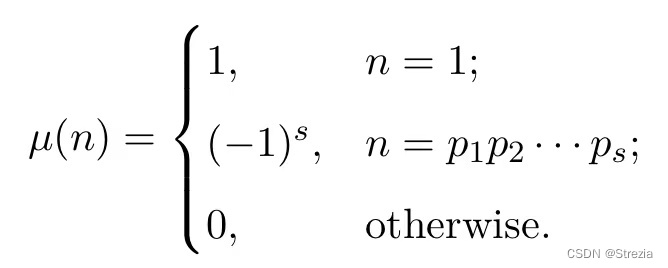
常用卷积
ϵ = μ ∗ 1 , I d = ϕ ∗ 1 \epsilon=\mu *1, Id=\phi*1 ϵ=μ∗1,Id=ϕ∗1 。
即 ϵ ( n ) = ∑ d ∣ n μ ( d ) \epsilon(n) = \sum_{d|n}\mu(d) ϵ(n)=∑d∣nμ(d) 。其中 ϵ ( n ) = 1 \epsilon(n)= 1 ϵ(n)=1 当且仅当 n = 1 n=1 n=1,否则 = 0 =0 =0 。
引例
给定 n , m n, m n,m,求二元组 ( x , y ) (x,y) (x,y) 的个数,满足 1 ≤ x ≤ n 1\leq x\leq n 1≤x≤n , 1 ≤ y ≤ m 1\leq y\leq m 1≤y≤m ,且 g c d ( x , y ) = 1 gcd(x,y)=1 gcd(x,y)=1 。 n , m ≤ 1 0 7 n,m\leq 10^7 n,m≤107 ,至多 1 0 4 10^4 104 组数据。
思路
∑ x = 1 n ∑ y = 1 m [ g c d ( x , y ) = 1 ] = ∑ x = 1 n ∑ y = 1 m ϵ ( g c d ( x , y ) ) = ∑ x = 1 n ∑ y = 1 m ∑ d ∣ g c d ( x , y ) μ ( d ) \sum_{x=1}^n\sum_{y=1}^m[gcd(x,y)=1]=\sum_{x=1}^n\sum_{y=1}^m\epsilon (gcd(x,y))=\sum_{x=1}^n\sum_{y=1}^m\sum_{d|gcd(x,y)}\mu(d) x=1∑ny=1∑m[gcd(x,y)=1]=x=1∑ny=1∑mϵ(gcd(x,y))=x=1∑ny=1∑md∣gcd(x,y)∑μ(d)
= ∑ x = 1 n ∑ y = 1 m ∑ d ∣ x , d ∣ y μ ( d ) = = ∑ d = 1 m i n ( n , m ) μ ( d ) ⌊ n d ⌋ ⌊ m d ⌋ =\sum_{x=1}^n\sum_{y=1}^m\sum_{d|x,d|y}\mu(d)==\sum_{d=1}^{min(n,m)}\mu(d)\lfloor\frac nd\rfloor\lfloor\frac md\rfloor =x=1∑ny=1∑md∣x,d∣y∑μ(d)==d=1∑min(n,m)μ(d)⌊dn⌋⌊dm⌋
对上式预处理 μ \mu μ 及其前缀和,按 ⌊ n d ⌋ ⌊ m d ⌋ \lfloor\frac nd\rfloor\lfloor\frac md\rfloor ⌊dn⌋⌊dm⌋ 的取值分段,就可以每组询问 O ( n ) O(\sqrt n) O(n) 解决问题。
例题3 莫比乌斯反演
题目描述
设 d ( x ) d(x) d(x) 为 x x x 的约数个数,给定 n , m n,m n,m,求
∑ i = 1 n ∑ j = 1 m d ( i j ) \sum_{i=1}^n\sum_{j=1}^md(ij) i=1∑nj=1∑md(ij)
【数据范围】
对于 100 % 100\% 100% 的数据, 1 ≤ T , n , m ≤ 50000 1\le T,n,m \le 50000 1≤T,n,m≤50000。
思路
首先有结论:
d ( i j ) = ∑ x ∣ i ∑ y ∣ j [ g c d ( x , y ) = 1 ] d(ij)=\sum_{x|i}\sum_{y|j}[gcd(x,y)=1] d(ij)=x∣i∑y∣j∑[gcd(x,y)=1]
则原式:
∑ i = 1 n ∑ j = 1 m d ( i j ) = ∑ i = 1 n ∑ j = 1 m ∑ x ∣ i ∑ y ∣ j [ g c d ( x , y ) = 1 ] = ∑ x = 1 n ∑ y = 1 m [ g c d ( x , y ) = 1 ] ⌊ n x ⌋ ⌊ m y ⌋ \sum_{i=1}^n\sum_{j=1}^md(ij)=\sum_{i=1}^n\sum_{j=1}^m\sum_{x|i}\sum_{y|j}[gcd(x,y)=1]=\sum_{x=1}^n\sum_{y=1}^m[gcd(x,y)=1]\lfloor\frac nx\rfloor\lfloor\frac my\rfloor i=1∑nj=1∑md(ij)=i=1∑nj=1∑mx∣i∑y∣j∑[gcd(x,y)=1]=x=1∑ny=1∑m[gcd(x,y)=1]⌊xn⌋⌊ym⌋
将引例中结论带入:
= ∑ d = 1 m i n ( n , m ) μ ( d ) ∑ x = 1 ⌊ n d ⌋ ∑ y = 1 ⌊ m d ⌋ ⌊ n x d ⌋ ⌊ m y d ⌋ = ∑ d = 1 m i n ( n , m ) μ ( d ) ∑ x = 1 ⌊ n d ⌋ ∑ y = 1 ⌊ m d ⌋ ⌊ ⌊ n d ⌋ x ⌋ ⌊ ⌊ m d ⌋ y ⌋ =\sum_{d=1}^{min(n,m)}\mu(d)\sum_{x=1}^{\lfloor\frac nd\rfloor}\sum_{y=1}^{\lfloor\frac md \rfloor}\lfloor\frac n{xd}\rfloor\lfloor\frac m{yd}\rfloor=\sum_{d=1}^{min(n,m)}\mu(d)\sum_{x=1}^{\lfloor\frac nd\rfloor}\sum_{y=1}^{\lfloor\frac md \rfloor}\lfloor\frac{\lfloor\frac nd\rfloor}x\rfloor\lfloor\frac {\lfloor \frac md\rfloor}y\rfloor =d=1∑min(n,m)μ(d)x=1∑⌊dn⌋y=1∑⌊dm⌋⌊xdn⌋⌊ydm⌋=d=1∑min(n,m)μ(d)x=1∑⌊dn⌋y=1∑⌊dm⌋⌊x⌊dn⌋⌋⌊y⌊dm⌋⌋
令 f ( n ) = ∑ i = 1 n ⌊ n i ⌋ f(n)=\sum_{i=1}^n\lfloor\frac ni\rfloor f(n)=∑i=1n⌊in⌋ ,则
= ∑ d = 1 m i n ( n , m ) μ ( d ) f ( ⌊ n d ⌋ ) f ( ⌊ m d ⌋ ) =\sum_{d=1}^{min(n, m)}\mu(d)f(\lfloor\frac nd\rfloor)f(\lfloor\frac md\rfloor) =d=1∑min(n,m)μ(d)f(⌊dn⌋)f(⌊dm⌋)
又 f ( n ) = ∑ i = 1 n ⌊ n i ⌋ = ∑ k = 1 n d ( k ) f(n)=\sum_{i=1}^n\lfloor\frac ni\rfloor=\sum_{k=1}^nd(k) f(n)=∑i=1n⌊in⌋=∑k=1nd(k)
故可以通过欧拉筛 O ( n ) O(n) O(n) 预处理出 d ( n ) d(n) d(n),通过前缀和得到 f ( n ) f(n) f(n),可以每次询问 O ( n ) O(\sqrt n) O(n) 回答。
代码
int mu[maxn], prim[maxn], sum[maxn], cnt;
int g[maxn];
bool vis[maxn];
void get_mu(int n)
{
mu[1]=1;
for(int i=2;i<=n;i++)
{
if(!vis[i]){
prim[++cnt]=i;mu[i]=-1;}
for(int j=1;j<=cnt&&prim[j]*i<=n;j++)
{
vis[prim[j]*i]=1;
if(i%prim[j]==0)break;
else mu[i*prim[j]]=-mu[i];
}
}
for(int i=1;i<=n;i++)sum[i]=sum[i-1]+mu[i];
for(int i=1;i<=n;i++)
{
long long ans=0;
for(int l=1,r;l<=i;l=r+1)
{
r=(i/(i/l));
ans+=1ll*(r-l+1)*1ll*(i/l);
}
g[i]=ans;
}
}
void solve() {
int n, m;
cin >> n >> m;
int ans = 0;
int mn = min(n, m);
for(int l = 1, r; l <= mn; l = r + 1){
r = min(n/(n/l), m/(m/l));
ans += (sum[r] - sum[l-1]) * g[n/l] * g[m/l];
}
cout << ans << endl;
}
边栏推荐
- Moco is not suitable for target detection? MsrA proposes object level comparative learning target detection pre training method SOCO! Performance SOTA! (NeurIPS 2021)...
- [phantom engine UE] the difference between running and starting, and the analysis of common problems
- Aperçu en direct | Services de conteneurs ACK flexible Prediction Best Practices
- level17
- Pointer function (basic)
- File upload bypass summary (upload labs 21 customs clearance tutorial attached)
- The 22nd Spring Festival Gala, an immersive stage for the yuan universe to shine into reality
- Stage experience
- 2022-2028 global and Chinese video coding and transcoding Market Research Report
- How to carry out "small step reconstruction"?
猜你喜欢
Raki's notes on reading paper: soft gazetteers for low resource named entity recognition
函數(易錯)
level18
![[groovy] closure (Introduction to closure class closure | this, owner, delegate member assignment and source code analysis)](/img/aa/3c8b7b27e322417777d1315b9a5a8f.jpg)
[groovy] closure (Introduction to closure class closure | this, owner, delegate member assignment and source code analysis)
![[popular science] basic knowledge of thermal design: heat dissipation analysis of 5g optical devices](/img/45/380e739f5eed33626c363756f814d3.png)
[popular science] basic knowledge of thermal design: heat dissipation analysis of 5g optical devices

Web开发人员应该养成的10个编程习惯
电源管理总线 (PMBus)
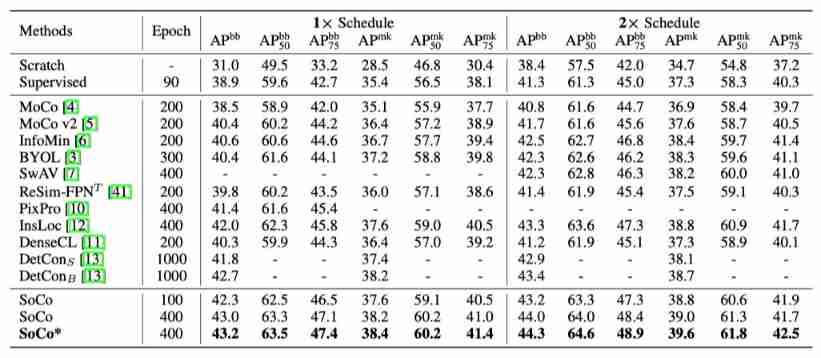
Moco is not suitable for target detection? MsrA proposes object level comparative learning target detection pre training method SOCO! Performance SOTA! (NeurIPS 2021)...

Power management bus (pmbus)
![[phantom engine UE] the difference between running and starting, and the analysis of common problems](/img/e2/49d6c4777c12e9f4e3f8b6ca6db41c.png)
[phantom engine UE] the difference between running and starting, and the analysis of common problems
随机推荐
Rk3399 platform development series explanation (network debugging) 7.29 summary of network performance tools
如何进行「小步重构」?
揭秘技术 Leader 必备的七大清奇脑回路
Managed service network: application architecture evolution in the cloud native Era
windows下Redis-cluster集群搭建
History of web page requests
直播預告 | 容器服務 ACK 彈性預測最佳實踐
Error statuslogger log4j2 could not find a logging implementation
【acwing】240. food chain
Inline built-in function
Is $20billion a little less? Cisco is interested in Splunk?
[goweb development] Introduction to authentication modes based on cookies, sessions and JWT tokens
2022-2028 global and Chinese video coding and transcoding Market Research Report
Label exchange experiment
Group counting notes (1) - check code, original complement multiplication and division calculation, floating point calculation
A survey of automatic speech recognition (ASR) research
2021 higher education social cup mathematical modeling national tournament ABCD questions - problem solving ideas - Mathematical Modeling
Is there a sudden failure on the line? How to make emergency diagnosis, troubleshooting and recovery
[AI bulletin 20220211] the hard core up owner has built a lidar and detailed AI accelerator
CUDA Programming atomic operation atomicadd reports error err:msb3721, return code 1