当前位置:网站首页>F - true liars (category and search set +dp)
F - true liars (category and search set +dp)
2022-07-06 06:14:00 【RCyyds】
Portal : True Liars


The question : give n To the relationship ,p1 I'm a good man ,p2 I'm a bad guy . Requirements according to n Find out what good people are in the relationship , If the scheme is unique , Then output good people one by one , The final output end; If the scheme is not unique / Can't find , Then output no
Ideas : It's the first time to encounter this type of problem , After thinking for a long time, I have no idea , Refer to others' blogs Just understand .
The following is the idea of the referenced blog :
Through simple analysis, we can get , For each relationship (x,y,yes/no), If the relationship is yes, be x and y Belong to the same category ; If the relationship is no, be x and y Belong to the opposite class .
According to the preliminary n To the relationship , We can only introduce those who belong to the same category , Which people belong to the opposite category , But it's not sure what kind of good people are / The bad guys . We can define the opposite two kinds of people as a large set , Then there are two small sets in the large set . According to the meaning , We just need from each large set , Choose one of the small sets , Make the total number of people in all the selected small sets be p1 that will do .
Code :
#include <cstdio>
#include <cstring>
#include <iostream>
#define MAXN 600
using namespace std;
int parent[MAXN], path[MAXN][MAXN], dp[MAXN][MAXN];
int num[MAXN]; // Record that the root node of the large set is i Yes, it is num[i] A large collection
int flag[MAXN][2]; // Mark the small set selected to the final result in each large set
int cnt[MAXN][2]; // The root node is i when , Record the number of people contained in the two small sets
int relat[MAXN];
/* relation
* relat=0/1.0 Express i And root nodes parent[i] Related to , That is, it belongs to the same small set ;1 Express i Not associated with root node , That is, different small sets belonging to the same large set */
int find(int x) {
if (x != parent[x]) { // Here parent[x] It's the old father
int px = find(parent[x]); // px It's the new father
relat[x] ^= relat[parent[x]];
/* Due to path compression x The parent node of points directly to the root node , That is, it changes x The father node of , So update the node x Relationship with root node .
*/
parent[x] = px;
}
return parent[x];
}
/*
about "relat[x] ^= relat[parent[x]];" XOR interpretation :
It can be concluded through simple analysis :
1. If ( relation[x And old father ]=0 && relation[ Old father and new father ]=0 ) perhaps (
relation[x And old father ]=1 && relation[ Old father and new father ]=1 ), be relation[x And the new father ]=0
2. If (relation[x And old father ]=1 && relation[ Old father and new father ]=0 ) perhaps (
relation[x And old father ]=0 && relation[ Old father and new father ]=1 ), be relation[x And the new father ]=1
You can find , Final relatino[x And the new father ] = relation[x And old father ] ^ relation[ Old father and new father ]
namely : relat[x] ^= relat[parent[x]];
For the following merge XOR in function is the same
*/
void merge(int x, int y, int d) { // d Either for 0, Either for 1
int px = find(x);
int py = find(y);
if (px != py) {
parent[py] = px;
relat[py] = relat[x] ^ relat[y] ^ d;
}
}
int main(void) {
int m, p, q;
while (scanf("%d%d%d", &m, &p, &q)) {
if (m + p + q == 0) {
break;
}
for (int i = 1; i <= p + q; i++) { //** Initial remarks
relat[i] = 0;
parent[i] = i;
}
while (m--) {
int x, y, d = 1;
char ch[5];
scanf("%d%d%s", &x, &y, ch);
if (ch[0] == 'y') {
d = 0;
}
merge(x, y, d);
}
memset(num, 0, sizeof(num)); //**num Store the number of sets and number them
memset(path, 0, sizeof(path));
memset(cnt, 0, sizeof(cnt));
memset(dp, 0, sizeof(dp));
memset(flag, 0, sizeof(flag));
int tot = 0;
for (int i = 1; i <= p + q; i++) { //** Count the number of sets and number
if (find(i) == i) {
num[i] = ++tot; // tot Number of connected blocks ,num Record root=i The number of the connected block of
}
}
for (int i = 1; i <= p + q;
i++) { //** Count the number of two types of each set and store them in cnt in
cnt[num[find(i)]][relat[i]]++;
}
dp[0][0] = 1;
for (int i = 1; i <= tot; i++) { // tot=2
for (int j = 0; j <= p+q; j++) { // p+q=7
//**dp[i][j] Store to page i Select the kind and for sets j The number of ways
if (j - cnt[i][0] >= 0 && dp[i - 1][j - cnt[i][0]]) {
dp[i][j] += dp[i - 1][j - cnt[i][0]];
path[i][j] =
cnt[i][0]; //**path Array record path , That is 1 still 0
}
if (j - cnt[i][1] >= 0 && dp[i - 1][j - cnt[i][1]]) {
dp[i][j] += dp[i - 1][j - cnt[i][1]];
path[i][j] = cnt[i][1];
}
}
}
/*
There are two in this part if It's a little hard to understand , Because you may think , Two if If you are satisfied , So the final result is dp[i][j] It is possible that two small sets are selected from the same large set , It's against “ Select only one small set from a large set ” The situation of . but , You can find , We finally limited the number of methods to dp[tot][p]=1 when , There is only one plan . If it's like the two just mentioned if All satisfied with , that dp[i][j] A certain >1, It will not meet the following dp[tot][p]=1 Limit , So it solved the problem .
*/
if (dp[tot][p] != 1) {
printf("no\n");
} else {
for (int i = tot, j = p; j > 0 && i > 0; i--) { //** Tag path
if (path[i][j] == cnt[i][0]) {
flag[i][0] = 1;
} else {
flag[i][1] = 1;
}
j -= path[i][j];
// Remember to reduce . Because I just choose enough backpacks p, So follow p Gradually reduce the items at that time , Find the way
}
for (int i = 1; i <= p + q; i++) {
if (flag[num[find(i)]][relat[i]]) {
printf("%d\n", i);
}
}
printf("end\n");
}
}
return 0;
}
边栏推荐
- D - How Many Answers Are Wrong
- Software test interview questions - Test Type
- RestTemplate、Feign实现Token传递
- Postman核心功能解析-参数化和测试报告
- Title 1093: character reverse order
- 【Postman】动态变量(也称Mock函数)
- LeetCode 732. 我的日程安排表 III
- 公司视频加速播放
- The ECU of 21 Audi q5l 45tfsi brushes is upgraded to master special adjustment, and the horsepower is safely and stably increased to 305 horsepower
- 【Tera Term】黑猫带你学TTL脚本——嵌入式开发中串口自动化神技能
猜你喜欢

Investment strategy discussion and market scale prediction report of China's solid state high power amplifier industry from 2022 to 2028
VINS-Mono: A Robust and Versatile Monocular Visual-Inertial State Estimator
![[postman] collections - run the imported data file of the configuration](/img/85/7ac9976fb09c465c88f376b2446517.png)
[postman] collections - run the imported data file of the configuration
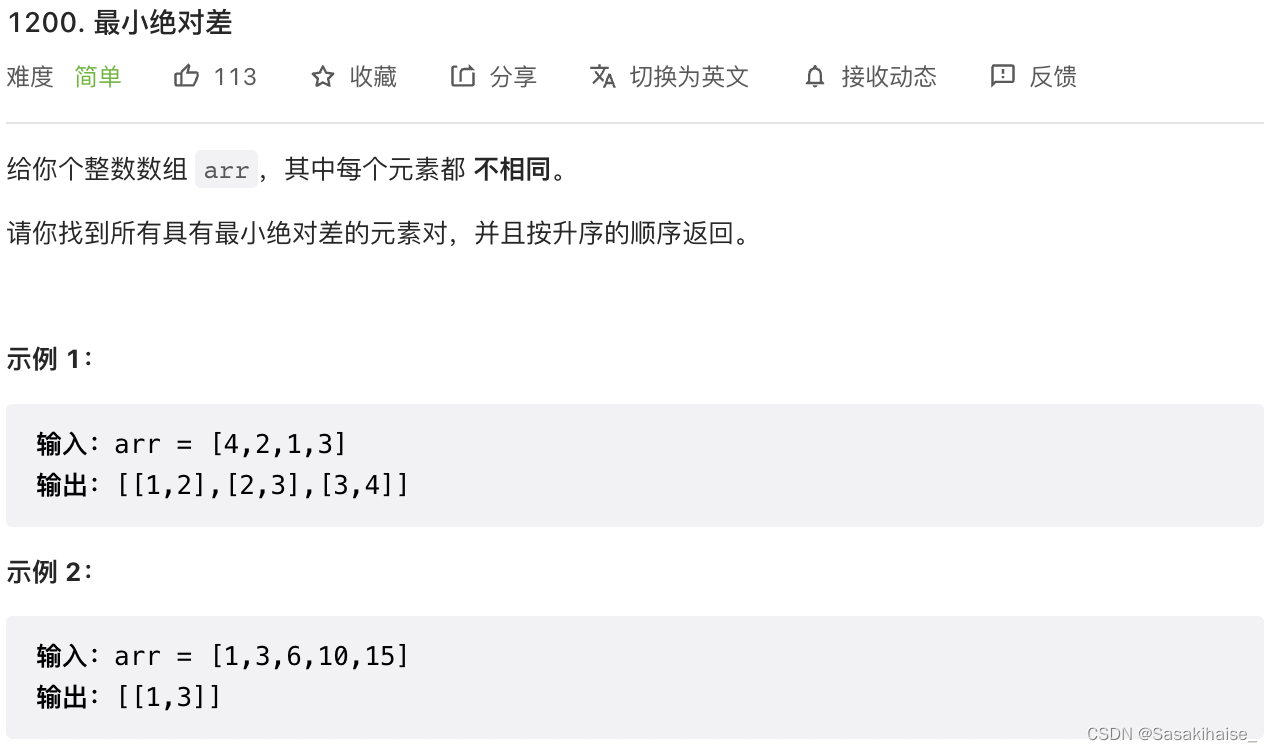
LeetCode 1200. 最小绝对差
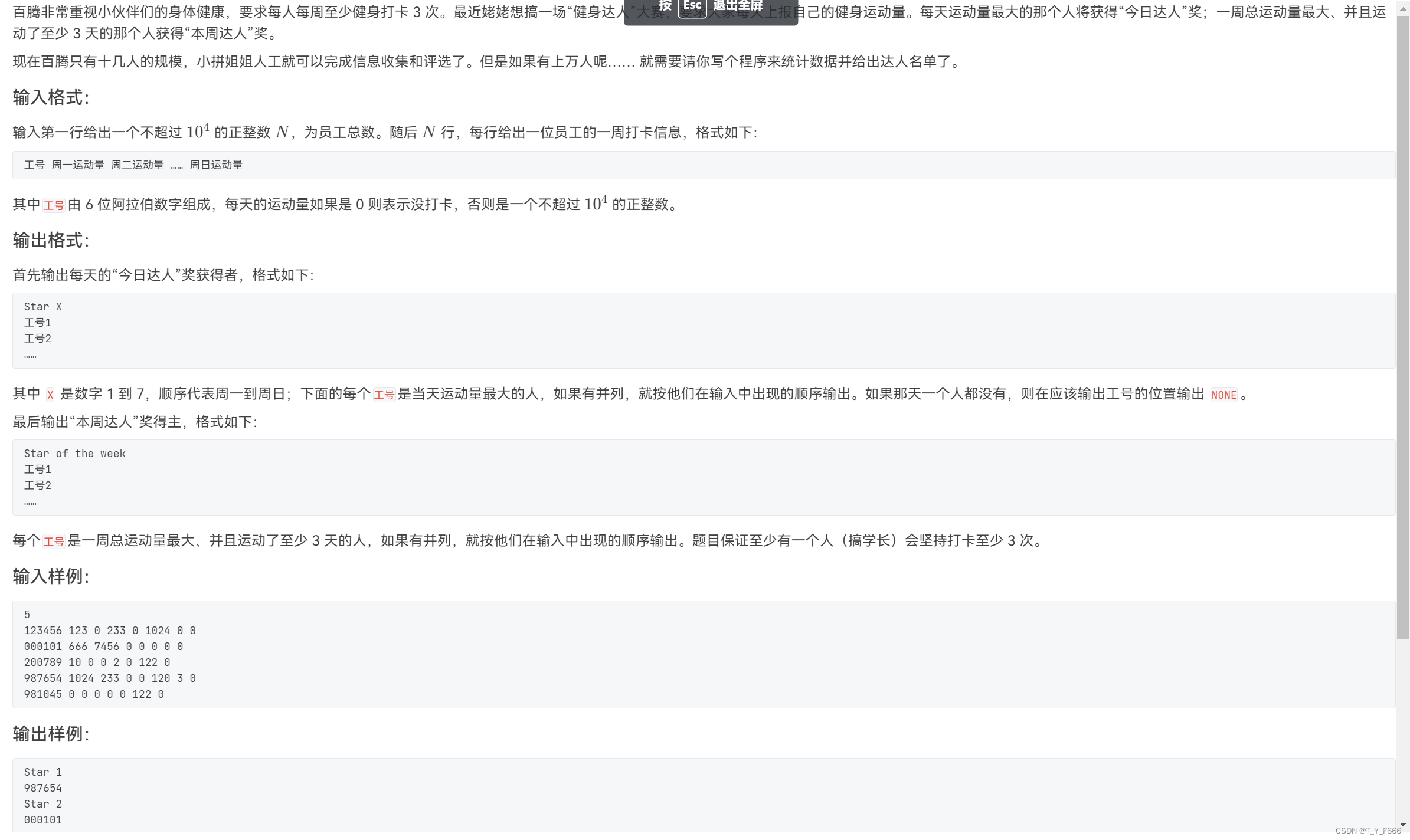
Pat (Grade B) 2022 summer exam
isam2运行流程
全程实现单点登录功能和请求被取消报错“cancelToken“ of undefined的解决方法
ESP32 ESP-IDF看门狗TWDT
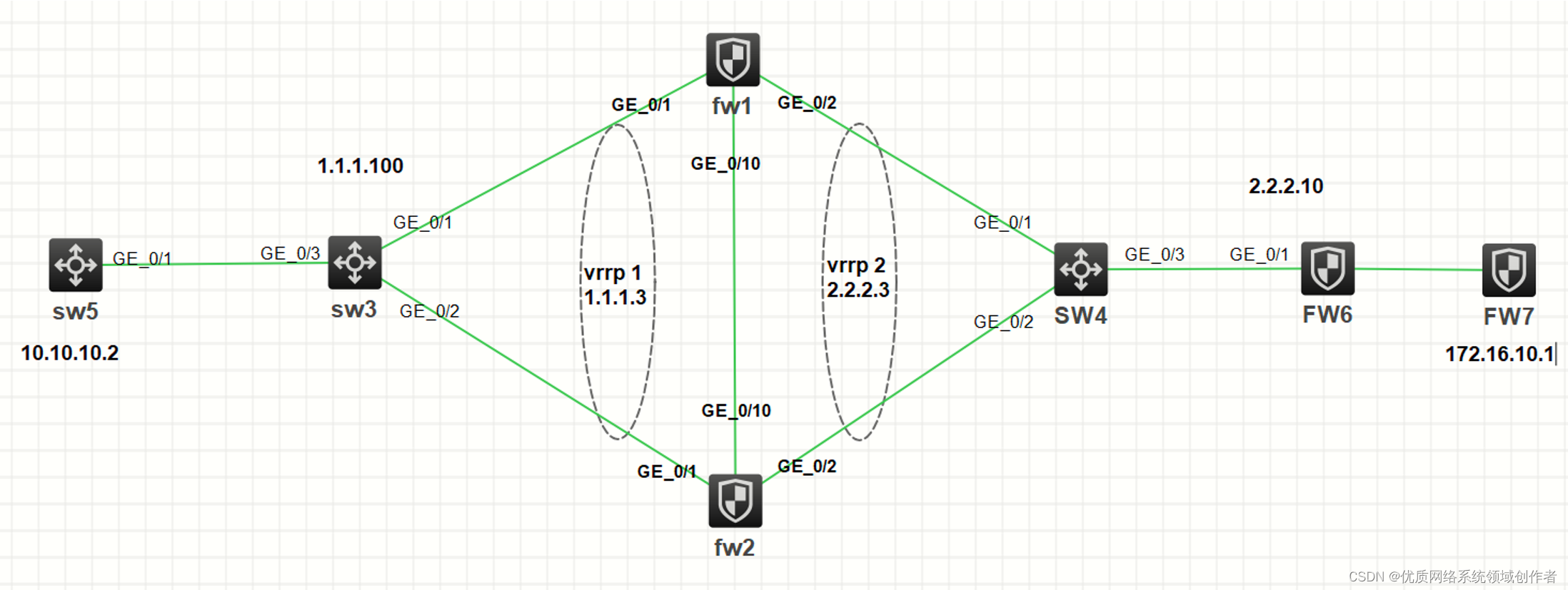
H3C firewall rbm+vrrp networking configuration

二维码的前世今生 与 六大测试点梳理
随机推荐
Buuctf-[bjdctf2020]zjctf, but so (xiaoyute detailed explanation)
Configuring OSPF GR features for Huawei devices
IP day 16 VLAN MPLS configuration
Fault, error, failure of functional safety
单元测试的意义
【C语言】字符串左旋
【LeetCode】Day96-第一个唯一字符&赎金信&字母异位词
SQLMAP使用教程(三)实战技巧二
H3C S5820V2_ Upgrade method after stacking IRF2 of 5830v2 switch
【Postman】动态变量(也称Mock函数)
[leetcode] day96 - the first unique character & ransom letter & letter ectopic word
LeetCode 732. 我的日程安排表 III
Web界面元素的测试
功能安全之故障(fault),错误(error),失效(failure)
對數據安全的思考(轉載)
黑猫带你学UFS协议第8篇:UFS初始化详解(Boot Operation)
selenium源码通读·9 |DesiredCapabilities类分析
Basic knowledge of error
Caused by:org.gradle.api.internal.plugins . PluginApplicationException: Failed to apply plugin
[C language] qsort function