当前位置:网站首页>Path problem before dynamic planning
Path problem before dynamic planning
2022-07-06 15:39:00 【Xiaogeng who loves learning】
Path problem before Dynamic Planning
routing problem : This chapter only talks about different paths (Leecode 62)、 Different paths (Leecode 63)、 Paths and issues (Leedcode 64)
Tips : After writing the article , Directories can be generated automatically , How to generate it, please refer to the help document on the right
List of articles
Preface
Path problem is the prequel of knapsack problem as a classical dynamic programming problem , For understanding dp The thought model of dynamic programming .
One 、 Different path problems (Leecode 62 secondary )
1. Problem description
A robot is in a m x n The top left corner of the grid ( The starting point is marked as “Start” ). The robot can only move down or right one step at a time . The robot tries to reach the bottom right of the grid ( In the figure below, it is marked as “Finish” ). Ask how many different paths there are in total ?
2. Problem analysis
In fact, dynamic problems only need to consider how to define the data structure 、 The meaning of structure 、 Establishment of state transition equation .
2.1 Define the data structure
The question is how many different paths , In this case, you should use dp[m-1][n-1] To express the final result , The example in the last blog is the longest palindrome string , Namely dp[i][j] To express from i To j String ,leedcode 678 The longest increasing subsequence of can be used dp[i] To express , Because it's continuous .
2.2 Interpretation of structural meaning
In this question dp[i][j] It can be interpreted as :i Represents the row of the map ,j Representative column
In the longest palindrome string dp[i][j]:i Is the starting point of palindrome string idx,j Is the end of the palindrome string
The longest increasing subsequence :dp[i] Is the end of the subsequence idx, Because the starting point is the end of the last cycle , So you don't need to define it as a two-dimensional array , Defined as a two-dimensional array to traverse will exceed the time limit , It takes too long
2.3 Establishment of state transition equation
In this question :
Due to the limitation of the topic, we can only go down or to the right Move , So we follow 「 Current optional direction 」 Analyze :
Current location can only 「 Down 」 Move , That is to say dp[i][j] = dp[i-1][j]
Current location can only 「 To the right 」 Move , That is to say dp[i][j] = dp[i][j-1]
The current position can 「 Down 」 Can also 「 To the right 」 Move , That is to say dp[i][j] =dp[i][j-1] + dp[i-1][j]
Derivation process :
dp[i][j] It represents the arrival map grid[i][j] The number of points reaching the path , Then step up : How to get there grid[i][j] spot , There are only two ways : Enter this point from the front point down or to the right , So arrive at grid[i][j] The only way is to arrive dp[i][j-1] The way + arrive dp[i-1][j] The way , From this we can deduce dp[i][j] State transfer equation of :dp[i][j] =dp[i][j-1] + dp[i-1][j]
3. Code display :
The code is as follows ( Example ):
class Solution {
public:
int uniquePaths(int m, int n) {
vector<vector<int>> dp(m, vector<int>(n));
dp[0][0]=1;
for(int i=0;i<m;i++)
{
for(int j=0;j<n;j++)
{
if(i>0&&j>0)
{
dp[i][j]=dp[i-1][j]+dp[i][j-1];
}
else if(i>0)
{
dp[i][j]=dp[i-1][j];
}
else if(j>0){
dp[i][j]=dp[i][j-1];
}
}
}
return dp[m-1][n-1];
}
};
Two 、 Different path problems ll(Leecode 63 secondary )
1. Problem description
This is a LeetCode Upper 「63. Different paths II」, The difficulty is Medium.
A robot is in a m x n The top left corner of the grid ( The starting point is marked as “Start” ). The robot can only move down or right one step at a time . The robot tries to reach the bottom right corner of the grid ( In the figure below, it is marked as “Finish”). Now consider the obstacles in the grid . So how many different paths will there be from the top left corner to the bottom right corner ? Obstacles and empty positions in the grid are used respectively 1 and 0 To express .
2. Problem analysis
In fact, dynamic problems only need to consider how to define the data structure 、 The meaning of structure 、 Establishment of state transition equation .
2.1 Define the data structure
The question is how many different paths , In this case, you should use dp[m-1][n-1] To express the final result
2.2 Interpretation of structural meaning
In this question dp[i][j] It can be interpreted as :i Represents the row of the map ,j Representative column
2.3 Establishment of state transition equation
In this question :
Due to the limitation of the topic, we can only go down or to the right Move , So we follow 「 Current optional direction 」 Analyze :
Current location can only 「 Down 」 Move , That is to say dp[i][j] = dp[i-1][j]
Current location can only 「 To the right 」 Move , That is to say dp[i][j] = dp[i][j-1]
The current position can 「 Down 」 Can also 「 To the right 」 Move , That is to say dp[i][j] =dp[i][j-1] + dp[i-1][j]
Derivation process :
dp[i][j] It represents the arrival map grid[i][j] The number of points reaching the path , Then step up : How to get there grid[i][j] spot , There are only two ways : Enter this point from the front point down or to the right , So arrive at grid[i][j] The only way is to arrive dp[i][j-1] The way + arrive dp[i-1][j] The way , Of course, the difference between this problem and another problem is : Whether the last point can be moved left or right , From this we can deduce dp[i][j] State transfer equation of :dp[i][j] =dp[i][j-1] + dp[i-1][j], The equation of state does not change , But the conditions must be controlled :i>0&&j>0&&obstacleGrid[i-1][j]==0&&obstacleGrid[i][j-1]==0
3. Code display :
The code is as follows ( Example ):
class Solution {
public:
int uniquePathsWithObstacles(vector<vector<int>>& obstacleGrid) {
int n=obstacleGrid[0].size();
int m=obstacleGrid.size();
vector<vector<int>> dp(m, vector<int>(n));
dp[0][0]=1;
if(obstacleGrid[m-1][n-1]==1)
{
return 0;
}
for(int i=0;i<m;i++)
{
for(int j=0;j<n;j++)
{
if(i>0&&j>0&&obstacleGrid[i-1][j]==0&&obstacleGrid[i][j-1]==0)
{
dp[i][j]=dp[i-1][j]+dp[i][j-1];
}
else if(i>0&&obstacleGrid[i-1][j]==0)
{
dp[i][j]=dp[i-1][j];
}
else if(j>0&&obstacleGrid[i][j-1]==0){
dp[i][j]=dp[i][j-1];
}
}
}
return dp[m-1][n-1];
}
};
Two 、 Minimum path sum (Leecode 64 secondary )
1. Problem description
Given a... That contains a nonnegative integer m x n grid grid , Please find a path from the top left corner to the bottom right corner , Make the sum of the numbers on the path the smallest .
explain : You can only move down or right one step at a time
2. Problem analysis
In fact, dynamic problems only need to consider how to define the data structure 、 The meaning of structure 、 Establishment of state transition equation .
2.1 Define the data structure
The question is how many different paths , In this case, you should use dp[m-1][n-1] To express the final result
2.2 Interpretation of structural meaning
In this question dp[i][j] It can be interpreted as :i Represents the row of the map ,j Representative column
2.3 Establishment of state transition equation
In this question :
Due to the limitation of the topic, we can only go down or to the right Move , So we follow 「 Current optional direction 」 Analyze :
Current location can only 「 Down 」 Move , That is to say dp[i][j] = dp[i-1][j]
Current location can only 「 To the right 」 Move , That is to say dp[i][j] = dp[i][j-1]
The current position can 「 Down 」 Can also 「 To the right 」 Move , That is to say dp[i][j] =dp[i][j-1] + dp[i-1][j]
Derivation process :
dp[i][j] It represents the arrival map grid[i][j] The sum of the numbers of points reaching the path , Then step up : How to get there grid[i][j] spot , There are only two ways : Enter this point from the front point down or to the right , You'll get (dp[i][j-1] The sum of the numbers +grid[i][j])、(dp[i-1][j] The sum of the numbers of +grid[i][j]) Two ways , The result of the problem is to find the one with the smallest number , So just find the smallest one in these two ways , because dp[i][j-1]、dp[i-1][j] These two methods are the minimum value before , So the calculation is dp[i][j] Is the smallest . From this we can deduce dp[i][j] State transfer equation of :dp[i][j]=min(dp[i-1][j]+grid[i][j],dp[i][j-1]+grid[i][j])
3. Code display :
The code is as follows ( Example ):
class Solution {
public:
int minPathSum(vector<vector<int>>& grid) {
int n=grid[0].size();
int m=grid.size();
vector<vector<int>> dp(m, vector<int>(n));
dp[0][0]=grid[0][0];
for(int i=0;i<m;i++)
{
for(int j=0;j<n;j++)
{
if(i>0&&j>0)
{
dp[i][j]=min(dp[i-1][j]+grid[i][j],dp[i][j-1]+grid[i][j]);
}
else if(i>0)
{
dp[i][j]=dp[i-1][j]+grid[i][j];
}
else if(j>0){
dp[i][j]=dp[i][j-1]+grid[i][j];
}
}
}
return dp[m-1][n-1];
}
};
summary
In fact, dynamic problems only need to consider how to define the data structure 、 The meaning of structure 、 Establishment of state transition equation .
Most of the data structures are dp[i][j], It is difficult to define dp[i][j] The meaning of 、i The meaning of representation 、j The meaning of representation
State transition equation : In fact, it's about thinking about changes :dp[i][j] and dp[i-1][j]、dp[i][j-1] The relationship between , This needs to be analyzed according to the problem , Analyze through the results of the problem dp[i][j] How to get to the last step of dp[i][j], such as dp[i][j]–> arrive grid[i][j] The minimum sum of numbers ,dp[i-1][j]–> arrive grid[i-1][j] The minimum sum of numbers ,dp[i-1][j]–> arrive grid[i][j-1] The minimum sum of numbers , This is a recursive idea , You can't always think about how to find the smallest one step by step at the beginning , Instead, you just need to find out how to get from the last smallest to the next smallest , This is the idea of recursion .
边栏推荐
- LeetCode#204. Count prime
- UCORE lab5 user process management experiment report
- Accounting regulations and professional ethics [3]
- 编程到底难在哪里?
- Cost accounting [14]
- Research Report on market supply and demand and strategy of China's Medical Automation Industry
- Do you know the performance testing terms to be asked in the software testing interview?
- 学习记录:串口通信和遇到的错误解决方法
- Learning record: STM32F103 clock system overview working principle
- MATLAB实例:阶跃函数的两种表达方式
猜你喜欢
![[C language] twenty two steps to understand the function stack frame (pressing the stack, passing parameters, returning, bouncing the stack)](/img/3a/aadde60352c42199ba287a6997acfa.jpg)
[C language] twenty two steps to understand the function stack frame (pressing the stack, passing parameters, returning, bouncing the stack)
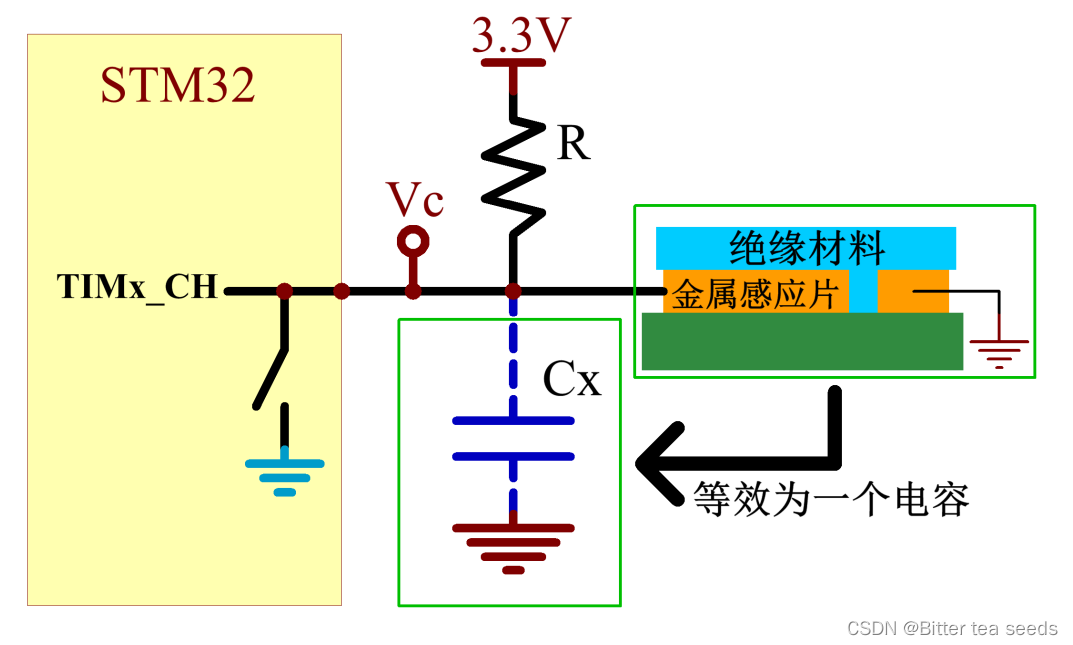
Learning record: Tim - capacitive key detection

Winter vacation daily question - maximum number of balloons
Es6---es6 content details
Learning record: use stm32f1 watchdog
ucore lab 6
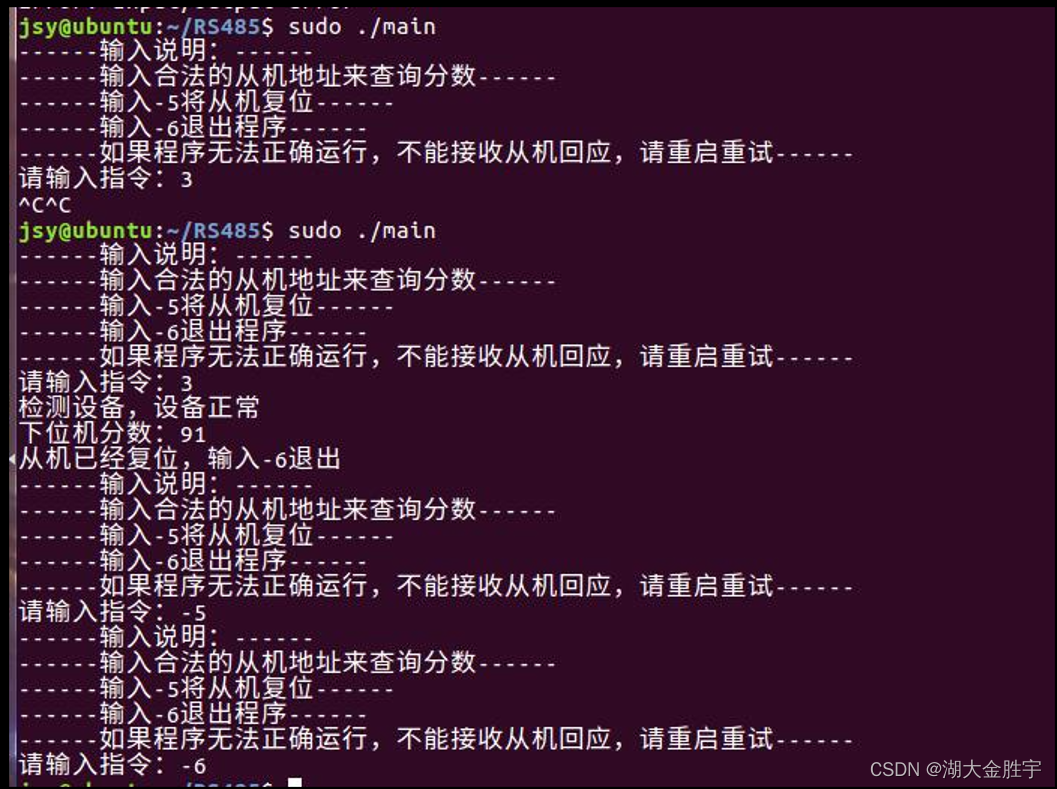
Scoring system based on 485 bus
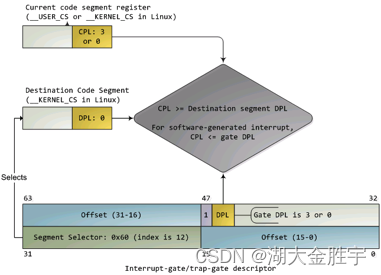
UCORE Lab 1 system software startup process

Leetcode notes - dynamic planning -day7
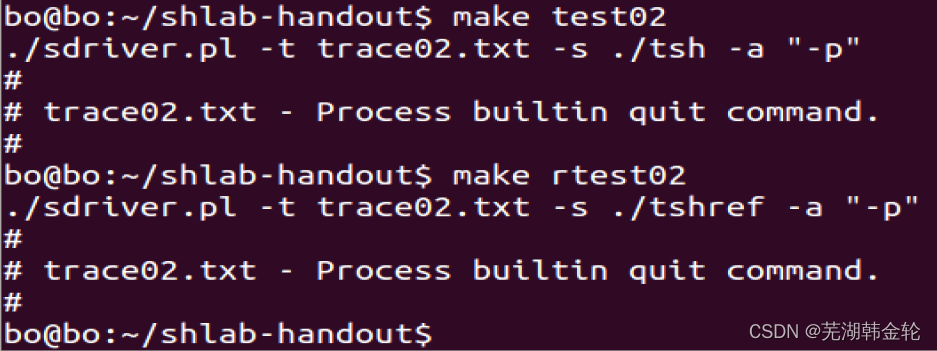
CSAPP shell lab experiment report
随机推荐
Visual analysis of data related to crawling cat's eye essays "sadness flows upstream into a river" | the most moving film of Guo Jingming's five years
cs零基础入门学习记录
China earth moving machinery market trend report, technical dynamic innovation and market forecast
力扣刷题记录
学习记录:TIM—基本定时器
How to write the bug report of software test?
Unpleasant error typeerror: cannot perform 'ROR_‘ with a dtyped [float64] array and scalar of type [bool]
Leetcode notes - dynamic planning -day7
学习记录:理解 SysTick系统定时器,编写延时函数
UCORE lab5 user process management experiment report
Learning record: USART serial communication
LeetCode#268. Missing numbers
Es6---es6 content details
TCP的三次握手与四次挥手
Research Report on market supply and demand and strategy of China's land incineration plant industry
What if software testing is too busy to study?
Learning record: Tim - capacitive key detection
Market trend report, technical innovation and market forecast of geosynthetic clay liner in China
Word macro operation: convert the automatic number in the document into editable text type
Brief introduction to libevent