当前位置:网站首页>The difference between monotonicity constraint and anti monotonicity constraint
The difference between monotonicity constraint and anti monotonicity constraint
2022-07-07 10:40:00 【Xminyang】
▚ 01 Monotone Constraints Monotonicity constraint
1.1 Definitions Definition
【Monotone Constraints | SpringerLink 】
A constraint C is monotone if and only if for all itemsets S and S′:
if S ⊇ S′ and S violates C, then S′ violates C.
1.2 Key Points The main points of
Monotone constraints possess the following property. If an itemset S violates a monotone constraint C, then any of its subsets also violates C. Equivalently, all supersets of an itemset satisfying a monotone constraint C also satisfy C (i.e., C is upward closed). By exploiting this property, monotone constraints can be used for reducing computation in frequent itemset mining with constraints. As frequent itemset mining with constraints aims to find frequent itemsets that satisfy the constraints, if an itemset S satisfies a monotone constraint C, no further constraint checking needs to be applied to any superset of S because all supersets of S are guaranteed to satisfy C. Examples of monotone constraints include min(S. Price) ≤ $30, which expresses that the minimum price of all items in an itemset S is at most $30. Note that, if the minimum price of all items in S is at most $30, adding more items to S would not increase its minimum price (i.e., supersets of S would also satisfy such a monotone constraint).

▚ 02 Anti-monotone Constraints Anti monotonicity constraint
2.1 Definitions Definition
【Anti-monotone Constraints | SpringerLink】
A constraint C is anti-monotone if and only if for all itemsets S and S′:
if S ⊇ S′and S satisfies C, then S′ satisfies C.
2.2 Key Points The main points of
Anti-monotone constraints possess the following nice property. If an itemset S satisfies an anti-monotone constraint C, then all of its subsets also satisfy C (i.e., C is downward closed). Equivalently, any superset of an itemset violating an anti-monotone constraint C also violates C. By exploiting this property, anti-monotone constraints can be used for pruning in frequent itemset mining with constraints. As frequent itemset mining with constraints aims to find itemsets that are frequent and satisfy the constraints, if an itemset violates an anti-monotone constraint C, all its supersets (which would also violate C) can be pruned away and their frequencies do not need to be counted. Examples of anti-monotone constraints include min(S. Price) ≥ $20 (which expresses that the minimum price of all items in an itemset S is at least $20) and the usual frequency constraint support(S) ≥ minsup (i.e., frequency(S) ≥ minsup). For the former, if the minimum price of all items in S is less than $20, adding more items to S would not increase its minimum price (i.e., supersets of S would not satisfy such an anti-monotone constraint). For the latter, it is widely used in frequent itemset mining, with or without constraints. It states that (i) all subsets of a frequent itemset are frequent and (ii) any superset of an infrequent itemset is also infrequent. This is also known as the Apriori property.

▚ 03 Explanation explain
hypothesis : We will S violates C As an event A,S′ violates C As an event B; be S satisfies C For events not A,then S′ satisfies C For events not B.
here , according to Monotone Constraints Definition knowledge (A → B), That is to say (not B → not A);
according to Anti-monotonicity Constraints Definition knowledge (not A → not B), That is to say (B → A);
because (A → B) It doesn't necessarily mean (B → A), So both (Monotone Constraints & Anti-monotonicity Constraints) The statement is different .

▚ 04 Example Example
For an example. Consider-
C1 = Sum of elements is greater than 5
C2 = Sum of elements is at most 5
U(universe) = Set of non-negative real numbers
In case of C1,
If S violates C1, then S’ obviously violates C1 as well (S being a superset of S’)
Eg. S = {1, 2}, S’ = {2}
Hence C1 is monotonic.
In case of C2,
If S satisfies C2, then S’ obviously satisfies C2 as well (S being a superset of S’)
Eg. S = {1, 2}, S’ = {2}
Hence C2 is anti-monotonic.

▚ 05 The constraints of data mining
Constraint-Based Mining — A General Picture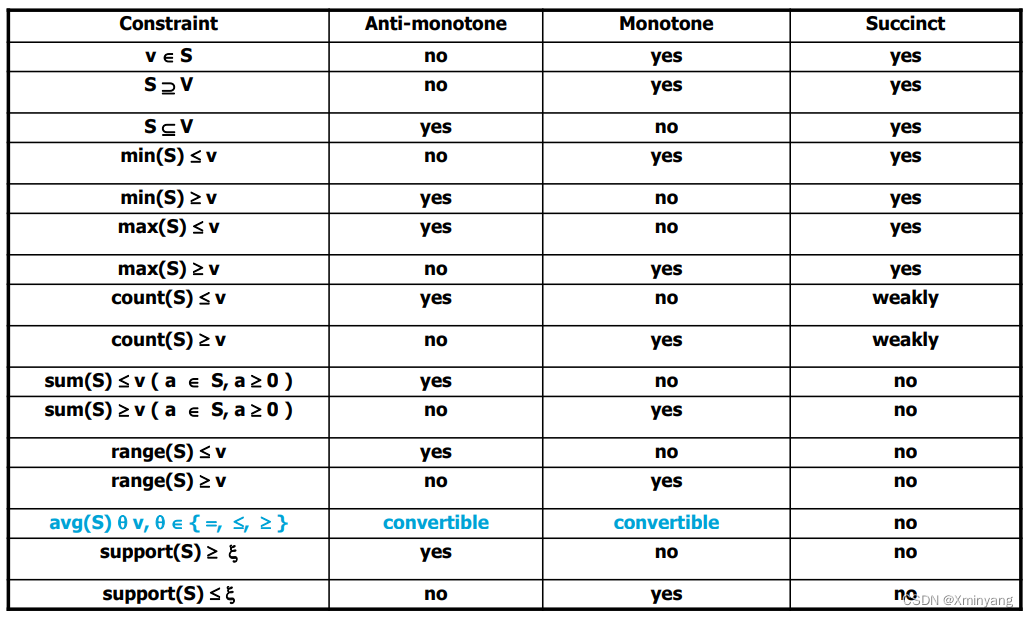

Reference article
边栏推荐
- IIC Basics
- 1324:【例6.6】整数区间
- 深入理解Apache Hudi异步索引机制
- BUUCTF---Reverse---reverse1
- 2022年7月10日“五心公益”活动通知+报名入口(二维码)
- Experience sharing of software designers preparing for exams
- Yarn的基础介绍以及job的提交流程
- MySQL insert data create trigger fill UUID field value
- How much review time does it usually take to take the intermediate soft exam?
- Adb 实用命令(网络包、日志、调优相关)
猜你喜欢
Some properties of leetcode139 Yang Hui triangle
Cluster task scheduling system lsf/sge/slurm/pbs based on HPC scenario
P2788 math 1 - addition and subtraction
高级软考(网络规划设计师)该如何备考?
原型与原型链

Pre knowledge reserve of TS type gymnastics to become an excellent TS gymnastics master
How to successfully pass the senior system architecture designer in the second half of the year?
ArrayList thread insecurity and Solutions
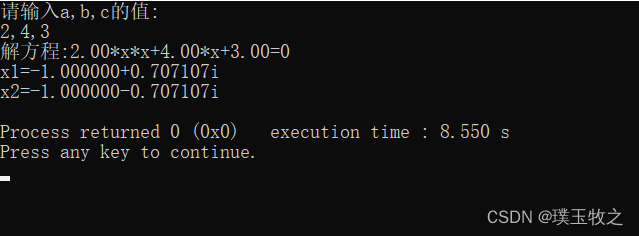
求方程ax^2+bx+c=0的根(C语言)
Applet jump to H5, configure business domain name experience tutorial
随机推荐
[detailed explanation of Huawei machine test] tall and short people queue up
软考一般什么时候出成绩呢?在线蹬?
1323:【例6.5】活动选择
P2788 数学1(math1)- 加减算式
MONAI版本更新到 0.9 啦,看看有什么新功能
2022年7月10日“五心公益”活动通知+报名入口(二维码)
Schnuka: machine vision positioning technology machine vision positioning principle
php \n 换行无法输出
那些易混淆的概念(三):function和class
Cluster task scheduling system lsf/sge/slurm/pbs based on HPC scenario
[STM32] solution to the problem that SWD cannot recognize devices after STM32 burning program
Some properties of leetcode139 Yang Hui triangle
施努卡:机器人视觉抓取工作原理 机器视觉抓取
How embedded engineers improve work efficiency
leetcode-304:二维区域和检索 - 矩阵不可变
[dai6] mirror image of JZ27 binary tree
Deeply analyze the main contents of erc-4907 agreement and think about the significance of this agreement to NFT market liquidity!
Gym installation pit records
使用Tansformer分割三维腹部多器官--UNETR实战
CAS mechanism