Four traversal methods of binary tree, as well as the creation of binary tree from middle order to post order, pre order to middle order, pre order to post order, and sequence [specially created for t

Blogger introduction ：

– I am a 了 all WeChat official account 【 The Milky way 】 Looking forward to your attention . In the future, let's cheer together ~

---

Preface

The three traversal methods here need not be introduced too much , It is believed that anyone who has learned data structure can easily use recursive method to traverse , The idea of non recursive method is also consistent .
According to the previous order and the middle order 、 The middle order and the last order 、 The preface and the following preface are written with reference to the solution to the question of force deduction , Only sequence traversal is to make it inconvenient to solve problems again, so we choose to solve problems locally , But local problem solving cannot be tested , Using the other three creation methods is too cumbersome , So I want to use sequence to create binary tree , The thinking is relatively simple for your reference , Problems can be discussed in time .

---

The former sequence traversal

Because it is a preorder traversal, the root node must be in front , Traversal order is （ root 、 Left 、 Right ）

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

//  The former sequence traversal 
func preorderTraversal(root *TreeNode) []int {
    
	nums := make([]int, 0)
	var dfs func(node *TreeNode)
	dfs = func(node *TreeNode) {
    
		if node != nil {
    
			nums = append(nums, node.Val) //  root 
			dfs(node.Left) //  Left 
			dfs(node.Right) //  Right 
		}
	}
	dfs(root)
	return nums
}

In the sequence traversal

Because it is a medium order traversal, the root node must be in the middle , Traversal order is （ Left 、 root 、 Right ）

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

//  In the sequence traversal 
func inorderTraversal(root *TreeNode) []int {
    
	nums := make([]int, 0)
	var dfs func(node *TreeNode)
	dfs = func(node *TreeNode) {
    
		if node != nil {
    
			dfs(node.Left)  //  Left 
			nums = append(nums, node.Val) //  root 
			dfs(node.Right) //  Right 
		}
	}
	dfs(root)
	return nums
}

After the sequence traversal

Because it is post order traversal, the root node must be behind , Traversal order is （ Left 、 Right 、 root ）

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

//  Subsequent traversal 
func postorderTraversal(root *TreeNode) []int {
    
	nums := make([]int, 0)
	var dfs func(node *TreeNode)
	dfs = func(node *TreeNode) {
    
		if node != nil {
    
			dfs(node.Left)
			dfs(node.Right)
			nums = append(nums, node.Val)
		}
	}
	dfs(root)
	return nums
}

Sequence traversal

Sequence traversal must be line by line , The idea is BFS（ Like a drop of water into the pool of ripples, layer by layer ）, Here, the queue is used to constantly store the next child as the next root node to traverse its child .

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

//  Sequence traversal 
func levelOrder(root *TreeNode) [][]int {
    
	ret := [][]int{
    }
	if root == nil {
    
		return ret
	}
	q := []*TreeNode{
    root}
	for i := 0; len(q) > 0; i++ {
    
		ret = append(ret, []int{
    })
		p := []*TreeNode{
    }
		for j := 0; j < len(q); j++ {
    
			node := q[j]
			ret[i] = append(ret[i], node.Val)
			if node.Left != nil {
    
				p = append(p, node.Left)
			}
			if node.Right != nil {
    
				p = append(p, node.Right)
			}
		}
		q = p
	}
	return ret
}

Sequence creation binary tree

It's the key moment , Take reference sequence traversal as the opposite thinking , First, split the one-dimensional array into nodes of each layer , Make a two-dimensional array , After that, according to traversing each child as the current root node, add its left and right children . This writing method is ugly and has low performance , Hope to buckle the bottom patiently . here -1 For null

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

//  Sequence creation binary tree 
func sequenceCreation(nums []int) *TreeNode {
    
	if len(nums) == 0 {
    
		return nil
	}
	if len(nums) == 1 {
    
		return &TreeNode{
    nums[0], nil, nil}
	}
	//  First, the array is divided into layers 
	sliNums := make([][]int, 0)
	count := 1
	for i := 0; i < len(nums); {
    
		cont := make([]int, 0)
		j := i
		for ; j < i+count; j++ {
    
			cont = append(cont, nums[j])
		}
		i = j
		sliNums = append(sliNums, cont)
		count *= 2
	}
	root := new(TreeNode)
	root.Val = sliNums[0][0]
	queue := []*TreeNode{
    root}
	count = 0
	for i := 1; i < len(sliNums); i++ {
    
		for j := 0; j < len(sliNums[i]); j += 2 {
    
			if sliNums[i][j] != -1 {
    
				queue[count].Left = &TreeNode{
    Val: sliNums[i][j]}
				queue = append(queue, queue[count].Left)
			} else {
    
				if queue[count] != nil {
    
					queue[count].Left = nil
					queue = append(queue, queue[count].Left)
				}
			}
			if sliNums[i][j+1] != -1 {
    
				queue[count].Right = &TreeNode{
    Val: sliNums[i][j+1]}
				queue = append(queue, queue[count].Right)
			} else {
    
				if queue[count] != nil {
    
					queue[count].Right = nil
					queue = append(queue, queue[count].Right)
				}
			}
			count++
		}
	}
	return root
}

Use cases

Here take the simple question of Li Kou as an example ：104. The maximum depth of a binary tree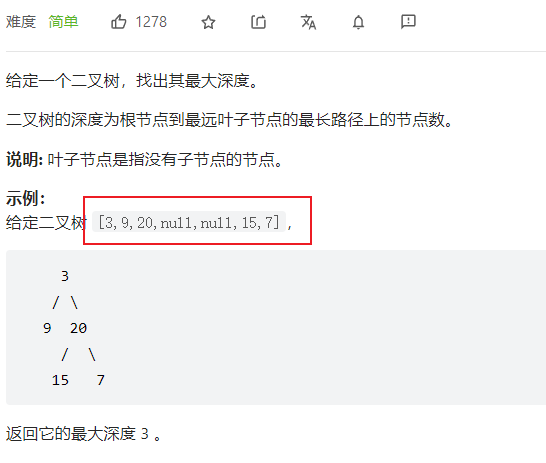

Code implementation

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

func max(a, b int) int {
    
	if a > b {
    
		return a
	}
	return b
}

func maxDepth(root *TreeNode) int {
    
	if root == nil {
    
		return 0
	}
	return max(maxDepth(root.Left), maxDepth(root.Right)) + 1
}

func main() {
    
	nums := []int{
    3, 9, 20, -1, -1, 15, 7}
	root := sequenceCreation(nums) //  This function is the above sequence traversal , Don't paste again here 
	fmt.Println(maxDepth(root))
}

test result

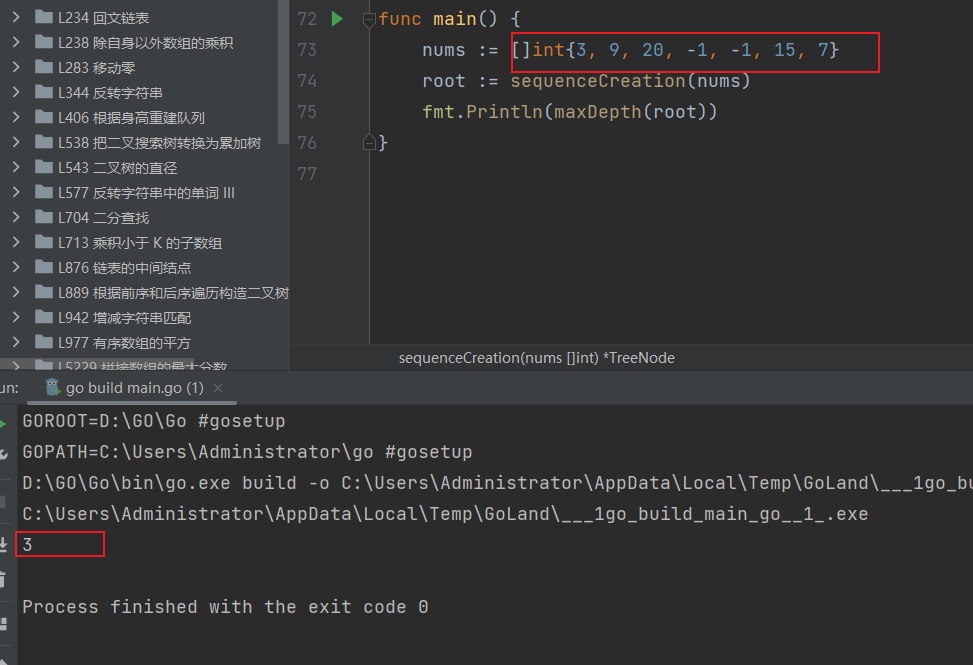
Results the correct

Create a binary tree in the previous order and the middle order

Here we refer to the solution of the problem of force deduction , Thinking is relatively simple ,preorder The beginning of the slice is the root node , And then inorder Slice and compare to find the left and right children , The creation can be completed by repeating the comparison downward .

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

//  Find the root node in the previous sequence , Find the left-right boundary in the middle order 
func pIBuildTree(preorder []int, inorder []int) *TreeNode {
    
	if len(preorder) == 0 {
    
		return nil
	}
	root := &TreeNode{
    preorder[0], nil, nil}
	i := 0
	for ; i < len(inorder); i++ {
    
		if inorder[i] == preorder[0] {
    
			break
		}
	}
	root.Left = pIBuildTree(preorder[1:len(inorder[:i])+1], inorder[:i])
	root.Right = pIBuildTree(preorder[len(inorder[:i])+1:], inorder[i+1:])
	return root
}

Create a binary tree in middle order and later order

It is basically consistent with the previous order and the middle order , But this time it's the comparison between middle order and post order

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

//  Create a binary tree from the middle order 
func iPBuildTree(inorder []int, postorder []int) *TreeNode {
    
	idxMap := map[int]int{
    }
	for i, v := range inorder {
    
		idxMap[v] = i
	}
	var build func(int, int) *TreeNode
	build = func(inorderLeft, inorderRight int) *TreeNode {
    
		//  No remaining nodes 
		if inorderLeft > inorderRight {
    
			return nil
		}

		//  The end element of post order traversal is the root node of the current subtree 
		val := postorder[len(postorder)-1]
		postorder = postorder[:len(postorder)-1]
		root := &TreeNode{
    Val: val}

		//  according to  val  The position traversed in the middle order , Divide the middle order traversal into left and right subtrees 
		//  Because we always take the elements from the end of the post order traversal , So we should traverse the right subtree first and then the left subtree 
		inorderRootIndex := idxMap[val]
		root.Right = build(inorderRootIndex+1, inorderRight)
		root.Left = build(inorderLeft, inorderRootIndex-1)
		return root
	}
	return build(0, len(inorder)-1)
}

Create a binary tree before and after

The result here may not be unique

type TreeNode struct {
    
	Val   int
	Left  *TreeNode
	Right *TreeNode
}

//  Build a binary tree according to the previous sequence 
func constructFromPrePost(preorder []int, postorder []int) *TreeNode {
    
	//  Recursion end condition 
	if len(preorder) == 0 {
    
		return nil
	}
	//  When there is only one element , Is a leaf node . This step 889 Do not omit 
	if len(preorder) == 1 {
    
		return &TreeNode{
    Val: preorder[0]}
	}

	//  The root node 
	root := &TreeNode{
    Val: preorder[0]}
	//  Find the position equal to the next node under the root node of the previous sequence in the subsequent sequence 
	for pos, node := range postorder {
    
		if node == preorder[1] {
    
			//  Build the left subtree 
			root.Left = constructFromPrePost(preorder[1:pos+2], postorder[:pos+1])
			//  Build the right subtree 
			root.Right = constructFromPrePost(preorder[pos+2:], postorder[pos+1:len(postorder)-1])
			break
		}
	}
	return root
}

---

Reference link ：

• Construct binary tree from middle order and post order traversal sequence ：https://leetcode.cn/problems/construct-binary-tree-from-inorder-and-postorder-traversal/solution/cong-zhong-xu-yu-hou-xu-bian-li-xu-lie-gou-zao-14/
• Construct binary tree according to preorder and postorder traversal ：https://leetcode.cn/problems/construct-binary-tree-from-preorder-and-postorder-traversal/solution/889-gen-ju-qian-xu-he-hou-xu-bian-li-gou-zw0i/
• Construction of binary trees from traversal sequences of preorder and middle order ：https://leetcode.cn/problems/construct-binary-tree-from-preorder-and-inorder-traversal/solution/cong-qian-xu-yu-zhong-xu-bian-li-xu-lie-gou-zao-9/

It's not easy to create , Give me some advice. ！
If you need to see a collection later ！
If you are interested in my article, please pay attention to ！
If there are questions , You can pay attention to the official account. 【 The Milky way 】 Click to contact me for private chat .

---