二叉树的遍历：递归法/ 迭代法/ 统一迭代法(强QAQ）

最近刷题刷到二叉树...算法面试的常客，数据结构基石...

见到许多厉害的解法，膜拜啊~

特此记录一下2022/3/24

力扣对应题目：144,145,94

一：递归法

一看就会，一写就废！~简单解法

前序遍历：

class Solution {
    public List<Integer> preorderTraversal(TreeNode root) {
        List<Integer> result = new ArrayList<Integer>();
        preorder(root, result);
        return result;
    }

    public void preorder(TreeNode root, List<Integer> result) {
        if (root == null) {
            return;
        }
        result.add(root.val);
        preorder(root.left, result);
        preorder(root.right, result);
    }
}

中序遍历：

class Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<>();
        inorder(root, res);
        return res;
    }

    void inorder(TreeNode root, List<Integer> list) {
        if (root == null) {
            return;
        }
        inorder(root.left, list);
        list.add(root.val);      // 注意这一句
        inorder(root.right, list);
    }
}

后序遍历：

class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
        List<Integer> res = new ArrayList<>();
        postorder(root, res);
        return res;
    }

    void postorder(TreeNode root, List<Integer> list) {
        if (root == null) {
            return;
        }
        postorder(root.left, list);
        postorder(root.right, list);
        list.add(root.val);     // 注意这一句
    }
}

二：迭代法

递归的实现就是：每一次递归调用都会把函数的局部变量、参数值和返回地址等压入调用栈中，然后递归返回的时候，从栈顶弹出上一次递归的各项参数，所以这就是递归为什么可以返回上一层位置的原因。so 可以用栈！

前序遍历：

// 前序遍历顺序：中-左-右，入栈顺序：中-右-左
class Solution {
    public List<Integer> preorderTraversal(TreeNode root) {
        List<Integer> result = new ArrayList<>();
        if (root == null){
            return result;
        }
        Stack<TreeNode> stack = new Stack<>();
        stack.push(root);
        while (!stack.isEmpty()){
            TreeNode node = stack.pop();
            result.add(node.val);
            if (node.right != null){
                stack.push(node.right);
            }
            if (node.left != null){
                stack.push(node.left);
            }
        }
        return result;
    }
}

中序遍历：

lass Solution {
    public List<Integer> inorderTraversal(TreeNode root) {
          //中序遍历：左中右  入栈顺序：左右
         List<Integer> result = new ArrayList<>();
        if (root == null){
            return result;
        }
        Stack<TreeNode> stack = new Stack<>();
        TreeNode cur = root;
        while (cur != null || !stack.isEmpty()){
           if (cur != null){
               stack.push(cur);//头结点入栈
               cur = cur.left;//一直遍历放左节点，到最左的叶子结点，然后到else取出最左的结点，然后放右结点。左边-中间-右边
           }else{
               cur = stack.pop();
               result.add(cur.val);
               cur = cur.right;
           }
        }
        return result;
    }
}

后续遍历：

class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
        //左右中 入栈顺序：中左右 出栈顺序：中右左 翻转：左右中
        List<Integer> res = new ArrayList<>();
        if(root == null){
            return res;
        }
        Stack<TreeNode> stack1 = new Stack<>();
        stack1.push(root);//头结点入栈
        while(!stack1.isEmpty()){
            TreeNode node = stack1.pop();
            res.add(node.val);
            if(node.left != null){
                stack1.push(node.left);
            }
            if(node.right != null){
                stack1.push(node.right);
            }
        }
        Collections.reverse(res);
        return res;
    }
}

三：统一迭代法

迭代法实现的先中后序，其实风格也不是那么统一，除了先序和后序，有关联，中序完全就是另一个风格了，一会用栈遍历，一会又用指针来遍历。

其实针对三种遍历方式，使用迭代法是可以写出统一风格的代码！

重头戏来了，接下来介绍一下统一写法。

用栈的话，无法同时解决访问节点（遍历节点）和处理节点（将元素放进结果集）不一致的情况。那我们就将访问的节点放入栈中，把要处理的节点也放入栈中但是要做标记。如何标记呢，就是要处理的节点放入栈之后，紧接着放入一个空指针作为标记。 这种方法也可以叫做标记法。

前序遍历：

class Solution {
    public List<Integer> preorderTraversal(TreeNode root) {
    //前序：中左右  入栈顺序：右左中
        List<Integer> res = new ArrayList<>();//LinkedList也可以
        Stack<TreeNode> st = new Stack<>();
        if(root != null) st.push(root);
        while(!st.empty()){
            TreeNode node = st.peek();
            if(node != null){
                st.pop();//把该节点弹出，避免重复操作
                if(node.right != null) st.push(node.right);
                if(node.left != null) st.push(node.left);
                st.push(node);
                st.push(null);//中间结点访问过，但还没有处理，加入空节点标记
            }else{
                st.pop();//弹出空节点
                node = st.pop();//取出被标记的结点
                res.add(node.val);//加入res中
            }
        }
        return res;
    }
}

中序遍历：

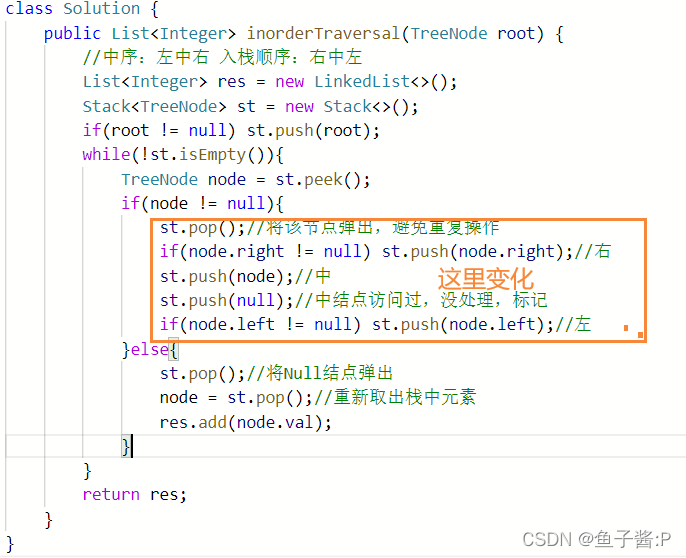
 后续遍历：

class Solution {
    public List<Integer> postorderTraversal(TreeNode root) {
        //后序：左右中 入栈顺序：中右左
        List<Integer> res = new LinkedList<>();
        Stack<TreeNode> st = new Stack<>();
        if(root != null ) st.push(root);
        while(!st.empty()){
            TreeNode node = st.peek();
            if(node != null){
                st.pop();//弹出该节点，避免重复
                st.push(node);
                st.push(null);//中方结点访问过，没处理，标记
                if(node.right != null) st.push(node.right);
                if(node.left != null) st.push(node.left);
            }else{
                st.pop();//把null结点弹出
                node = st.pop();
                res.add(node.val);
            }
        }
        return res;
    }
}