当前位置:网站首页>Key review route of probability theory and mathematical statistics examination
Key review route of probability theory and mathematical statistics examination
2022-07-05 04:23:00 【IOT classmate Huang】
The key review route of probability theory and mathematical statistics exam
List of articles
Preface
Hope to pass a simple route , Achieve accurate and efficient preparation for tomorrow's exam . Don't talk much , To rush !
The content is divided into two parts: probability theory and mathematical statistics , The middle series is the law of large numbers and central limit theorem in Chapter 5 .
MindMap
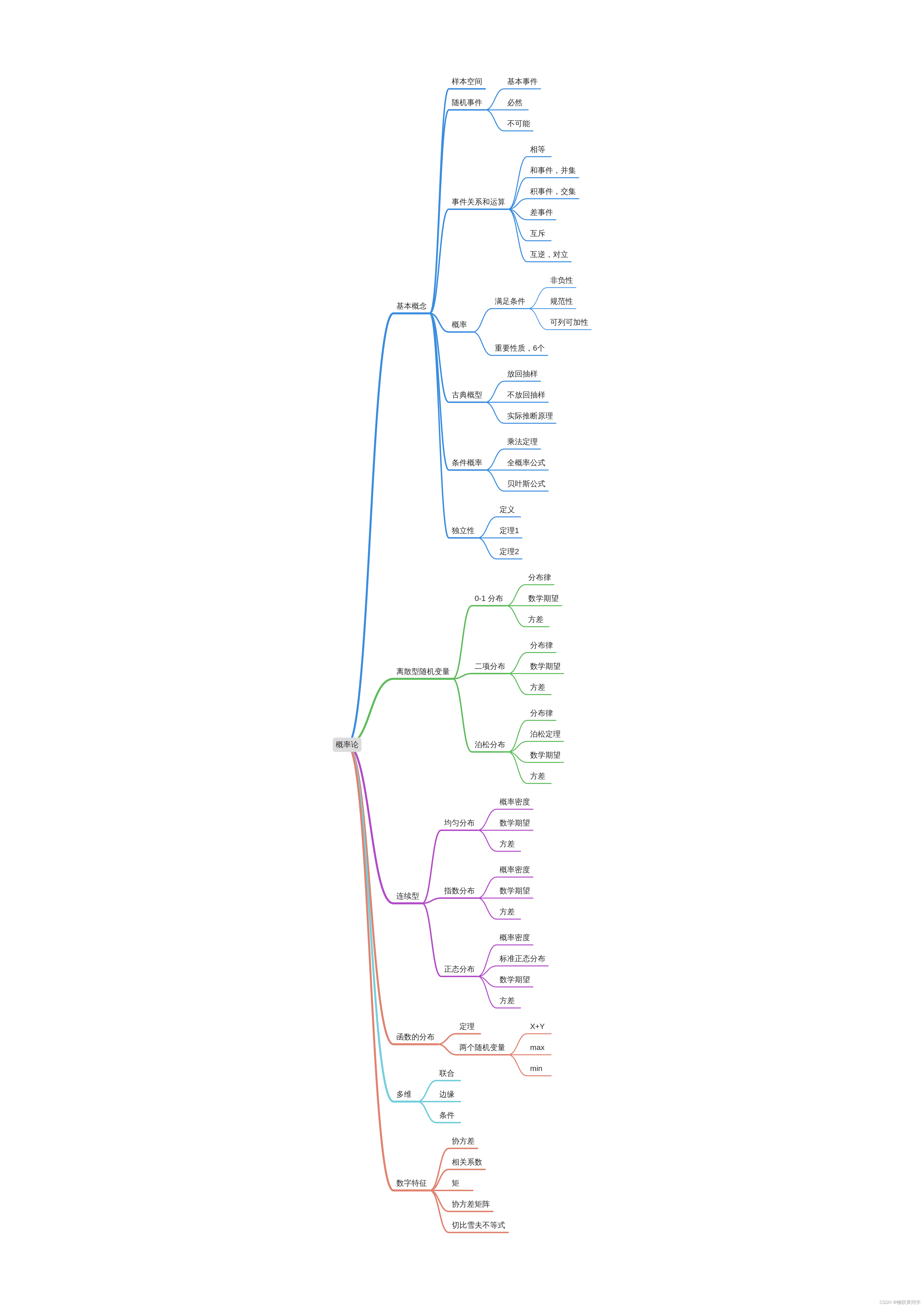
Probability theory part
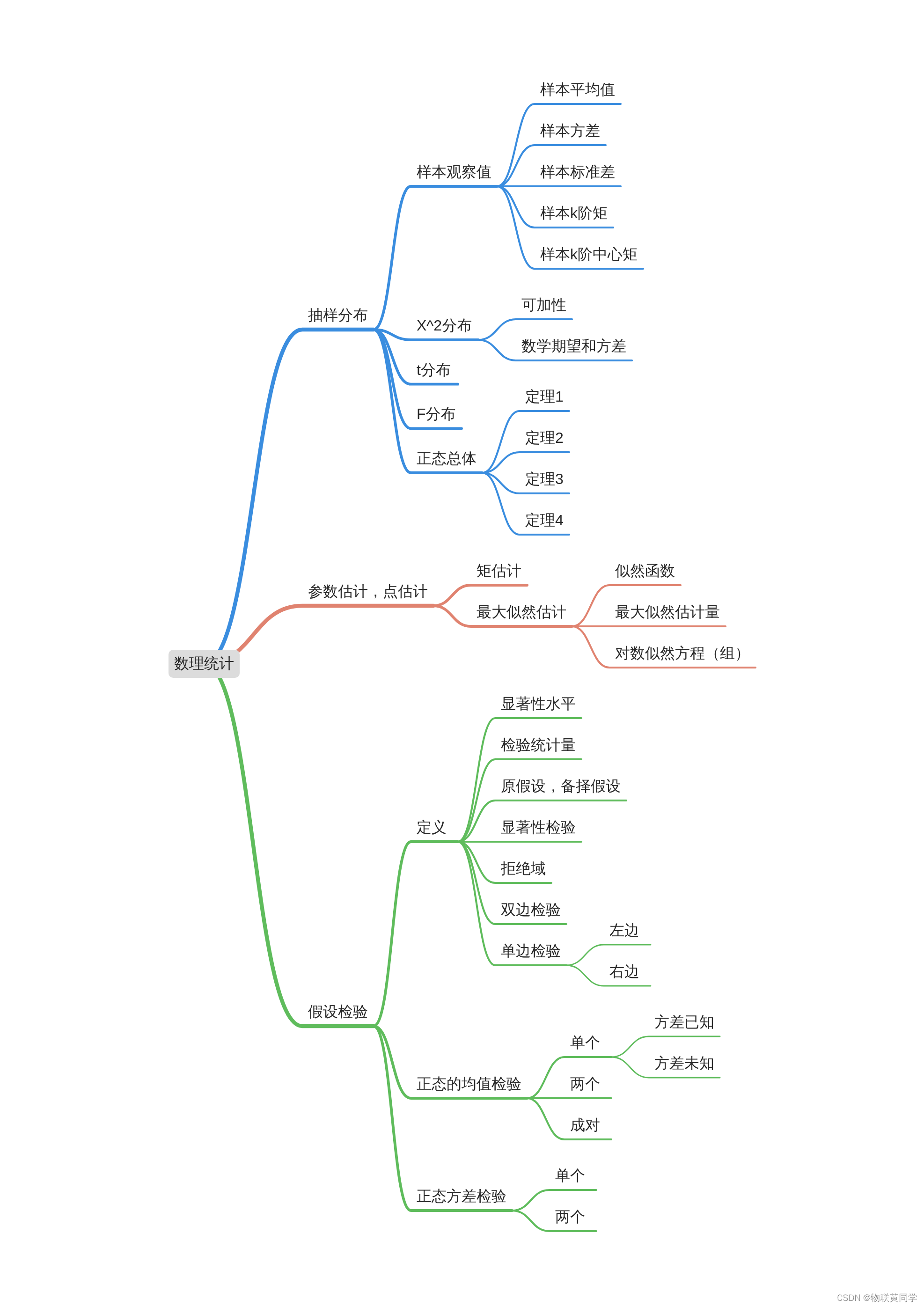
Mathematical statistics
probability theory
Basic concepts
The content of this part , My suggestion is to look directly at my previous blog, Or reading books and other online classes ppt And so on. .
I won't enumerate the distribution function of random variables , You can deduce it directly through the distribution law or probability density
discrete
0-1 Distribution
X~b§
Distribution law
P { X = k } = p k ( 1 − p ) 1 − k , k = 1 , 0 P\{X=k \} = p^k(1-p)^{1-k}, \qquad k = 1, 0 P{ X=k}=pk(1−p)1−k,k=1,0
| X | 0 | 1 |
|---|---|---|
| p_k | 1-p | p |
Mathematical expectation
E ( X ) = p E(X) = p E(X)=p
variance
D ( X ) = ( 1 − p ) ⋅ p D(X) = (1-p)\cdot p D(X)=(1−p)⋅p
The binomial distribution
X~b(n, p)
Distribution law
P { X = k } = p k ( 1 − p ) 1 − k P\{X=k \} = p^k(1-p)^{1-k} P{ X=k}=pk(1−p)1−k
Mathematical expectation
E ( X ) = n p E(X) = np E(X)=np
variance
D ( X ) = n ( 1 − p ) ⋅ p D(X) = n(1-p)\cdot p D(X)=n(1−p)⋅p
Poisson distribution
X~π(λ)
Distribution law
P { X = k } = λ k e − λ k ! , k = 0 , 1 , 2... P\{X=k \} = \frac{\lambda^ke^{-\lambda}}{k!}, \qquad k=0,1,2... P{ X=k}=k!λke−λ,k=0,1,2...
Poisson's theorem
Is to use Poisson to approximate binomial ,np=λ
lim n → ∞ C n k ( 1 − p n ) n − k = λ k e − λ k ! \lim_{n\rightarrow \infty}{C_n^k(1-p_n)^{n-k}} = \frac{\lambda^ke^{-\lambda}}{k!} n→∞limCnk(1−pn)n−k=k!λke−λ
Mathematical expectation
E ( X ) = λ E(X) = \lambda E(X)=λ
variance
D ( X ) = λ D(X) = \lambda D(X)=λ
Continuous type
Uniform distribution
X~U(a, b)
Probability density
KaTeX parse error: No such environment: align at position 26: …eft \{ \begin{̲a̲l̲i̲g̲n̲}̲ &\frac{1}{b…
expect
E ( X ) = a + b 2 E(X) = \frac {a+b}{2} E(X)=2a+b
variance
D ( X ) = ( b − a ) 2 12 D(X) = \frac{(b-a)^2}{12} D(X)=12(b−a)2
An index distribution
X~E(θ)
Probability density
KaTeX parse error: No such environment: align at position 26: …eft \{ \begin{̲a̲l̲i̲g̲n̲}̲ &\frac{1}{\…
expect
E ( X ) = θ E(X) = \theta E(X)=θ
variance
D ( X ) = θ 2 D(X) = \theta^2 D(X)=θ2
Normal distribution
X~N(μ, σ)
Probability density
f ( x ) = 1 2 π σ e − ( x − u ) 2 2 σ 2 , − ∞ < x < ∞ f(x) = \frac{1}{\sqrt{2\pi}\sigma}e^{-\frac{(x-u)^2}{2\sigma^2}}, \qquad -\infty < x < \infty f(x)=2πσ1e−2σ2(x−u)2,−∞<x<∞
Standard normal distribution
X ∼ N ( 0 , 1 2 ) φ ( x ) = 1 2 π e − x 2 / 2 X\sim N(0, 1^2)\\ \varphi(x) = \frac{1}{\sqrt{2\pi}}e^{-x^2/2} X∼N(0,12)φ(x)=2π1e−x2/2
Expectation and variance , In general, as long as it is converted into a standard normal distribution , Then it can be solved with the variance and expectation of the standard normal distribution .
expect
E ( x ) = μ E(x) = \mu E(x)=μ
variance
D ( X ) = σ 2 D(X) = \sigma^2 D(X)=σ2
In addition to these, there are actually random variable functions in probability theory , Multi dimensional edges and conditions and combinations , There are also covariance and moments in Chapter 4 . But I won't mention these contents , If necessary, you can see blog Or textbooks .
mathematical statistics
It's swinging , Look at this directly . I'm going back to bed .
边栏推荐
- Uni app change the default component style
- Convert Boolean to integer value PHP - Convert Boolean to integer value PHP
- 可观测|时序数据降采样在Prometheus实践复盘
- 【虚幻引擎UE】实现测绘三脚架展开动画制作
- Rome chain analysis
- A应用唤醒B应该快速方法
- Longyuan war "epidemic" 2021 network security competition web easyjaba
- Threejs rendering obj+mtl model source code, 3D factory model
- Common features of ES6
- Study notes 7
猜你喜欢
Sword finger offer 04 Search in two-dimensional array
蛇形矩阵
What is the reason why the webrtc protocol video cannot be played on the easycvr platform?

SPI read / write flash principle + complete code
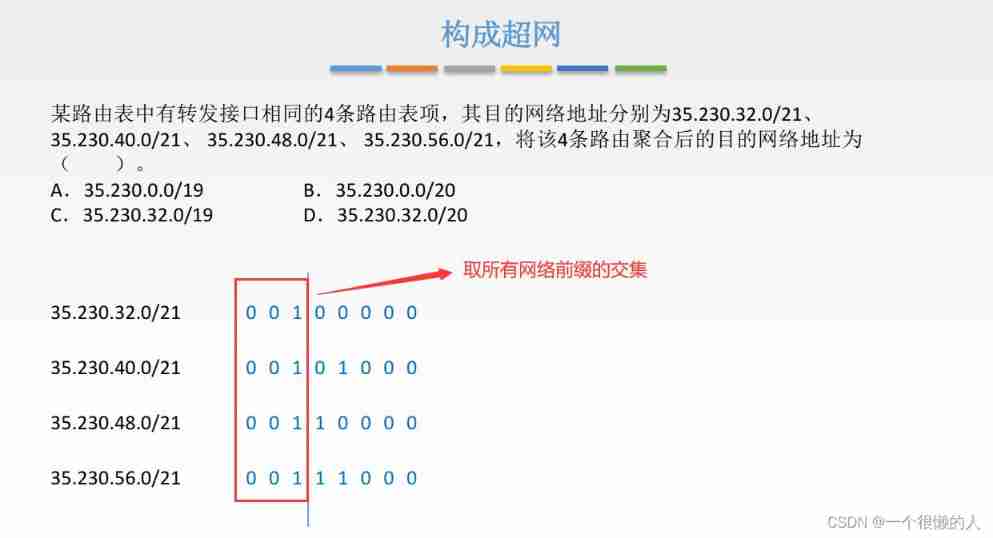
Network layer - forwarding (IP, ARP, DCHP, ICMP, network layer addressing, network address translation)
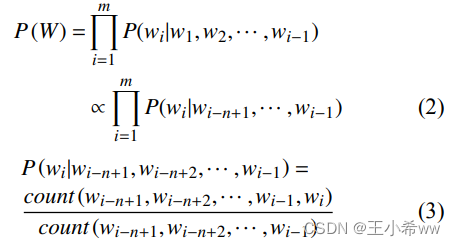
自动语音识别(ASR)研究综述

File upload bypass summary (upload labs 21 customs clearance tutorial attached)
Threejs realizes sky box, panoramic scene, ground grass

Rust blockchain development - signature encryption and private key public key
函数(基本:参数,返回值)
随机推荐
A real day for Beijing programmers!!!!!
Introduction to RT thread kernel (5) -- memory management
All in one 1413: determine base
File upload bypass summary (upload labs 21 customs clearance tutorial attached)
这是一个不确定的时代
Scheduling system of kubernetes cluster
蛇形矩阵
provide/inject
指针函数(基础)
【FineBI】使用FineBI制作自定义地图过程
根据入栈顺序判断出栈顺序是否合理
Learning notes 8
函數(易錯)
OWASP top 10 vulnerability Guide (2021)
Sword finger offer 04 Search in two-dimensional array
Longyuan war "epidemic" 2021 network security competition web easyjaba
Threejs realizes rain, snow, overcast, sunny, flame
Serpentine matrix
Why can't all browsers on my computer open web pages
程序员应该怎么学数学