概率论与数理统计考试重点复习路线

前言

希望能够通过一份简单的路线，实现精准高效的备战明天的考试。话不多说，冲冲冲！

内容分为概率论与数理统计两个部分，中间的串联是第五章的大数定律和中心极限定理。

MindMap

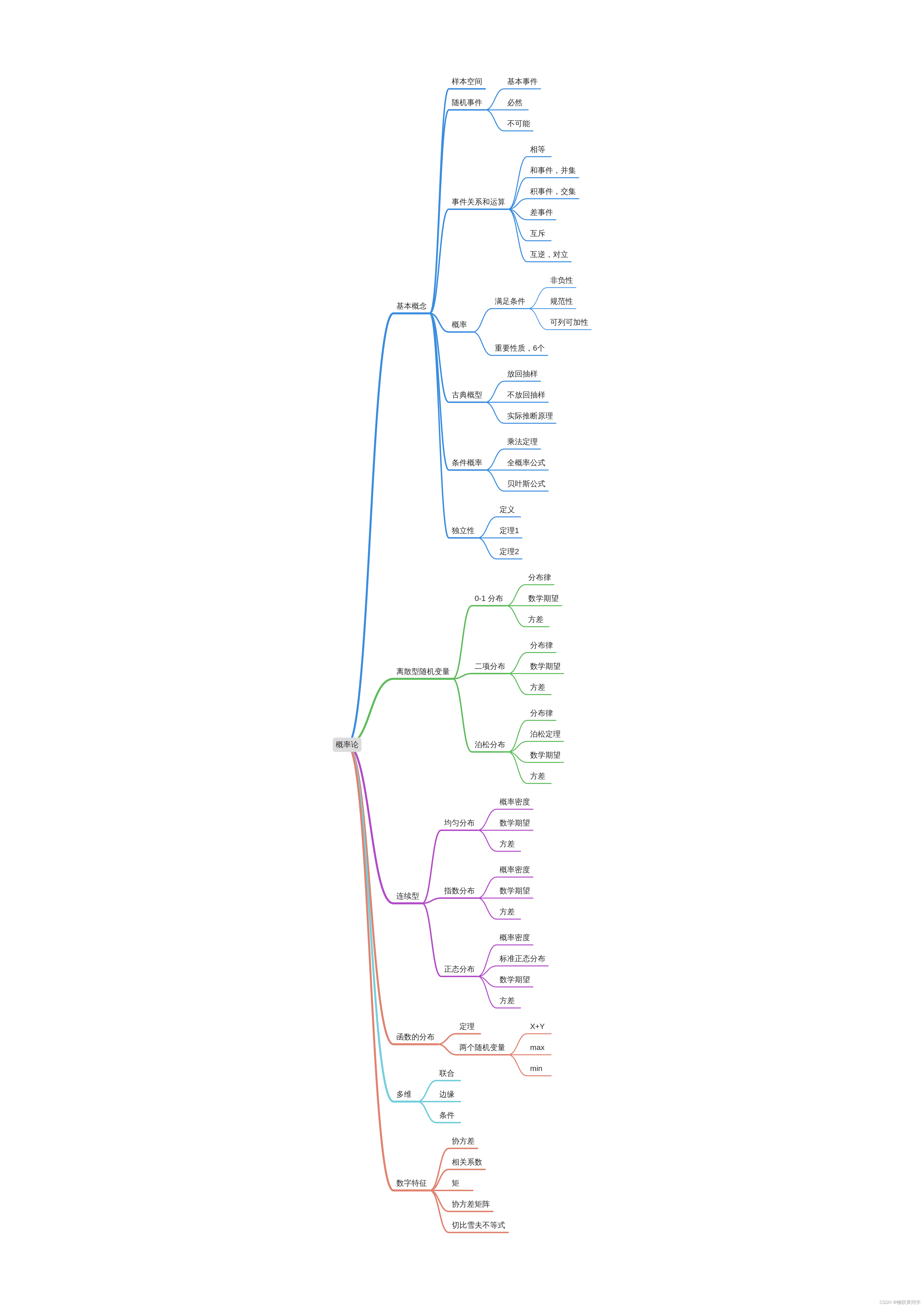
概率论部分

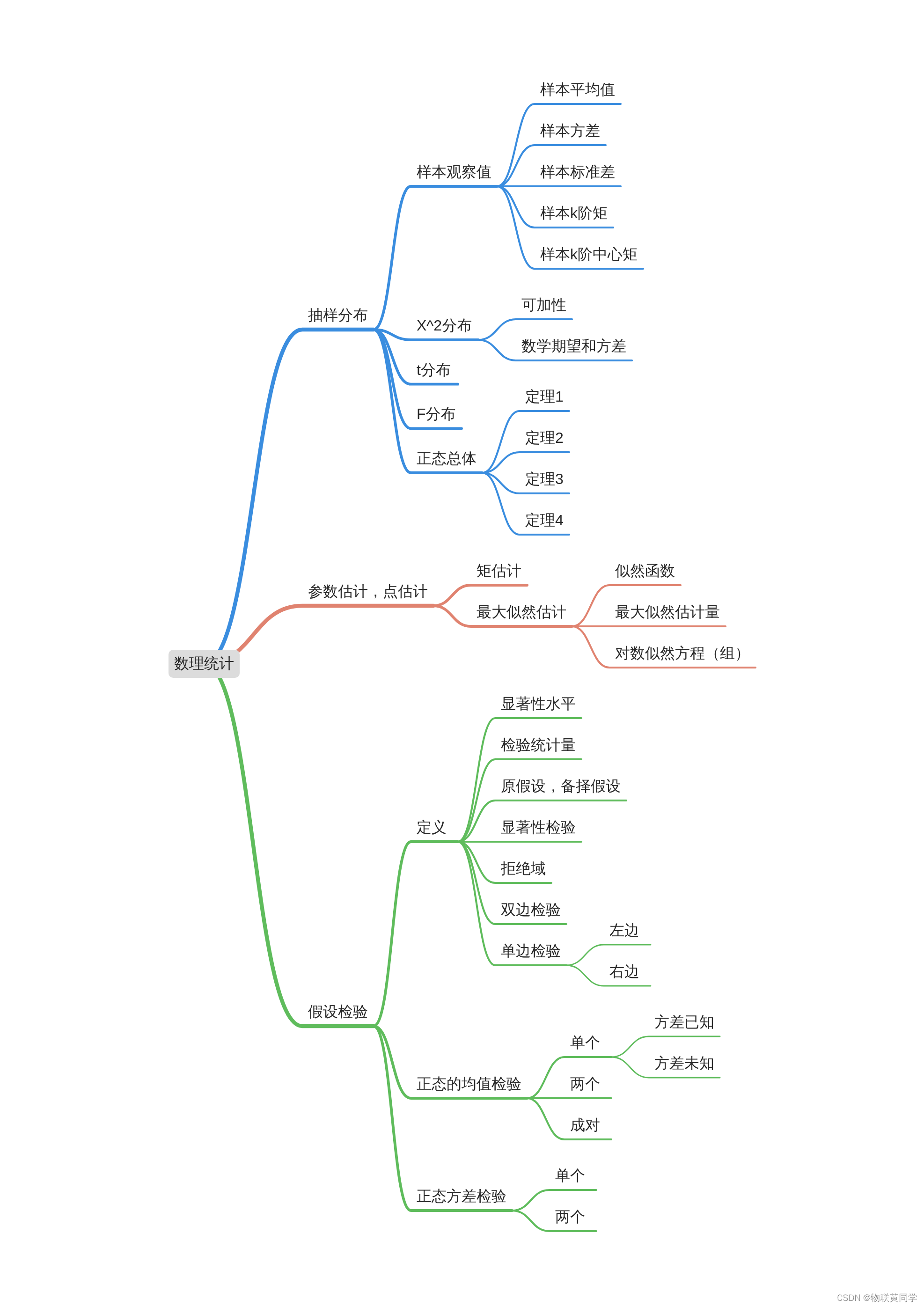
数理统计部分

概率论

基本概念

这个部分的内容，我的建议是直接看我之前的blog，或者看书以及其他网课ppt之类的。

关于随机变量的分布函数我不去列举，大家可以直接通过分布律或者概率密度推导

离散型

0-1 分布

X~b§

分布律

$$P\{X=k\}=p^k(1-p{)}^{1-k},k=1,0$$

X	0	1
p_k	1-p	p

数学期望

$$E(X)=p$$

方差

$$D(X)=(1-p)\cdot p$$

二项分布

X~b(n, p)

分布律

$$P\{X=k\}=p^k(1-p{)}^{1-k}$$

数学期望

$$E(X)=np$$

方差

$$D(X)=n(1-p)\cdot p$$

泊松分布

X~π(λ)

分布律

$$P\{X=k\}=\frac{{\lambda }^k{e}^{-\lambda }}{k!},k=0,1,2...$$

泊松定理

就是用泊松去逼近二项，np=λ
$$\underset{n\to \infty }{\lim }{C}_n^k(1-p_n{)}^{n-k}=\frac{{\lambda }^k{e}^{-\lambda }}{k!}$$

数学期望

$$E(X)=\lambda$$

方差

$$D(X)=\lambda$$

连续型

均匀分布

X~U(a, b)

概率密度

KaTeX parse error: No such environment: align at position 26: …eft \{ \begin{̲a̲l̲i̲g̲n̲}̲ &\frac{1}{b…

期望

$$E(X)=\frac{a+b}{2}$$

方差

$$D(X)=\frac{(b-a{)}^2}{12}$$

指数分布

X~E(θ)

概率密度

KaTeX parse error: No such environment: align at position 26: …eft \{ \begin{̲a̲l̲i̲g̲n̲}̲ &\frac{1}{\…

期望

$$E(X)=\theta$$

方差

$$D(X)={\theta }^2$$

正态分布

X~N(μ, σ)

概率密度

$$f(x)=\frac{1}{\sqrt{2\pi }\sigma }{e}^{-\frac{(x-u{)}^2}{2{\sigma }^2}},-\infty <x<\infty$$

标准正态分布

$$\begin{gathered} X\sim N(0,1^2) \\ \phi (x)=\frac{1}{\sqrt{2\pi }}{e}^{-x^2/2} \end{gathered}$$

期望和方差，一般情况下只要先化成标准正态分布，然后用标准的正态分布的方差和期望求解即可。

期望

$$E(x)=\mu$$

方差

$$D(X)={\sigma }^2$$

概率论部分的除了这些其实还有像随机变量函数，多维的边缘和条件以及联合，还有第四章的协方差和矩。但是这些内容我就不提了，有需要的可以看blog或者课本。

数理统计

开摆了，这个直接看吧。我要回去睡觉了。